Beer F.P., Johnston E.R., DeWolf J.T., Mazurek D.F. Mechanics of Materials
Подождите немного. Документ загружается.


Apago PDF Enhancer
or, since y 5 R 2 r,
E ¢
u
u
#
1R 2 r2
2
r
dA 5 M
Expanding the square in the integrand, we obtain after reductions
E ¢
u
u
cR
2
#
dA
r
2 2RA 1
#
r dA d5 M
Recalling Eqs. (4.66) and (4.67), we note that the first term in the
brackets is equal to RA, while the last term is equal to
rA. We have,
therefore,
E ¢
u
u
1RA 2 2RA 1 rA25 M
and, solving for E Duyu,
E ¢
u
u
5
M
A
1
r 2 R
2
(4.68)
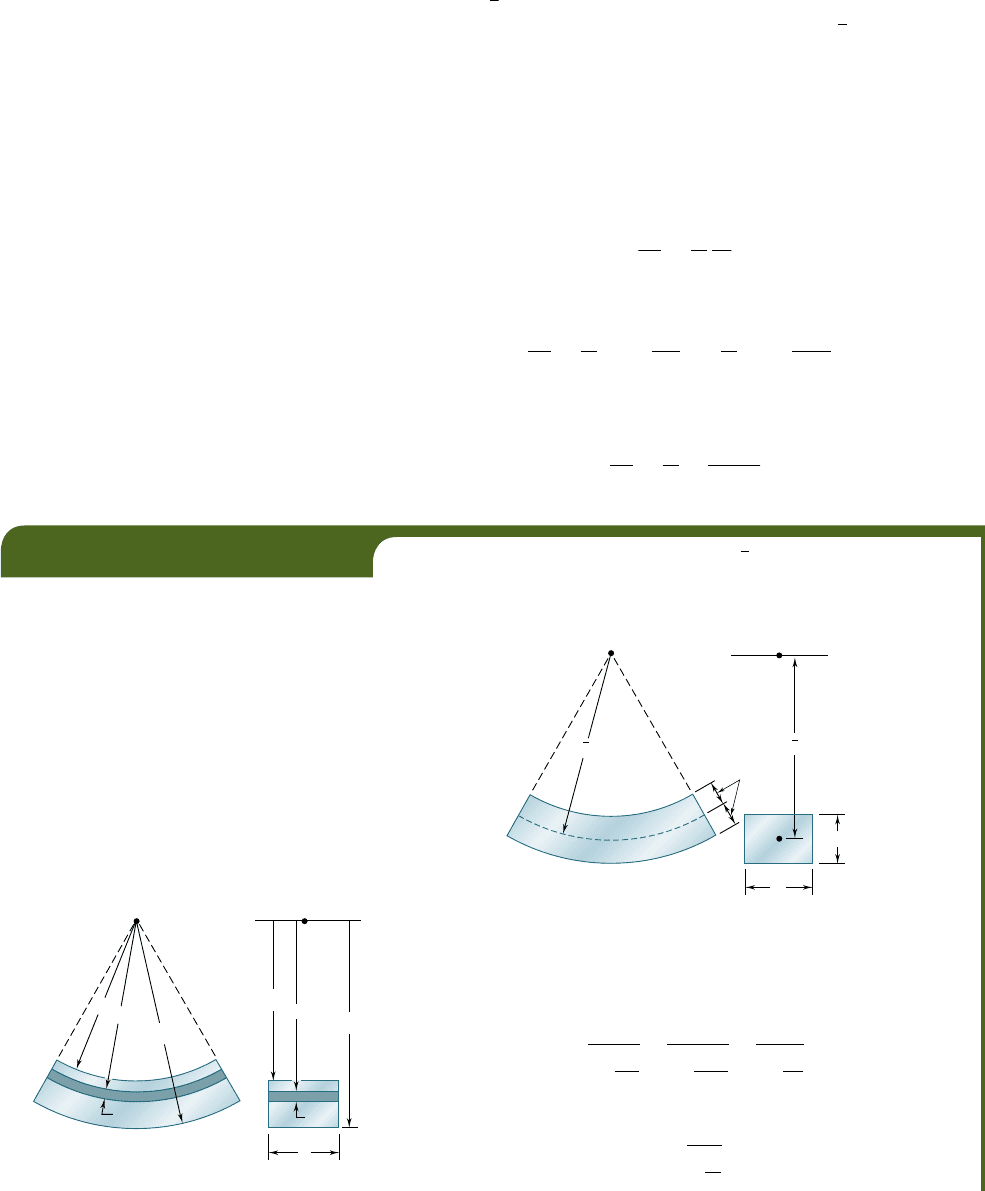
Referring to Fig. 4.70, we note that Du . 0 for M . 0. It follows
that
r 2 R . 0, or R , r, regardless of the shape of the section.
Thus, the neutral axis of a transverse section is always located between
the centroid of the section and the center of curvature of the mem-
ber (Fig. 4.72). Setting
r 2 R 5 e, we write Eq. (4.68) in the form
E ¢
u
u
5
M
Ae
(4.69)
Substituting now for E Duyu from (4.69) into Eqs. (4.64) and (4.65),
we obtain the following alternative expressions for the normal stress
s
x
in a curved beam:
s
x
52
M
y
Ae1R 2 y2
(4.70)
and
s
x
5
M
1
r 2 R
2
Aer
(4.71)
297
r
1
r
2
b
R
h
h
r
2
C
c
C
Rectangle
r
1
ln
r
1
r
2
b
R R
1
h
h
r
2
C
r
1
r
2
h
C
TriangleCircle
h
r
2
(r r
2
c
2
)
r
1
2
1
2
1
ln
R
h
2
(b
1
b
2
)
h(b
1
b
2
)(b
1
r
2
b
2
r
1
)
Trapezoid
r
2
r
1
2
1
ln
b
1
b
2
r
Fig. 4.73 Radius of neutral surface for various cross-sectional shapes.
bee80288_ch04_220-313.indd Page 297 11/11/10 3:03:19 PM user-f499bee80288_ch04_220-313.indd Page 297 11/11/10 3:03:19 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04
Apago PDF Enhancer
298
Pure Bending
We should note that the parameter e in the previous equations
is a small quantity obtained by subtracting two lengths of comparable
size, R and
r. In order to determine s
x
with a reasonable degree of
accuracy, it is therefore necessary to compute R and
r very accurately,
particularly when both of these quantities are large, i.e., when the
curvature of the member is small. However, as we indicated earlier,
it is possible in such a case to obtain a good approximation for s
x
by
using the formula s
x
5 2MyyI developed for straight members.
Let us now determine the change in curvature of the neutral sur-
face caused by the bending moment M. Solving Eq. (4.59) for the cur-
vature 1yR9 of the neutral surface in the deformed member, we write
1
R¿
5
1
R
u¿
u
or, setting u9 5 u 1 Du and recalling Eq. (4.69),
1
R¿
5
1
R
a
1 1
¢u
u
b
5
1
R
a
1 1
M
EAe
b
from which it follows that the change in curvature of the neutral
surface is
1
R¿
2
1
R
5
M
EAeR
(4.72)
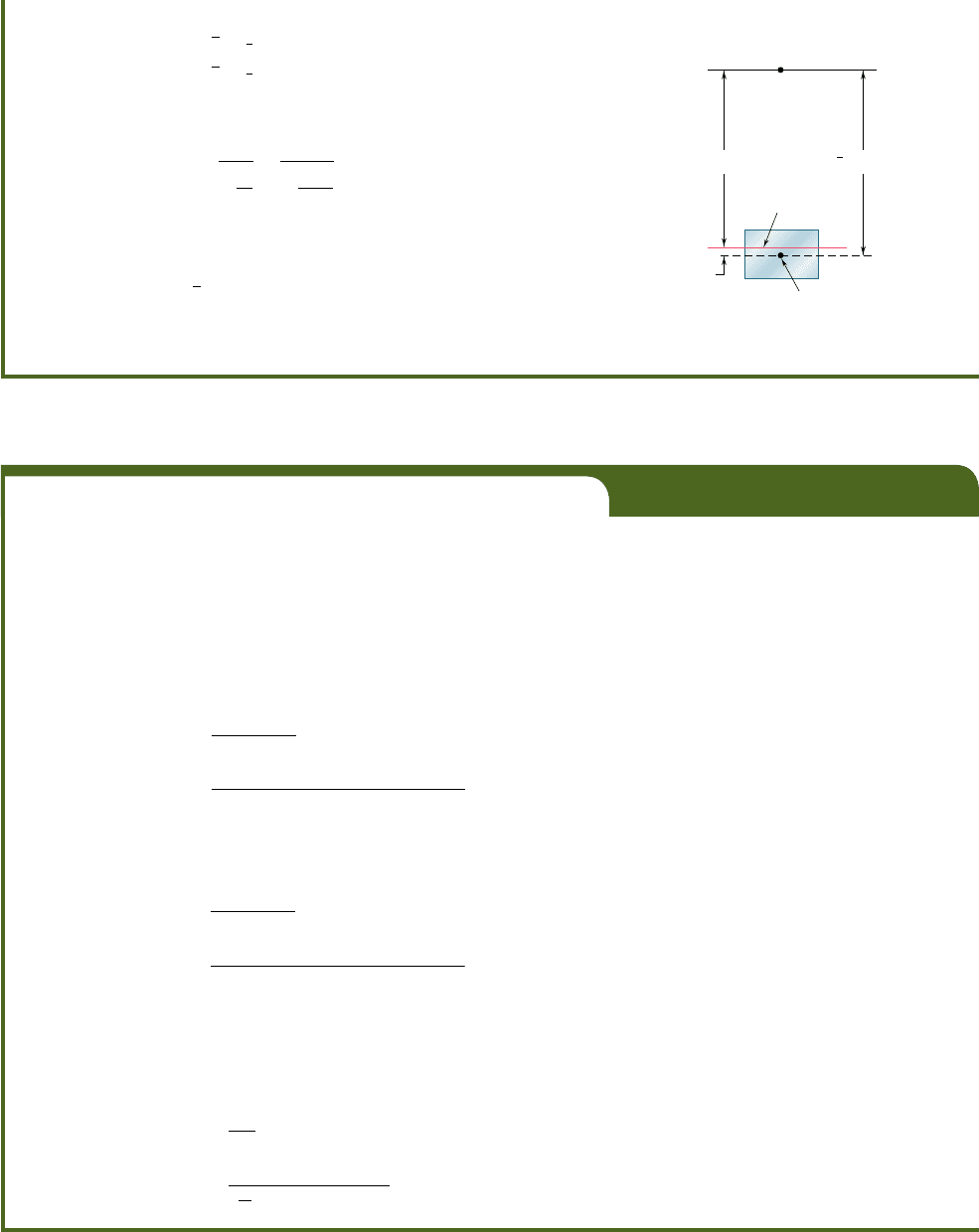
A curved rectangular bar has a mean radius r 5 6 in
.
and a cross section
of width b 5 2.5 in. and depth h 5 1.5 in. (Fig. 4.74). Determine the
distance e between the centroid and the neutral axis of the cross section.
EXAMPLE 4.10
h
b
h/2
C
C
r
r
Fig. 4.74
We first derive the expression for the radius R of the neutral sur-
face. Denoting by r
1
and r
2
, respectively, the inner and outer radius of
the bar (Fig. 4.75), we use Eq. (4.66) and write
R 5
A
#
r
2
r
1
dA
r
5
bh
#
r
2
r
1
b dr
r
5
h
#
r
2
r
1
dr
r
R 5
h
ln
r
2
r
1
(4.73)
r
2
r
2
b
dr
dr
r
1
r
1
r
CC
r
Fig. 4.75
bee80288_ch04_220-313.indd Page 298 10/26/10 4:34:31 PM user-f499bee80288_ch04_220-313.indd Page 298 10/26/10 4:34:31 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles
Apago PDF Enhancer
For the given data, we have
r
1
5 r 2
1
2
h 5 6 2 0.75 5 5.25 in.
r
2
5 r 1
1
2
h 5 6 1 0.75 5 6.75 in.
Substituting for h, r
1
, and r
2
into Eq. (4.73), we have
R 5
h
ln
r
2
r
1
5
1.5 in.
ln
6.75
5.25
5 5.9686 in.
The distance between the centroid and the neutral axis of the cross sec-
tion (Fig. 4.76) is thus
e
5 r 2 R 5 6 2 5
.
9686 5 0
.
0314 in
.
We note that it was necessary to calculate R with five significant figures
in order to obtain e with the usual degree of accuracy.
r 6 in.
C
R 5.9686 in.
e 0.0314 in.
Neutral axis
Centroid
Fig. 4.76
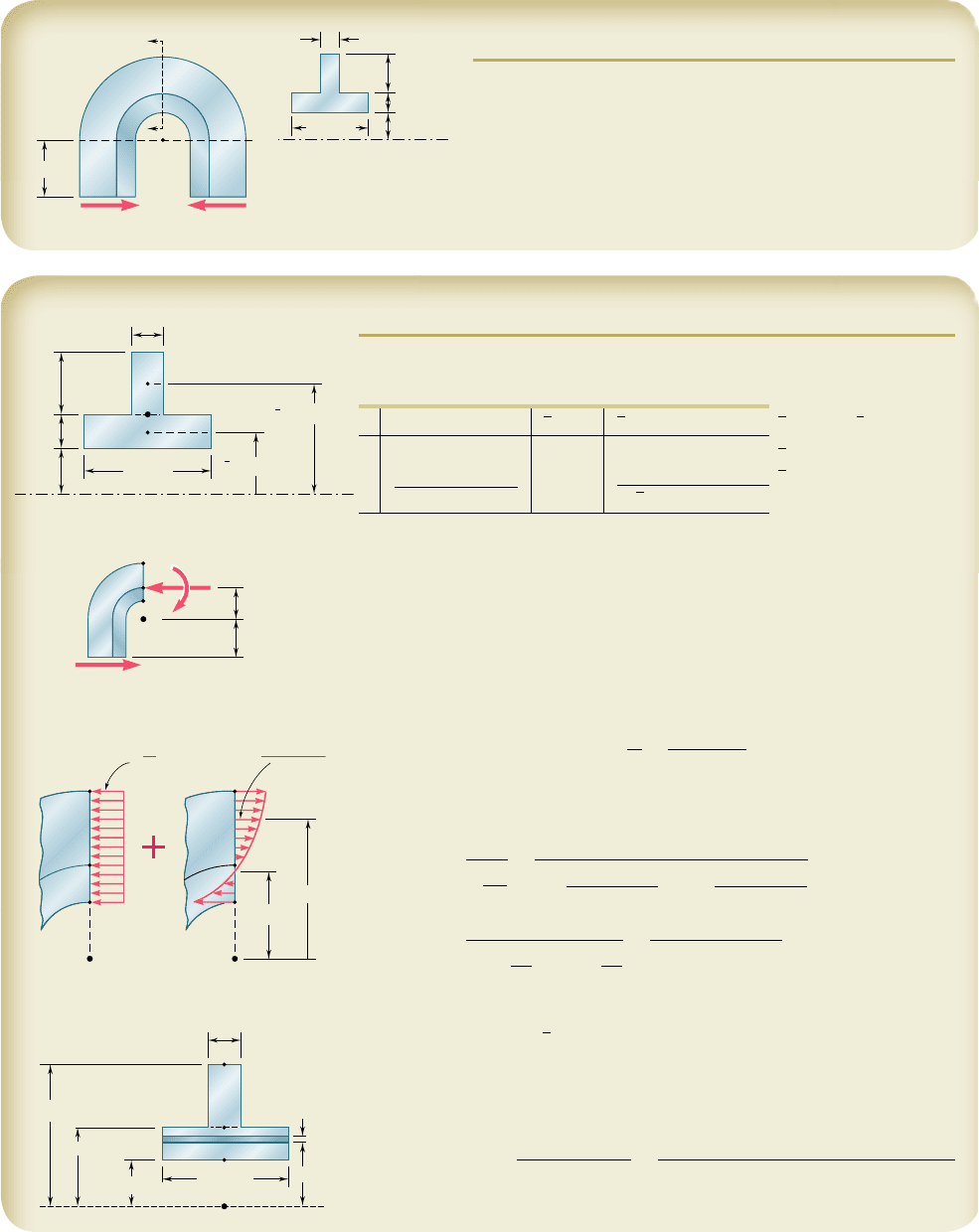
For the bar of Example 4.10, determine the largest tensile and compressive
stresses, knowing that the bending moment in the bar is M 5 8 kip ? in.
We use Eq. (4.71) with the given data,
M 5 8 kip ? in. A 5 bh 5 (2.5 in.)(1.5 in.) 5 3.75 in
2
and the values obtained in Example 4.10 for R and e,
R 5 5.969 e 5 0.0314 in.
Making first r 5 r
2
5 6.75 in. in Eq. (4.71), we write
s
max
5
M
1
r
2
2 R
2
Aer
2
5
1
8 kip ? in.
21
6.75 in. 2 5.969 in.
2
1
3.75 in
2
21
0.0314 in.
21
6.75 in.
2
s
m
a
x
5 7.86
k
si
Making now r 5 r
1
5 5.25 in. in Eq. (4.71), we have
s
min
5
M
1
r
1
2 R
2
Aer
1
5
1
8 kip ? in.
21
5.25 in. 2 5.969 in.
2
1
3.75 in
2
21
0.0314 in.
21
5.25 in.
2
s
min
529.30
k
si
Remark. Let us compare the values obtained for s
max
and s
min
with
the result we would get for a straight bar. Using Eq. (4.15) of Sec. 4.4, we
write
s
max, min
56
M
c
I
56
1
8 kip ? in.
21
0.75 in.
2
1
12
1
2.5 in.
21
1.5 in.
2
3
568.53 ksi
299
EXAMPLE 4.11
bee80288_ch04_220-313.indd Page 299 10/27/10 5:46:26 PM user-f499bee80288_ch04_220-313.indd Page 299 10/27/10 5:46:26 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04
Apago PDF Enhancer
300
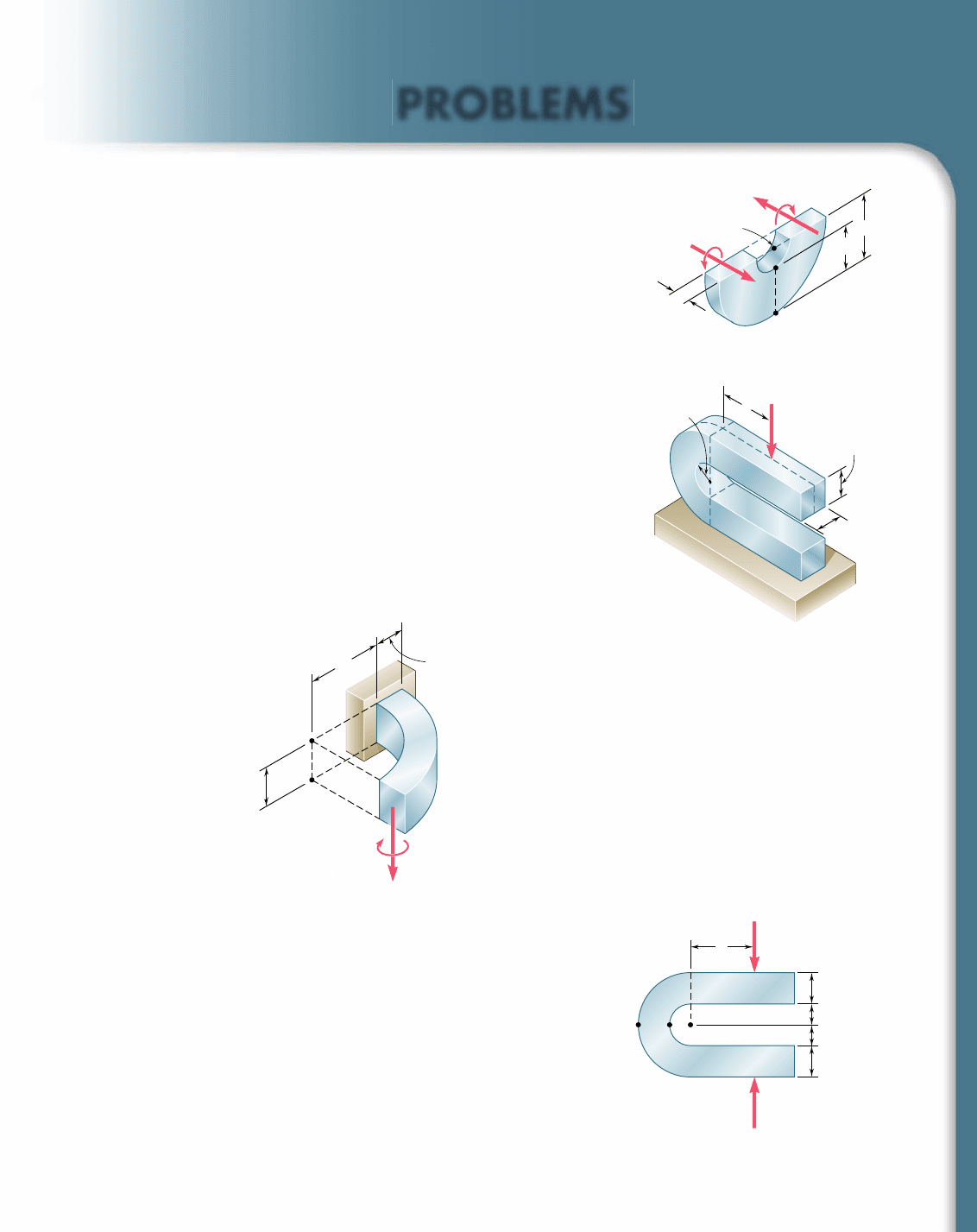
SAMPLE PROBLEM 4.11
A machine component has a T-shaped cross section and is
loaded as shown. Knowing that the allowable compressive
stress is 50 MPa, determine the largest force P that can be
applied to the component.
SOLUTION
Centroid of the Cross Section. We locate the centroid D of the cross
section
A
i
, mm
2
r
i
, mm
r
i
A
i
, mm
3
r
©
A
i
5
©
r
i
A
i
1
1
20
21
80
2
5 1600 40 64 3 10
3
r
1
2400
2
5 120 3 10
3
2
1
40
21
20
2
5 800 70 56 3 10
3
r 5 50 mm 5 0.050 m
©
A
i
5 2400 © r
i
A
i
5 120 3 10
3
Force and Couple at D. The internal forces in section a-a are equiva-
lent to a force P acting at D and a couple M of moment
M 5 P(50 mm 1 60 mm) 5 (0.110 m)P
Superposition. The centric force P causes a uniform compressive
stress on section a-a. The bending couple M causes a varying stress distribu-
tion [Eq. (4.71)]. We note that the couple M tends to increase the curvature
of the member and is therefore positive (cf. Fig. 4.70). The total stress at a
point of section a-a located at distance r from the center of curvature C is
s 52
P
A
1
M
1
r 2 R
2
Aer
(1)
Radius of Neutral Surface. We now determine the radius R of the
neutral surface by using Eq. (4.66).
R 5
A
#
dA
r
5
2400 mm
2
#
r
2
r
1
180 mm2 d
r
r
1
#
r
3
r
2
120 mm2 d
r
r
5
2400
80 ln
50
30
1 20 ln
90
50
5
2400
40.866 1 11.756
5 45.61 mm
5 0
.
04561 m
We also compute:
e
5 r 2 R 5 0
.
05000 m 2 0
.
04561 m 5 0
.
00439 m
Allowable Load. We observe that the largest compressive stress will
occur at point A where r 5 0.030 m. Recalling that s
all
5 50 MPa and using
Eq. (1), we write
250 3 10
6
Pa 52
P
2.4 3 10
23
m
2
1
1
0.110 P
21
0.030 m 2 0.04561 m
2
1
2.4 3 10
23
m
2
21
0.00439 m
21
0.030 m
2
250 3 10
6
52417P 2 5432P P 5 8.55 kN
◀
60 mm
20 mm
Section a-a
40 mm
20 mm
30 mm80 mm
a
a
P' P
40 mm
20 mm
2
1
r
1
40 mm
20 mm
30 mm
80 mm
r
2
70 mm
M
P
B
A
C
50 mm
60 mm
D
P'
B
P
A
D
A
C
–
B
M (r – R)
Aer
D
A
R
r
C
B
A
D
C
d
r
r
20 mm
80 mm
r
3
90 mm
r
2
50 mm
r
1
30 mm
bee80288_ch04_220-313.indd Page 300 11/11/10 3:03:20 PM user-f499bee80288_ch04_220-313.indd Page 300 11/11/10 3:03:20 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04
Apago PDF Enhancer
PROBLEMS
301
4.161 For the machine component and loading shown, determine the
stress at point A when (a) h 5 2 in., (b) h 5 2.6 in.
4.162 For the machine component and loading shown, determine the
stress at points A and B when h 5 2.5 in.
4.163 The curved portion of the bar shown has an inner radius of 20 mm.
Knowing that the allowable stress in the bar is 150 MPa, determine
the largest permissible distance a from the line of action of the
3-kN force to the vertical plane containing the center of curvature
of the bar.
4.164 The curved portion of the bar shown has an inner radius of 20 mm.
Knowing that the line of action of the 3-kN force is located at a
distance a 5 60 mm from the vertical plane containing the center
of curvature of the bar, determine the largest compressive stress
in the bar.
4.165 The curved bar shown has a cross section of 40 3 60 mm and an
inner radius r
1
5 15 mm. For the loading shown determine the
largest tensile and compressive stresses.
C
B
A
0.75 in.
4 kip · in.
3 in.
h
4 kip · in.
Fig. P4.161 and P4.162
25 mm
25 mm
r 20 mm
P 3 kN
a
Fig. P4.163 and P4.164
40 mm
60 mm
120 N · m
r
1
Fig. P4.165 and P4.166
4.166 For the curved bar and loading shown, determine the percent
error introduced in the computation of the maximum stress by
assuming that the bar is straight. Consider the case when (a) r
1
5
20 mm, (b) r
1
5 200 mm, (c) r
1
5 2 m.
4.167 The curved bar shown has a cross section of 30 3 30 mm. Knowing
that a 5 60 mm, determine the stress at (a) point A, (b) point B.
4.168 The curved bar shown has a cross section of 30 3 30 mm. Knowing
that the allowable compressive stress is 175 MPa, determine the
largest allowable distance a.
20 mm
20 mm
30 mm
30 mm
BA
C
a
5 kN
5 kN
Fig. P4.167 and P4.168
bee80288_ch04_220-313.indd Page 301 10/26/10 4:34:54 PM user-f499bee80288_ch04_220-313.indd Page 301 10/26/10 4:34:54 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles
Apago PDF Enhancer
302
Pure Bending
4.169 Steel links having the cross section shown are available with dif-
ferent central angles b. Knowing that the allowable stress is 12 ksi,
determine the largest force P that can be applied to a link for
which b 5 908.
0.4 in.
0.4 in.
0.3 in.
0.8 in.
0.8 in.
1.2 in.
A
A
C
B
P' P
B
Fig. P4.169
4.170 Solve Prob. 4.169, assuming that b 5 608.
4.171 A machine component has a T-shaped cross section that is orien-
tated as shown. Knowing that M 5 2.5 kN ? m, determine the
stress at (a) point A, (b) point B.
4.172 Assuming that the couple shown is replaced by a vertical 10-kN
force attached at point D and acting downward, determine the
stress at (a) point A, (b) point B.
4.173 Three plates are welded together to form the curved beam shown.
For the given loading, determine the distance e between the neu-
tral axis and the centroid of the cross section.
Dimensions in mm
100
60
5040 20
20
B
C
A
D
AB
M
2.5 kN · m
Fig. P4.171 and P4.172
A
C
B
M'
M
2 in.
3 in.
0.5 in. 2 in.
3 in.
0.5 in.
0.5 in.
Fig. P4.173 and P4.174
4.174 Three plates are welded together to form the curved beam shown.
For M 5 8 kip ? in., determine the stress at (a) point A, (b) point B,
(c) the centroid of the cross section.
4.175 The split ring shown has an inner radius r
1
5 20 mm and a circular
cross section of diameter d 5 32 mm. For the loading shown,
determine the stress at (a) point A, (b) point B.
4.176 The split ring shown has an inner radius r
1
5 16 mm and a circular
cross section of diameter d 5 32 mm. For the loading shown,
determine the stress at (a) point A, (b) point B.
d
B
A
r
1
2.5 kN
Fig. P4.175 and P4.176
bee80288_ch04_220-313.indd Page 302 11/11/10 3:03:21 PM user-f499bee80288_ch04_220-313.indd Page 302 11/11/10 3:03:21 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04
Apago PDF Enhancer
303
Problems
4.177 The curved bar shown has a circular cross section of 32-mm diameter.
Determine the largest couple M that can be applied to the bar about
a horizontal axis if the maximum stress is not to exceed 60 MPa.
16 mm
12 mm
M
C
Fig. P4.177
4.178 The bar shown has a circular cross section of 0.6 in.-diameter. Know-
ing that a 5 1.2 in., determine the stress at (a) point A, (b) point B.
4.179 The bar shown has a circular cross section of 0.6-in. diameter.
Knowing that the allowable stress is 8 ksi, determine the largest
permissible distance a from the line of action of the 50-lb forces
to the plane containing the center of curvature of the bar.
4.180 Knowing that P 5 10 kN, determine the stress at (a) point A,
(b) point B.
4.181 and 4.182 Knowing that M 5 5 kip ? in., determine the stress
at (a) point A, (b) point B.
50 lb
50 lb
0.5 in.
0.6 in.
a
BAC
Fig. P4.178 and P4.179
90 mm
80 mm
A
B
100 mm
P
Fig. P4.180
4.183 For the curved beam and loading shown, determine the stress at
(a) point A, (b) point B.
2.5 in.
3 in.
2 in.
2 in.
3 in.
B
C
M
A
M
Fig. P4.181
3 in.
M
M
B
A
C
3 in.
2 in.
2 in.
2.5 in.
Fig. P4.182
20 mm
30 mm
35 mm
40 mm
a
a
B
A
B
A
250 N · m
250 N · m
Section a-a
Fig. P4.183
35 mm
60 mm
25 mm
40 mm
60 mm
15 kN
a
a
Section a-a
Fig. P4.184
4.184 For the crane hook shown, determine the largest tensile stress in
section a-a.
bee80288_ch04_220-313.indd Page 303 11/11/10 3:03:21 PM user-f499bee80288_ch04_220-313.indd Page 303 11/11/10 3:03:21 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04
Apago PDF Enhancer
304
Pure Bending
4.185 Knowing that the machine component shown has a trapezoidal
cross section with a 5 3.5 in. and b 5 2.5 in., determine the stress
at (a) point A, (b) point B.
4.186 Knowing that the machine component shown has a trapezoidal
cross section with a 5 2.5 in. and b 5 3.5 in., determine the stress
at (a) point A, (b) point B.
4.187 Show that if the cross section of a curved beam consists of two or
more rectangles, the radius R of the neutral surface can be
expressed as
R 5
A
ln
ca
r
2
r
1
b
b
1
a
r
3
r
2
b
b
2
a
r
4
r
3
b
b
3
d
where A is the total area of the cross section.
4.188 through 4.190 Using Eq. (4.66), derive the expression for R
given in Fig. 4.73 for
*4.188 A circular cross section.
4.189 A trapezoidal cross section.
4.190 A triangular cross section.
*4.191 For a curved bar of rectangular cross section subjected to a bend-
ing couple M, show that the radial stress at the neutral surface is
s
r
5
M
Ae
a
1 2
r
1
R
2 ln
R
r
1
b
and compute the value of s
r
for the curved bar of Examples 4.10
and 4.11.
(Hint: consider the free-body diagram of the portion of the beam
located above the neutral surface.)
6 in. 4 in.
C
B
B
A
A
b
a
80 kip · in.
Fig. P4.185 and P4.186
r
1
r
2
b
1
b
2
b
3
r
3
r
4
Fig. P4.187
r
r
x
x
C
R
b
r
1
2
2
Fig. P4.191
bee80288_ch04_220-313.indd Page 304 10/26/10 4:35:20 PM user-f499bee80288_ch04_220-313.indd Page 304 10/26/10 4:35:20 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles
Apago PDF Enhancer
305
REVIEW AND SUMMARY
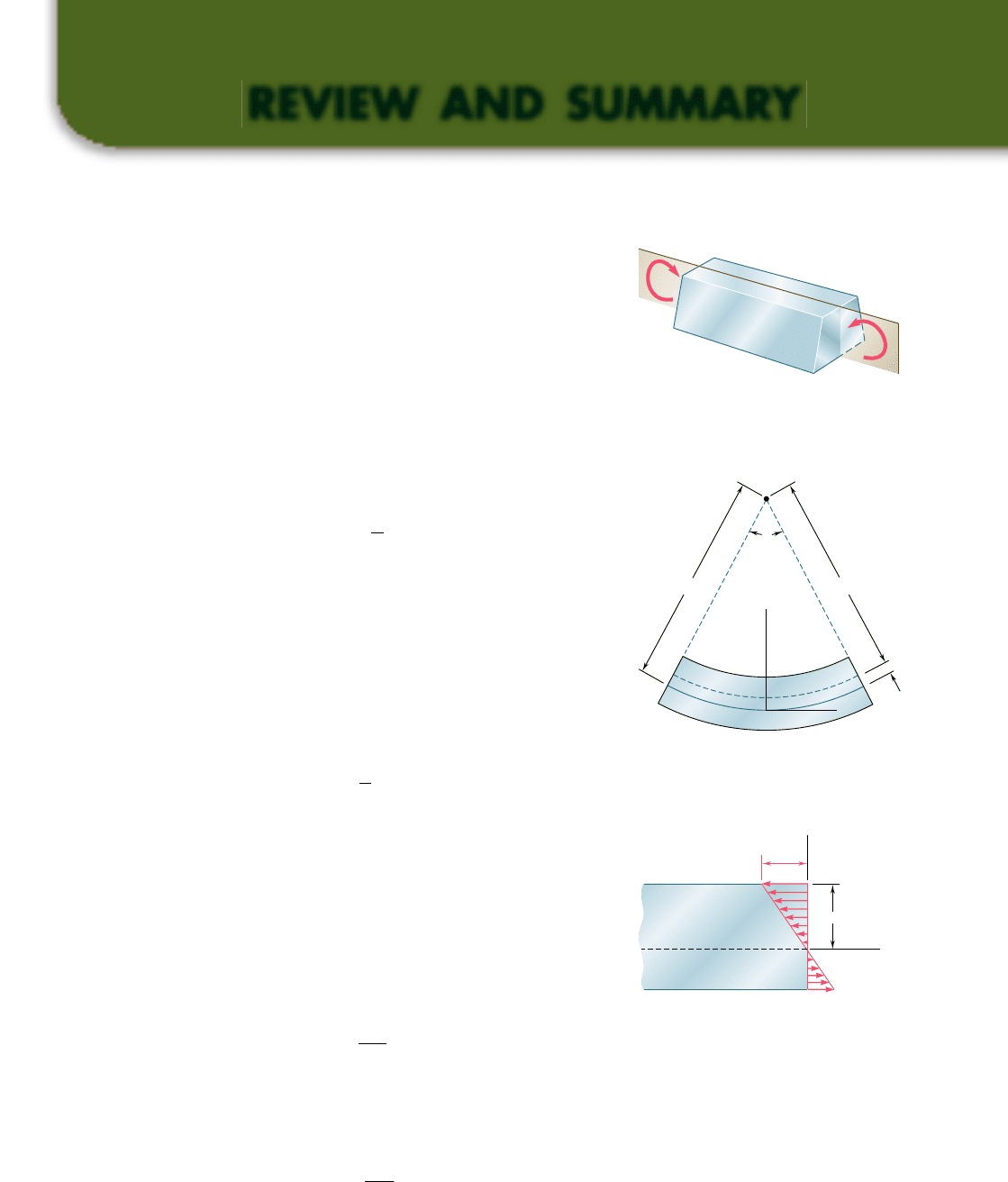
This chapter was devoted to the analysis of members in pure bend-
ing. That is, we considered the stresses and deformation in members
subjected to equal and opposite couples M and M9 acting in the
same longitudinal plane (Fig. 4.77).
We first studied members possessing a plane of symmetry and sub-
jected to couples acting in that plane. Considering possible deforma-
tions of the member, we proved that transverse sections remain plane
as a member is deformed [Sec. 4.3]. We then noted that a member
in pure bending has a neutral surface along which normal strains and
stresses are zero and that the longitudinal normal strain P
x
varies
linearly with the distance y from the neutral surface:
P
x
52
y
r
(4.8)
where r is the radius of curvature of the neutral surface (Fig. 4.78).
The intersection of the neutral surface with a transverse section is
known as the neutral axis of the section.
For members made of a material that follows Hooke’s law [Sec. 4.4],
we found that the normal stress s
x
varies linearly with the distance
from the neutral axis (Fig. 4.79). Denoting by s
m
the maximum
stress we wrote
s
x
52
y
c
s
m
(4.12)
where c is the largest distance from the neutral axis to a point in the
section.
By setting the sum of the elementary forces, s
x
dA, equal to zero, we
proved that the neutral axis passes through the centroid of the cross
section of a member in pure bending. Then by setting the sum of the
moments of the elementary forces equal to the bending moment, we
derived the elastic flexure formula for the maximum normal stress
s
m
5
Mc
I
(4.15)
where I is the moment of inertia of the cross section with respect to
the neutral axis. We also obtained the normal stress at any distance
y from the neutral axis:
s
x
52
My
I
(4.16)
Normal strain in bending
Normal stress in elastic range
A
B
M
M'
Fig. 4.77
y
y
– y
A
J
D
O
C
B
K
E
x
A⬘
B⬘
Fig. 4.78
y
c
m
x
Neutral surface
Fig. 4.79
Elastic flexure formula
bee80288_ch04_220-313.indd Page 305 10/26/10 4:35:28 PM user-f499bee80288_ch04_220-313.indd Page 305 10/26/10 4:35:28 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles
Apago PDF Enhancer
306
Pure Bending
Noting that I and c depend only on the geometry of the cross sec-
tion, we introduced the elastic section modulus
S 5
I
c
(4.17)
and then used the section modulus to write an alternative expression
for the maximum normal stress:
s
m
5
M
S
(4.18)
Recalling that the curvature of a member is the reciprocal of its
radius of curvature, we expressed the curvature of the member as
1
r
5
M
EI
(4.21)
In Sec. 4.5, we completed our study of the bending of homogeneous
members possessing a plane of symmetry by noting that deforma-
tions occur in the plane of a transverse cross section and result in
anticlastic curvature of the members.
Next we considered the bending of members made of several materi-
als with different moduli of elasticity [Sec. 4.6]. While transverse
sections remain plane, we found that, in general, the neutral axis
does not pass through the centroid of the composite cross section
(Fig. 4.80). Using the ratio of the moduli of elasticity of the materials,
1
2
N. A.
x
– —
x
x
y
2
– —–
E
2
y
1
– —–
E
1
y
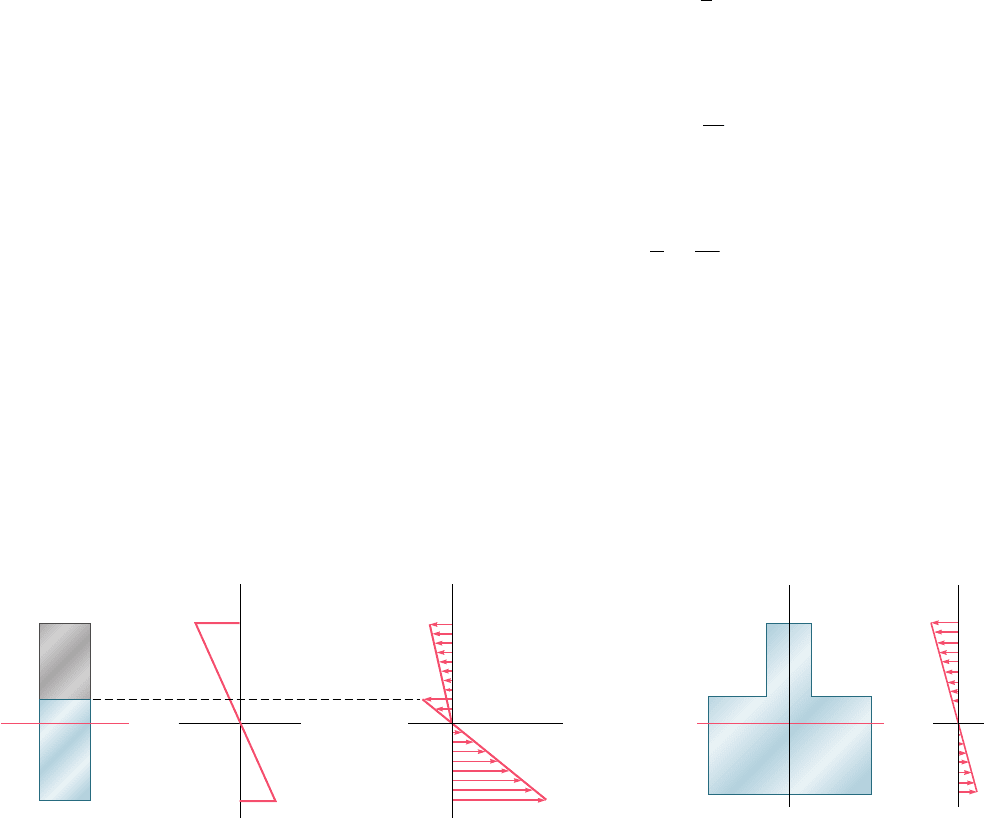
yy
(a)(b)(c)
Fig. 4.80
C
N. A.
x
– —–
My
I
yy
x
Fig. 4.81
we obtained a transformed section corresponding to an equivalent
member made entirely of one material. We then used the methods
previously developed to determine the stresses in this equivalent
homogeneous member (Fig. 4.81) and then again used the ratio of
the moduli of elasticity to determine the stresses in the composite
beam [Sample Probs. 4.3 and 4.4].
In Sec. 4.7, stress concentrations that occur in members in pure bend-
ing were discussed and charts giving stress-concentration factors for flat
bars with fillets and grooves were presented in Figs. 4.27 and 4.28.
Members made of several materials
Elastic section modulus
Curvature of member
Anticlastic curvature
Stress concentrations
bee80288_ch04_220-313.indd Page 306 11/11/10 3:03:22 PM user-f499bee80288_ch04_220-313.indd Page 306 11/11/10 3:03:22 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04
