Basdevant J.-L., Rich J., Spiro M. Fundamentals in Nuclear Physics: From Nuclear Structure to Cosmology

Подождите немного. Документ загружается.

9.7 Cosmological nucleosynthesis 433

the data. In this case, it is necessary to suppose that the two lines in Fig. 9.12

near 555.8 nm are correctly identiﬁed and to suppose that the measured abun-

dances are primordial. If either hypothesis is false, the measurement must be

reinterpreted. For instance, the “deuterium” line could be a hydrogen line of

a second cloud of a slightly diﬀerent redshift. This would cause the observers

to overestimate the deuterium and therefore underestimate Ω

. On the other

hand, if the measured deuterium is not primordial, the primordial deuterium

is underestimated since stellar processes generally destroy deuterium. This

would cause an overestimation of Ω

It is clear that the value of η derived from the

H abundance requires

conﬁrmation from independent measurements. The measured abundances of

He and

Li (Table 9.3) give qualitative conﬁrmation though some contro-

versy continues [106]. The total amount of gas in the Ly-α forest gives a lower

limit on Ω

that is consistent with the nucleosynthesis value [107]. Finally,

the spectra of CBR anisotropies [94] favors a similar value.

We end this section with some comments on how the results of cosmo-

logical nucleosynthesis depends on the fundamental constants. The situation

is similar to that in astrophysical nucleosynthesis where the production of

elements with A>8 depended strongly on the alignment of states of

Be,

Cand

O (Fig. 8.5). In the cosmological case, we have already mentioned

two important facts

• m

by 0.1%. If this mass-ordering were reversed, the now stable

neutron would be more abundant than protons when the weak-interactions

froze their ratio. Not suﬀering from the Coulomb barrier, these neutrons

would attach themselves to

Htoform

H which could fuse to form

He by

the reaction

H →

He nn. The end products of cosmological nucleosyn-

thesis could then be a mixture of stable neutrons and

He. Later, in stars,

nuclear burning would start with n n →

−

. Once heavy elements are

formed, the free neutrons would be rapidly absorbed by radiative capture.

• The proton–proton system is unbound by ∼ 50 keV. If it were bound,

diprotons would be formed that would then β-decay to

H. The end product

of cosmological nucleosynthesis would be a mixture of

He,

He and any

H that failed to fuse to form helium. Later, in stars, nuclear burning would

start with

H →

He γ since no weak interactions are required.

In the m

scenario, very little hydrogen would be available for

the development of life based on organic chemistry. The pp-stable scenario

is a bit less hopeless since organic chemistry could still be possible with the

small amount of surviving

H. Note that the fact that stellar nuclear burning

would not have to start with a weak interaction does not mean that stars

would burn faster. Indeed, we saw in Chap. 8 that the luminosity of a star

does not depend on the cross-section for the nuclear reactions producing the

luminosity. The higher d-d cross-section compared to p-p cross-section would

just mean that hydrogen-burning stars would burn at a lower temperature

andbestableatalargerradius.

434 9. Nuclear Cosmology

The results of cosmological nucleosynthesis depend on another relation

between the weak interactions and gravity. The neutron–proton ratio was

frozen when the expansion rate, ∼ (G(kT)

/(¯hc)

)

1/2

, was equal to the weak-

interaction rate, ∼ G

(kT)

/(¯h

). The fact that the freeze-out, ∼ 0.8MeV

is very near the neutron–proton mass diﬀerence ∼ 1.29 MeV is due to the

“coincidence”

(∆mc

)



G¯h

11/2

9/2

∼ 1 . (9.98)

If the left side were much greater than unity, the weak interactions would

have maintained chemical equilibrium longer, resulting in a smaller neutron–

proton ratio. Only hydrogen would have been produced. On the other hand,

if the left side were much smaller than unity, chemical equilibrium would have

been broken sooner, leading to equal numbers of neutrons and protons. Only

He would have been produced. In fact, nature ﬁnds itself just at the frontier

between these two scenarios resulting in the production of an “interesting”

mixture of hydrogen and helium.

9.8 Wimps

The three known neutrino species were relativistic when they decoupled

(kT

 m

).Theconsequenceofthisisthattodaythenumberdensityof

neutrinos is of the same order of magnitude as that of photons n

=(3/11)n

If one of these neutrinos is suﬃciently massive to be non-relativistic today,

its present mass density would be ρ

= m

.ThisgivesΩ

∼ 0.3 for a

neutrino mass of order 10 eV/c

Any hypothetical stable particle with mc

> 10 eV that decoupled when

it was relativistic would create problems for cosmology because the calculated

mass density would be overcritical. A heavy weakly interacting particle can

give an appropriate cosmological density only if it has an annihilation cross-

section suﬃciently large to keep it in equilibrium until the particle was non-

relativistic, kT

 mc

. In this case its number density would be suppressed

by the Boltzmann factor and the present density might not be too large.

In fact, if the cross-section is chosen correctly, the particle can give a relic

density near critical and constitute the desired nonbaryonic dark matter.

Such a compensation between relic density and mass would seem a priori

improbable, but stranger things have happened in cosmology. In fact, as

it turns out, particles with weak interaction and masses in the GeV range

naturally give relic densities within an order of magnitude or so of critical.

Such particles are called “wimps” for “weakly interacting massive particle.”

A stable wimp is generally predicted by supersymmetric extensions of the

standard model of particle physics. The particle is called the “LSP” (lightest

supersymmetric particle) and is denoted by χ. Supersymmetric wimps are

9.8 Wimps 435

usually “Majorana” particles, i.e. they are their own antiparticle. Supersym-

metric theories have many parameters that are not (yet) ﬁxed by experiment

so one can generally choose parameters that give a cross-section yielding the

required relic density. The fact that they have not been seen at accelerators

means that probably m

> 30 GeV [102].

As with electrons and positrons, an approximate solution of wimp Boltz-

mann equation is

∼ n

(T ) T  T

(9.99)

∼ constant T  T

, (9.100)

where T

is the freeze-out temperature corresponding to the moment when

the annihilation rate was equal to the expansion rate:

) σv = H(T

) ∼



(¯hc)



1/2

. (9.101)

−5

−10

−15

0.1 1

10 100

/ kTmc

/ n

v/c = 10

−39

−37

−35

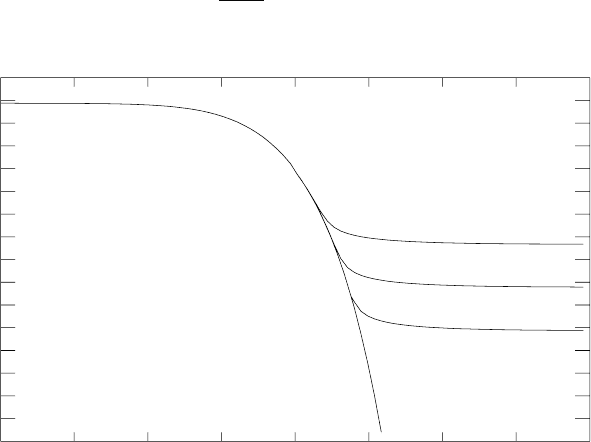
Fig. 9.14. log(n

) versus temperature for three values of the annihilation cross-

section σv/c. The wimp mass was taken to be m

= 50 GeV. The dotted line

shows log(n

) in thermal equilibrium. Freeze-out occurs around T

∼ m

/20.

We see that to good approximation the relic density is inversely proportional to the

cross-section.

The numerical solution is shown for three values of the cross-section in

Fig. 9.14. We see that, because of the exponential dependence of n

(T )for

436 9. Nuclear Cosmology

T<m

, the freeze-out temperature is relatively insensitive to the cross-

section, T

∼ m

/20. We can therefore easily estimate the χ relic density by

equating the annihilation rate and the expansion rate. This gives:

)

∼

)

∝

σvm

. (9.102)

We see that the ratio is inversely proportional to the wimp mass and an-

nihilation cross section. This ratio stays roughly constant after the freeze

so today’s wimp-proton ratio is of the same order of magnitude. Using the

present-day photon density, this gives a present-day wimp mass density of

= n

∝

σv

. (9.103)

It turns out that the a cross-section of σv/c∼10

−41

gives the observed

density of cold dark matter. Supersymmetric models that give this cross-

section can be constructed.

If it turns out that such supersymmetric models describe nature, the

dark-matter mystery would be solved. Eﬀorts are underway to detect super-

symmetric particles at accelerators (LHC) . Experiments are also attempting

to observe directly Galactic wimps by detecting particles recoiling from rare

wimp-nucleus scattering events (Exercise 9.7).

Bibliography

1. J. Rich, Fundamentals of Cosmology, Springer, Berlin, 2001.

Exercises

9.1 The luminosity density of the Universe (photons produced by stars)is

∼ 1.2 ×10



Mpc

−3

. Supposing that stellar light output has been relatively

constant since the formation of the ﬁrst stars one Hubble time ago, esti-

mate the number of photons (E ∼ 2 eV) that have been produced by stars.

Compare the number of stellar photons with the number of CBR photons.

Stellar energy is mostly produced by the fusion of hydrogen to helium

4p →

He+2e

+2ν

. This transformation occurs through a series of reactions

in stellar cores that liberate a total of ∼ 25 MeV. After thermalization, the

energy emerges from stellar surfaces in the form of starlight. Estimate the

number of protons (per Mpc

) that have been transformed into helium over

the last Hubble time. Compare this number with the number of protons

available n

∼ Ω

9.2 Estimate the contribution to the universal photon mean free path of the

following processes:

Exercises for Chapter 9 437

• Thomson scattering of photons on free electrons of number density n

∼ n

• Absorption by stars of number density n

stars

∼ Ω

stars



, Ω

stars

∼

0.003 and cross-section ∼ πR



• Absorption by dust in galaxies with n

gal

∼ 0.005 Mpc

−3

and cross-section

∼ πR

gal

where R

gal

∼ 10 kpc and the fraction of visible light absorbed

when passing through a galaxy is  ∼ 0.1.

Compare these distances with the “Hubble distance,” d

≡ c/H

(∼ the

distance of the most distant visible objects). Is the Universe “transparent”?

9.3 The cross-section times velocity for the reaction np →

H γ is

σv ∼ 7.4 × 10

−20

−1

(v  c) . (9.104)

(a) Showthattherateperneutronofthisreactionissmallerthantheex-

pansion rate at kT ∼ 60 keV if η<4 × 10

−12

. It follows that there is no

nucleosynthesis if η is less than this value.

If η>4 × 10

−12

H is in thermal equilibrium with neutrons and pro-

tons. The abundances of

H, protons and neutrons are governed by the Saha

equation:

=(2π¯hc)



2π



3/2

B/T

where B =2.2 MeV is the

H binding energy.

(b) Show that for η ∼ 5 × 10

−10

the great majority of neutrons are free

until kT ∼ 60 keV. (Since the majority of baryons are protons, you can

approximate n

∼ ηn

9.4

To derive (9.47) in the case of a Universe dominated by non-relativistic

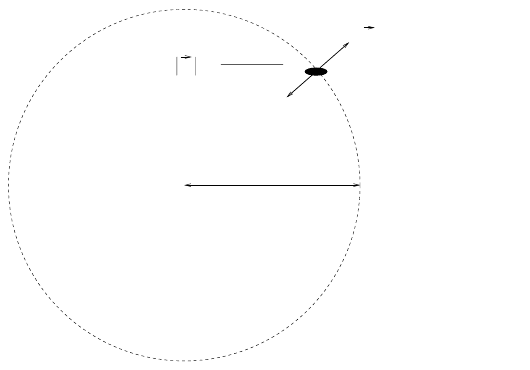
matter (p ∼ 0), we can use a simple Newtonian argument. Referring to Fig.

9.15, we place a galaxy of mass m at a distance R = χa(t) from the “center”

of a universe of uniform density ρ. Since the mass distribution is spherically

symmetric, Gauss’s theorem “suggests” that the galaxy is subject to a grav-

itational force directed toward the origin that is proportional to the total

mass at a distance <χa(t) from the origin:

|F | =

GM(χ)m

, (9.105)

where

M(χ)=4π(χa)

ρ/3 . (9.106)

(We ignore the question of whether Gauss’s theorem applies in an inﬁnite

medium.) Using |F | = m

R = mχ¨a, ﬁnd the equation (9.47) for the decelera-

tion of the Universe.

438 9. Nuclear Cosmology

GM( )m

) =

ρ 4π(

)

T = m( )

U = −GmM(

|v| =

a(t)

( )

a(t)

Fig. 9.15. A Newtonian treatment of the universal expansion. A galaxy of mass

m placed in a universe of uniform density ρ at a distance χa(t) from the “center of

the Universe.” The spherical symmetry suggests that the Newtonian force on the

galaxy will be directed toward the origin with a magnitude F = GM(χ)m/(χa(t))

where M(χ) is the total mass at a distance <χa(t) from the origin. For a uniform

mass density ρ, M (χ)=4π(χa)

ρ/3.

9.5 Estimate the time available for neutron decay, corresponding to the

temperature range 800 keV >kT >50 keV, by integrating (9.56) between

these limits and using the approximation a ∝ 1/T . Take g(T )=4isan

appropriate mean value over the interval.

9.6 Consider a universe with equal numbers of electrons and positrons. If

the universe is in thermal equilibrium at kT  m

, use the principle of

detailed balance (Sect. 4.1.5) applied to the reaction e

−

↔ γγ to show

that the number density of electrons is proportional to a Boltzmann factor

exp(−m

/kT ). For kT  m

, explain why it is a good approximation to

ignore the eﬀects of stimulated emission.

9.7 The dark matter in Milky Way is believed to be made up of weakly-

interacting massive particles (wimps). The mass density of galactic wimps at

thepositionoftheEarthisestimatedtobeρc

∼ 0.3 GeV cm

−2

.Thewimps

move around the galaxy with the same mean speed as stars, v ∼ 10

−3

Suppose that the wimps have a mass of 50 GeV/c

. What is their typical

kinetic energy? In a collision with a nucleus, would you expect a wimp to be

able to excite or break the nucleus?

Exercises for Chapter 9 439

Suppose that the elastic wimp cross section is 10

−35

on moderate

mass nuclei. What is the mean free path of wimps in the Earth?

Experiments have attempted to detect the wimps with germanium detec-

tors via the elastic scattering

χ Ge → χ Ge . (9.107)

The recoiling nucleus creates a signal in the diode. What is the scattering rate

for the assumed cross-section? Compare this rate the decay rate of cosmogenic

Ge, ∼ 0.3 mBq kg

−1

(Sect. 5.2.2).

Current experiments [103] have not observed a signal that must be as-

cribed to wimp scattering. This negative result places an upper limit on the

wimp scattering cross-section.

A. Relativistic kinematics

In this appendix, we brieﬂy review some facts from the special theory of rela-

tivity that are useful in nuclear physics. Relativity is used in nuclear physics

primarily through the relativistic expressions for the energy and momentum

of a free particle of (rest) mass m and velocity v:

E =



1 − v

p =



1 − v

. (A.1)

The energy and momentum deﬁned in this way are conserved quantities.

They satisfy

= m

+ p

. (A.2)

In nuclear physics, the non-relativistic limit v  c (⇒ pc  E) usually

applies for nuclei, in which case we have

E ∼ mc

v =

. (A.3)

For neutrinos and photons, the limit mc  p generally applies:

E ∼ pc +

.v= c



1 −



. (A.4)

It is customary to group energy and momentum in a single object called

the energy-momentum 4-vector

P ≡ (E, p) . (A.5)

In a particle’s rest-frame, it takes the value (mc

, 0, 0, 0). The squared mag-

nitude of the 4-vector is deﬁned as

≡ P · P ≡ E

− p · p = m

, (A.6)

where the last form follows from (A.1). The magnitude is clearly independent

of the energy of the particle, i.e. it is invariant with respect to changes of

reference frame.

Consider the energy-momentum of a particle, P ,viewedinaninertialref-

erence frame. Consider another inertial reference frame moving with velocity

v in,say,thez direction with respect to the ﬁrst. The energy-momentum

442 A. Relativistic kinematics

4-vector in the second is related to that in the ﬁrst by a Lorentz transforma-

tion:

⎛

⎜

⎝



⎞

⎟

⎠

⎛

⎜

⎝

γ 00−βγ

0100

0010

−βγ 00 γ

⎞

⎟

⎠

⎛

⎜

⎝

⎞

⎟

⎠

, (A.7)

where

β = v/c γ =



1 − β

. (A.8)

The generalizations to other directions are obvious. This relation follows triv-

ially from (A.1) if one of the frames is the rest-frame. It is less obvious in the

general case but note that the transformation has the virtue of maintaining

the magnitude (A.6), as it must.

Energy momentum conservation can be economically expressed by using

4-vectors. Consider the decay

A → BC . (A.9)

Energy-momentum conservation is

= P

+ P

, (A.10)

which is entirely equivalent to

= E

+ E

= p

+ p

. (A.11)

One is often called upon to calculate the momentum of the decay products

in the rest frame of the decaying particle (p

= 0). Momentum conservation

gives p

= −p

so energy conservation gives



+ m



+ m

, (A.12)

where p is the common momentum we would like to ﬁnd. This equation is

not especially easy to solve. It is much easier to write the 4-vector equation

= P

− P

. (A.13)

We now take the squared magnitude of both sides of this equation:

=(P

− P

)

= P

+ P

− 2P

· P

. (A.14)

The ﬁrst two terms on the right give m

+ m

. Since the scalar product

· P

is Lorentz invariant, we can evaluate it in the rest frame of A:

· P

≡ E

− p

· p

= m



+ p

. (A.15)

We thus deduce



+ m

− m

, (A.16)

A. Relativistic kinematics 443



+ m

− m



− m

. (A.17)

We note that in nuclear physics we can often use directly energy con-

servation (A.12) because all the particles are either ultra-relativistic or non-

relativistic so we can eliminate the square roots. For example, in radiative

decay of an excited nucleus

(A, Z)

∗

→ (A, Z) γ , (A.18)

the two nuclei are non-relativistic so energy conservation is

∗

= mc

+ pc , (A.19)

The nuclear kinetic energy is pv/2  pc so we have immediately

pc ∼ (m

∗

− m)c

. (A.20)

This also follows from (A.17) in the limit m

− m

∼ 2m

− m

)and

=0.