Baggott J. The Quantum Story: A History in 40 Moments
Подождите немного. Документ загружается.

the quantum story
240
considered as a solid ‘ball’ of material substance, with mass and charge
distributed evenly, much like snow and ice is distributed in a snowball.
Or it could be thought of as a region of largely empty space containing
discrete, point-like charged constituents. High-energy electrons encoun-
tering such point-like constituents could be expected to be scattered in
greater numbers and at larger angles.
Bjorken found that, for these different conceptions of the proton struc-
ture, the theory predicted quite different results in the region of deep
inelastic collisions. He realized that an experimental test was possible,
comparable in many ways to the Geiger–Marsden experiments which
supported the view that the mass of an atom is concentrated in a small
positively charged nucleus. Just as the scattering of alpha-particles from
their target gold atoms revealed the interior structure of the atom, so the
observations of electrons scattered from target protons would reveal the
interior structure of the proton.
Bjorken speculated that such point-like constituents might be quarks
and performed some calculations for the spectrum of scattered electron
energies that would result. But his heart wasn’t yet in it. The quark model
was still treated with derision by the physics community and there were
alternative theories available that were better regarded. He quickly
backed off from proposing that these experiments could be used as a
test of the quark model. ‘I brought it in mainly as a desperate attempt
to interpret the rather striking phenomenon of point-like behaviour,’ he
explained when challenged on this view at a conference held at Stanford
in September 1967.
Studies of deep inelastic scattering at relatively small angles from a
liquid-hydrogen target began at SLAC around this time and continued
through October 1967. They were carried out by a small experimental
group including MIT physicists Jerome Friedman and Henry Kendall and
Canadian-born SLAC physicist Richard Taylor. The experiments did not
initially go smoothly.
The analysis programs had to be rewritten as we continued to test the
workings of the spectrometer hardware. After a couple of weeks of total
chaos, things began to settle down. We took a full set of data at 6° with sev-
eral initial energies and with scattered electron energies down to 2–3 GeV.
deep inelastic scattering
241
The raw data had to be corrected for all the radiative processes involving
the swarm of virtual particles surrounding the electron. Although these
corrections were signifi cant, accounting for up to half of the measure-
ments recorded in the SLAC experiments, they could be handled satisfac-
torily using quantum electrodynamics.
Even at the relatively low scattering angles of 6° and 10°, the physicists
observed many more scattered electrons than they had anticipated. They
focused their attention on the behaviour of something called the ‘struc-
ture function’ as a function of the difference between the initial electron
energy and the scattered electron energy. This difference is related to the
energy lost by the electron in the collision or the energy of the virtual
photon that is exchanged. They saw that as the virtual photon energy
was increased, the structure function showed marked peaks correspond-
ing to the expected proton resonances. However, as the energy increased
further, these peaks gave way to a broad, featureless plateau that fell away
gradually as it extended well into the range of deep inelastic collisions.
Bjorken had anticipated something like this. It was indeed the signa-
ture of point-like proton constituents. He made a further suggestion.
If the structure function declined gradually with increasing energy of
the virtual photon, then the product of these (called F), plotted against
the photon energy (or frequency, n) divided by the square of something
called the four-momentum transfer (q
2
), might show some interesting
behaviour.
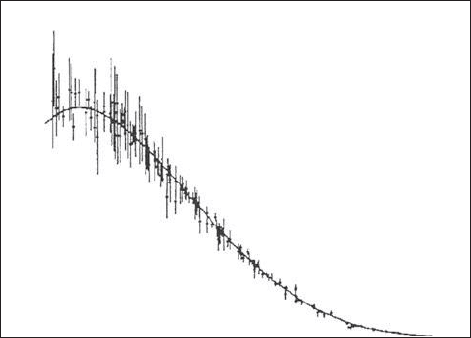
5
Kendall drew the graph by hand, using two sheets of orange graph
paper that had been taped together. It showed F increasing to a maxi-
mum for n/q
2
around 3 GeV, and remaining fairly constant thereafter.
Bjorken called it ‘scaling’. The curve was the same irrespective of the
electron energy. The mathematics said it had to be this way, but it was
not at all clear what this meant.
Irrespective of what it meant, Kendall was struck by the way the
experimental data had so closely conformed to Bjorken’s predictions.
Years later he recalled ‘. . . wondering how Balmer may have felt when
he saw, for the fi rst time, the striking agreement of the formula that
5
The four-momentum transfer is calculated from the initial and fi nal electron energies and
the scattering angle.

the quantum story
242
bears his name with the measured wavelengths of the atomic spectra
of hydrogen.’
There was as yet no rush to embrace these experimental results as
proof of the existence of an internal structure for the proton, and cer-
tainly no rush to declare the results as evidence for the existence of
quarks. There were other possible theoretical explanations for the scat-
tering results and for the phenomenon of scaling, and arguments broke
out even within the MIT-SLAC group. Nevertheless, on Kendall’s insist-
ence the graph that Bjorken had suggested was included in a paper on the
group’s results presented at a conference in Vienna in August 1968.
Richard Feynman had collected a Nobel Prize in December 1965 for his
contribution to the development of quantum electrodynamics, alongside
Julian Schwinger and Sin-Itiro Tomonaga. In the years that followed he had
adapted to the celebrity status it afforded, but had at the same time lost touch
with contemporary high-energy physics. He had helped resolve some of the
0.5
0.4
6° 16 GeV
6° 13.5 GeV
6° 10 GeV
0.3
0.2
0.1
F =Frequency x Structure Function
0.0
01 2 3 4 5 6 7
Frequency/Four-momentum Transfer (per GeV)
fig 15 Prompted by suggestions by Bjorken, Kendall drew up this graph by hand.
The shape of the resulting curve was the same irrespective of the electron energy (in
this case, 10, 13.5 and 16 GeV, with a scattering angle of 6°). Bjorken called it ‘scaling’. It
was the tell-tale signature of point-like constituents inside the proton. Adapted from
Michael Riordan, The Hunting of the Quark: A True Story of Modern Physics, Simon &
Shuster, New York, 1987, p. 146.
deep inelastic scattering
243
problems of QED and, with Gell-Mann, had made important contributions
to the theory of the weak force. But the strong force had eluded him.
He had resolved to play catch-up. In June 1968 he developed an out-
line of a theory of the hadrons in which he imagined them to consist of
point-like constituents which, for want of a better name, he had dubbed
partons, meaning simply the ‘parts’ of hadrons. The parton model was not
grounded in a detailed quantum fi eld theory, it was simply an idea he was
toying with. What he wanted to know was that if the hadrons could be
thought to be composed of partons, what were the consequences?
He had also developed an image in his mind of two hadrons colliding
together. At high speeds, special relativity says that each hadron ‘sees’ the
other not as a fully fl eshed-out three-dimensional object but rather as a
two-dimensional fl at pancake. If these pancakes were dotted with tiny,
hard partons, then a hadron–hadron collision would really be the sum of
a series of individual collisions between the constituent partons.
Feynman’s sister Joan lived in a house just across the road from SLAC,
and during visits to her he would take the opportunity to ‘snoop around’
the facility. He would chat to the physicists at a picnic table in the shade
of the oak trees near the SLAC cafeteria, regaling them with stories from
his Los Alamos days. He would also listen carefully as they told him
about the latest developments in high-energy physics.
In August 1968 he heard about the work of the MIT-SLAC group on
deep inelastic scattering. A second round of experiments was about to
begin, but the physicists were still puzzling over the interpretation of the
data from the previous year. Bjorken was out of town, but his new post-
doctoral student Emmanuel Paschos told Feynman about scaling and
asked him what he thought.
When Feynman saw Kendall’s graph he collapsed to his knees, his
hands raised to the heavens. ‘All my life,’ he exclaimed, ‘I’ve looked for an
experiment like this, one that can test a fi eld theory of the strong force!’
Feynman knew that the graph was telling him something, but he was
not quite sure what it was. He fi gured it out that night in his motel room.
The graph was related to the distribution of momentum of the point-like
constituents—the partons—in the proton. In fact, simply by inverting
the function that Kendall had plotted along the horizontal axis (in other
words, by plotting q
2
/n), the graph became a probability distribution,
the quantum story
244
each point a measure of the probability of the electron colliding with a
parton carrying a specifi c fraction of the total momentum of the proton.
This could be pushed a little further. When divided by the proton mass,
the quantity q
2
/n is related to the fraction of the proton mass carried by
the parton.
‘I’ve really got something to show youse guys,’ Feynman declared to
Friedman and Kendall the next morning, ‘I fi ggered it all out in my motel
room last night!’ This was followed by much enthusiastic arm-waving.
Bjorken had arrived at most of the conclusions that Feynman had now
drawn, but Bjorken had expressed them in the esoteric language of current
algebra. When he had had a chance to read Bjorken’s papers, Feynman
acknowledged his priority. But, once again, Feynman was describing the
events in a far simpler, yet richer, more visual way. When he returned to
SLAC in October 1968 to deliver a lecture on the parton model, it was
like setting a fi re. Suddenly, all the talk among SLAC physicists was of
0.5
0
0.4
0.3
0.2
0.1
F = Frequency x Structure Function
0.0
Parton Mass Fraction
0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0
fig 16 Feynman fi gured that he could re-plot Kendall’s graph in terms of the frac-
tion of the proton mass carried by the point-like constituents, which he called ‘par-
tons’. The result would be a distribution of the probability for the electron colliding
with a parton carrying a specifi c fraction of the proton’s total momentum. Adapted
from Michael Riordan, The Hunting of the Quark: A True Story of Modern Physics, Simon
& Shuster, New York, 1987, p. 152.
deep inelastic scattering
245
partons. Nothing is more guaranteed to breed confi dence in a bold idea
than its enthusiastic reception by a Nobel Laureate.
Were partons actually quarks? Feynman didn’t know and didn’t care. Some
of the SLAC theorists developed models in which the partons were pions and
protons, stripped of their clouds of virtual particles. But Bjorken and Paschos
soon had a detailed model of partons based on a triplet of quarks.
Further experiments at scattering angles of 18°, 26°, and 34° confi rmed
the original impressions and reproduced the scaling behaviour. By 1970
experiments had been performed using a liquid-deuterium target. By
carefully separating the contributions to scattering from the proton, it
was possible to determine the equivalent structure function for the neu-
tron and so probe its interior structure in the same way.
These were diffi cult experiments, and it was not all plain sailing. One
theoretical model describing collisions between electrons and protons and
electrons and neutrons suggested that the extent of deep inelastic scattering
should depend on the relative strengths of the electric charges of the parton
constituents. If the partons were considered to be quarks, then the ratio of
scattering events for the neutron versus the proton should be given as the
ratio of the sum of the squares of the charges of their quarks constituents.
For the neutron, thought to consist of an up quark and two down quarks
(udd), the sum of the squares of the fractional charges is
6
⁄
9
or
2
⁄
3
. For the
proton, consisting of two up quarks and a down quark (uud), the sum is
9
⁄
9
,
or 1. The ratio of neutron versus proton scattering events (N/P), was there-
fore expected to fall with increasing q
2
/n to a limiting value of
2
⁄
3
.
Early results appeared to be consistent with this simple model. But as
q
2
/n increased, the ratio N/P was discovered to fall substantially below
2
⁄
3
.
These results ruled out a variety of competing theories, but seemed also
to tell against the model of fractionally charged quarks.
Theorists had a fi eld day trying to rationalize these results with exist-
ing models. There was still some hope for quarks, however. The model
that predicted a limiting value of N/P of
2
⁄
3
was rather naïve. It assumed
that the momentum of the nucleon was evenly distributed over the three
quark constituents. There were other models in which the three quarks
which characterize the nucleon (called ‘valence’ quarks) swim in a ‘sea’ of
quark–anti-quark pairs.
the quantum story
246
In addition, the quarks (or, more generally, partons) were now thought
to be bound together through the exchange of strong force fi eld particles
called gluons, the ‘glue’ that holds the ‘parts’ together. These short-range
force particles were required to be massive. So, the momentum of the
nucleons may be shared among quark–anti-quark pairs and gluons, and
is in any case not necessarily obliged to be shared equally among the
valence quarks. It was recognized that N/P could indeed fall below
2
⁄
3
.
But it could not fall below a lower limit of ¼, in which only a down quark
from the neutron and an up quark from the proton contribute to the
scattering.
When experimental results suggested that the ratio actually did fall
below ¼, both Bjorken and Feynman insisted that no further modifi ca-
tions could save the quark theory. If the results were right, then the par-
tons simply could not be fractionally charged quarks.
The results were wrong. An error was discovered in the computer
program used to correct for the effects of ‘smearing’ due to the inter-
nal motions of the protons and neutrons in the hydrogen and deuterium
target atoms. When this error was removed, the ratio of N/P was seen to
fall to a limiting value close to ¼.
Earlier experimental studies had revealed that the partons were spin-½
fermions. The N/P data now strongly supported the idea of fractional
charges. Experimental results from studies of deep inelastic scattering of
neutrinos from protons at CERN provided further supporting evidence.
By mid-1973, quarks had offi cially ‘arrived’. They might have been con-
ceived partly in jest as a strange quirk of nature, but they had now taken
a decisive step towards acceptance as real constituents of the hadrons.
Some questions remained unanswered. If 20-GeV electrons were strik-
ing fractionally charged quarks inside protons or neutrons, resulting in
the destruction of the target nucleons, how come no free quarks had ever
been seen? More worrying, perhaps, was the fact that the quarks inside
the proton and neutron behaved as though they were largely independ-
ent of each other. The phenomenon of scaling suggested that the quarks
could be considered to roam freely inside the nucleons.
How was this possible? Surely, the strong force must keep the quarks
tightly bound inside the nucleons; so tightly bound that they are forever
confi ned?

247
The SLAC results on deep inelastic scattering of electrons from protons were still inter-
preted with some caution. There were many questions that remained unanswered. But, for
the advocates of the quark model, here was the fi rst evidence that these ultimate constitu-
ents of matter might actually be real.
Sheldon Glashow was an early convert to the quark model. In fact, based on little more
than an appeal to an essential tidiness in nature, he had speculated with James Bjorken in
1964 that there should be a fourth quark, which they had called charm. At that time the
four leptons—electron, electron neutrino, muon, and muon neutrino—seemed at odds
with just three quarks—up, down, and strange. A fourth quark was surely needed to even
things up.
Glashow had collaborated with Bjorken whilst visiting the Niels Bohr Institute in
Copenhagen. For Bjorken, this was an unusual line of reasoning. More used to anticipat-
ing the properties and behaviour of particles likely to have implications for experiment,
this was for him, at best, a highly speculative proposal. ‘You have to remember the fl a-
vour of the time,’ he explained, ‘It was an easygoing time for models. Models came and
went.’ He was nevertheless rather ambivalent about the proposal. Instead of using his own
name, James Daniel Bjorken, he signed the paper ‘B.J. Bjørken’, using his nickname, ‘BJ’,
and a Danish interpretation of his originally Swedish surname.
Glashow’s attempts to build an SU(2) × U(1) fi eld theory of the weak and electromag-
netic forces had foundered on the problem of the Z
0
boson and the weak neutral currents it
implied. With Bjorken he had actually arrived at the solution to this problem, but he had
25
Of Charm and Weak
Neutral Currents
Harvard, February 1970
the quantum story
248
failed to make the connection. It seems that Glashow had forgotten all about his earlier
efforts. ‘The problem which was explicitly posed in 1961 was solved, in principle, in 1964.
No one, least of all me, knew it.’
He returned to the problems of the SU(2) × U(1) theory in 1970, in the company of
two Harvard postdoctoral students, Greek physicist John Iliopoulos and Italian Luciano
Maiani. Glashow had fi rst met Iliopoulos at CERN and had been impressed with his
efforts to fi nd ways to renormalize a fi eld theory of the weak force. Maiani arrived at
Harvard with some curious ideas about the strength of weak-force interactions. All three
realized that their interests converged. At this stage none was aware of Weinberg’s use of
spontaneous symmetry-breaking and the Higgs mechanism.
The three physicists now wrestled with the theory once again. But they kept running up
against the same problems. Admitting the fi eld particle masses by hand produced unruly
divergences. Then there was the problem of the weak neutral currents. The theory pre-
dicted that a neutral kaon should in principle decay by emission of a Z
0
boson, changing
the strangeness of the particle in the process and producing two muons. However, this was
a decay mode for which there was absolutely no experimental evidence. For some reason,
this particular reaction was being suppressed. But how?
Glashow was fi nally ready to put two-and-two together. In doing so he would help to
sow the seeds of a major revolution in particle physics.
Even among strange particles, the neutral kaon, K
0
, and its antiparticle,
K
−
0
, can be regarded as ‘odd’. According to the quark model, the positive
kaon is a composite of an up quark and an anti-strange quark (us
−
), with
charges of +²⁄
³
and +¹⁄
³
adding to a total of +1. Its anti-particle is the nega-
tive kaon, a composite of a strange quark and an anti-up quark (su
−
), with
charges of −¹⁄
³
and −²⁄
³
adding to a total of −1.
For the neutral kaon, however, we have two possibilities. Both the
combinations down/anti-strange (ds
−
) and strange/anti-down (s
−
d) yield
zero net charge and, with no means to distinguish them, these two par-
ticles can form a quantum ‘superposition’, their wavefunctions overlap-
ping and combining. The result is two new neutral kaon states. The fi rst
is formed from the sum of the wavefunctions of K
0
and K
-
0
and is referred
to as K
0
1
. The second is formed from the difference of the wavefunctions
and is referred to as K
0
2
.
The particles suffer something of an identity crisis. Although the kaons
are formed in their initial K
0
and K
-
0
states by strong-force interactions,
of charm and weak neutral currents
249
by the time the weak force acts on them they have transformed into K
0
1
and K
0
2
.
However, this is not quite the end of the story. When parity was shown
to be violated in weak-force interactions, it was assumed that a combi-
nation of charge conjugation (the symmetry between a particle and its
anti-particle) and parity would nevertheless be preserved.
1
Thus, the
superposition K
0
1
, which is even under the charge conjugation-parity (CP)
symmetry operation, can decay only into particles which are also even
under this symmetry. K
0
1
was found to decay into two pions, consistent
with this view. Likewise K
0
2
, which has odd CP symmetry, was found to
decay into three pions.
However, experiments performed at Brookhaven National Labora-
tory by Princeton physicists James Cronin and Val Fitch showed that K
0
2
kaons formed in a beam would very occasionally decay into two pions.
These events were few in number, about 50 in a total of 23,000 observed
decays, but they were much higher than expected from any background
K
0
1
particles left in the beam. Cronin and Fitch concluded that CP sym-
metry, too, is violated by weak-force interactions.
2
The explanation for this is that the states K
0
1
and K
0
2
‘contaminate’ each
other slightly. What are actually seen in experiments are not pure K
0
1
and K
0
2
, but K
0
L
, which is mostly K
0
2
contaminated with a little bit of K
0
1
,
and K
0
S
, which is mostly K
0
1
with a little bit of K
0
2
. The subscripts L and S
stand for ‘long’ and ‘short’, identifying these kaon states as long-lived and
short-lived neutral kaons, respectively.
The problem confronting Glashow, Iliopoulos, and Maiani in February
1970 was principally concerned with taming the divergences in their SU(2)
× U(1) fi eld theory. They tried all kinds of different approaches. Iliopou-
los later described their plight. ‘Every day,’ he said, ‘invariably, one of us
would come up with an idea. Then the other two would prove to him he
was wrong. We tried all sorts of different recipes, and nothing worked.’
1
For example, the application of the CP symmetry operation to a spin-up electron trans-
forms it into a spin-down positron.
2
The reason for CP violation is still the subject of active research. Cronin and Fitch were
awarded the Nobel Prize for physics in 1980.
