Astakhov V. Tribology of Metal Cutting

Подождите немного. Документ загружается.

Basics, Deﬁnitions and Cutting Tool Geometry 397

SECTION N−N

VIEW S

SECTION P−P

(Plane P

)

(Plane P

)

(Plane P)

SECTION O−O

(Plane P

)

SECTION F−F

SECTION O'−O'

(Plane O')

−l

−g

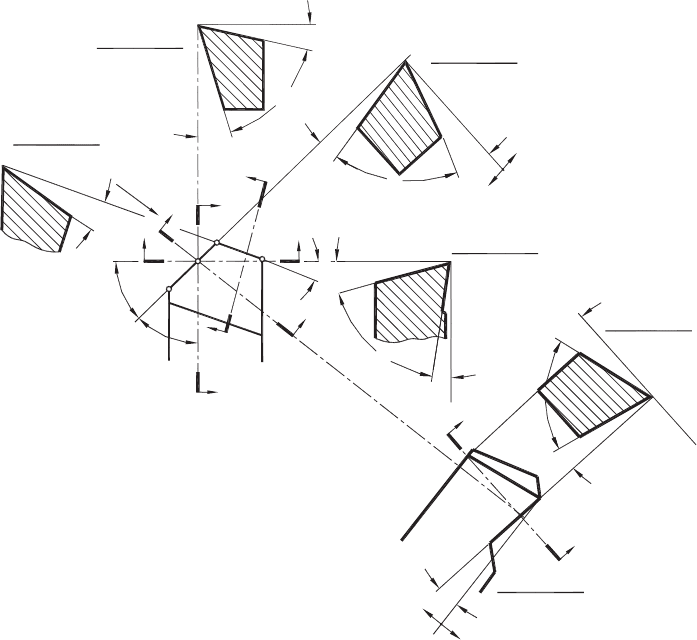
Fig. A4. Tool angles in the tool-in-hand system.

of rake, wedge and clearance angles is 90

◦

, i.e.

+β

+α

= γ

+β

+α

= γ

+β

+α

= γ

+β

+α

= 90

◦

(A1)

• The orientation and inclination of the cutting edge are speciﬁed in the tool cutting

edge plane P

. In this plane, the cutting edge inclination angle λ

is the angle

between the cutting edge and the reference plane. This angle is deﬁned as always

being acute and positive if the cutting edge, when viewed in a direction away from

the selected point at the tool corner being considered, lies on the opposite side of

the reference plane from the direction of primary motion. This angle can be deﬁned

at any point of the cutting edge. The sign of the inclination angle is well deﬁned

in Fig. A4.

• The deﬁnition of the tool cutting edge angle κ

is shown in Fig. A2. It is deﬁned

as the acute angle that the tool cutting edge plane makes with the working plane

398 Tribology of Metal Cutting

assumed and is measured in the reference plane P

. It can also be deﬁned as the acute

angle between the projection of the main cutting edge into the reference plane and

the x direction. Angle κ

is always positive and it is measured in a counter-clockwise

direction from the position of the working plane assumed.

• Similarly, the tool minor (end) cutting edge angle κ

is the acute angle that the minor

cutting edge plane makes with the working plane assumed and is measured in the

reference plane P

. It can also be deﬁned as the acute angle between the projection of

the minor (end) cutting edge into the reference plane and the x direction (Fig. A4).

Angle κ

is always positive (including zero) and it is measured in a clockwise

direction from the position of the working plane assumed.

A3.1 Cutting edge angle

The tool cutting edge angle signiﬁcantly affects the cutting process because, for a given

feed and cutting depth, it deﬁnes the uncut chip thickness, width of cut, and thus tool

life. The physical background of this phenomenon can be explained as follows: when κ

decreases, the chip width increases correspondingly because the active part of the cutting

edge increases. The latter results in improved heat removal from the tool and hence the

tool life increases. For example, if the tool life of a High Speed Steel (HSS) face milling

tool having κ

= 60

◦

is taken to be 100%, when κ

= 30

◦

, its tool life is 190%, and

when κ

= 10

◦

its tool life is 650%. Even more profound effect of κ

is observed in

the machining with single-point cutting tools. For example, in rough turning of carbon

steels, the change of κ

from 45 to 30

◦

sometimes leads to a ﬁvefold increase in the

tool life.

The reduction of κ

, however, has its drawbacks. One of them is the corresponding

increase of the radial component, (F

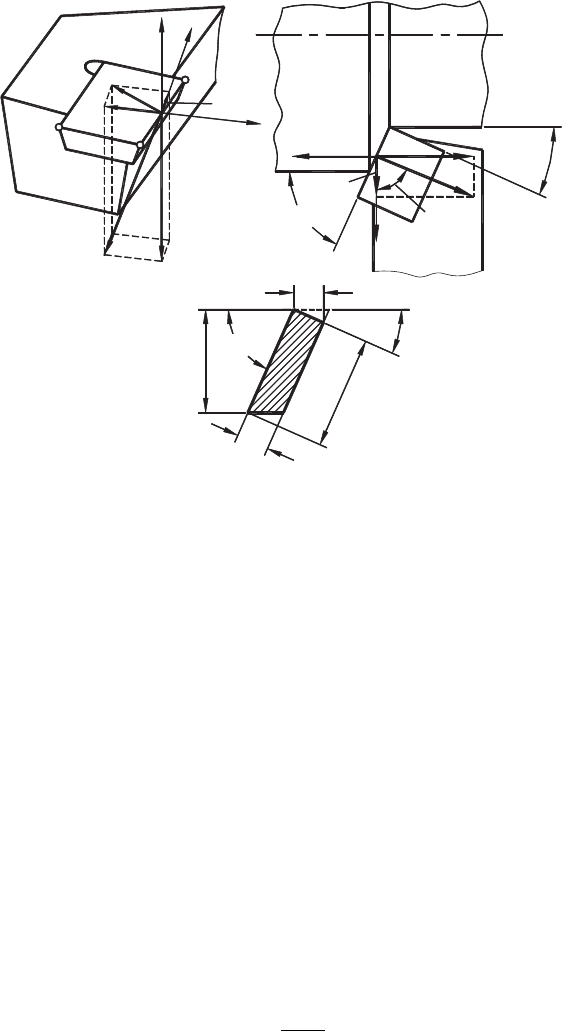
) of the cutting force. Figure A5(a) shows that in

general, the cutting force, (R) is a 3D vector and thus it can be resolved into three orthogo-

nal components, namely, the power (tangential, torque) component (F

) (often referred to

in literature on metal cutting as (F

), axial component (F

) and radial component (F

For simplicity, these are often called tangential, axial and radial forces, respectively.

As shown in Fig. A5(b), the radial and axial forces are related as

= tan κ

(A2)

From Eq. (A2), lowering κ

from 45 to 20

◦

results in more than twofold increase in

the radial force that increases the bending force acting on the workpiece and thus may

reduce the accuracy of machining because the rigidity of the workpiece varies along its

length. When the workpiece is machined between the centers, an increased radial force

causes the so-called barreling, and when the workpiece is clamped only in the chuck,

tapering may occur. Besides, since lowering κ

leads to an increased radial force, this

force often causes vibrations so that the advantages of small cutting edge angle may not

become too profound.

Basics, Deﬁnitions and Cutting Tool Geometry 399

(a) (b)

(c)

Fig. A5. The cutting force system and the uncut chip thickness for a single-point cutting tool.

Another drawback of small κ

is the reduction in the uncut chip thickness t

. From

Fig. A5(c)

= f sin κ

, (A3)

where f is the cutting feed per revolution.

It follows from Eq. (A3) that under a given feed f, the uncut chip thickness can be varied

in a wide range by changing the angle κ

. When this angle becomes zero, the uncut chip

thickness is also zero (no cutting) and when κ

= 90

◦

, the uncut chip thickness reaches

its maximum becoming t

= f as in orthogonal cutting. Hence, t

∈

(

0,f

)

The following equation for the uncut chip area correlates the feed (f ) and the depth of

cut (d

) with the uncut chip thickness (t

) and non-orthogonal chip width d

= b

f = t

= A

(A4)

and

= f sin κ

(A5)

sin κ

, (A6)

where A

is the cross-sectional area of the uncut chip.

400 Tribology of Metal Cutting

From Eq. (A4), under a given feed and depth of cut, any change of t

causes a

corresponding change of d

= b

. As shown, when t

∈

(

0,f

)

, the chip width

∈

(

∞,d

)

In general, the speciﬁc cutting force (the force that is acting on the unit length of the

cutting edge) is proportional to the uncut chip thickness. For this reason, the uncut chip

thickness is often referred to as the chip load in literature on metal cutting. As discussed

earlier in this book, this parameter is of great importance in determining many tribological

characteristics of the cutting process. A rule of thumb here is: the smaller the chip load,

the higher the tool life, and vice versa. However, there is the minimum uncut chip

thickness (under other given cutting parameters) below which the opposite effect is true.

Two factors primarily limit the minimum allowable uncut chip thickness:

• Decreasing the uncut chip thickness, one should increase the width of cut according

to Eq. (A4). When t

→ 0, d

→∞, i.e. the length of the cutting edge should be

increased to inﬁnity. Obviously, this is not practical.

• Uncut chip thickness cannot be less than the radius of the cutting edge.

Real cutting cannot be regarded as free cutting because real tools always have more than

one cutting edge engaged in cutting. There have been a number of attempts to allow

for the inter-inﬂuence of the neighboring cutting edges in determining the direction of

chip ﬂow. They are well summarized in [2]. Klushin [3] and Stabler [4] suggested the

determination of the true uncut chip thickness t

in a plane perpendicular to the direction

of chip ﬂow while the true uncut chip width b

(

w1T

)

in the perpendicular direction

and equal to the length of the segment AB, which joins the ends of the major and minor

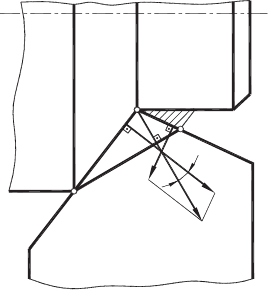
cutting edges engaged in cutting, as shown in Fig. A6. In this ﬁgure, the directions

AC and BC are orthogonal chip ﬂow directions of the major and minor cutting edges,

respectively, and the direction AB is the resultant chip-ﬂow direction. The angle between

AC and AB is referred to as the chip-ﬂow angle η

. The segment AB is often referred

Fig. A6. Chip-ﬂow direction.

Basics, Deﬁnitions and Cutting Tool Geometry 401

(a) (b)

1.1f

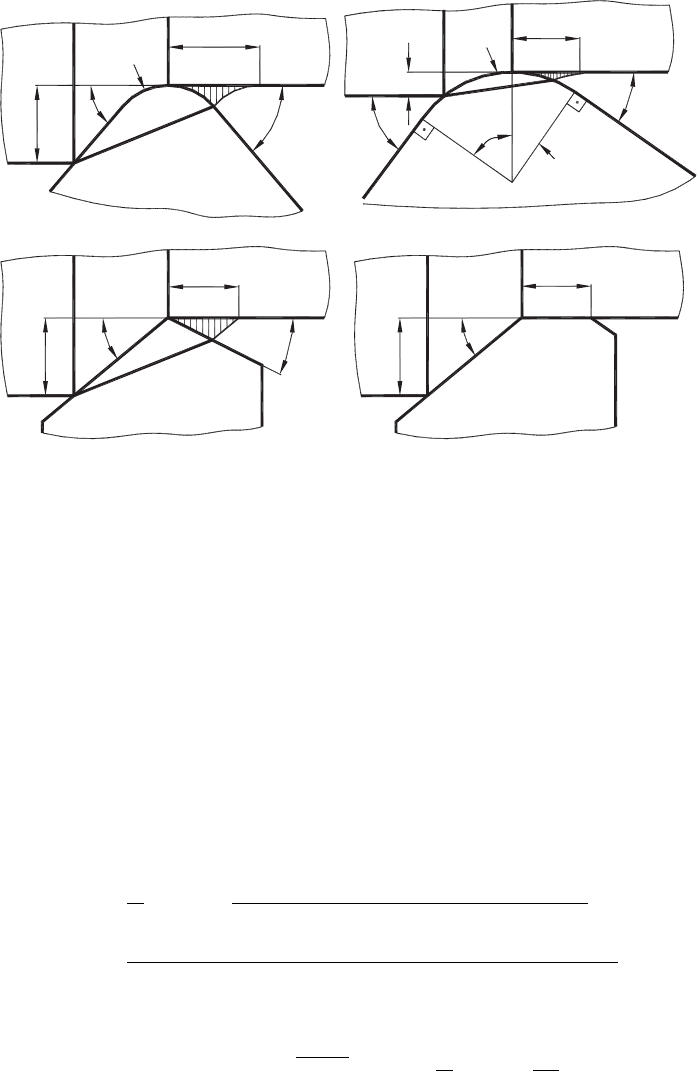
Fig. A7. Four basic conﬁgurations in non-free cutting.

to as the equivalent cutting edge, as suggested by Colwell [5]. Therefore, in non-free

cutting, the uncut chip thickness depends on the orientation of the equivalent cutting edge.

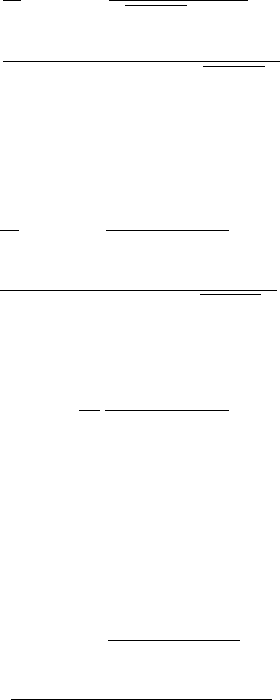
Figure A7 presents four basic conﬁgurations in non-free cutting. The ﬁrst conﬁguration,

shown in Fig. A7(a), represents the most common single-point tools in use today. The cut-

ting tool is made with a nose radius r

and set so that the depth of cut is greater than the

nose radius.

If the following relationships are justiﬁed

≥ r

(

1 − cos κ

)

,f≤ 2r

sin κ

(A7)

then the formulas for calculation of t

and b

are as follows:

sin arctan

[

1 − e

(1 − cos κ

)

]

cot κ

(sin κ

)

(A8)

sin arctan {c

([

1 − e

(1 − cos κ

)

]

cot κ

(sin κ

)

}

, (A9)

where

= 1 −e



1 −



1 − g



(A10)

402 Tribology of Metal Cutting

The second conﬁguration, shown in Fig. A7(b) is similar to the ﬁrst except that only the

radius part of the cutting edge is engaged in cutting. If the following relationships are

justiﬁed

(

1 − cos κ

)

,f≤ 2r

sin κ

(A11)

the formulas for calculation of t

and b

are as follows:

sin arctan

√

−1 +e

(A12)

sin arctan



/(e

√

−1)



(A13)

In the third conﬁguration (Fig. A7(c)), the nose radius is rather small compared to the

depth of cut, so that only the straight parts of the major and minor cutting edges are

considered. Then the formulas for calculation of t

and b

are as follows:

sin arctan

1 + cot κ

−q

(A14)

sin arctan



/(e

√

−1)



, (A15)

where

= 1 −

cot κ

+cot κ

(A16)

In the fourth conﬁguration (Fig. A7(d)), the nose radius is rather small so that only

the straight parts of the major and minor cutting edges are considered. To improve the

machined surface ﬁnish, the minor cutting edge is positioned parallel to the axis of

rotation of the workpiece so that κ

= 0. As such, the length of the minor cutting edge

is usually selected to be equal to 1.1f . The formulas for calculation of t

and b

are

as follows:

= f sin arctan

cot κ

+2.2e

(A17)

sin arctan

(

1/(cot κ

+2.2e

)

(A18)

Although the fourth conﬁguration has been known for many years and its advantages are

well documented, its implementation has often been accompanied by vibration and thus

was restricted by the rigidity of the machine tools. Only recently, when machine tools

became rigid enough, it became possible to use tools with such geometry on rigid milling

machines. Cutting inserts of such geometry known now as wiper inserts are widely used

in practice.

Basics, Deﬁnitions and Cutting Tool Geometry 403

In operation, “wiper” technology is designed to improve surface ﬁnishes in milling

applications. It works by positioning an insert with a ﬂat, just-below-the-other ordinary

parallel land inserts on a face mill. As the “wiper” passes through the cut, it smoothens

the surface. It allows using much higher feed rate without compromising surface ﬁnish

of the machined areas.

Example 1

Problem: Find the uncut chip area for the major and minor cutting edges (assuming

= 0

◦

) when feed f = 0.3mm/rev, depth of cut d

= 2 mm for tools having the

cutting edge angle κ

= 45

◦

and κ

= 85

◦

Solution: Using Eq. (A4), we can calculate the uncut chip area of the major cutting

edge for the ﬁrst and second tools because the depth of cut and the cutting feed are the

same for both the tools

= f × d

= 0.3 ×2 = 0.6mm

Using Eq. (A25), we can calculate the maximum uncut chip area for the minor cut-

ting edge

For the ﬁrst tool

= 0.5f

tan κ

= 0.5 ×0.3

tan 45

◦

= 0.045 mm

For the second tool

= 0.5f

tan κ

= 0.5 ×0.3

tan 85

◦

= 0.515 mm

As shown, the uncut chip area for the minor cutting edge for the second tool is almost

the same as that for the major cutting edge. Therefore, the cutting forces generated due

to the minor cutting edge cannot be ignored because the cross-sectional area of the uncut

chip for this edge is almost the same like the major cutting edge. Moreover, since this

edge is not meant to cut any signiﬁcant amount of the work material, its cutting geometry

(the rake and the ﬂank angles) are not selected to perform efﬁcient cutting, so the cutting

forces generated by this edge are normally much higher than those generated by the

major cutting edge under the same conditions. The problem gets worsened when the

depth of cut decreases.

A3.2 Rake angle

Rake angles are three types, positive, zero (sometimes referred to as neutral) and negative,

as shown in Figs. A8(a)–(c), respectively. It is generally accepted that the increase in

the rake angle reduces the horsepower consumption per unit volume of the layer being

removed as 1% per degree starting from γ =−20

◦

. As a result, the cutting force and

tool–chip contact temperature change in approximately the same way. So, it seems to be

reasonable to select a high positive rake angle for practical cutting operations. Everyday

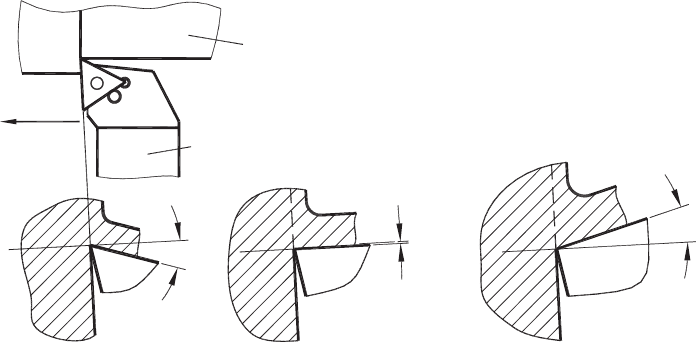
404 Tribology of Metal Cutting

Workpiece

Tool

−

g = 0°

(a) (b) (c)

Fig. A8. (a) Positive, (b) zero and (c) negative rake angles.

machining practice, however, shows that there are number of drawbacks in increasing

the rake angle.

The main drawback is that the strength of the cutting wedge decreases when the rake

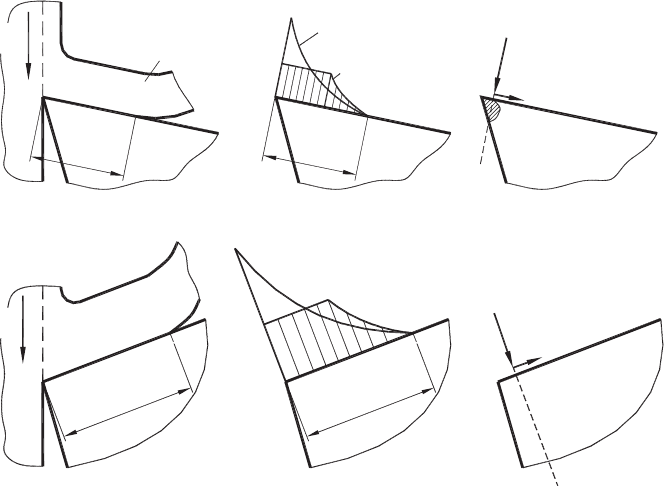
angle increases. The reason for that is illustrated in Fig. A9. When cutting with a positive

rake angle, as shown in Fig. A9(a), the interaction of the tool rake face with the moving

chip results in certain distributions of the normal and tangential stresses over the contact

length l

. As discussed in Chapter 3, these distributions can be represented in terms

of the corresponding resultant normal N and tangential F forces acting on the cutting

wedge. As shown, the normal force N causes the bending of the tip of the cutting

wedge. The presence of bending signiﬁcantly reduces the strength of the cutting wedge

causing its chipping. Moreover, the tool–chip contact area reduces with the rake angle,

so the point of application of the normal force shifts closer to the cutting edge. On the

contrary, when cutting with a tool having a negative rake angle (Fig. A9(b)), the normal

force N acting on the tool rake face does not cause the bending mentioned. Instead,

it results in the compression of the tool material. Because hard tool materials have very

high compressive strength, the strength of the cutting edge in this case is much higher

although the normal force N is greater than that for tool with positive rake angles. This is

particularly true when superhard tool materials (for example, Poly-Crystalline Diamond

(PCD) and Cubic Boron Nitride (CBN)) are used in interrupted and hard turning. It has

to be mentioned, however, that rapid development of PCD and CBN materials led to

their increased bending strength, so tools with positive geometries are used in machining

of high-silicon aluminum alloys in the automotive industry even in interrupted cutting

operations.

Another important drawback is that the region of the maximum contact temperature at

the tool–chip interface shifts toward the cutting edge when the rake angle is increased,

which lowers the tool life, as discussed in Chapter 3.

Basics, Deﬁnitions and Cutting Tool Geometry 405

Chip

Cutting

direction

max

Normal contact stress

Tangential contact stress

Tool

(a)

(b)

Cutting

direction

Fig. A9. Forces acting on the cutting edge having: (a) positive rake angle and (b) negative rake

angle.

Realistically, the rake angle is not an independent variable in the tool geometry selection

process because the effect of rake angle depends upon other parameters of the cutting

tool geometry and the cutting process. Moreover, the necessity of applying chip breakers

of different shapes often dictates the resulting rake angle rather than other parameters of

the cutting process like tool life, power consumption and cutting force.

A3.3 Flank angle

If the Flank (relief) angle α = 0

◦

, then the ﬂank surface of the cutting tool is in full

contact with the workpiece. As such, due to spring-back of the workpiece material, there

is signiﬁcant friction force in such a contact that leads usually to tool breakage. The ﬂank

angle affects the performance of the cutting tool mainly through decreasing the rubbing

on the tool’s ﬂank surfaces. When the uncut chip thickness is small (less than 0.02 mm),

this angle should be in the range of 30–35

◦

to achieve maximum tool life.

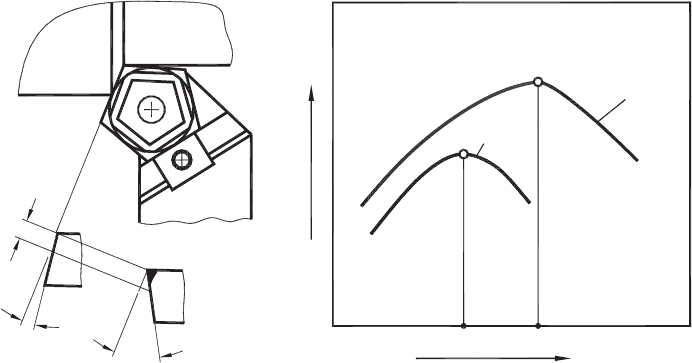
The ﬂank angle directly affects the tool life. When angle α increases, wedge angle β

decreases, as shown from Fig. A4. As such, the strength of the region adjacent to the

cutting edge decreases as well as heat dissipates through the tool. These factors lower

the tool life. On the other hand, by increasing the ﬂank angle the following advantages

are obtained:

406 Tribology of Metal Cutting

8°

30°

1−2

> t

1−1

Flank Angle

Tool Life

1−1

1−2

(a) (b)

Fig. A10. Inﬂuence of ﬂank angle: (a) the difference in the worn tool materials under the same

ﬂank wear for different ﬂank angles and (b) on tool life.

The cutting edge radius decreases with the ﬂank angle that leads to corresponding

decrease in the frictional and deformation components of the ﬂank force. This effect

becomes noticeable in cutting with small feeds. As a result, less heat is generated that

leads to an increase in tool life.

As the ﬂank angle becomes larger, more tool material should be removed (worn out)

to reach the same ﬂank wear as shown in Fig. A10(a). As a result, the tool life

increases.

Due to such contradictive effects, the inﬂuence of ﬂank angle on tool life always has a

well-deﬁned maximum, as shown in Fig. A10(b).

One has to remember the most important rule in assigning the ﬂank angle which reads:

“The actual ﬂank angle should not be less than 3

◦

” (exceptions are some form of tools

such as broaching tools, gear hobs and shapers, shaving tools, etc.). Otherwise the

normal cutting operation can be hardly performed due to vibrations and excessive tool

wear. Therefore, it is very important to estimate the actual ﬂank angle(s) accounting

for the tool design, its installation in a machine, its working conditions including the

direction of the prime and feed motions. One should also remember that in machining

difﬁcult-to-machine materials, a 2

◦

change in the ﬂank angle might change the tool life

by twice. The same can be said about the integrity of machined surfaces.

If the actual ﬂank angle is close to 0

◦

or negative, the interference of the tool ﬂank surface

and the workpiece takes place, i.e. the tool proﬁle runs into the workpiece proﬁle. In the

theory of proﬁle generation by cutting tools, the interference of the ﬁrst, second and third