Astakhov V. Tribology of Metal Cutting
Подождите немного. Документ загружается.

Generalized Model of Chip Formation 67
[91] Chandrashekhar, S., Sankar, T.S., Osman, M.O.M., A stochastic characterization of the
tool-workpiece system in BTA deep hole machining. Part 1: Mathematical modeling and
analysis, Adv. Manuf. Process. J., 2 (1987), 37–48.
[92] Mills, B., Redford, A.H., Machinability of Engineering Materials, Applied Science
Publishers, London, 1983.
[93] Trent, E.M., Metal Cutting, Butterworth Heinemann, London, Inglaterra, 1991.
[94] Ekinovic, S., Dolinsek, S., Brdarevic, S., Kopac, J., Chip formation process and some
particularities of high-speed milling of steel materials. In Trends in the Development of
Machinery and Associated Technology, TMT 2002, B&H, Neum, 2002.
[95] Tonshoff, H.K., Amor, P.B., Amdrae, P., Chip formation in high speed cutting (HSC), SME
Paper MR99–253, 1999.
[96] Talantov, N.V., Unstable plastic deformation and self-exited vibrations in metal cutting
(in Russian) in Metalworking Technology and Automatization of Manufacturing Processes,
N.V. Talantov, Editor. VPI, Volgograd, 1984, pp. 37–41.
[97] Lindberg, B., Lindstrom, B., Measurements of the segmentation frequency in the chip
formation process, Annals of the CIRP, 32 (1983), 17–20.
[98] Press, W.H., Flannery, B.P., Teukolsky, S.A., Vetterling, W.T., Power spectra estimation
using the FFT, in Numerical Recipes in FORTRAN: The Art of Scientific Computing,
Second Edition. Cambridge University Press, Cambridge, UK, 1992, pp. 542–551.
[99] Astakhov, V.P., Shvets, S.V., A novel approach to operating force evaluation in high
strain rate metal-deforming technological processes, Journal of the Materials Processing
Technology, 117 (2001), 226–237.
[100] Nakayama, K., Formation of saw tooth chips. In International Conference on Production
Engineering, Tokyo, 1974.
[101] Kishawy, M.A., Elbestawi, M.A., Effect of process parameters on chip morphology when
machining hardened steel. In Int. Mech. Eng. Congress and Exposition, Manufacturing
Science and Technology, ASME, Dallas, Texas, 1999.
[102] Elbestawi, M.A., Srivastawa, S.A.K., El-Wardany, T.I., Model for chip formation during
machining of hardened steel, Annals of the CIRP, 45 (1996), 71–76.
[103] Grady, D.E., Kipp, M.E., The growth of unstable thermoplastic shear with application to
steady-wave shock compression in solids, Journal of Mechanics and Physics of Solids, 35
(1987), 95–118.
[104] Komanduri, R., Brown, R.H., On the mechanism of chip segmentation in machining, ASME
Journal of Engineering for Industry, 103 (1981), 33–51.
This Page Intentionally Left Blank
CHAPTER 2
Energy Partition in the Cutting System
2.1 Introduction
As pointed out by Bhushan [1], tribology is the art of applying operational analysis to the
problems of great economic significance. Surface interactions in tribological interfaces
are highly complex, and their understanding requires knowledge of various disciplines
including physics, chemistry, applied mathematics, solid mechanics, fluid mechanics,
thermodynamics, heat transfer, materials science, rheology, lubrication, machine design,
performance and reliability. Understanding the tribological interactions discussed can
only be possible if the energy involved is known, because any interaction should be
thought of as a kind of energy exchange. The amount of energy transmitted through a
tribological interface defines to a large extent the actual occurrence of various physical
and chemical processes that might happen at this interface because any of these processes
requires a certain level of energy to trigger and maintain this process.
As discussed in Chapter 1, the cutting process takes place in the cutting system consisting
of the cutting tool, workpiece and chip [2]. The major system properties, such as the
system time and the dynamic interaction between the system components were used to
reveal the essential characteristics of the cutting process. Besides, it is necessary to point
out that the cutting system is a sub-system of a more general system defined as the
machining system.
The machining system includes a number of sub-systems such as the machine tool, the
control system, the coolant supply system, the loading–unloading system, etc. The main
objective of the machining system is to provide optimum conditions for the performance
of the cutting system because the quality of the machine part and the efficiency of
machining are determined by the performance of the cutting edge of the cutting tool(s).
Therefore, the system interactions between the sub-systems of the machining system and
the cutting system should be established, optimized and maintained to achieve optimum
performance of the cutting system. The most general level of such interactions is their
energy levels, so that these interactions can be thought of as the energy flows in the
machining system.
69
70 Tribology of Metal Cutting
This chapter clarifies the energy aspects of the metal cutting tribology. It considers a
complete model of energy partition and flows in the metal cutting system for the first
time. It introduces the concept of physical efficiency of the cutting system and presents a
simple way for its determination. It also reveals the significance of the chip compression
ratio in the study and optimization of the cutting processes. It discusses distinctive ways
of increasing the physical efficiency of the cutting system.
2.2 Energy Flows in the Cutting System
Generalization of the theoretical and experimental results on the performance of the
cutting system allows the graphical representation of energy flows and conversions in
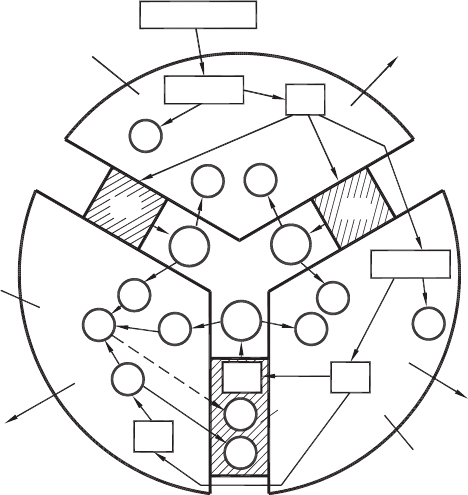
the cutting system, as shown in Fig. 2.1 [3]. The total energy entering the cutting system,
U
cs
is a part of the energy produced by the drive motor, U
em
. Obviously that
U
cs
= U
em
η
pt
, (2.1)
where η
pt
is the efficiency of the power train that connects the cutting system and the
drive motor of the machine tool.
U
cs
=U
em
.h
pt
W
Tc
+W
Tb
W
ta
Q
2
Q
3
Q
ta
Q
tg
W
tg
U
T
Q
1
Q
4
Q
tc
Q
fc
Q
fw
W
cc
+W
cb
Q
6
U
c
W
f
Q
wr
Q
wf
U
w
Q
5
Q
tw
Q
w
Q
eT
Q
e
c
Q
ew
TOOL
CHIP
WORKPIECE
U
f
Fig. 2.1. Graphical representation of energy flows in the metal cutting system (after Astakhov [3]).
Energy Partition in the Cutting System 71
Ideally, if there is no energy loss in the cutting system, the energy transmitted through
the cutting tool having the cutting tool energy U
T
to the chip with the chip energy
U
c
and then to the chip formation zone, having the deformation energy W
f
is equal to
the energy needed for the separation of the layer being removed, U
f
[4], i.e. U
cs
=
U
T
= U
c
= W
f
= U
f
. In real cutting systems, however, the energy losses occur due
to elastic and plastic deformations of their components as well as friction losses during
various interactions of these components. These losses do not correlate directly with the
separation of the layer being removed. These energy losses are converted into heat (or
the thermal energy), which, in turn, affect these losses even further.
2.2.1 Cutting tool
Consider the energy flows in the components of the cutting system. The first component
is the cutting tool. According to the energy conservation law, the work done over com-
pression (W
Tc
) and bending (W
Tb
) of the cutting tool transforms into its potential energy
(U
T
) and also is partially spent on internal friction during deformations, which results in
heat generation (Q
1
). Since the cutting tool is normally subjected only to elastic defor-
mation, Q
1
is small. However, when the cutting tool is not rigid and/or when it vibrates
with an appreciable amplitude during machining, the share of this thermal energy may
become significant.
The potential energy U
T
is then spent as the work done by the frictional forces on the
tool–chip and tool–workpiece interfaces, W
tα
and W
tγ
, respectively, and as the work
done over the chip. The works of the frictional forces, W
tα
and W
tγ
, convert into the
thermal energies, Q
2
and Q
3
, respectively. The thermal energy Q
3
is generated at the
tool–chip interface. It is then conducted into the tool, Q
tγ
, and into the chip, Q
tc
.
The portions of heat conducted into the tool and the chip are in inverse proportion to
their thermal resistances. Similarly, the thermal energy generated at the tool flank(s)–
workpiece interface (Q
2
) is then distributed between the tool (Q
tα
) and the workpiece
(Q
tw
) in inverse proportion to their thermal resistances. In the current consideration,
the notion of thermal resistance is used as being more general compared to simple
thermoconductivity because the cutting system is so dynamic that a number of system
parameters contribute to the thermal resistances of its components, which are in relative
motion with respect to each other.
Because the directions of heat flow are as shown in Fig. 2.1, the tool contact surfaces
(the tool–chip and tool–workpiece interfaces) always have higher temperatures than the
rest of the cutting tool. The temperature gradient decreases with the distance from the
tool rake face. As a result, the thermal energy flows in the direction of the rake face until
the temperature gradient becomes zero. The same can be said about the heat flow from
the tool flank contact surface. The exception is a small region adjacent to the cutting
edge. Here, with the decreasing distance between the tool rake and flank surfaces, it
can be assumed that the power of one heat source (acting at the rake or flank) exceeds
that of the other. As such, heat would flow from the tool into the workpiece or the chip
whichever heat source is weaker. In reality, however, it does not happen because the
friction conditions on the tool rake and flank surfaces as well as their temperatures in the
region adjacent to the cutting edge are balanced so that the temperature gradient is zero.
72 Tribology of Metal Cutting
Therefore, it can be concluded that the thermal energy flows only into the cutting tool
from the tool–chip and tool–workpiece interfaces. The other surfaces of the cutting tool
dissipate the thermal energy into the environment (Q
eT
).
As explained in Chapter 1, the working part of the cutting tool is referred to as the
cutting wedge. This is a part of the cutting tool enclosed between the tool rake and
flank contact surfaces, which intersect to form the cutting edge. This cutting wedge is
under the action of stresses applied on the tool–chip and tool–workpiece contact surfaces.
Moreover, due to the thermal energy that flows into the cutting tool, this wedge has a high
temperature. As a result, gradual exhaustion of the resource of the tool material takes
place as explained in Chapter 4 [5,6]. Besides, high temperature due to this heat flow
causes the plastic deformation of this wedge that appears as plastic lowering of the cutting
edge. Such a deformation should be regarded as high-temperature creep (Chapter 4).
2.2.2 Chip
The potential energy of the cutting tool is spent on the deformation of the chip. As dis-
cussed in Chapter 1, the chip serves as a lever to transmit the applied load into the
chip formation zone [2,4,7]. A part of the work done by the compressive force and the
bending moment, W
cc
+ W
cb
over the chip, is dissipated in the chip converting into
heat Q
6
. The other part is the potential energy of the chip (U
c
) that makes its con-
tribution W
f
to the formation of the fracture energy (U
f
), which includes the energy
needed for the formation of new surfaces. As discussed above, a part Q
tc
of the thermal
energy Q
3
generated at the tool–chip interface is also conducted into the chip. Besides,
the chip also receives Q
fc
, a certain part of the thermal energy generated in the chip
formation zone due to the plastic deformation of the layer being removed and heat due
to fracture (Q
4
).
The thermal energy (heat) Q
tc
from the chip interface flows into the chip primarily
due to thermal conductivity and partially due to mass transfer because the chip moves
over the tool–chip interface. Heat Q
fc
enters the chip due to mass transfer because
the velocity of chip is normally higher than that of heat conduction in the chip [2].
In other words, the heat generated in the conversion of the layer being removed into
the chip is transferred by the mass of the moving chip from the deformation zone, and
not due to its thermal conductivity. As a result, the moving mass of the layer being
removed is converted into the chip and the moving chip changes its energy along the
tool–chip interface. This is true for each chip formation cycle. The chip temperature
results in its higher plasticity and thus the energy losses in the transmission of the
compressive force and bending moment into the chip formation zone increase which,
in turn, increases Q
6
. Additionally, some thermal energy (heat) Q
en–c
is released to the
environment.
2.2.3 Workpiece
It was demonstrated in Chapter 1 that the surface of the maximum combined stress,
which eventually becomes the surface of fracture or chip separation, changes its position
Energy Partition in the Cutting System 73
within each chip formation cycle. Due to this fact, the energy of fracture of the layer
being removed is distributed over a certain volume of the work material causing plastic
deformation of this region, which normally extends below the surface that separates the
layer being removed and the rest of the workpiece. Obviously, some part of the potential
energy transferred into the chip formation zone is spent on the plastic deformation of this
region (U
w
). It is confirmed experimentally and manifests itself by the cold working of
the machined surface and results in machining the residual stress. As such, the thermal
energy (heat) Q
5
is released.
Besides heat Q
5
, heat Q
tw
from the tool flank–workpiece contact and heat Q
fw
from
the chip formation zone are also supplied into the workpiece forming the total thermal
energy Q
w
= Q
5
+ Q
tw
+ Q
fw
. As conclusively proven by Astakhov [8], this thermal
energy cannot increase the energy level in the fracturing of the layer being removed in
the direction of the cutting speed because the velocity of heat conduction is much lower
than the cutting speed. However, part of the thermal energy, transferred in the direction
of the feed motion, can change this energy level. This is explained later in this chapter.
A special case takes place in the machining of a workpiece of small diameter. As such,
heat Q
w
, having been reflected many times by the outer surface of the workpiece, affects
the energy level of its entire cross section, and thus is added to the energy of fracture U
f
.
Additionally, some heat, Q
en−w
, is released to the environment through convection,
conduction and radiation.
2.3 Physical Efficiency of the Cutting System
The word efficiency is a term used virtually everyday in a myriad of circumstances; thus,
it needs to be clearly defined for any specific usage. This term, if used in the design
of various technical systems, aims at obtaining the most effective engineering solutions
at the expense of multidimensional optimization of system model and the assurance
of the extremeness of one or several efficiency criteria in a wide range of operational
modes. It is also used in the definition of optimal control laws of the complex technical
systems for different operational modes; in the definition of optimum design solutions
regarding the complex criterion as “efficiency−cost”; in the definition of the set of
optimum engineering solutions, directed on efficiency increase of elements and of a
technical system as a whole, in the comparative analysis of alternative optimum versions
and substantiation of the final choice of technical solution.
Nowadays the word efficiency is associated with process economy rather than with its
physical nature. To distinguish efficiency as a techno-economic term and as a physically
based entity, the term physical efficiency will be used in further considerations. Physical
efficiency is defined in the classical way as a ratio of the useful energy provided by
the cutting system to the total energy required by this system. As such, every change
made in the cutting system would affect its efficiency in either way, hence the physical
efficiency discussed can be thought of as an objective gauge to judge this change made
in terms of the extent of improvement of the physical efficiency of the cutting system.
Unfortunately, today, specialists in metal cutting at different levels (from the research

74 Tribology of Metal Cutting
lab to the shop floor) do not have such a gauge. Therefore, in the author’s opinion, a
need is felt to develop such a practical and physically based objective gauge.
It is obvious that not all the energy required by the cutting system (U
cs
) is spent for the
separation of the layer being removed, i.e. for performing useful work U
f
. As discussed
above, a part of the energy spent in the cutting system is dissipated in the components
of this system changing their properties, and in the environment. As a result, the cutting
system consumes more energy than needed for the separation of the layer being removed.
It is clear that better the organization of the components of the cutting system, smaller
the difference between these two energies. Therefore, it appears to be reasonable to
introduce the term of physical efficiency of the cutting system as the ratio (expressed as
percentage) of the actual energy required for the separation of the layer being removed
and the total energy spent in the cutting system
η
cs
=
U
f
U
cs
(2.2)
The amount of energy required for separating a unit volume of the work material depends
on many factors. However, as demonstrated in [9], the state of stress, strain rate and
temperature are of prime importance. For a given cutting system, the system parameters,
including system geometry and regime, uniquely define the stress and strain at fracture
and thus U
f
.
In the design of practical cutting systems, achieving maximum efficiency may not be the
ultimate goal. Rather, its optimization should be considered. This is because one needs
to know the required time period of existence of the cutting system. In other words, for a
given tool material, the cutting wedge should be so shaped and the cutting regime should
be so selected that the tool wear rate is within the required limits. The latter condition
may be at odds with the requirement of maximum efficiency. Moreover, a particular
level of cutting system efficiency may be further corrected accounting for the required
quality, including the integrity of the machined surface, productivity and other practical
constraints.
As discussed in [2], the specific energy of fracture of the layer being removed is
determined under the state of stress in this layer as
U
f
=
ε
f
0
σ(ε)dε, (2.3)
where ε
f
is the strain at fracture under the state of stress imposed by the cutting wedge.
This strain is the major controlling parameter in metal cutting as it determines the energy
spent in cutting, tool life, chip shape, and many other important characteristics and
outcomes of the cutting process. The specific cutting energy required by the cutting

Energy Partition in the Cutting System 75
system can be determined as
U
cs
=
P
c
τ
ct
V
c
, (2.4)
where P
c
is the cutting power, τ
ct
is the machining time and V
c
is the volume of the
work material cut during time τ
ct
.
Because V
c
= fd
w
ντ
c
, where f is the cutting feed (m/rev), d
w
is the depth of the cut
(m), ν is the cutting speed (m/s); P
c
= F
z
ν, where F
z
is the power component of the
cutting force (N), the final expression for the physical efficiency of the cutting system
can be written as
η
cs
=
fd
w
F
z
ε
f
0
σ(ε)dε (2.5)
As follows from Eq. (2.5), the physical efficiency can be determined by knowing the
stress–strain curve of the work material (
ε
f
0
σ(ε)dε represents the area under the stress–
strain curve of the work material), cutting regime (f and d
w
), and by measuring the
cutting force F
z
.
Although Eq. (2.5) can be directly used to determine the physical efficiency of the cutting
system, there are at least two problems:
• The first and foremost is that the cutting force cannot be measured with reasonable
accuracy although this fact has never been honestly admitted by the specialists
in this field. To appreciate the issue, one should consider the results of the joint
program conducted by College International pour la Recherche en Productique–The
International Academy for Production Engineering, http://www.cirp.net (CIRP) and
National Institute of Standards and Technology (NIST) to measure the cutting force
in the simplest case of orthogonal cutting [10]. The experiments were carefully
prepared (the same batches of the workpiece (steel AISI 1045), tools, etc.) under
the supervision of NIST and replicated at four different most advanced metal cutting
laboratories in the world. Interestingly, although extraordinary care was taken while
performing these experiments, there was significant variation (up to 50%) in the
measured cutting force across these four laboratories. Obviously, such an accuracy
is not acceptable in the calculations of the physical efficiency of the cutting system.
• Second, many tool and cutting inserts manufacturers (not to mention manufactur-
ing companies), do not have adequate dynamometric equipment to measure the
cutting force and so it would be rather difficult to determine the cutting force for
the calculations discussed. Many dynamometers used in this field are not properly
calibrated because the known literature sources did not present proper experimental
methodology for cutting force measurements using piezoelectric dynamometers.
Therefore, to make practical calculations of the physical efficiency of the cutting system
another approach has to be found.

76 Tribology of Metal Cutting
2.4 Determination of the Work of Plastic Deformation in Metal Cutting
2.4.1 The known measures of plastic deformation
The concept of efficiency of the cutting system can be practical if there is a simple
measure of the work of plastic deformation in metal cutting, which could be used even
at the shop-floor level to estimate the efficiency of a given cutting process. The objective
of this section is to present such a measure.
As discussed earlier, in machining, the combined stress in the chip formation zone
exceeds the strength of the work material (under a given state of stress imposed by the
cutting tool), whereas other forming processes are performed by applying stress suffi-
cient to achieve the well-known shear flow stress in the deformation zone. The ultimate
objective of machining is to separate a certain layer from the rest of the workpiece with
minimum possible plastic deformation and thus the energy consumption. Therefore, the
energy spent on plastic deformation in machining must be considered as wasted. On the
other hand, any other metal-deforming process, especially those involving high strains
(e.g. deep drawing, extrusion) uses plastic deformation to accomplish the process. Parts
are formed into useful shapes such as tubes, rods and sheets by displacing the material
from one location to another [11]. Therefore, the better material, from the viewpoint of
metal forming, should exhibit higher strain before the fracture occurs. It is understood
that this is not the case in metal cutting where it is desired that the work material should
have as small a strain at fracture as possible. Unfortunately, this does not follow from the
traditional metal cutting theory which normally utilizes the shear strength or, at the best,
the shear-flow stress (this term was specially invented for metal cutting to cover up the
discrepancies between the theoretical and experimental results) to calculate the process
parameters (cutting force, temperatures, contact characteristics) although the everyday
machining practice shows that these parameters are lower in cutting brittle materials of
higher strength.
Historically, the chip compression ratio (CCR) (ζ) (or its reciprocal, the chip ratio),
which is determined as the ratio of the length of cut (L
l
) to the corresponding length of
the chip (L
c
) or the ratio of the chip thickness (t
2
) to the uncut chip thickness (t
1
), i.e.
ζ =
L
l
L
c
=
t
2
t
1
(2.6)
was introduced in the earlier studies on metal cutting as a measure of plastic deformation
of the work material in its transformation into the chip [2,12] (Fig. 2.2). Due to the
relative simplicity of its experimental determination, CCR was widely used in metal
cutting studies as a quantitative measure of the total plastic deformation [12]. Numerous
attempts have been made to establish analytically a relationship to predict CCR in terms
of the fundamental variables of the cutting process. However, none of these attempts has
produced results that match the experimentally obtained data for a reasonable variety of
input conditions. Later, researchers abandoned this route in favor of the “modern” metal
cutting approach in which this parameter is expected to be determined experimentally
[12–20]. Because CCR competes with shear strain for the role of a measure of plastic
