ASM Metals HandBook Vol. 8 - Mechanical Testing and Evaluation
Подождите немного. Документ загружается.

resolution, high-speed photography can be used to monitor sample strain during testing. The photographic data,
once digitized, can then be used to measure the actual instantaneous sample area as a function of time in the
sample for use in determining the true sample strain and strain rate. Finally, this data, when combined with the
transmitted wave data, can provide the means to calculate true stress in the sample.
References cited in this section
3. G.T. Gray III, W.R. Blumenthal, C.P. Trujillo, and R.W. Carpenter II, Influence of Temperature and
Strain Rate on the Mechanical Behavior of Adiprene L-100, J. Phys. (France) IV Colloq., C3
(EURODYMAT 97), Vol 7, 1997, p 523–528
13. S.M. Walley and J.E. Field, Strain Rate Sensitivity of Polymers in Compression from Low to High
Strain Rates, DYMAT J., Vol 1, 1994, p 211–228
15. G.T. Gray III, D.J. Idar, W.R. Blumenthal, C.M. Cady, and P.D. Peterson, High- and Low-Strain Rate
Compression Properties of Several Energetic Material Composites as a Function of Strain Rate and
Temperature, 11th Detonation Symposium, (Snow Mass, CO), J. Short, Ed., Amperstand Publishing,
2000
16. J.E. Field, S.M. Walley, N.K. Bourne, and J.M. Huntley, Review of Experimental Techniques for High
Rate Deformation Studies, Proc. Acoustics and Vibration Asia '98, 1998 (Singapore), p 9–38
17. G. Gary, J.R. Klepaczko, and H. Zhao, Generalization of Split Hopkinson Bar Technique to Use
Viscoelastic Materials, Int. J. Impact Eng., Vol 16, 1995, p 529–530
18. G. Gary, L. Rota, and H. Zhao, Testing Viscous Soft Materials at Medium and High Strain Rates,
Constitutive Relation in High/Very High Strain Rates, K. Kawata and J. Shioiri, Ed., Springer-Verlag,
Tokyo, 1996, p 25–32
21. L.L. Wang, K. Labibes, Z. Azari, and G. Pluvinage, On the Use of a Viscoelastic Bar in the Split
Hopkinson Bar Technique, Proc. Int. Symp. on Impact Eng., I. Maekawa, Ed., ISIE, Sendai, Japan,
1992, p 532–537
22. G. Gary, J.R. Klepaczko, and H. Zhao, Corrections for Wave Dispersion and Analysis of Small Strains
with Split Hopkinson Bar, Proc. Int. Symp. on Impact Eng., I. Maekawa, Ed., ISIE, Sendai, Japan, 1992,
p 73–78
23. L. Wang, K. Labibes, Z. Azari, and G. Pluvinage, Generalization of Split Hopkinson Bar Technique to
Use Viscoelastic Bars, Int. J. Impact Eng., Vol 15, 1994, p 669–686
24. H. Zhao and G. Gary, A Three Dimensional Analytical Solution of the Longitudinal Wave Propagation
in an Infinite Linear Viscoelastic Cylindrical Bar: Application to Experimental Techniques, J. Mech.
Phys. Solids, Vol 43, 1995, p 1335–1348
25. L. Wang, K. Labibes, Z. Azari, and G. Pluvinage, Authors Reply to “Generalization of Split Hopkinson
Bar Technique to Use Viscoelastic Bars,” Int. J. Impact Eng., Vol 16, 1995, p 530–531
26. G. Gary and H. Zhao, Inverse Methods for the Dynamic Study of Nonlinear Materials with a Split
Hopkinson Bar, IUTAM Symposium on Nonlinear Waves in Solids, J.L. Wegner and F.R. Norwood, Ed.,
American Society of Mechanical Engineers, 1995, p 185–189
27. O. Sawas, N.S. Brar, and A.C. Ramamurthy, High Strain Rate Characterization of Plastics Using
Polymeric Split Hopkinson Bar, Shock Compression of Condensed Matter—1995, S.C. Schmidt and
W.C. Tao, Ed., American Institute of Physics, Woodbury, NY, 1996, p 581–584

28. S. Rao, V.P.W. Shim, and S.E. Quah, Dynamic Mechanical Properties of Polyurethane Elastomers
Using a Nonmetallic Hopkinson Bar, J. Appl. Polym. Sci., Vol 66, 1997, p 619–631
29. H. Zhao, G. Gary, and J.R. Klepaczko, On the Use of a Viscoelastic Split Hopkinson Pressure Bar, Int.
J. Impact Eng., Vol 19, 1997, p 319–330
30. H. Zhao, Testing of Polymeric Foams at High and Medium Strain Rates, Polym. Test., Vol 16, 1997, p
507–516
31. H. Zhao, A Study of Specimen Thickness Effects in the Impact Tests on Polymers by Numeric
Simulations, Polymer, Vol 39, 1998, p 1103–1106
32. O. Sawas, N.S. Brar, and R.A. Brockman, High Strain Rate Characterization of Low-Density Low-
Strength Materials, Shock Compression of Condensed Matter—1997, S.C. Schmidt, D.P. Dandekar, and
J.W. Forbes, Ed., American Institute of Physics, Woodbury, NY, 1998, p 855–858
33. O. Sawas, N.S. Brar, and R.A. Brockman, Dynamic Characterization of Compliant Materials Using an
All-Polymeric Split Hopkinson Bar, Exp. Mech., Vol 38, 1998, p 204–210
34. P.S. Follansbee and C. Frantz, Wave Propagation in then SHPB, J. Eng. Mater. Technol. (Trans.
ASME), Vol 105, 1983, p 61–66
35. C. Bacon, An Experimental Method for Considering Dispersion and Attenuation in a Viscoelastic
Hopkinson Bar, Exp. Mech., Vol 38, 1998, p 242–249
36. C. Bacon, Separation of Waves Propagating in an Elastic or Viscoelastic Hopkinson Pressure Bar with
Three-Dimensional Effects, Int. J. Impact Eng., Vol 22, 1999, p 55–69
37. S.F. Wang and A.A. Ogale, Effects of Physical Aging on Dynamic Mechanical and Transient Properties
of Polyetheretherketone, Polym. Eng. Sci., Vol 29, 1989, p 1273–1278
38. W. Chen, B. Zhang, and M.J. Forrestal, A Split-Hopkinson Bar Technique for Low Impedance
Materials, Exp. Mech., Vol 39, 1999, p 1–5
39. G.T. Gray III, C.M. Cady, and W.R. Blumenthal, Influence of Temperature and Strain Rate on the
Constitutive Behavior of Teflon and Nylon, Plasticity 99: Constitutive and Damage Modeling of
Inelastic Deformation and Phase Transformation, A.S. Khan, Ed., Neat Press, Fulton, MD, 1998, p
955–958
Split-Hopkinson Pressure Bar Testing of Soft Materials
George T. (Rusty) Gray III and William R. Blumenthal, Los Alamos National Laboratory
Stress-State Equilibrium of Soft Materials
The stress-state equilibrium in a sample during testing in an SHPB can be checked by comparing the 1- and 2-
wave (or 3-wave) stress-strain response of the sample as described in the article “Classic Split-Hopkinson
Pressure Bar Testing” and illustrated in Fig. 2 (Ref 34, 40, 41, 42, and 43). Recent studies have documented
that both inertia and wave propagation effects during testing of a compression SHPB sample can significantly
affect the stress differences across the length of a specimen during loading (Ref 3, 15, 41, and 43).
Experimental results have revealed that the slow longitudinal sound speeds typical of some polymeric materials
make longitudinal stress equilibrium during SHPB testing difficult to achieve (Ref 13, 15, 18, and 44).
Assuming frictional effects on the stress state are insignificant, the validity of an SHPB test on polymeric
materials can be evaluated by examining the incident and transmitted pressure-bar data for stress-state
equilibrium in addition to assessing whether a constant strain-rate condition has been achieved during a test.
When the stress state is uniform throughout an SHPB sample, the 2-wave stress oscillates equally above and
below the 1-wave stress. In the 1-wave analysis, the sample stress is directly proportional to the bar strain
measured from the transmitted bar. This waveform characteristically exhibits low oscillation amplitude because
the deforming sample effectively damps much of the ringing inherent in the incident pulse as it propagates
through the sample. This damping can be quite significant in low-sound-speed materials such as polymers.
The 1-wave stress analysis represents the conditions at the sample transmitted bar interface and is referred to as
the sample “back stress.” In a 2-wave analysis, the sum of the synchronized incident and reflected bar
waveforms is proportional to the sample “front stress” and represents the conditions at the incident bar sample
interface. However, both the incident and reflected waveforms contain substantial inherent oscillations that give
rise to large uncertainty in the stress level, especially near the yield point, compared with the stress calculated
from the transmitted waveform. These harmonic oscillations are also subject to dispersion due to the wave-
speed dependence of different frequencies propagating through the pressure bars. This causes asynchronization
of the overlapped waveforms and, therefore, inaccuracy in the calculation of the front stress. Mathematical
methods have been developed to correct the primary mode of wave dispersion, and this dispersion correction
results in higher-accuracy calculations of the stress at low strains (Ref 34). Finally, a third stress-calculation
variation that considers the complete set of three measured bar waveforms, the 3-wave analysis, is simply the
average of the front and the back stress.
Figure 2 shows the 1-wave, 2-wave, and strain-rate data as a function of strain for an SHPB test conducted on
an Estane-based polymer binder sample. In this illustration, the front and back stress data reductions exhibit
substantially different responses up to moderate strain values, verifying that the sample did not attain a uniform
stress state until after a strain of 0.06. Attempts to achieve higher strain rates (>5000 s
-1
) at 25 °C (77 °F)
proved difficult to unsuccessful. For example, at a strain rate of 7000 s
-1
, the 1- and 2-wave signals diverged for
the entire test (invalidating the stress analysis as discussed previously). The data in Fig. 2 illustrate the
difficulty in testing polymeric materials possessing low impedance independent of whether a conventional
metallic SHPB or polymeric-pressure-bar SHPB setup is used. Achievement of stress-state equilibrium in the
sample is dependent on both the viscoelastic nature of the sample material and on sample geometry, which is
discussed subsequently.
Achievement of a constant strain rate in a polymer sample throughout an SHPB test serves as validation of the
careful balance of striker-bar length, striker-bar velocity, and tip material and thickness. In addition, a constant
strain rate is a further qualitative check of the attainment of stress equilibrium in the specimen.
References cited in this section
3. G.T. Gray III, W.R. Blumenthal, C.P. Trujillo, and R.W. Carpenter II, Influence of Temperature and
Strain Rate on the Mechanical Behavior of Adiprene L-100, J. Phys. (France) IV Colloq., C3
(EURODYMAT 97), Vol 7, 1997, p 523–528
13. S.M. Walley and J.E. Field, Strain Rate Sensitivity of Polymers in Compression from Low to High
Strain Rates, DYMAT J., Vol 1, 1994, p 211–228
15. G.T. Gray III, D.J. Idar, W.R. Blumenthal, C.M. Cady, and P.D. Peterson, High- and Low-Strain Rate
Compression Properties of Several Energetic Material Composites as a Function of Strain Rate and
Temperature, 11th Detonation Symposium, (Snow Mass, CO), J. Short, Ed., Amperstand Publishing,
2000
18. G. Gary, L. Rota, and H. Zhao, Testing Viscous Soft Materials at Medium and High Strain Rates,
Constitutive Relation in High/Very High Strain Rates, K. Kawata and J. Shioiri, Ed., Springer-Verlag,
Tokyo, 1996, p 25–32
34. P.S. Follansbee and C. Frantz, Wave Propagation in then SHPB, J. Eng. Mater. Technol. (Trans.
ASME), Vol 105, 1983, p 61–66

40. P.S. Follansbee, High Strain Rate Deformation of FCC Metals and Alloys, Metallurgical Applications
of Shock Wave and High Strain Rate Phenomena, L.E. Murr, K.P. Staudhammer, and M.A. Meyers,
Ed., Marcel Dekker, 1986, p 451–480
41. X.J. Wu and D.A. Gorham, Stress Equilibrium in the Split Hopkinson Pressure Bar Test, J. Phys.
(France) IV Colloq., C3 (EURODYMAT 97), Vol 7, 1997, p 91–96
42. G.T. Gray III, W.R. Blumenthal, C.P. Trujillo, and R.W. Carpenter, Influence of Temperature and
Strain Rate on the Mechanical Behavior of Adiprene L-100, J. Phys. (France) IV Colloq., C3
(EURODYMAT 97), 1997, p 523–528
43. G.T. Gray III, High-Strain-Rate Testing of Materials: The Split-Hopkinson Pressure Bar, Methods in
Materials Research, John Wiley Press, 2000
44. S.M. Walley, J.E. Field, and N.A. Safford, A Comparison of the High Strain Rate Behaviour in
Compression of Polymers at 300K and 100K, J. Phys. (France) IV Colloq., C3 (DYMAT 91), Vol 1,
1991, p 185–190
Split-Hopkinson Pressure Bar Testing of Soft Materials
George T. (Rusty) Gray III and William R. Blumenthal, Los Alamos National Laboratory
Sample-Size Effects
The sample thickness used in an SHPB test is constrained by the need to assure a uniform stress state for
accurate and reproducible constitutive data (Ref 3, 4, 38, and 45). Given the documented influence of
temperature on the elastic and plastic properties of ductile polymers, it is also important to evaluate sample
geometry effects over a range of temperatures (Ref 3).
The pronounced difference in the initial 1-, 2-, and 3-wave signals for several polymers has demonstrated the
importance of carefully assessing sample size to achieve a uniform uniaxial stress state in the sample (Ref 3,
15). Differences in the 1- and 2-wave stress levels for sample length/diameter (l/d) aspect ratio = 1 samples
(6.35 mm, or 0.25 in., thick) of a polyurethane elastomer (Adiprene [Uniroyal Chemical Company Inc.,
Middlebury, CT] L100) (Ref 3), polyamide (nylon), or Teflon (E.I. DuPont de Nemours & Co., Inc.,
Wilmington, DE) (Ref 39) indicate a sluggish ringup to stress-state equilibrium and, therefore, a marginally
valid Hopkinson bar test at strains <5%, even though a constant strain rate is indicated throughout the entire
test. Similar to the early findings of Kolsky (Ref 1) and more recent studies of Dioh and others (Ref 4, 45) and
Chen and others (Ref 38), the high-rate stress-strain response of polymeric samples exhibits pronounced
sample-size dependence. The 1-wave stress analysis for Adiprene L100 samples tested at ambient temperature
were found to depend on sample thickness as shown in Fig. 3. While the maximum flow stress attained for each
sample thickness was nominally the same for a strain of 10%, the details of the ringing up of the sample and the
falloff in flow stress after the maximum stress level was reached is seen to be significantly different. The 1 to 1
aspect ratio sample exhibits the most dispersive oscillatory ringup, as well as the most rapid falloff in flow
stress for the three sample thicknesses. The 3.2 mm (0.125 in.) thick sample (l/d = 0.5) exhibited a clear yield
strength and the lowest ringing amplitude. Chen and others (Ref 38) similarly observed substantial wave
attenuation in 6.34 mm (0.25 in.) thick RTV630 rubber samples compared with 1.54 mm (0.06 in.) thick
samples, which exhibited significantly faster ringup during testing.
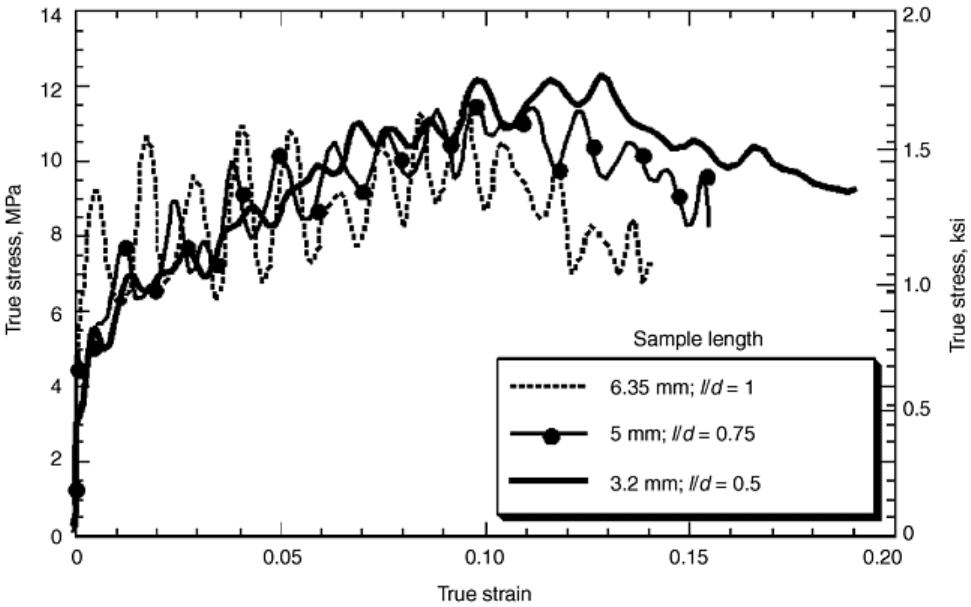
Fig. 3 Stress-strain response of 6.35 mm (0.25 in.) diam Adiprene L100 samples as a function of sample
length at high strain rate (2500 s
-1
)
The ambient temperature data in Fig. 3 for Adiprene L100 illustrates the need to use lower sample aspect ratios
when studying the entire high-strain-rate constitutive response of low-sound-speed dispersive materials such as
polymers. The measurements on Adiprene L100 (Ref 3) and RTV630 rubber (Ref 38) suggest that, depending
on the polymer and temperature range of testing, sample aspect ratios (l/d) of 0.5 or 0.25 can be effective in
minimizing wave attenuation while also keeping frictional effects under control. Another interesting
observation from Fig. 3 is the difference in the stress falloff as a function of sample thickness at strains over
10%. Taking into account that the lubrication conditions were the same for all samples, these results suggest
that the dynamic stress relaxation of Adiprene L100 is also a function of sample size. The sample aspect ratio
needed to achieve satisfactory uniaxial stress-state equilibrium also was found to be strongly dependent on the
test temperature. While an l/d = 0.5 sample facilitated achieving equilibrium at 25 °C (77 °F) on Adiprene
L100, samples with higher aspect ratios, such as l/d = 1, proved suitable at temperatures below approximately
240 K (-28 °F). These observations clearly demonstrate that achievement of stress equilibrium within polymeric
samples is influenced by their initial elastic properties. Further experiments also are needed to quantify the
stress-relaxation kinetics of viscoelastic materials as well as damage evolution processes in polymeric
composites (Ref 43). A novel approach to studying these processes is to compare finite-element modeling
(FEM) predictions for the bar outputs with actual bar outputs using proposed material models. This work is
described in detail later.
References cited in this section
1. H. Kolsky, An Investigation of the Mechanical Properties of Materials at Very High Rates of Loading,
Proc. Phys. Soc. (London) B, Vol 62, 1949, p 676–700
3. G.T. Gray III, W.R. Blumenthal, C.P. Trujillo, and R.W. Carpenter II, Influence of Temperature and
Strain Rate on the Mechanical Behavior of Adiprene L-100, J. Phys. (France) IV Colloq., C3
(EURODYMAT 97), Vol 7, 1997, p 523–528

4. N.N. Dioh, A. Ivankovic, P.S. Leevers, and J.G. Williams, The High Strain Rate Behaviour of
Polymers, J. Phys. (France) IV Colloq., C8 (DYMAT 94), Vol 4, 1994, p 119–124
15. G.T. Gray III, D.J. Idar, W.R. Blumenthal, C.M. Cady, and P.D. Peterson, High- and Low-Strain Rate
Compression Properties of Several Energetic Material Composites as a Function of Strain Rate and
Temperature, 11th Detonation Symposium, (Snow Mass, CO), J. Short, Ed., Amperstand Publishing,
2000
38. W. Chen, B. Zhang, and M.J. Forrestal, A Split-Hopkinson Bar Technique for Low Impedance
Materials, Exp. Mech., Vol 39, 1999, p 1–5
39. G.T. Gray III, C.M. Cady, and W.R. Blumenthal, Influence of Temperature and Strain Rate on the
Constitutive Behavior of Teflon and Nylon, Plasticity 99: Constitutive and Damage Modeling of
Inelastic Deformation and Phase Transformation, A.S. Khan, Ed., Neat Press, Fulton, MD, 1998, p
955–958
43. G.T. Gray III, High-Strain-Rate Testing of Materials: The Split-Hopkinson Pressure Bar, Methods in
Materials Research, John Wiley Press, 2000
45. N.N. Dioh, P.S. Leevers, and J.G. Williams, Thickness Effects in Split Hopkinson Pressure Bar Tests,
Polymer, Vol 34, 1993, p 4230–4234
Split-Hopkinson Pressure Bar Testing of Soft Materials
George T. (Rusty) Gray III and William R. Blumenthal, Los Alamos National Laboratory
Split-Hopkinson Bar Testing as a Function of Temperature
While numerous studies have investigated the influence of strain rate on the constitutive response of a range of
polymers, the influence of temperature at high strain rate on polymer mechanical behavior has been less
extensively researched (Ref 14, 44). This lack of data is in contradiction to the fact that robust constitutive
modeling descriptions of polymers used in structural applications must accurately describe polymer response as
a function of service temperature. Polymer and polymer-composite responses for temperatures from -40 to +40
°C (-40 to 105 °F) are relevant for arctic to desert service environments. Testing at temperatures below 25 °C
(77 °F) for a range of polymers has shown that both the measured “loading elastic” modulus and the measured
peak flow stresses increase with decreasing temperature (Ref 14, 44). Based on these results, it is apparent that
the construction of robust material models for polymeric constitutive behavior requires systematic knowledge
of the independent effects of temperature and strain rate.
Constant temperature conditions between 200 and 350 K (-100 and 170 °F) on a split-Hopkinson bar have been
achieved with the use of a specially designed gas manifold system developed at the Los Alamos National
Laboratory. Here, samples can be cooled and heated using helium gas within a type 304 stainless steel
containment chamber held at a partial vacuum, as shown in Fig. 4. Helium gas, due to its inertness and high
thermal conductivity, was selected as the heat-transfer medium to heat/cool a range of materials including
polymers, energetics, and propellant materials. The helium gas is cooled below ambient temperature by passing
the helium through a copper coil positioned within a liquid nitrogen dewar, while elevated temperatures are
achieved by heating the helium in a parallel coil within a glycerin-filled beaker warmed to approximately 450 K
(350 ° F) by a heating plate.
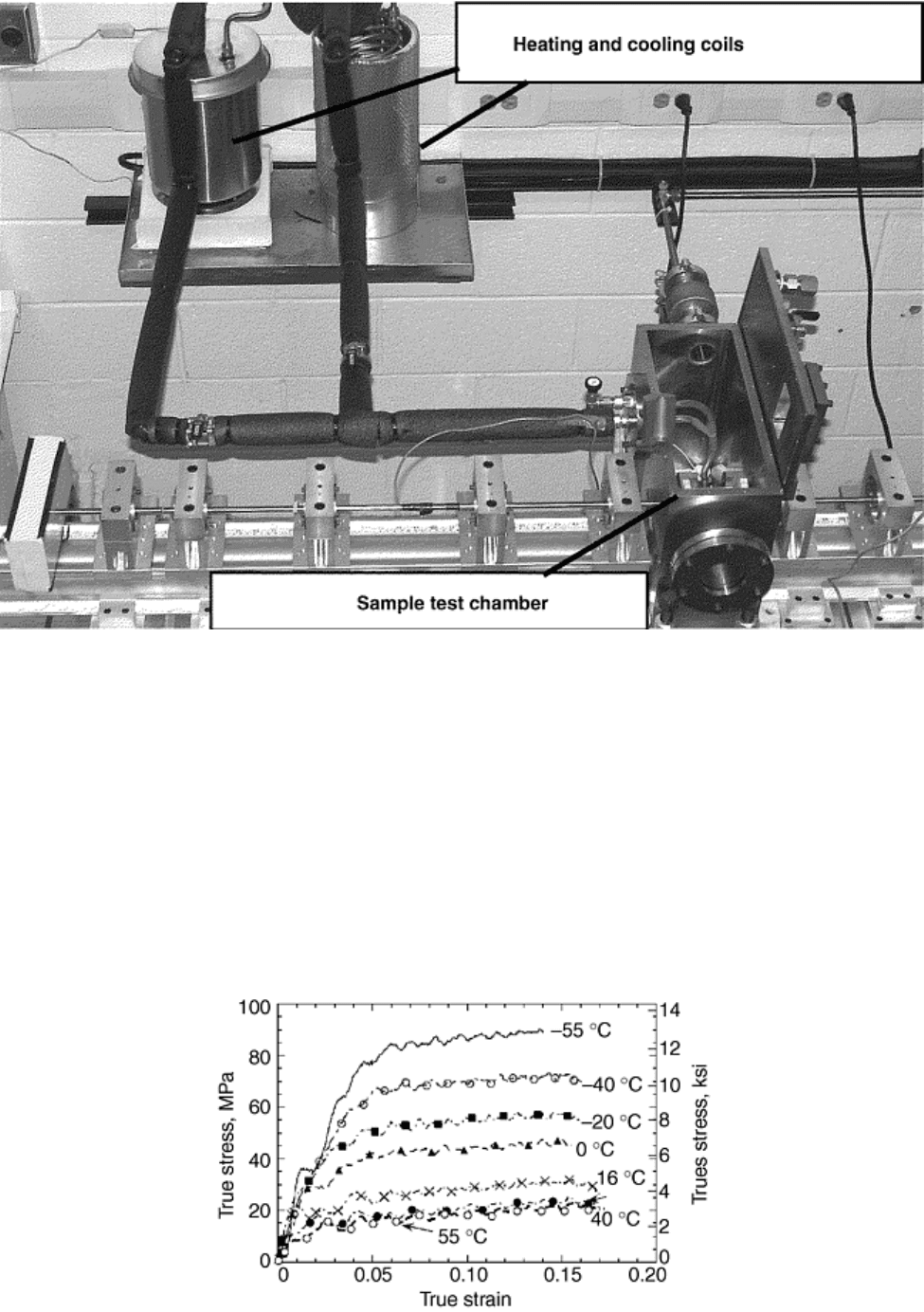
Fig. 4 Photograph of specialized split-Hopkinson pressure bar setup using either Ti-6Al-4V or
magnesium pressure bars and a helium-gas manifold heating/cooling system to allow controlled
temperature testing at high strain rates of polymers
Sample temperature in this heating/cooling scheme is monitored using a thermocouple positioned to lightly
contact the outside of the sample. Regulating the helium gas flow rate to the manifold surrounding the sample
allows fine temperature control. The heat transfer time required to heat or cool a 1 to 1 aspect ratio sample of
Adiprene L-100 was determined using a thermocouple inserted into the middle of a dummy sample. A time
duration of 5 min was determined to be necessary to equilibrate a polymer sample at temperature after attaining
a stable helium gas temperature within the manifold surrounding the sample. A similar procedure is being used
at Los Alamos National Laboratory for evaluating the temperature dependency of the high-strain-rate response
of a range of engineering polymers and energetic materials (Ref 39, 43). An example of the type of data that
can be measured for a polymer is shown in Fig. 5 for Teflon.
Fig. 5 Stress-strain response of Teflon at 2500 s
-1
as a function of temperature

To yield the highest fidelity stress measurements while still testing below the yield strength of the pressure bars
themselves using either Ti-6Al-4V or magnesium pressure bars and l/d = 0.5 aspect ratio samples, the high-
strain-rate response of Teflon as a function of test temperature from -55 to +55 °C (-65 to 130 °F) was
measured (Ref 39). The anelastic flow stress response (post-yield behavior) of Teflon is shown in Fig. 5 to
exhibit nominally parallel stress-strain curves as a function of temperature. This nominally constant
“hardening” behavior suggests that the deformation mechanisms controlling post-yield anelastic flow in Teflon
is not substantially temperature dependent, while its yield strength varies with temperature for a fixed loading
rate. Invariant strain hardening as a function of temperature is similar to the response of body-centered-cubic
(bcc) metals where a Peierls stress dominates yielding behavior. This suggests that a thermally activated
approach to modeling polymer constitutive behavior appears fruitful and demonstrates the importance of
studying temperature effects on polymer constitutive behavior.
References cited in this section
14. S.N. Kukureka and I.M. Hutchings, Yielding of Engineering Polymers at Strain Rates of up to 500 s
-1
,
Int. J. Mech. Sci., Vol 26, 1984, p 617–623
39. G.T. Gray III, C.M. Cady, and W.R. Blumenthal, Influence of Temperature and Strain Rate on the
Constitutive Behavior of Teflon and Nylon, Plasticity 99: Constitutive and Damage Modeling of
Inelastic Deformation and Phase Transformation, A.S. Khan, Ed., Neat Press, Fulton, MD, 1998, p
955–958
43. G.T. Gray III, High-Strain-Rate Testing of Materials: The Split-Hopkinson Pressure Bar, Methods in
Materials Research, John Wiley Press, 2000
44. S.M. Walley, J.E. Field, and N.A. Safford, A Comparison of the High Strain Rate Behaviour in
Compression of Polymers at 300K and 100K, J. Phys. (France) IV Colloq., C3 (DYMAT 91), Vol 1,
1991, p 185–190
Split-Hopkinson Pressure Bar Testing of Soft Materials
George T. (Rusty) Gray III and William R. Blumenthal, Los Alamos National Laboratory
Test Sample Preparation
Due to the soft, viscoelastic nature of some polymers and polymeric composites at ambient temperatures, a
special procedure has been adopted at Los Alamos National Laboratory and other facilities to machine SHPB
specimens with parallel loading surfaces within a tolerance of 0.03 mm (0.001 in.). First, the rough-cut
specimens are slowly cooled (<20 °C/min, or 36 °F/min) on a metal platen surrounded by liquid nitrogen. Then,
during machining, the polymeric specimens are sprayed with cold nitrogen gas from the vaporization of liquid
nitrogen to keep the sample in a hardened state well below the glass transition temperature. The increased
stiffness of the cooled samples significantly increases the ease of single-point turning of samples. Similarly, the
specimens must be slowly warmed back to ambient conditions to minimize thermal shock, residual stresses, and
specimen distortion.

Split-Hopkinson Pressure Bar Testing of Soft Materials
George T. (Rusty) Gray III and William R. Blumenthal, Los Alamos National Laboratory
Finite-Element Modeling (FEM) of the Split-Hopkinson Bar
In recent years, a number of new innovations have been demonstrated for the SHPB, and their value has been
demonstrated in improving both the accuracy and timeliness of high-rate testing measurements. One-, two-, and
three-dimensional FEMs of the SHPB have proved their ability to simulate test parameters and allow pretest
setup validation checks as an aid to planning. Finite-element models, therefore, offer a means to validate the
data and dispersion analysis used in the Hopkinson bar to probe for the effects of inertia and friction and to
provide an opportunity to extend the use of the Hopkinson bar. An example is the characterization of polymeric
materials for which a uniaxial stress state cannot be achieved. Previous research using this approach on soil
samples has shown the utility of a combined FEM/SHPB analysis (Ref 46).
Systematic FEM modeling of the SHPB with concurrent experimental SHPB measurements is a means to
extend the use of the SHPB technique beyond that of a conventional measurement apparatus (Ref 47). It can
afford the characterization of materials for which the SHPB technique was previously invalid using classic one-
dimensional data analysis, serving as a validation tool for complex sample geometries, and facilitating data
analysis and constitutive model development for sample materials exhibiting nonuniform and/or
nonconservative behaviors such as compaction, shear band formation, and fracture.
In the first instance, a coupled experimental and FEM approach can facilitate the high rate characterization of
materials for which the attainment of a stable uniaxial stress state during testing is problematic or impossible,
such as polymer foams. In the second case, FEM can provide a valuable tool to verify and validate sample
geometry design where equilibrium considerations are paramount, such as tensile SHPB specimens. Unlike the
compressive Hopkinson bar where right-regular sample shapes are most often used, tensile Hopkinson bar
testing uses complex sample geometries. During tensile Hopkinson bar testing, the signals measured in the
pressure bars record the structural response of the entire tensile sample, not just the gage section, where plastic
deformation is assumed to be occurring. Because the split-Hopkinson bar data analysis only provides data on
the relative displacement between the ends of the incident and transmitter bars, an effective gage length
generally must be used. Finite-element modeling coupled with high-speed photographic characterization of the
sample gage section as a function of time also may provide a technique to extract quantitative stress-strain
constitutive data from a tensile SHPB test, even in the presence of unavoidable strain gradients in the sample.
The time—resolved local measurement of strain in the sample gage length is also important to validate
modeling of the constitutive response of the sample material.
In the final instance, FEM can be used to examine the validity of constitutive and damage evolution models for
sample materials for which conventional Hopkinson-bar analysis is limited. Examples include the high-rate
deformation response of materials such as metallic and polymeric foams and honeycomb structures where
nonuniform compaction occurs, materials that undergo nonuniform plasticity such as shear banding and
kinking, and materials that undergo damage or fracture processes that invalidate the tacit SHPB data analysis
assumption of homogeneous deformation in the calculation of sample stress. Local strain quantification using
high-speed photographic techniques coupled with FEM modeling of “crush-up” densification could use SHPB
test data to validate the accuracy of the densification modeling scheme for a metallic foam. In this approach, the
stress-time histories for the incident and transmitted pressure bars are used as a validation check against FEM
simulations of the metallic foam response for a range of applied strain rates, sample geometries, and
temperatures.
The SHPB configuration can be modeled using a Lagrangian mesh as shown in Fig. 6 (Ref 47). In this example,
solid cylindrical lengths of steel, titanium, aluminum, or magnesium alloy can represent the striker, incident,
and transmitted bars. An axis of symmetry can be presumed about the longitudinal (length) axis of the pressure
bars, and as such, modeling of the pressure bars is performed assuming two-dimensional axisymmetry and
linear elasticity. The specimen, however, must be modeled using a three-dimensional Lagrangian mesh such
that nonuniform deformation processes within the sample, such as compaction in the case of foams, shear
banding, kinking, and so on, are to be physically described. Elemental and nodal quantities in the finite-element
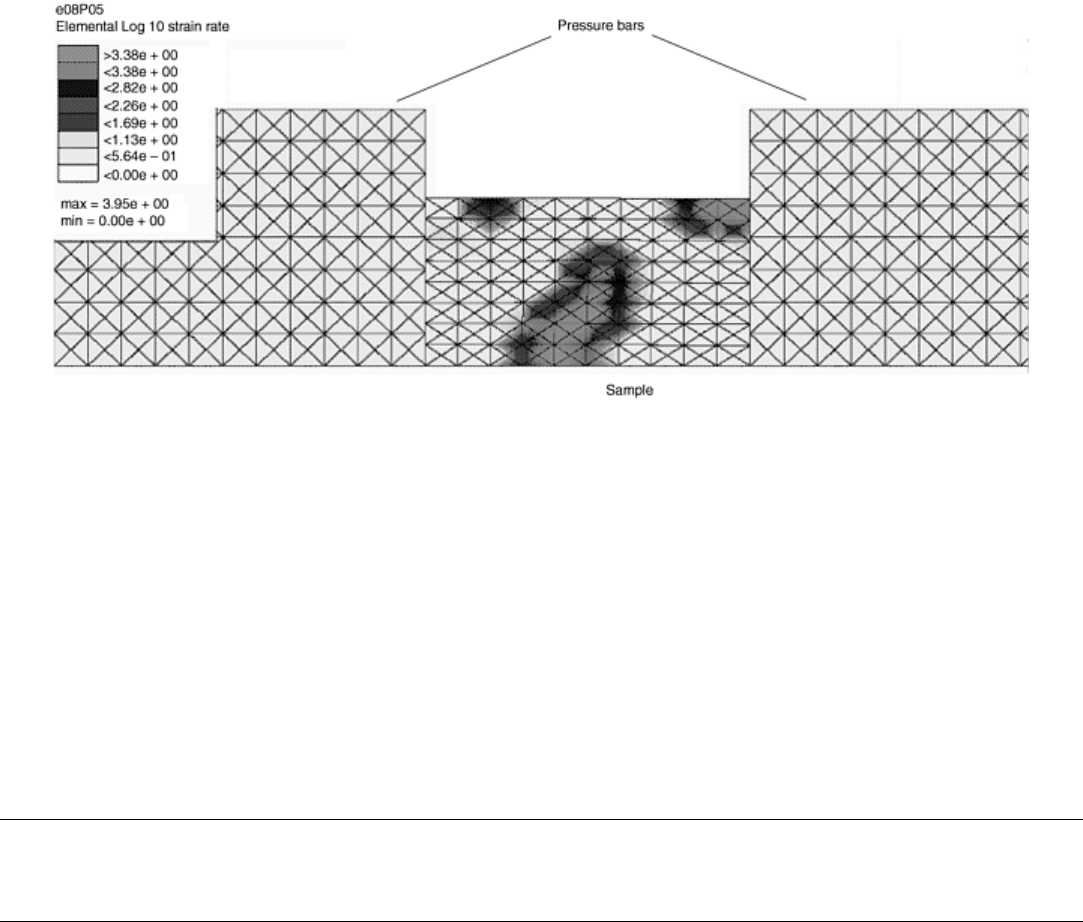
model are then recorded at various positions along the longitudinal (length) axis of the SHPB model. Figure 6
presents an FEM simulation snapshot plotting sample strain rate for an SHPB test of a Teflon sample using Ti-
6Al-4V pressure bars. The graphical data in Fig. 6 illustrate the nonuniformity in strain rate in a polymeric
sample during the initial stages of ringup prior to the achievement of a uniform uniaxial stress state in the
sample or a nominally constant strain rate of loading (Ref 47).
Fig. 6 Finite-element simulation of a Teflon. l/d = 1 sample in a split-Hopkinson pressure bar test. The
early stages of the ringup of the Teflon sample toward achieving a uniform uniaxial stress state is
reflected by the nonuniform strain rate as a function of position within the sample. Source: Ref 47
References cited in this section
46. C.W. Felice, E.S. Gaffney, and J.A. Brown, Extended Split-Hopkinson Bar Analysis for Attenuating
Materials, J. Eng. Mech., Vol 117, 1991, p 1119–1135
47. D.M. Goto, R.K. Garrett Jr., and G.T. Gray III, Finite Element Modeling of the Split-Hopkinson
Pressure Bar: Stress State Stability, Exp. Mech., in preparation
Split-Hopkinson Pressure Bar Testing of Soft Materials
George T. (Rusty) Gray III and William R. Blumenthal, Los Alamos National Laboratory
Summary
The Hopkinson pressure bar, as a research and engineering tool for the quantitative measurement of the high-
rate stress-strain behavior of soft materials, has undergone significant evolution in the past 5 years. Higher-
resolution measurements of the constitutive responses of viscoelastic solids under ambient conditions have been
developed using both polymeric and magnesium pressure bars compared with measurements with high-strength
steel bars. Due to their time- and temperature-dependent dispersive and dissipative nature, viscoelastic solids
pose unique challenges during Hopkinson bar testing. The most daunting of these challenges is the attainment
of stress-state equilibrium. Careful selection of sample geometry and the use of 1- and 2-wave stress-wave
analyses are crucial to verifying the attainment of a uniaxial stress state during Hopkinson bar testing. Finite-
element modeling of SHPB measurements coupled with experimental SHPB measurements offers great
promise as a means to extend the use of the SHPB technique beyond that of solely a measurement method.
Finite-element modeling of the SHPB can support the quantification of the constitutive behavior of materials,
which were previously invalid using only classic one-dimensional SHPB stress-wave analysis. These
