Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V

Подождите немного. Документ загружается.

78 Chapter 2

1. Type 1 or Dirichlet condition (vanishing of the zero derivative at the boundary)

y(a) = 0

2. Type 2 or Neumann condition (vanishing of the ﬁrst derivative at the boundary)

(a) = 0

3. Type 3 or Robin condition (linear dependence between zero and ﬁrst derivative at

boundary)

(a) +hy(a) = 0

The constant h in the Robin condition has the dimension of the reciprocal of displacement.

When we consider actual boundary value problems, we will be able to attach physical

signiﬁcance to each of these conditions. We now provide an illustration of a typical

Sturm-Liouville eigenvalue problem.

DEMONSTRATION: We seek the eigenvalues and corresponding eigenfunctions for the

Euler differential equation shown:

y(x) +λy(x) = 0

over the interval I ={x |0 <x<1} with the boundary conditions

(0) = 0

and

y(1) = 0

From our earlier classiﬁcation of boundary conditions, we have a type 2 condition at the left

and a type 1 condition at the right.

By comparison, we see the Euler differential equation to be a special case of a Sturm-Liouville

differential equation

L(y) +λ w(x)y = 0

where L is the Sturm-Liouville operator

L(y) = D(p(x)D(y)) +q(x)y

For the Euler differential equation, we identify the coefﬁcients p(x) = 1, q(x) = 0, and

w(x) = 1.

Sturm-Liouville Eigenvalue Problems and Generalized Fourier Series 79

SOLUTION: To solve for the eigenvalues, we consider three possible cases for values of λ.

We ﬁrst consider the case for λ<0. We set λ =−μ

. From Chapter 1, a set of basis vectors

for this case is

y1(x) = sinh(μx)

and

y2(x) = cosh(μ x)

and the general solution can be written in terms of this basis as

y(x) = C1 sinh(μ x) +C2 cosh(μ x)

Substituting the boundary conditions at x = 0 and at x = 1, we get

C1μ = 0

and

C2μ sinh(1) = 0

The only solution to the preceding is the trivial solution C1 = 0 and C2 = 0.

We next consider the case for λ = 0. A set of system basis vectors is

y1(x) = 1

and

y2(x) = x

and the general solution in terms of this basis is

y(x) = C1 +C2 x

Substituting the boundary condition at x = 0 and at x = 1 gives

C2 = 0

and

C1 +C2 = 0

The only solution to the preceding is the trivial solution C1 = 0 and C2 = 0. From a geometric

standpoint, we expected this result because it is impossible to have a nontrivial line that will

satisfy the boundary conditions at both the left and right end points of the interval.

80 Chapter 2

Finally, we consider the case for λ>0. We set λ = μ

. From Chapter 1, a set of basis vectors

for this case is

y1(x) = sin(μ x)

and

y2(x) = cos(μ x)

The general solution here is

y(x) = C1 sin(μx) +C2 cos(μ x)

Substituting the boundary conditions at x = 0 and at x = 1, we get

C1μ = 0

and

C1 sin(μ) +C2 cos(μ) = 0

The only nontrivial solutions to the preceding are that C1 = 0, C2 is arbitrary, and μ has values

that are the roots of the eigenvalue equation

cos(μ) = 0

Thus, μ takes on the special values

(2n −1)π

for n = 1, 2, 3,....

The allowed eigenvalues λ

= μ

are

(2n −1)

and the corresponding eigenfunctions are

(x) = cos



(2n −1)πx



for n = 1, 2, 3,....

These eigenfunctions are not normalized. We must ﬁrst ﬁnd the norm of each eigenfunction by

evaluating the square root of the inner product of the eigenfunctions with respect to the weight

function w(x) = 1 over the interval.

Sturm-Liouville Eigenvalue Problems and Generalized Fourier Series 81

The norm is

norm =









cos



(2n −1)πx



Evaluating this integral yields

norm =

√

Dividing each eigenfunction by its norm yields the orthonormal set of eigenfunctions

(x) =

√

2 cos



(2n −1)πx



for n = 1, 2, 3,....

The statement of orthonormality for this set of eigenfunctions reads



2 cos



(2n −1)πx



cos



(2m −1)πx



dx = δ(n, m)

for n, m = 1, 2, 3,....

2.4 The Generalized Fourier Series Expansion

Since the eigenfunctions form a “complete” set with respect to any piecewise smooth function

f(x) over the interval I ={x |a<x<b}, then we can expand f(x) as a generalized Fourier

series. In terms of the orthonormalized eigenfunctions, the generalized expansion formula for

f(x) reads

f(x) =

∞



n=0

F(n)ϕ

(x)

where the F (n) are the generalized Fourier coefﬁcients of the function f(x). We proceed to

evaluate these coefﬁcients in a formal manner by taking advantage of the statement of

orthonormality given earlier.

If we take the inner product of both sides of the preceding equation, with respect to the weight

function w(x) over the interval, and we assume it is valid to interchange the order of the

summation and integration operators (see exercises on conditions for this), then we get



f(x)ϕ

(x)w(x)dx =

∞



n=0

F(n)

⎛

⎝



(x)ϕ

(x)w(x)dx

⎞

⎠

82 Chapter 2

Because of the statement of orthonormality, we can write the integral on the right in terms of

the Kronecker delta function as



f(x)ϕ

(x)w(x)dx =

∞



n=0

F(n)δ(n, m)

The sum on the right is easy to evaluate, since δ(n, m) is 0 for n = m and 1 for n = m.

Evaluating the sum (only one term survives), we get

F(m) =



f(x)ϕ

(x)w(x)dx

Thus, we see that the Fourier coefﬁcients are evaluated from the inner product of f(x) and the

corresponding orthonormal eigenfunction, with respect to the weight function w(x), over the

interval I.

Convergence of the Fourier Series

Before we provide some examples, we ﬁrst consider some important aspects on the

convergence of a series. We consider two important questions dealing with the convergence of

the Fourier series: (1) Will the series converge? (2) To what value does the series converge?

Both of these questions are addressed by the following two theorems (from references, see

Berg and McGregor, page 157).

Theorem 2.4.1 (Pointwise convergence): Let f(x) be piecewise smooth on the closed interval

[a, b], and let the set {ϕ

(x)} be the eigenfunctions of a regular Sturm-Liouville problem over

that interval. Then for each value of x in the corresponding open interval (a, b), the Fourier

series, relative to these eigenfunctions, converges “pointwise” in accordance with

∞



n=0

F(n)ϕ

(x) =

f(x +0) +f(x −0)

Here, the F(n) are the properly evaluated Fourier coefﬁcients over the interval, and the term on

the right is the average of the left- and right-hand limits of f(x) at the point. For piecewise

smooth functions, the quality of convergence of the series depends on the value of x in the

interval.

Theorem 2.4.2 (Uniform convergence): Let f(x) be deﬁned and continuous with continuous

ﬁrst and second derivatives on the closed interval [a, b], and let f(x) satisfy the same boundary

conditions as the eigenfunctions {ϕ

(x)}, which are the solutions to the regular Sturm-Liouville

problem over that interval. Then the Fourier series expansion of f(x), relative to these

eigenfunctions, converges “uniformly” to f(x) on the closed interval.

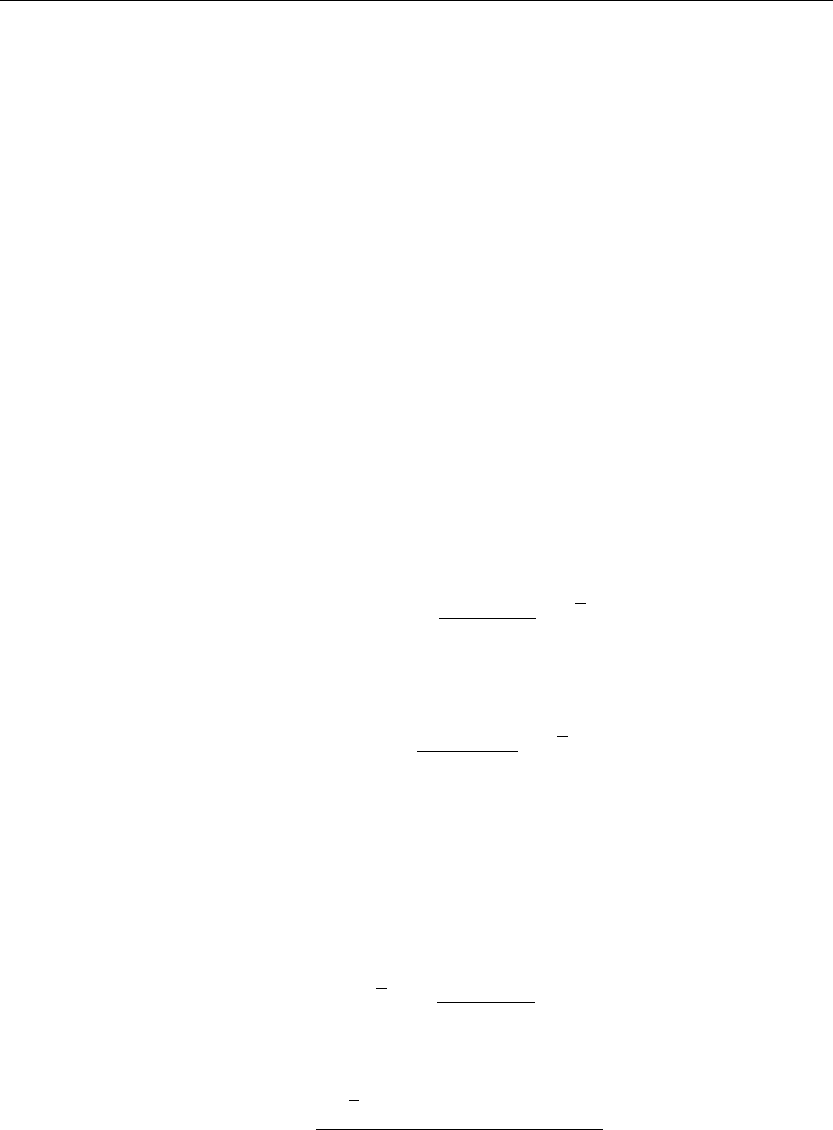
Sturm-Liouville Eigenvalue Problems and Generalized Fourier Series 83

Functions that satisfy the more rigid conditions for uniform convergence are more well

behaved and have Fourier series expansions that converge more rapidly and with better ﬁdelity

to the actual function. Uniform convergence obviously implies pointwise convergence at all

points on the closed interval, and, in addition, the convergence is independent of the value of x

on that interval. For more details on testing a series for uniform convergence, see Section 3.7,

which discusses the Weierstrass M-test for the uniform convergence of a series. For more

formal deﬁnitions of the concepts of pointwise and uniform convergence, please be advised

that these concepts are best discussed in more advanced texts in Fourier series (see references).

The more advanced concepts of periodic entensions and Gibb’s phenomena are best discussed

in these more advanced texts.

We now provide illustrations of Fourier series expansions of three different functions in terms

of those eigenfunctions derived in the problem in Section 2.3. This exercise demonstrates how

the quality of convergence of the resulting series depends on the character of the function and

how well it adheres to the boundary conditions on the problem.

DEMONSTRATION: We seek the generalized series expansion of a piecewise smooth

function f(x) over the interval I ={x |0 <x<1}, in terms of the orthonormal eigenfunctions

evaluated in Section 2.3.

SOLUTION: In terms of the evaluated orthonormal eigenfunctions, the expansion reads

f(x) =

∞



n=1

F(n) cos



(2n −1)πx



√

From the preceding, the Fourier coefﬁcients are determined from the integral

F(n) =



f(x) cos



(2 n −1)πx



√

2dx

We evaluate the series for three different functions f(x), each having a different character over

the interval.

Case 1 We let f 1(x) = x. For this case, f 1(x) does not satisfy either of the boundary

conditions at the left or right end points of the interval. The Fourier coefﬁcient integral reads

F(n) =



√

2 cos



(2n −1)πx



Evaluation of this integral yields

F(n) =−

√

2(2 π(−1)

n −π(−1)

+2)

(2 n −1)

(2.3)

84 Chapter 2

Thus, the Fourier series expansion of f 1(x) over the interval is

f 1(x) =

∞



n=1

⎛

⎝

−

4 (2 πn(−1)

−π(−1)

+2) cos



(2 n−1)πx



(2 n −1)

⎞

⎠

A plot of both f 1(x) and the ﬁrst ﬁve terms of the series expansion is shown in Figure 2.1.

0 0.2 0.4 0.6 0.8 1

0.2

0.4

0.6

0.8

Figure 2.1

Because the function f 1(x) does not satisfy either of the boundary conditions, the series

converges pointwise. The series converges very slowly, as an alternating

series, and the

quality of convergence appears to be poor.

Case 2 We let f 2(x) = 1 −x. For this case, f 2(x) does not satisfy the boundary condition

at the left, but it does satisfy the condition at the right end point of the interval. The Fourier

coefﬁcient integral reads

F(n) =



(1 −x)

√

2 cos



(2 n −1)πx



Evaluation of this integral yields

F(n) =

√

(2 n −1)

(2.4)

Thus, the Fourier series expansion of f 2(x) over the interval is

f 2(x) =

∞



n=1

8 cos



(2 n−1)πx



(2 n −1)

A plot of both f 2(x) and the ﬁrst ﬁve terms of the series expansion is shown in Figure 2.2.

Sturm-Liouville Eigenvalue Problems and Generalized Fourier Series 85

The function f 2(x) satisﬁes the boundary condition at the right but not at the left end point,

and the series converges pointwise. The series converges very rapidly as a

series, and, since

the function satisﬁes the boundary condition at one of the end points, the quality of

convergence appears to be much better than that for case 1.

Case 3 We let f 3(x) = 1 −x

. For this case, f 3(x) satisﬁes both boundary conditons at the

left and right end points of the interval. The Fourier coefﬁcient integral reads

F(n) =



(1 −x

)

√

2 cos



(2 n −1)πx



Evaluation of this integral yields

F(n) =

√

2(−1)

n+1

(2 n −1)

Thus, the Fourier series expansion of f 3(x) over the interval is

f 3(x) =

∞



n=1

32 (−1)

n+1

cos



(2 n−1)πx



(2 n −1)

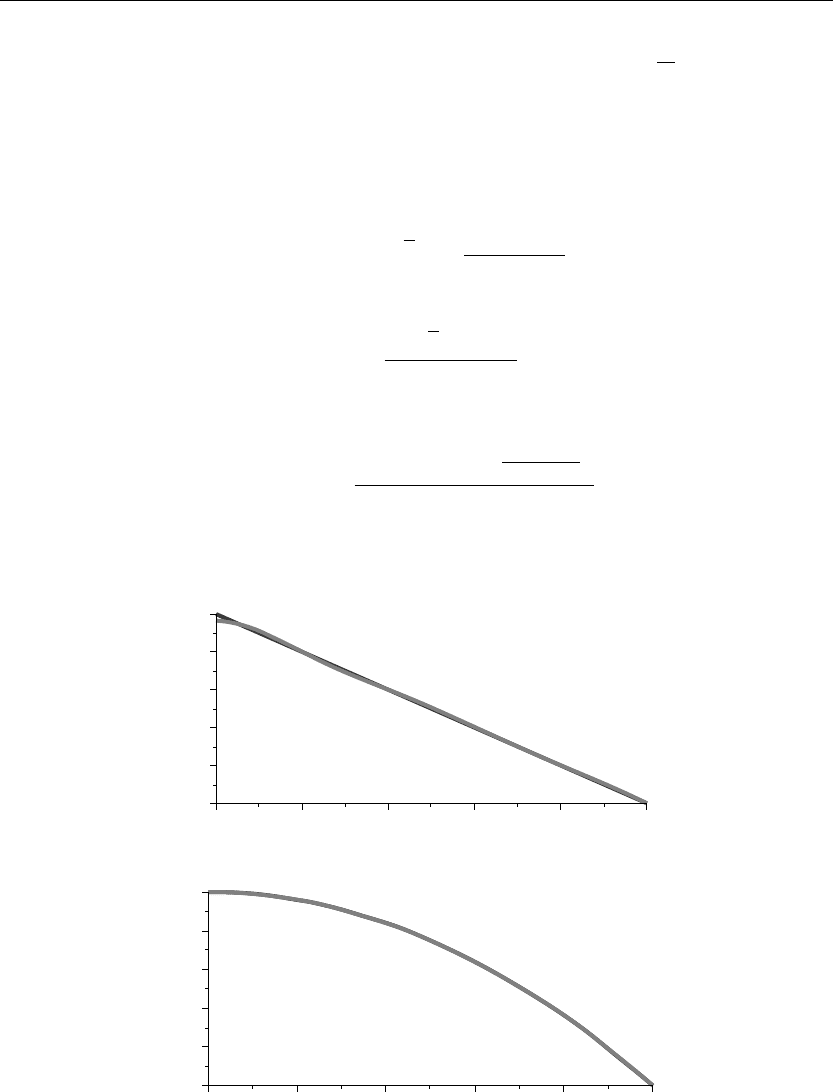
A plot of both f 3(x) and the ﬁrst ﬁve terms of the series expansion is shown in Figure 2.3.

0 0.2 0.4 0.6 0.8 1

0.2

0.4

0.6

0.8

Figure 2.2

0 0.2 0.4 0.6 0.8 1

0.2

0.4

0.6

0.8

Figure 2.3

86 Chapter 2

Because the function f 3(x) satisﬁes both of the boundary conditions at the left and right end

points, the series converges uniformly to f(x). The series converges very rapidly as a

series,

and the quality of convergence is considerably much better than either of the two cases already

discussed.

2.5 Examples of Regular Sturm-Liouville Eigenvalue Problems

We will now look at examples of regular Sturm-Liouville differential equations with various

combinations of the three types of boundary conditions discussed earlier. All of the examples

are special cases of the Sturm-Liouville differential equation

L(y) +λ w(x)y = 0

where L is the Sturm-Liouville operator

L(y) = D(p(x)D(y)) +q(x)y

We focus on three types of differential equations: Euler, Cauchy-Euler, and Bessel. Each one of

these differential equations is characterized by a different set of Sturm-Liouville coefﬁcients:

p(x), q(x), and w(x). Subject to a particular set of boundary conditions, we will generate the

eigenvalues, the corresponding eigenfunctions, and the statement of orthonormality. We will

then provide an example of a generalized Fourier series expansion of a given function in terms

of the particular eigenfunctions.

In solving for the allowed eigenvalues and corresponding eigenfunctions, we would ordinarily

consider three possibilities for values of λ: λ<0, λ = 0, and λ>0. However, to make our task

a little simpler, we will not consider the case for λ<0 because it can be shown, by way of the

Rayleigh quotient (see Exercises 2.24 through 2.26), that for the particular Sturm-Liouville

problems we will be considering, λ must be greater than or equal to zero.

EXAMPLE 2.5.1: Consider the Euler operator with Dirichlet conditions. We seek the

eigenvalues and corresponding orthonormal eigenfunctions for the Euler differential equation

[Sturm-Liouville type for p(x) = 1, q(x) = 0, w(x) = 1] over the interval I ={x|0 <x<b}.

The boundary conditions are type 1 at the left and type 1 at the right end points.

Euler differential equation

y(x) +λy(x) = 0

Boundary conditions

y(0) = 0 and y(b) = 0

Sturm-Liouville Eigenvalue Problems and Generalized Fourier Series 87

SOLUTION: We consider two possibilities for values of λ. We ﬁrst consider λ = 0. For this

case, the system basis vectors are

> restart:y1(x):=1;y2(x):=x;

y1(x) := 1

y2(x) := x (2.5)

General solution

> y(x):=C1*y1(x)+C2*y2(x);

y(x) := C1 +C2x (2.6)

Substitution into the boundary conditions yields

> eval(subs(x=0,y(x)))=0;

C1 = 0 (2.7)

> eval(subs(x=b,C1=0,y(x)))=0;

C2 b = 0 (2.8)

The only solution to the preceding is the trivial solution. We next consider λ>0. We set

λ = μ

and, for this case, the system basis vectors are

> y1(x):=sin(mu*x);y2(x):=cos(mu*x);

y1(x) := sin(μx)

y2(x) := cos(μx) (2.9)

General solution

> y(x):=C1*y1(x)+C2*y2(x);

y(x) := C1 sin(μx) +C2 cos(μx) (2.10)

Substituting into the boundary conditions, we get

> eval(subs(x=0,y(x)))=0;

C2 = 0 (2.11)

> eval(subs(x=b,y(x)))=0;

C1 sin(μb) +C2 cos(μb) = 0 (2.12)