Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V

Подождите немного. Документ загружается.

58 Chapter 1

> S02:=Sum(subs(n=n,simplify(a02(x)*c(n)*xˆ(n+r))),n=0..inﬁnity);

S02 :=

∞



n=0

(−m

c(n)x

n+r

) (1.179)

Adding all of the preceding, we get the homogeneous series equation

> S:=S2+S1+S01+S02=0;

S :=

∞



n=0

n+r

c(n)(n +r)(n +r −1) +

∞



n=0

n+r

c(n)(n +r) +

∞



n=2

n+r

c(n −2)

∞



n=0

(−m

c(n)x

n+r

) = 0 (1.180)

Note that the preceding series all have x terms raised to the same lowest power. We now take

steps to obtain a general summation term whose summing index n = 2 is the same for all

terms. Those terms that cannot be swept into the general summation give rise to residual terms,

which eventually give rise to what we call the “indicial” equations. Those terms remaining in

the generalized sum give rise to the “recursion” formula.

Extracting the ﬁrst two terms from the preceding ﬁrst, second, and fourth series, and sweeping

like terms under a general sum with the starting summation index n = 2, we get the ﬁnal

homogeneous series equation corresponding to the Bessel differential equation

> S:=c(0)*(r*r−1)+r−mˆ2)*xˆr+c(1)*(r*(r+1)+(r+1)−mˆ2)*xˆ(r+1)+Sum((c(n)*(n+r)*

(n+r−1)+c(n)*(n+r)+c(n−2)−c(n)*mˆ2)*xˆ(n+r),n=2..inﬁnity)=0;

S :=c(0)(r(r −1) +r −m

+c(1)(r(r +1) +r +1 −m

r+1

∞



n=2

(c(n)(n +r)(n +r −1)

+c(n)(n +r) +c(n −2) −c(n)m

n+r

= 0 (1.181)

The beginning terms that are not in the general summation constitute the residual equation

terms

> resid:=c(0)*(r*(r−1)+r−mˆ2)*xˆr+c(1)*(r*(r+1)+(r+1)−mˆ2)*xˆ(r+1);

resid := c(0)(r(r −1) +r −m

+c(1)(r(r +1) +r +1 −m

r+1

(1.182)

As stated earlier, since terms of unlike powers of x are linearly independent, then each of the

coefﬁcients of unlike powers of x in the preceding summation must equal zero. Setting the

coefﬁcient terms in the preceding inﬁnite sum equal to zero gives rise to the general recursion

formula

Ordinar y Linear Differential Equations 59

> recur:=c(n)*(n+r)*(n+r−1)+c(n)*(n+r)+c(n−2)−c(n)*mˆ2=0;

recur := c(n)(n +r)(n +r −1) +c(n)(n +r) +c(n −2) −c(n)m

= 0 (1.183)

Likewise, setting each of the two coefﬁcient terms in the residual equation equal to zero gives

rise to the two indicial equations

> ind1:=c(0)*(r*(r−1)+r−mˆ2)=0;

ind1 := c(0)(r(r −1) +r −m

) = 0 (1.184)

> ind2:=c(1)*(r*(r+1)+r+1−mˆ2)=0;

ind2 := c(1)(r(r +1) +r +1 −m

) = 0 (1.185)

Since both of the preceding terms must vanish, ideally, we solve for those values of r that will

make both indicial equations vanish simultaneously:

> solve(ind1,r);

m, −m (1.186)

> solve(ind2,r);

−m −1,m−1 (1.187)

We see that it is impossible to choose a value of r that will make both of the preceding terms

vanish simultaneously. In choosing a solution for r, we purposely avoid values that will give

negative exponents in the series solution, since this gives rise to singularities. Thus, from the

four solutions shown, the only two possible choices are

1. r = m, c(0) arbitrary, c(1) = 0

2. r = m −1, c(1) arbitrary, c(0) = 0

We choose the solution r = m,c(1) = 0, c(0) arbitrary. Setting r = m in the recursion formula

yields

> r:=m:recur:=subs(r=m,recur);

recur := c(n)(n +m)(n +m −1) +c(n)(n +m) +c(n −2) −c(n)m

= 0 (1.188)

From this, we evaluate the recursion relation

> c(n):=simplify(solve(recur,c(n)));

c(n) := −

c(n −2)

n(n +2 m)

(1.189)

60 Chapter 1

The preceding holds for 2 ≤ n. We now evaluate terms in the series. From the preceding

choices, we set

> c(1):=0;

c(1) := 0 (1.190)

>forkfrom2to12do

> c(k):=−c(k−2)/(k*(k+2*m))

> od:

The ﬁrst few terms in the expansion are

> y(x):=c(0)*xˆr+c(1)*xˆ(1+r)+eval(sum(c(n)*xˆ(n+r),n=2..5));

y(x) := c(0)x

−

c(0)x

2+m

2 +2 m

c(0)x

4+m

(2 +2 m)(4 +2 m)

(1.191)

Thus, one truncated solution to the Bessel differential equation of order m is

> y(x):=collect(y(x),c(0));

y(x) :=



−

2+m

2 +2 m

4+m

(2 +2 m)(4 +2 m)



c(0) (1.192)

For the special case when m is an integer, the convention used in most math textbooks is to set

the arbitary coefﬁcient c(0) to have the value

c(0) =

Thus, for m a positive number, the preceding terms are the ﬁrst three terms of the Bessel

function of the ﬁrst kind of order m, which, in general, can be expressed as

J(m, x) =

∞



n=0

(−1)

m+2 n

n!(n +m)!

For reasons that will become apparent when we study partial differential equations, the term m

is generally a positive integer. We now plot Bessel functions of the ﬁrst kind of integer order

zero, one, and two in Figure 1.4.

> plot({BesselJ(0,x),BesselJ(1,x),BesselJ(2,x)},x=0..10,thickness=10);

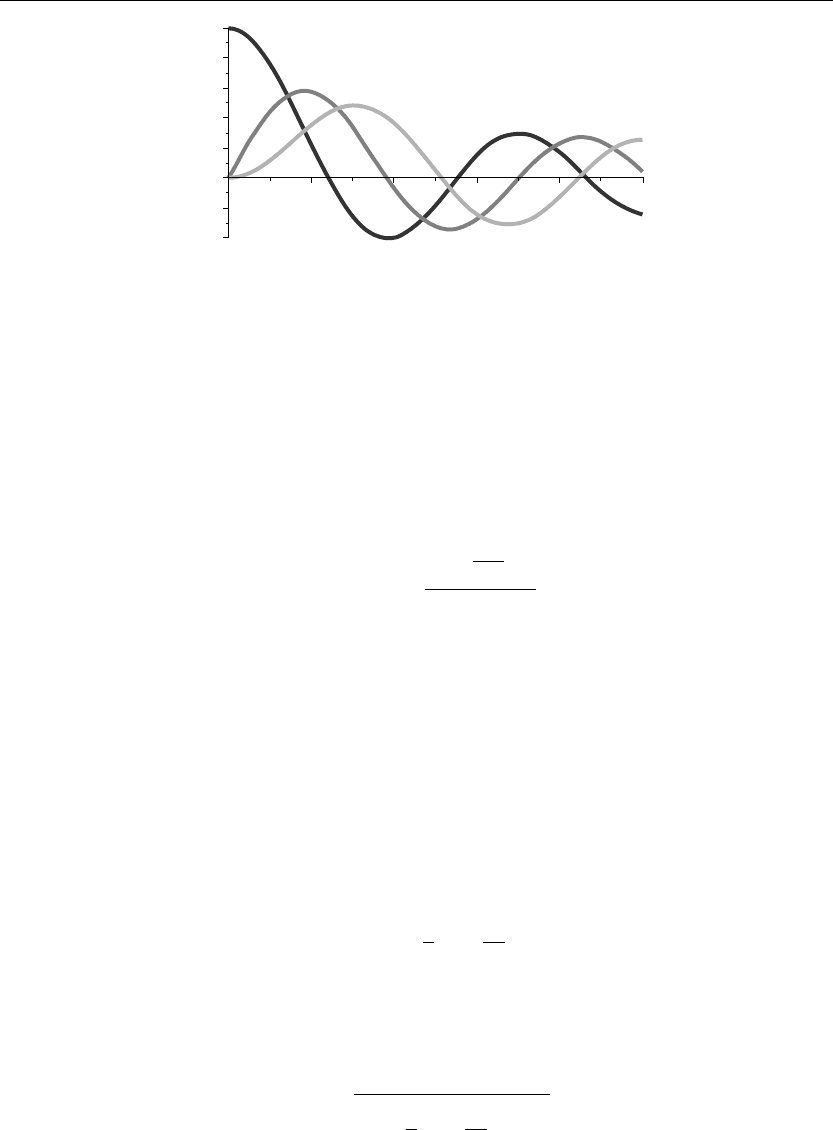
Ordinar y Linear Differential Equations 61

2610

0.4

0.2

0.4

0.6

0.8

1.0

Figure 1.4

EXAMPLE 1.11.2: Now that we have one solution to the Bessel differential equation of order

m, we seek a second basis vector using the method of reduction of order. For illustration

purposes and ease of calculation here, we focus on the special case for m = 0, and we consider

only the ﬁrst three terms in the series expansion of the ﬁrst basis vector y1(x).

SOLUTION: From Section 1.6, with knowledge of one solution vector y1(x), a second basis

vector y2(x) can be found from the equation

y2(x) = y1(x)

⎛

⎜

⎝



−





a1(x)

a2(x)



y1(x)

⎞

⎟

⎠

For the Bessel differential equation, we identify the coefﬁcients

> restart: a2(x):=xˆ2;a1(x):=x;

a2(x) := x

(1.193)

a1(x) := x

The ﬁrst few terms for y1(x) for m = 0 are

> y1(x):=1−xˆ2/4+xˆ4/64;

y1(x) := 1 −

(1.194)

Evaluation of the preceding integrand gives

> v(x):=(1/y1(x)ˆ2)*exp(−int(a1(x)/a2(x),x));

v(x) :=



1 −



(1.195)

62 Chapter 1

Taking just the ﬁrst three terms of the preceding integrand and integrating yields

> u(x):=int(convert(series(v(x),x,3),polynom),x);

u(x) := ln(x) +

(1.196)

Thus, our truncated series expansion of the second basis vector y2(x) becomes

> y2(x):=simplify(expand(y1(x)*u(x)));

y2(x) := ln(x) +

−

ln(x) −

ln(x) +

256

(1.197)

If we compare this second linearly independent solution with Y

(x), the Bessel function of the

second kind of order m = 0, we have

> Y[0](x):=expand((Pi/2)*(convert(series(BesselY(0,x),x,5),polynom)));

(x) :=−ln(2) +ln(x) +γ +

ln(2) −

ln(x) +

−

γ −

ln(2)

ln(x) −

128

γ (1.198)

Except for the conventions used in establishing certain constants (γ =Euler’s constant), we

see that our second series solution for y2(x) is equivalent to the Bessel function of the second

kind of order zero. Because x = 0 is a regular singular point of the differential equation, we

note that the preceding function fails to exist at the point x = 0.

We plot Bessel functions of the second kind of integer orders zero, one, and two in Figure 1.5.

> plot({BesselY(0,x),BesselY(1,x),BesselY(2,x)},x=0..8,y=−4..2,thickness=10);

134 8

2567

Figure 1.5

The signiﬁcant feature of the plot of Figure 1.5 shows that the Bessel functions of the second

kind, of integer order, all fail to exist at x = 0. The preceding system basis vectors will play a

Ordinar y Linear Differential Equations 63

signiﬁcant role when we consider partial differential equations in the cylindrical coordinate

system.

Chapter Summary

First-order linear nonhomogeneous differential equation

a1(t)



y(t)



+a0(t)y(t) = f(t)

System basis vector

y1(t) = e





−

a0(t)

a1(t)



Particular solution

(t) =



G1(t, s)f(s)ds

First-order Green’s function G1(t, s)

G1(t, s) =

y1(t)

a1(s)y1(s)

Second-order linear nonhomogeneous differential equation

a2(t)



y(t)



+a1(t)



y(t)



+a0(t)y(t) = f(t)

Second basis vector y2(t) as evaluated from the ﬁrst y1(t)

y2(t) = y1(t)

⎛

⎜

⎝



−





a1(s)

a2(s)



y1(s)

⎞

⎟

⎠

Particular solution in terms of the second-order Green’s function

(t) =



G2(t, s)f(s)ds

Second-order Green’s function G2(t, s)

G2(t, s) =

y1(s)y2(t) −y1(t)y2(s)

a2(s)W(y1(s), y2(s))

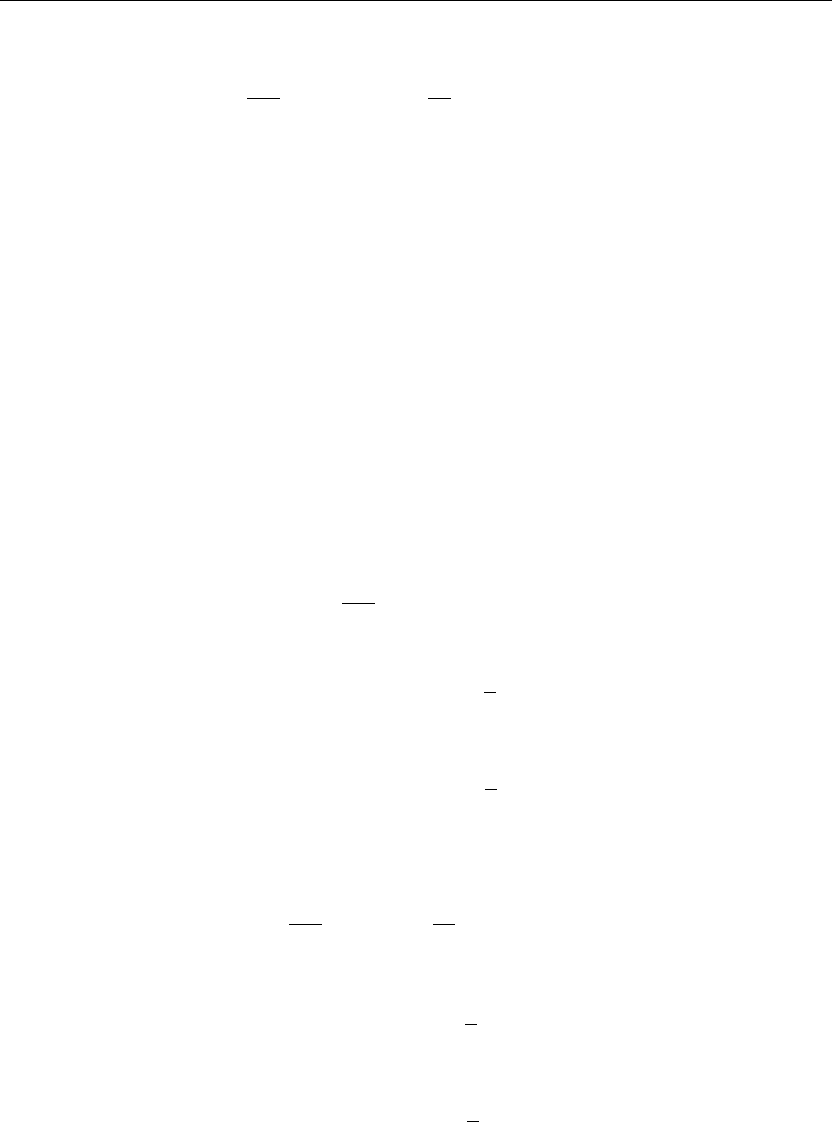
64 Chapter 1

Series solution to the second-order linear ordinary differential equation

a2(x)



y(x)



+a1(x)



y(x)



+a0(x)y(x) = 0

Assumed Frobenius solution about the origin

y(x) =

∞



n=0

c(n)x

n+r

Equivalent series equation

∞



n=0



a2(x)c(n)(n +r)(n +r −1)x

n+r−2

+a1(x)c(n)(n +r)x

n+r−1

+a0(x)c(n)x

n+r



= 0

From the preceding series equation, we develop the indicial equations and the recursion

formula for the ﬁnal solution.

Most often occurring differential equations

1. The Euler differential equation

y(x) +λy(x)= 0

A set of basis vectors for the Euler equation

y1(x) = sin



√

λx



and

y2(x) = cos



√

λx



2. The Cauchy-Euler differential equation



y(x)





y(x)



+λy(x) = 0

A set of basis vectors for the Cauchy-Euler equation

y1(x) = sin



√

λ ln(x)



and

y2(x) = cos



√

λ ln(x)



Ordinar y Linear Differential Equations 65

3. The Bessel differential equation of order m



y(x)





y(x)





−m



y(x) = 0

A set of basis vectors for the Bessel equation

y1(x) = J(m, x)

and

y2(x) = Y(m, x)

These procedures have shown that we can ﬁnd the general solution to both ﬁrst- and

second-order linear ordinary nonhomogeneous differential equations. For the case of

second-order nonhomogeneous differential equations, we demonstrated that from the

knowledge of only a single basis vector, we were able to generate the complete solution

to the differential equation. We have stressed the procedure of the method of variation of

parameters and the subsequent evaluation of the corresponding Green’s function. These

solutions will play a big role in the development of solutions to some partial differential

equations later.

Exercises

Consider the following ﬁrst-order linear nonhomogeneous differential equations. In all cases,

evaluate one basis vector y1(x) and the ﬁrst-order Green’s function G1(x, s), and then write

out the general solution. When possible, use the Maple command dsolve to verify the solution.

1.1.

y(x) +

y(x)

= 2 +x

1.2.



y(x)



+2 y(x) = e

+ln(x)

1.3.







y(x)



−2 xy(x) = x

1.4.

y(x) +tan(x)y(x) = sec(x)

66 Chapter 1

1.5.



y(x)



+(1 +x)y(x) = 3e

−x

Consider the following initial-value problems. In all cases, evaluate one basis vector y1(t) and

the ﬁrst-order Green’s function G1(t, s), and then evaluate the ﬁnal solution.

1.6. y(0) = 4

y(t) +2 y(t) = t +sin(t)

1.7. y(0) = 3

y(t) +ty(t) = t

1.8. y(0) =−5

y(t) +

4y(t)

t +2

= 3

1.9. y(0) = 7



2 t





y(t)



−10ty(t) = 4 t

1.10. y(0) = 1

y(t) +y(t) = e

−2 t

The following problems demonstrate applications of ﬁrst-order initial value problems.

Generate all solutions and develop a graph of the solution and an animated display as done in

the examples in Section 1.3.

1.11. A tank is initially ﬁlled with 40 gal of a salt solution containing 2 lb of salt per gal.

Fresh brine containing 3 lb of salt per gal runs into the tank at 4 gal per min, and the

mixture is kept uniform by constant stirring. (a) If the mixture runs out of the tank at the

same rate it runs in, ﬁnd the amount of salt q(t) at any time in the tank. (b) Evaluate the

time at which the amount of salt in the tank reaches 100 lb. Hint: This is an initial value

problem with q(0) = 80 lb, and the differential equation is

q(t) = 12 −

q(t)

1.12. Solve Exercise 1.11 for the case where the initial concentration of salt in the container

is 5 lb of salt per gal.

Ordinar y Linear Differential Equations 67

1.13. According to Newton’s law of cooling, a body loses heat at a rate proportional to the

instantaneous temperature difference between the body and its surroundings. Consider a

body that loses heat in accordance with this law, where the initial temperature of the

body is 70°C, the surrounding temperature is 20°C, and the temperature decay

coefﬁcient is k = 3/10 per min. (a) Find an expression for the temperature T(t) at any

time t. (b) At what time will the body temperature be 30°C? Hint: This is an initial

condition problem with T(0) = 70°C, and the differential equation is

T(t) =−k(T(t) −20)

1.14. Solve Exercise 1.13 for the case where k = 5/10/min and T(0) = 100°C.

1.15. A body of mass m undergoes freefall due to gravity in a viscous medium, where the

viscous force is proportional to the body speed. We would like to calculate the speed

v(t) of a body whose mass is m = 5 kg in freefall if its initial speed is 50 m/sec upward

and the damping coefﬁcient is γ = 1 N/m/sec. The acceleration due to gravity is

g = 9.8 m/sec/sec in the MKS system. Hint: This is an initial value problem with

v(0) = 50 m/sec, and the differential equation is



v(t)



=−mg −γv(t)

1.16. Solve Exercise 1.15 for the time at which the speed is zero.

1.17. Solve Exercise 1.15 for the case where the damping coefﬁcient is doubled.

1.18. We consider a resistance-inductance (RL) circuit with an impressed voltage e(t), where

the electric current in the circuit at any time t is i(t). The circuit resistance R = 20 ohms

and the inductance L = 100 henries. At time t = 0, the circuit switch is closed, and the

impressed voltage is e(t) = 20 volts. Solve for the current in the circuit. Hint: This is an

initial value problem with i(0) = 0 amps, and the differential equation is



i(t)



+Ri(t) = e(t)

1.19. Solve Exercise 1.18 for the case where the impressed voltage is e(t) = 40 sin(t) volts

and the initial current is i(0) = 10 amps.

1.20. We consider a resistance-capacitance (RC) circuit with an impressed voltage e(t), where

the electric charge on the capacitor at any time t is q(t). The circuit resistance R = 3

ohms, and the capacitance C = 2 farads. At time t = 0, the circuit switch is closed and

the capacitor has an initial charge of 200 coulombs. If the impressed voltage is

e(t) = 20 volts, solve for the charge in the circuit. Hint: This is an initial value problem