Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V

Подождите немного. Документ загружается.

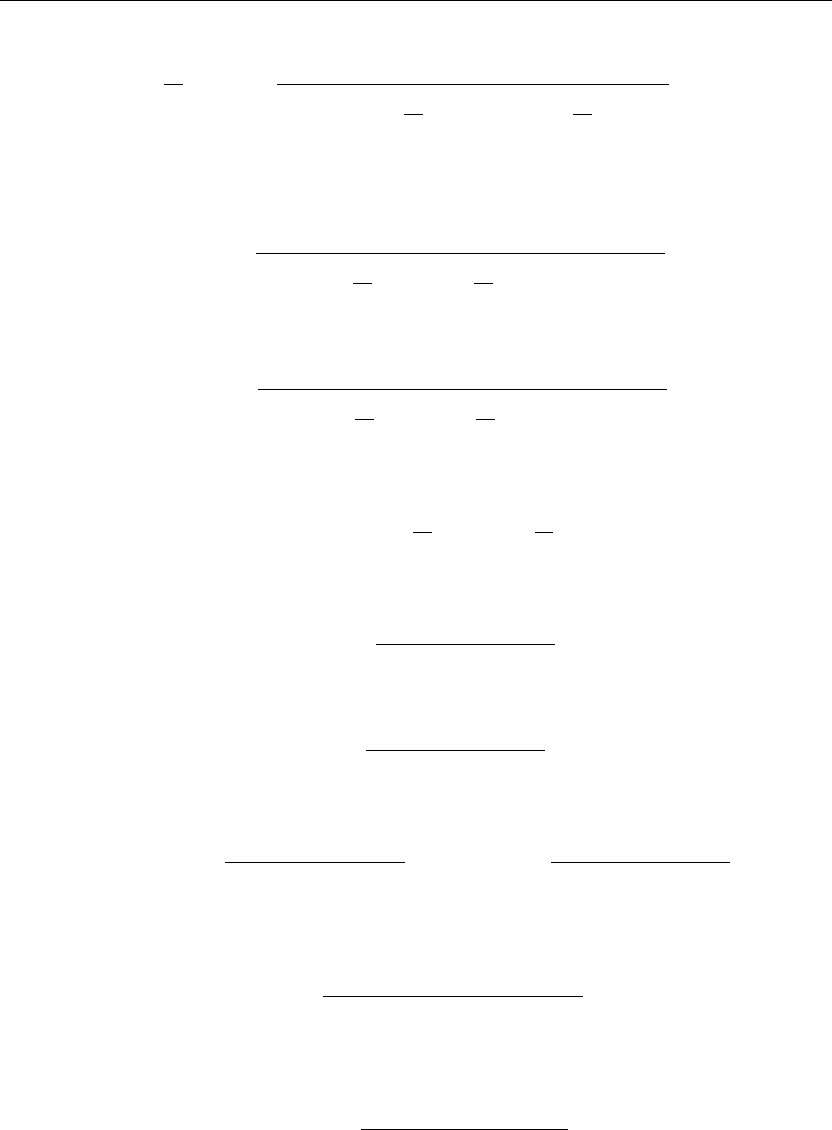
38 Chapter 1

and

u2(t) =−

f(t)y1(t)

a2(t)



y1(t)



y2(t)



−y2(t)



y1(t)



Integrating each of the preceding yields

u1(t) =



⎛

⎜

⎝

−

f(t)y2(t)



−y2(t)



y1(t)





y2(t)



y1(t)



a2(t)

⎞

⎟

⎠

and

u2(t) =



−

f(t)y1(t)



−y2(t)



y1(t)





y2(t)



y1(t)



a2(t)

Recognizing the denominator in the integrands as the Wronskian

W(y1(t), y2(t)) =−y2(t)



y1(t)





y2(t)



y1(t)

we get the following solutions

u1(t) =−





f(t)y2(t)

a2(t)W(y1(t), y2(t))



and

u2(t) =



f(t)y1(t)

a2(t)W(y1(t), y2(t))

Thus, our particular solution to the differential equation becomes

(t) = y2(t)





f(t)y1(t)

a2(t)W(y1(t), y2(t))



−y1(t)





f(t)y2(t)

a2(t)W(y1(t), y2(t))



Using the dummy variable s in the integrands, a more compact form of the particular solution

can be rewritten as

(t) =



(y1(s)y2(t) −y1(t)y2(s))f(s)

a2(s)W(y1(s), y2(s))

Similar to what we did for ﬁrst-order differential equations, we now deﬁne G2(t, s) as the

second-order Green’s function

G2(t, s) =

y1(s)y2(t) −y1(t)y2(s)

a2(s)W(y1(s), y2(s))

(1.90)

Ordinar y Linear Differential Equations 39

Of course, this result is valid only on an interval I, where the term a2(t) does not vanish—that

is, on an interval where the equation is normal. Further, since the vectors form a basis, they are

linearly independent, and the Wronskian is nonzero on this interval.

In terms of the second-order Green’s function given earlier, the particular solution takes on

the form

(t) =



G2(t, s)f(s)ds (1.91)

Again, we see that this form of the solution can accommodate any type of driving or source

function f(t). This procedure, by way of the method of variation of parameters, leads to an

integral solution whereby the Green’s function acts as the kernel of the integrand.

The beauty of the preceding form just derived becomes apparent when we compare it with the

alternate procedure of undetermined coefﬁcients. In that procedure, we have to make a

tentative guess at the solution. The guess, of course, depends on the character of the source

function f(t) and whether or not this function is linearly dependent on the system basis vectors.

With the preceding method, there is no need to guess, and we get immediate solutions.

DEMONSTRATION: We seek the particular solution to the second-order linear

nonhomogeneous differential equation

y(t) +2



y(t)



+y(t) = te

−t

SOLUTION: We identify the coefﬁcients of the differential equation a2(t) = 1, a1(t) = 2, and

a0(t) = 1, and the source term

f(t) = te

−t

Earlier, from Example 1.6.1, we found a set of basis vectors to be

y1(t) = e

−t

and

y2(t) = te

−t

We note the linear dependence of the source term on one of the basis vectors. Evaluation of the

Wronskian of the basis yields

W(e

,te

) = e

−2t

Evaluation of the second-order Green’s function from the formula

G2(t, s) =

y1(s)y2(t) −y1(t)y2(s)

a2(s)W(y1(s), y2(s))

40 Chapter 1

yields

G2(t, s) =

−s

−t

−e

−t

−s

−2s

The particular solution is the integral whose integrand is the product of the Green’s function

and the source function written in terms of the dummy variable s:

(t) =



−s

−t

−e

−t

−s

)se

−s

−2 s

ds (1.92)

Integrating ﬁrst with respect to the dummy variable s and then substituting t for s yields

(t) =

−t

Thus, the general solution to the problem is the sum of the homogeneous plus the particular

solution. Here C1 and C2 are arbitary constants.

y(t) = C1e

−t

+C2 te

−t

(1.93)

The example just described is especially interesting because the source term f(t) is linearly

dependent on one of the basis vectors. The ease with which we found this solution indicates the

method of variation of paramaters to be far more convenient than what we would have

encountered with the method of undetermined coefﬁcients.

EXAMPLE 1.7.1: Use the method of variation of parameters to generate the particular solution

to the differential equation that is not normal at the origin.



y(t)



y(t) = t +1

SOLUTION: We identify the coefﬁcients

> restart:a2(t):=t;a1(t):=1;a0(t):=0;

a2(t) := t

a1(t) := 1

a0(t) := 0 (1.94)

Source term

> f(t):=t+1;

f(t) := t +1 (1.95)

From earlier methods, we see that one basis vector is a constant and a second basis vector can

be found using methods of Section 1.6. Thus, two possible basis vectors are

Ordinar y Linear Differential Equations 41

> y1(t):=1;

y1(t) := 1 (1.96)

> y2(t):=ln(t);

y2(t) := ln(t) (1.97)

Wronskian

> W(y1(t),y2(t)):=y1(t)*diff(y2(t),t)−y2(t)*diff(y1(t),t);

W(1, ln(t)) :=

(1.98)

> y1(s):=subs(t=s,y1(t)):y2(s):=subs(t=s,y2(t)):W(y1(s),y2(s)):=subs(t=s,W(y1(t),y2(t))):a2(s)

:=subs(t=s,a2(t)):f(s):=subs(t=s,f(t)):

Green’s function

> G2(t,s):=(y1(s)*y2(t)−y1(t)*y2(s))/(a2(s)*W(y1(s),y2(s)));

G2(t, s) := ln(t) −ln(s) (1.99)

Particular solution (integrate ﬁrst with respect to s and then substitute s with t)

> y[p](t):=Int(G2(t,s)*f(s),s);

(t) :=



(ln(t) −ln(s))(s +1)ds (1.100)

> y[p](t):=subs(s=t,value(%));

(t) :=

+t (1.101)

General solution: here C1 and C2 are arbitrary constants.

> y(t):=C1*y1(t)+C2*y2(t)+y[p](t);

y(t) := C1 +C2 ln(t) +

+t (1.102)

This solution is valid everywhere except at the point t = 0. This follows, since the differential

equation fails to be normal at the origin.

Check: Using Maple dsolve command.

> restart:ode:=t*diff(y(t),t,t)+diff(y(t),t)=t+1:dsolve(ode);

y(t) =

+_C1 ln(t) +t +_C2 (1.103)

42 Chapter 1

EXAMPLE 1.7.2: We seek the solution to the nonhomogeneous Euler differential equation

y(t) +y(t) = sec(t)

SOLUTION: We identify the coefﬁcients

> restart:a2(t):=1;a1(t):=0;a0(t):=1;

a2(t) := 1

a1(t) := 0 (1.104)

a0(t) := 1

Source function

> f(t):=sec(t);

f(t) := sec(t) (1.105)

System basis vectors from earlier result

> y1(t):=sin(t);

y1(t) := sin(t) (1.106)

> y2(t):=cos(t);

y2(t) := cos(t) (1.107)

Wronskian

> W(y1(t),y2(t)):=simplify(y1(t)*diff(y2(t),t)−y2(t)*diff(y1(t),t));

W(sin(t), cos(t)) := −1 (1.108)

> y1(s):=subs(t=s,y1(t)):y2(s):=subs(t=s,y2(t)):W(y1(s),y2(s)):=subs(t=s,W(y1(t),y2(t))):a2(s)

:=subs(t=s,a2(t)):f(s):=subs(t=s,f(t)):

Green’s function

> G2(t,s):=(y1(s)*y2(t)−y1(t)*y2(s))/(a2(s)*W(y1(s),y2(s)));

G2(t, s) := −sin(s) cos(t) +sin(t) cos(s) (1.109)

Particular solution (integrate ﬁrst with respect to s and then substitute s with t)

> y[p](t):=Int(G2(t,s)*f(s),s);

(t) :=



(−sin(s) cos(t) +sin(t) cos(s)) sec(s)ds (1.110)

Ordinar y Linear Differential Equations 43

> y[p](t):=subs(s=t,value(%));

(t) := cos(t) ln(cos(t)) +sin(t)t (1.111)

General solution: here C1 and C2 are arbitrary constants.

> y(t):=C1*y1(t)+C2*y2(t)+y[p](t);

y(t) := C1 sin(t) +C2 cos(t) +cos(t) ln(cos(t)) +sin(t)t (1.112)

Check: Using Maple dsolve command.

> restart:ode:=diff(y(t),t,t)+y(t)=sec(t):dsolve(ode);

y(t) = sin(t) _C2 +cos(t) _C1 +t sin(t) + ln(cos(t)) cos(t) (1.113)

EXAMPLE 1.7.3: We are given one solution to the Cauchy-Euler homogeneous differential

equation shown here. From this single basis vector, we are asked to ﬁnd a complete solution to

the diffferential equation.



y(t)



−2t



y(t)



+2y(t) = t ln(t)

SOLUTION: We identify the coefﬁcients

> restart:a2(t):=tˆ2;a1(t):=−2*t;a0(t):=2;

a2(t) := t

a1(t) := −2t

a0(t) := 2 (1.114)

By inspection, we see one basis vector to be

> y1(t):=t;

y1(t) := t (1.115)

Source term

> f(t):=t*ln(t);

f(t) := t ln(t) (1.116)

A second basis vector by the method of reduction of order is

> y2(t):=y1(t)*int(exp(−int(a1(t)/a2(t),t))/y1(t)ˆ2,t);

y2(t) := t

(1.117)

44 Chapter 1

Wronskian

>W(y1(t),y2(t)):=simplify(y1(t)*diff(y2(t),t)−y2(t)*diff(y1(t),t));

W(t, t

) := t

(1.118)

>y1(s):=subs(t=s,y1(t)):y2(s):=subs(t=s,y2(t)):W(y1(s),y2(s)):=subs(t=s,W(y1(t),y2(t))):a2(s)

:=subs(t=s,a2(t)):f(s):=subs(t=s,f(t)):

Green’s function

> G2(t,s):=(y1(s)*y2(t)−y1(t)*y2(s))/(a2(s)*W(y1(s),y2(s)));

G2(t, s) :=

−ts

(1.119)

Particular solution (integrate ﬁrst with respect to s and then substitute s with t)

> y[p](t):=Int(G2(t,s)*f(s),s);

(t) :=



(st

−ts

) ln(s)

ds (1.120)

> y[p](t):=subs(s=t,value(%));

(t) := −t ln(t) −t −

t ln(t)

(1.121)

General solution: here C1 and C2 are arbitrary constants.

> y(t):=C1*y1(t)+C2*y2(t)+y[p](t);

y(t) := C1 t +C2 t

−t ln(t) −t −

t ln(t)

(1.122)

This solution is valid everywhere except at the origin.

Check: Using Maple dsolve command.

> restart:ode:=tˆ2*diff(y(t),t,t)−2*t*diff(y(t),t)+2*y(t)=t*ln(t):expand(dsolve(ode));

y(t) = t

_C2 +t _C1 −t ln(t) −t −

ln(t)

t (1.123)

This example demonstrates a signiﬁcant solution procedure in that, from knowledge of only a

single solution vector, we were able to construct a basis in addition to the complete solution to

the nonhomogeneous differential equation.

Ordinar y Linear Differential Equations 45

1.8 Initial-Value Problem for Second-Order

Differential Equations

Often in partial differential equations, we have to ﬁnd solutions to time-dependent,

second-order linear differential equations with initial conditions as constraints. We now

investigate the initial-value problem for the second-order differential equation.

Consider the following linear nonhomogeneous differential equation with constant coefﬁcients,

which is descriptive of the motion of a simple harmonic oscillator with damping. Here, m is the

mass, γ is the damping coefﬁcient, and k is the spring constant.



y(t)



+γ



y(t)



+ky(t) = f(t)

We assume damping in the system to be small (γ

< 4km) and we set

ω =



4km −γ

From Section 1.4, we evaluate a set of basis vectors

y1(t) = e

−

γt

sin(ω t)

and

y2(t) = e

−

γt

cos(ω t)

Evaluation of the Wronskian and the subsequent second-order Green’s function yields

G2(t, s) =

−

γ(t−s)

sin(ω t −ωs)

mω

Thus, we can write our general solution to the differential equation as

y(t) = C1 e

−

γt

sin(ωt) +C2 e

−

γt

cos(ωt) +



−

γ(t−s)

sin(ωt −ωs)f(s)

mω

Since the differential equation is of order two, we have two initial conditions, and, at time

t = 0, the two initial conditions on the problem are the initial position

y(0) = y

and the initial speed

v(0) = v

46 Chapter 1

Substituting these initial conditions, we evaluate the arbitrary constants C1 and C2, and we

arrive at the ﬁnal solution

y(t) =

(2 v

m +y

γ)e

−

γt

sin(ωt)

2ωm

−

γt

cos(ωt) +



−

γ(t−s)

sin(ωt −ωs)f(s)

mω

(1.124)

This form of the solution can accommodate any set of initial conditions and any source

function f(t). Note that there are no arbitrary constants in the preceding equations because the

initial conditions have already been incorporated into the solution.

EXAMPLE 1.8.1: We consider a mass m = 1 kg attached to a simple spring with a spring

constant k = 65/4 N/m, which is immersed in a damping medium with a damping coefﬁcient

γ = 1 N/m/sec. An applied force f(t) in units of N acts on the system and is given following.

The initial position of the mass is 10 m and the initial speed is 20 m/sec. From Newton’s

second-law, the dynamic equation of motion of the mass reads as

y(t) +

65y(t)

= f(t)

SOLUTION: We identify the coefﬁcients

> restart:a2:=1;a1:=1;a0:=65/4;

a2 := 1

a1 := 1

a0 :=

(1.125)

The driving function

> f(t):=10*t*exp(−t/2);

f(t) := 10t e

−

(1.126)

The damped angular oscillation frequency of the spring-mass system is

> omega:=sqrt(4*a2*a0−a1ˆ2)/(2*a2);

ω := 4 (1.127)

Initial conditions

> y(0):=10;

y(0) := 10 (1.128)

Ordinar y Linear Differential Equations 47

> v(0):=20;

v(0) := 20 (1.129)

System basis vectors

> y1(t):=exp(−a1*t/(2*a2))*sin(omega*t);

y1(t) := e

−

sin(4t) (1.130)

> y2(t):=exp(−a1*t/(2*a2))*cos(omega*t);

y2(t) := e

−

cos(4t) (1.131)

Wronskian

> W(y1(t),y2(t)):=simplify(y1(t)*diff(y2(t),t)−y2(t)*diff(y1(t),t));



−

sin(4t), e

−

cos(4t)



:= −4e

−t

(1.132)

> y1(s):=subs(t=s,y1(t)):y2(s):=subs(t=s,y2(t)):W(y1(s),y2(s)):=subs(t=s,W(y1(t),y2(t))):f(s):=

subs(t=s,f(t)):

Green’s function

> G2(t,s):=(y1(s)*y2(t)−y1(t)*y2(s))/(a2*W(y1(s),y2(s)));

G2(t, s) := −

−

sin(4s) e

−

cos(4t) −e

−

sin(4t) e

−

cos(4s)

−s

(1.133)

> G2(t,s):=simplify(combine(G2(t,s),trig));

G2(t, s) := −

sin(4s −4t)e

s−

(1.134)

Particular solution

> y[p](t):=Int(G2(t,s)*f(s),s=0..t);

(t) :=





−

sin

(

4s −4t

)

s−

s e

−



ds (1.135)

> y[p](t):=combine(value(%),trig);

(t) := −

−

sin(4t) +

−

(1.136)