Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.

380
R.
H.
Martin
and
M.
Pierre
where
p,
q
2
1
and
c
:
R
--*
R
is
a
regular function
with variable sign on
R.
Again uniform L’-estimate and equivalence of LP-norms hold, but global
existence does not follow. Partial “local” estimates have been obtained
for
(6.4)
in
[ll]
where this kind of systems are introduced. Note that numerical
experiments show that if dl and
d2
are very different
(d2
ss
c(z)upvq
can become very large
at
some time. However, in the situations considered,
the solutions seem
to
become “smoother” after
a
while.
There are actually elliptic versions of the same questions which could
perhaps be looked
at
first to provide some hints and which are
also
of interest
by themselves. One such example is
where (again)
0
<
X
2
1,
p,
q,
r,
s
>
1,
F,
G
are nonnegative and smooth
given functions on
R,
p
>
0
and where we denote
z
=
(11,.
. . ,
zN).
Again,
we easily prove (as in Proposition
4.1)
that
If
p
is
small enough, this allows
us
to
conclude existence of
a
solution in
(6.5).
For
large
p,
the question of existence seems
to
be open in general.
1.6.2
“Critical” Boundary Conditions
Although general boundary conditions
of
type
(4.5)
can be handled by
our
basic method, some “critical” cases are not (see the extra hypothesis
(4.6)).
For
instance, in the case of the Brusselator
(5.1),
it
is
not known if solutions
subject to the boundary conditions
i3V
an
u=
B/A
and
-
=O
ondRx(0,m)
Nonlinear Reaction-Diffusion
Systems
381
exist
or
not for
all
time. The same question holds for the simpler system
(even if
dl
=
d2)
~t
-
dlAu
=
-UV’
on
R
x
(0,
00)
I
vt
-
d2
AV
=
UIJ~
It would probably be worthwhile to first understand existence in the elliptic
version
u
-
dlA~
=
-UV~
+
F
on
R
I
v
1
d2A~
=
UV~
+
G
on
80
when
F,
G
are given nonnegative smooth functions on-
R.
of “Brusselator type”
One result in that direction
is
obtained by Hollis
[8]
for the hybrid system
ut
=
dlAu
-
UV~
+
BV
on
0
x
(0,
00)
vt
=
uv2
-
(B
+
1)”
+A
1
u
=
B/A
on
dR
x
(0,
00)
(6.6)
It
is
easy to see that solutions to the associated
O.D.E.
exist and are uni-
formly bounded on
[O,
00).
However, Hollis establishes the following:
[
if
max{v,(z)
:
z
E
an}
>
max{A, A/B} then there
is
a
T’
<
00
such that
t
lim
Ilv(.,t)lloo
=
00.
t-+T*-
To
see that
(6.7)
is
valid set
for
O<a<1
B
+
1
+
[(B
+
1)2
-
4aB]’/2
2aB/A
A(a)
I
and consider the ordinary differential equation
382
R.
H.
Martin
and
M.
Pierre
and observe that
20,
>
A(@)
implies
w(t)
00
as
t
T
T,
for
some
T,
<
00.
If it
is
assumed that
u,
v
exists on
(0,oo)
then there
is
an
11
E
dR
and
number
6
>
0
and
0
<
a
<
1
such that
v,(z)
>
A(a)
for
all
I
E
R
with
11
-
111
<
6.
Also, since
u
is
continuous on
a
x
[0,
T,]
there
is
an
I,
E
R
such that
Ii,
-
111
<
6
and
u(i,,t)
2
aB/A
for all
0
5
t
5
T,.
But this
implies
v(zo,t)
2
w(t)
for
t
E
[O,T,)
where
w,
=
vo(zo)
>
A((.).
Since
w(t)
oo
as
t
r
T,
we have a contradiction and this shows that the solution
to
(6.6)
must blow-up in finite time.
1.6.3
Nonlinear Diffusions
Our basic method heavily relies on LP-regularity theory for
linear
parabolic
operators. Obviously, this approach fails when the diffusions are nonlinear.
Let us indicate some of the corresponding systems. Considering
2x 2
systems
with the same nonlinearity
as
in Section
3
leads to the equations
where
0,
$
:
[0,
co)
are regular increasing functions from
[0,
oo)
into
[0,
co)
with
d(0)
=
$(O)
=
0.
Local existence
is
easy for nonnegative Lm-initial
data. Global existence
is
open in general.
However, the “L’-technique” introduced in
[21]
that we will describe in
Section
8
turns out to be very helpful for these nonlinear situations.
For
instance, partial results can be obtained for specific choices
of
4
and
$
(see
[13]).
More interesting
is
the possibility of proving global existence
of
weak
solutions in the following
~t
-
A,u
=
-uh(v)
+
F
~t
-
Apv
=
uh(v)
+
G
I
u=v=O
on
dRx(0,oo)
where Apu
=
div (Iv~lp-~Vu) and
p
>
1
(see
[13]).
Here, the basic method
would fail.
The problem becomes even more complicated when the diffusions are of
different types, such as
(Ap,
Aq) with
p
#
q,
or
(Ad(.),
A$(-)),
or
more gen-
eral nonisotropic diffusions. Diffusions with first order terms of the following
(6.9)
Nonlinear Reaction-Diffusion Systems
383
form are studied in
[lo]:
ui
-
dlAu
+
div
(ue)
=
-uvp
vt
-
dzAv
+
div
(urn)
=
uvp
u=v=O
on
aRx(O,
a).
on
R
x
(0,
co)
(6.10)
For
dimension
N
>
1,
global existence
is
obtained in
[lo]
for
The method
is
an extension of the one described in Section
4
for the non
obvious cases (small values of
m).
A
direct method of supersolutions takes
care
of
m
>
p.
However
a
gap still exists for the values of
m
between
(N
+
2)/2
and
p.
Note that elliptic version of the same problems could be considered first.
Some could be treated using the “LP-technique” of Section
4
such as
(6.11)
and
(6.12)
u
-
A$(u)
=
-UV@
+
F
v
-
A$(.)
=
uvp
+
G
+
boundary conditions
u
-
Apu
=
-uvp
+
F
v
-
Apv
=
uvp
+
G
+
boundary conditions.
However, many existence questions are left open for similar elliptic systems.
1.6.4
About
Polynomial
growth
It
is
well-known that LP-regularity theory fails for
p
=
1.
This
is
why
L’”-estimates cannot be directly obtained by the duality method used in
Proposition
4.1.
Therefore, the assumption
of
polynomial growth
is
neces-
sary for these conclusions. Note that LP-theory can be extended to some
Orlicz spaces. Although it has not been explicitly written yet, the same
approach should allow nonlinear growth up to less than exponential growth.

384
R.
H.
Martin
and
M.
Pierre
This
is
confirmed in
a
specific case with
a
different technique in
[7]
where,
as
mentioned before, growth
of
the
form
e"'
with
7
<
1
is handled.
One of the main advantages
of
the L1-technique described in Section
8
is
that it does not require any growth assumption
to
provide global existence
of weak solutions. However the question
of
existence
of
classical
solutions re-
mains open even in simple systems like
(3.1).
We give next
a
very particular
result in that direction.
1.7
A Particular Result Without Polynomial Growtk
Assumption
We consider the following system on the whole space
RN:
tit
-
dlAu
=
-f(u,
V)
on
R~
x
(0,
OO)
~t
-
d2Av
=
f(~,
V)
u(.,
0)
=
u,(.),
v(.,
0)
=
v,(.)
on
R~
vu,
v
2
0
f(0, v)
=
0
I
f(u,
0).
i
(7.1)
where
f
:
[0,
m)'
+
[0,
m)
is locally Lipschitz continuous and satisfies
(7.2)
(Note that
f(u,
v)
2
0
for
all
u,
v
2
0).
Theorem
7.1
Assume
(7.3)
d2
I
dl
and
(7.4)
u,,
v,
E
L"(R~),
u,,
v,
2
o
a.e.
on
R~.
Then
(7.1)
has
a
classical solution on
R
x
(0,
m).
Lemma
7.2
Let
g
be
a
nonnegative regular function on
RN
x
(0,
m).
For
all
X
>
0,
let
wx
be
the solution of
WA~
-
XAwx
=
9
OR
RN
x
(0,
OO),
9
f
0
wx(.,
0)
=
o
on
R~
(7.5)
{
Then
(7.6)
x
c.,
~~/'wX(t,
z)
is increasing for all
(t,
z).

Nonlinear Reaction-Diffusion Systems
385
Proof.
g,
namely
We use (for instance) the explicit representation of
wx
in terms
of
wx(t,
z)
=
~~JIN(4aXs)-N/2exp(-ly12/4Xs)g(t
-
s,
z
-
y)dsdy.
Since
g
2
0,
(7.6) follows.
Proof
of
Theorem
7.1
Local existence
of
a
classical nonnegative solution
(u,
u)
to (7.1)
on
a
maximal interval of existence
[0,
T')
is obtained
as
usual.
We denote by
Sx(t)
the semigroup generated by
-Ah
on
RN
and by
zx
the solution of
Then, by linearity, we have for all
t
E
(0,
T,)
(7.8)
u(t)
=
sdl
(t)uo
-
zdl
(t)
(7.9)
u(t)
=
Sdz(t)vo
+
zdz(t).
By Lemma 7.2, since
d2
5
dl
we have
(7.10)
zdz(t)
5
(dl/d2)N/2zdl
(t).
From (7.8)-(7.10) and
u
2
0
we
deduce
(7.11)
v(t)
5
Sd2
(t)vo
+
(di/d2)N/2Sdl
(t)uo.
This provides a uniform Loo-estimate for
v.
On the other hand we already
know that
.(t)
5
Sdl(t)uo.
This proves global existence of classical solutions.
1.8
The L'-technique
Again we will describe this technique for the 2x 2 system introduced in (4.1)-
(4.9). For simplicity, we assume that the diffusions are linear and dissipative
in
U(Q)
for
all
p
E
[l,
031.
More precisely, we assume (4.1), (4.2),
(4.3)
and
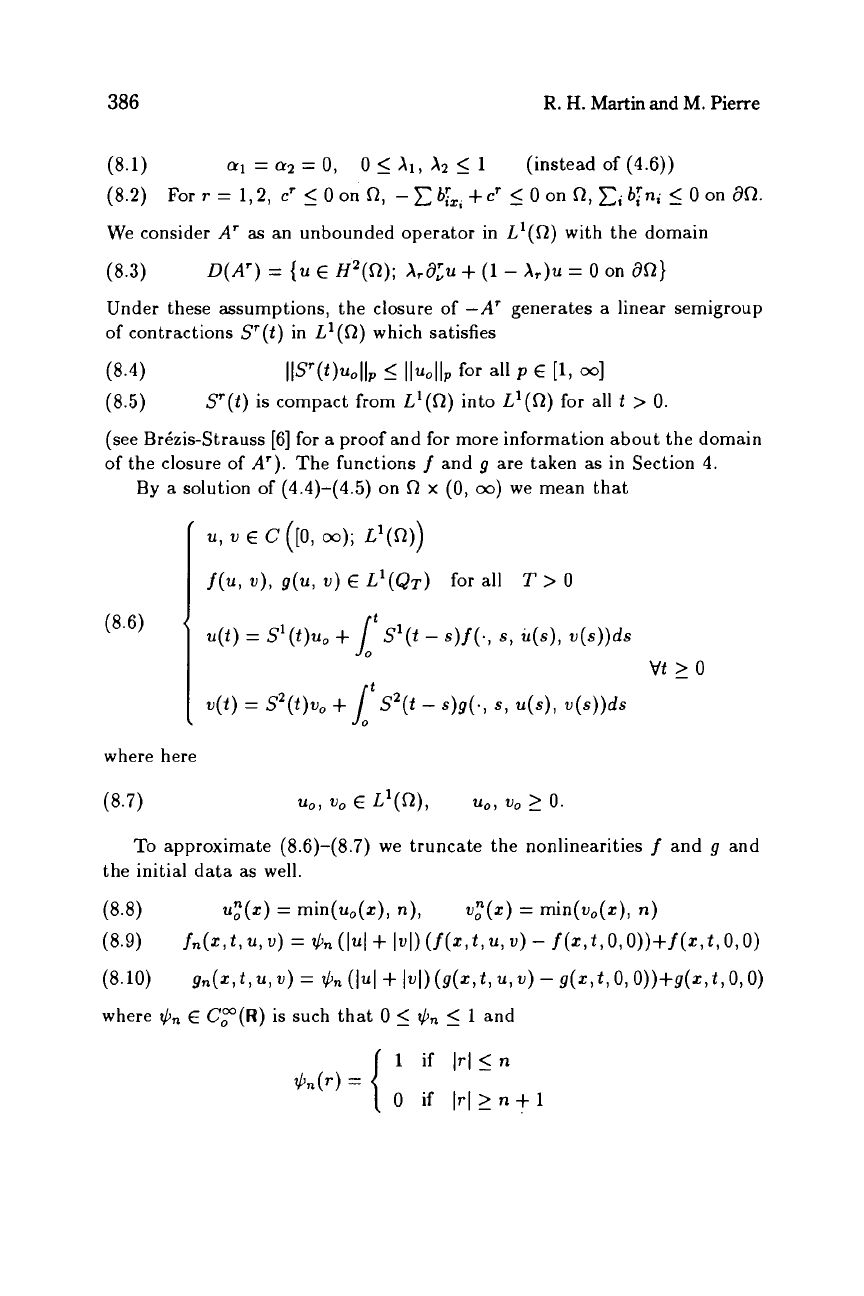
386
R.
H.
Martin
and
M. Pierre
f(ul
v),
g(u,
v)
E
L'(QT)
for
all
T
>
0
t
0
Qt
2
0
v(t)
=
S2(t)v0
+
S2(t
-
s)g(.,
s,
U(S),
v(s))ds
We consider
A'
as
an unbounded operator in
L'(R)
with the domain
(8.3)
D(A')
=
{u
E
HZ(R);
A,aLu
+
(1
-
X,)u
=
0
on
an}
Under these assumptions, the closure of
-A'
generates
a
linear semigroup
of contractions
S'(t)
in
L'(R)
which satisfies
(8.4)
IIS'(t)~oIlp
5
lluollp
for
all
P
E [I,
ml
(8.5)
ST(t)
is compact from
~'(0)
into
L'(R)
for all
t
>
0.
(see Br6zis-Strauss
[6]
for
a
proof and for more information about the domain
of the closure of
A').
The functions
f
and
g
are taken
as
in Section 4.
By
a
solution of (4.4)-(4.5) on
R
x
(0,
co)
we mean that
To
approximate
(8.6)-(8.7)
we truncate the nonlinearities
f
and
g
and
the initial data as well.

Nonlinear Reaction-Diffusion
Systems
387
Note that
f(:,
',
0,O)
E
L"
(R
x
[0,
co))
and that
fn,
gn
satisfy the same
hypotheses as
f
and
g
with the same constants independent of
n.
In addition
there exists
M(n)
such that
Proposition
8.1
We assume
(4,l), (d.,?), (4.3), (4.8), (4.9), (8.l), (8.2),
(8.7)-(8.10).
Then,
for
all
n,
there exists
a
classical nonnegative solution
(unj
vn)
of
(8.12)
Proof
Local existence
is
classical. Global existence follows from
(8.11)
which ensures that, for
all
t
for which
(unl
vn)
exists,
with
M(n,
t)
<
00
for all
n,
t
>
0
Our goal
is
now to prove that, under suitable additional assumptions the
solution
(unl
vn)
of
(8.12)
converges to
a
solution of
(8.6)
as
n
tends to
00.
This will be realized by obtaining appropriate estimates on
un,
vn.
Thanks to the two main structure assumptions
(4.8),
(4.9)
on the non-
linearity, we immediately have
Lemma
8.2
Under assumptions
of
Proposition
8.1
Proof
From
(8.12)
and
(4.9)
we have
(8.14)
&(un
+
vn)
5
A1un
+
A2vn
+
L(un
+
vn)
+
M

388
R. H.
Martin
and
M.
Pierre
We integrate this over
R.
Since
un,
vn
2
0
and
A’, A2
are dissipative in
L’W)
(8.15)
As
a
consequence,
an(t)
=
un(t)
+
vn(t)
satisfies the differential inequality
ak(t)
5
La,(t)
+
M.
The relation (8.13) follows.
In order to get better estimates on
u,,
v,,
we need to put more structure
on
f,
g.
According to examples we assume that
(8.16)
Remark
The condition
a
#
p
ensures that relation (8.16) be independent
of
the previous assumption
(4.9)
concerning
f
+
g.
Note that both are
satisfied for the model example of Section 3 with
a
=
1,
P
=
0
=
L1
=
Mi
=
L
=
M.
Lemma
8.3
Zf
the assumptions
of
Proposition
8.1
hold and
if
(8.16)
holds,
then for allT
>
0,
there exists
R(T)
depending only on
T,
lluolll, llvolll, L, M
L1, MI,
a,
/3
such
that
3a,
P
0,
a
#
P
and
L1,
MI
2
0
such that
{
vu,
‘u
L
0
af(u,
v)
+
Pg(u,
v)
5
L1(u,
.)
+
Ml
(8.17)
Proof.
lead to
(8.18)
We set
(8.19)
where the nonnegativeness of
Rn
and
Sn
follows by (4.9) and (8.16), respec-
tively. Therefore, by (8.18),
Integrating each
of
the two first equations in (8.12) and using (8.15)
-1
u:
I
J,
f(un,
vn),
-1
vr
I
J,
g(un,
vn).
R,
=
M
+
L(un
+
vn)
-
[fn(Un,
vn)
+
gn(un,
vn)]
2
0
{
Sn
=
MI
+
Ll(un
+
vn)
-
[afn(un,
vn)
+
PSn(un,
vn)]
2
0

Nonlinear Reaction-Diffusion Systems
389
From
(8.20)-(8.22),
we obtain
IP-~IJ
If(Unl
vn)~
+
\g(Un,
vn)~
QT
4M1
+
2(P
+
a)h.p
+
(4L1
+
2(P
+
a)L)(un
+
Vn)
J,,
J,
+
(3(a
+
P)U?
+
(a
+
3P)(a
-
P).,".
The estimate
(8.17)
follows using also
(8.13)
and
(8.8).
Estimate
(8.17)
of Lemma
8.3
provides compactness
for
the sequence
(un,
v,)
thanks to the following result.
Lemma
8.4
For
r
=
1,2
and for all
T
>
0,
the mapping
where
(8.24)
is
compact. Moreover, it is continuous from
L'(R)xL1(Q~)
into
C([O,
TI;
L'(R)).
w(t)
=
S'(t)w,
+
Sr(t
-
s)g(s)
ds
J,'
Proof
Continuity into
C([O,
TJ);
L'(R))
is
classical. Compactness into
L'(QT)
comes from the fact that
S'(t)
is
compact
for
t
>
0
and the general
abstract result in Raras, Hassan and VQron
[4].
Proposition
8.5
quence
(unk,
vn,)
and
(u,
v)
E
L~0,([0,00];L'(R))2
such that
Under assumptions of Lemma
8.3,
there exists
a
subse-
