Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


370
R.
H.
Martin
and
M.
Pierre
first given in
[17].
A similar result was obtained in
[12].
A
different and
more general proof
is
given in next section. Note that global existence is
unresolved if
A1
=
1,
A2
=
0.
R
2.
This restriction of polynomial growth has been slightly improved to
allow
h(v)
-
eu7,
7
<
1
in
[7].
The method
is
different and seems more
specific to the particular structure
of
(3.1)
while the next section carries over
to more general systems.
R
3.
existence of classical solutions of
(3.1)
for
any
growth of
h
but with
A rather particular approach indicated in Section
7
provides global
R
=RN,
dl
5
d2.
A
completely different and very general method based on the use of
a
priori
estimates in
L'(R)
allows us to handle any kind of growth for
h
and
works for general diffusion.
However, it only provides weak solutions. In
particular, we do not know whether
or
not they remain in
Lm(R)
for all
time. On the other hand, L'-initial data are possible:
R
4.
Assume
(3.6)
holds and
u,,
v,
E
L'(R),
u.,,
V,
2
0.
Then
(3.1), (3.2)
has a global "weak" solution with
u(.,
0)
=
u,(.),
v(.,
0)
=
vo(.).
(Note
that we do not assume
(3.5)
here.) The idea
of
the proof will be indicated
in Section
8.
1.4
The
Basic
Method
for
Polynomial
Growth
The basic method will be presented here for
a
2
x
2
system with general and
diflerent
diffusions. Extensions and limits
of
the method will be described
in Sections
5
and
6.
For
r
=
1,2,
we set
(4.1)
where we use the usual summation convention on indices and
(44
ofj,
bf
E
C'(n),
cr
E
C"(n)
r
=
1,2
Aru
=
a;(u:jaj~)
+
bf&u
+
C'U
We consider the following system

Nonlinear Reaction-Diffusion Systems
371
subject to the boundary conditions
x1a;u
+
(1
-
X,)(u
-
a1)
=
0
on
8R
x
(0,~)
{
x2a;v
+
(1
-
X2)(v
-
cr2)
=
0
(4.5)
where
a:
is the conormal derivative on
8R
corresponding to
A',
that
is
a;.
=
ar.a.u
13 3
ni
where
n'
=
(nl,
.
. . ,
nN)
is
the unit outward normal derivative to
aR.
We
assume
Also,
(4.8)
f(x,t,O,v)20,
g(z,t,u,0)20
Vu,v>O,
a.e.r,t
and
(4.9)
f(z,t,u,v)
+
g(z,t,u,
v)
L(u
+
v)
+
M
Vu,
v
2
0,
a.e.2,
t
where
L,
M
2
0.
noncontinuable solution in
According to the discussions in the introduction, this system has a unique
x
[0,
?).
If?
<
00,
then
(4.10)
(see for instance
[14]
for this more general situation).

372
R.
H.
Martin and M.
Pierre
We now state a proposition which contains the main idea of the basic
method. Essentially, it exploits the "balance condition"
(4.9).
For this we
introduce the following notation.
Notation:
QT
=
Rx(0,
T),
CT
=
ORx(0,
T),
llullp
=
[s,
lu(z)[Pdz]'lP,
1IP
1
lP
(LP-estimates). Assume
(4.1)-(4.9).
For
allp
E
(1,
co)
IIullP,T
=
[J&
I'(zl
t)lPdz
dt]
1
II"IIP>CT
=
[JET
lu(b,
t)IPdudt]
'
Proposition
4.1
and
all
T
>
0,
there exists
C1, C2
such that
where
C1, C2
depend only on the data,
p
and
T.
Remark.
By symmetry, we also have
In other words, the LP-norms of
u
and
v
on
QT
can only blow up
at
the same
time
T.
As
a
consequence, the next result naturally follows from Proposition
4.1.
Theorem
4.2
Assume that the noncontinuable solution
(u,
v)
to
(4.4)-
(4.7)
on
R
x
(0,
?)
has the property that there ezists a continuous, increasing
function
N1
:
[O,co)
+
[0,
m)
such that
(4.12)
II.(.,
t)llm
I
Nl(t)
for
0
I
t
I
fF
and
that there are positive numbers
Ll(r), Ml(r)
and
n
such that
Id%
.)I
5
L1(r)lvlU
+
M1(r)
(4.13)
for all
(ti,
v)
E
[0,
00)~
with
IuI
5
r.
A
Then
T
=
00
and
hence
(u,
v)
ezists globally.
Remark.
The condition
(4.12)
is satisfied when for instance
f(u,
v)
5
Lu+M
for
u
2
0
which is the case in example of Section
1.3.
This is
a
direct consequence of
the
maximum
principle. But more general systems can be treated in this
way (see the discussion in Section
5).
Nonlinear Reaction-Diffusion
Systems
373
Proof
of
Proposition
4.1.
(4.9) we obtain
(4.14)
ut
-
A'u
+
vt
-
A2v
5
L(u
+
v)
+
M.
In order to use a duality argument, we introduce
x
E
Cm(Q~),
x
2
0
and
4
a nonnegative solution
of
By adding up the equations in
(4.4)
and using
-4t
-
(A2)*4
=
x
on
QT
(4.15)
x2(a;)*4
+
(1
-
x2)4
-
x2bz.n'4
=
o
on
cT
=
aR
x
(0,
T)
I
4(.,
T)
=
0
on
R
where
(A')*
denotes the adjoint
of
Ar
and
(a;)*
its
associated conormal
derivative
(A')*$
=
ajP(Qrjai4)
-
a,(bT4)
+
cr4,
(a;)*$
=
aijaj4nj
-+
and
br
=
(b;,
.
. .
,
bh).
Recall (see e.g.
[24])
that
4
exists and
is
nonnegative.
Moreover,
for
all
q
E
(1,
w)
and
T
>
0
there exists
C
such that
(4.16)
where
W21'*q(Q~)
=
{4
E
Lq(Q~);
natural norm (see [24, p.
1331).
II~IIWJ~~(QT)
+
II4(.,
0)llq
L
Cllxllq,~
4t,
OZ,,
E
Lq(Q~)}
with its
We multiply
(4.14)
by
eFtL4
to obtain
(4.17)
(e-tL(u
+
v))
4
-
e-lL(A'u
+
A2v)O
5
Me-tLq5.
at
Now we use integration by parts
and the same
for
u
to obtain
374
R.
H.
Martin
and
M.
Pierre
Using the definition of
d
in
(4.15),
we rewrite this
as
+
J
e-tL{d(aiu+a;u+ug
.
~+u~.ii)-u(ai)*d-u(a;)*~}.
CT
Denote by
Z1,
Z2,
Z3
the integrals on the right hand side in
(4.18)
and let
p
E
(1,
co)
and
q
=
p/(p
-
1).
Using
(4.16),
we have
(4.19)
To
bound the boundary term
Z3,
we use
(4.5)
and
(4.15).
0
<
XI,
A2
5
1.
11
+
12
5
C
[lluo
+
voIIp
+
IIullp,~
+
M(me~s
&)'"I
IIXIIq,T.
Assume first
13
=
J,,
e-tL
{-u
[&'(1
-
X1)d
-
b?.iiq!J
+
(a;)*d]
I
(ad;'
+
a2x;')
J
4
+
CII"IIP,CT
.
IIdIILn(O,T;W'~~(an))
(4.20) (aiXT1(1
-
Xi)
%&'(1
-
A2))
d}
CT
where
C
depends only
on
a:j,
3,
A'.
By
(4.16)
and the continuity
of
the
trace operator from
W2iq(!2)
into
W1*q(d!2),
(see
[14]),
we obtain
(4.21)
If now
X1
=
X2
=
0,
then
0
=
0,
u
=
01,
u
=
a2
on
80
so
that
13
can be
bounded by
Z3
5
[
(al~;'
+
a2~;')
(meas
G)'/P
+
CIbllP,cT]
c11x11q,T.
13
L
J,
a1
l(ai)*dl+a21(a,2)*dI
I
c(al+az)(meQs
~T)'~p11411L~(O,T;w~~~(an)),
T
or,
by
(4.16)
and the continuity of the trace
(4.22)
13
I
C(a1
+
a2)(meQs
RJyPCIIXIlq,T
Finally, from
(4.18), (4.19), (4.21), (4.22)
we obtain the existence of
(21,
C2
depending only on the data and
T
such that
Nonlinear Reaction-Diffusion Systems
375
Since
x
is
arbitrary nonnegative in
C,”(Q,)
and
u,
v
2
0,
this implies
The result of Proposition
4.1
follows.
Remark.
According to the proof above, it appears that we do not need
the term
IIuJlp,~,
in
(4.11)
if
0
=
A1
=
A2
(see
(4.22))
or
if there exists
d
>
0
such that
This can be seen from
(4.20).
This
is
the case if
A’
=
dA2
and
A1
5
A2/(d+
A2
-
dAz)
which essentially means that the boundary conditions are
of the same type for
u
and
v.
Proof
of
Theorem
4.2.
applied with
T
=
?
shows
If
(4.12)
holds, then
(4.11)
in Proposition
4.1
where
C1,
C2
depend on the data and on
?.
Therefore, for all
p
<
00,
the
LP-
norms of
u
and
v
are finite on
Q-
From the polynomial growth assumption
?-.
(4.13),
this implies that
g(u,
v)
is
also in
Lp(Q~)
for
all
p
E
(1,
m).
Going
back to the second equation in
(4.4)
and taking
p
>
(N
+
1)/2,
we deduce
that
v
is
Lo3(Q~)
(see
[14]):
.-.
This implies
T
=
00.
1.5
Extensions
of
the
Basic
Method
The method described in Section
4
applies to a large class of sytems. One
of them
is
the widely analyzed so-called “Brusselator” which appears
as
an
important model in chemical reactions and has the form

376
R.
H.
Martin
and
M.
Pierre
where
A
and
B
are positive constants and the boundary conditions are
as
in
(3.2).
The usual boundary conditions for this model are
u=
B/A, v=A
or
&u=&v=O
on
dC2x
(0,m).
Note that
u
=
B/A
and
v
=
A
is
an equilibrium solution in each of these
cases.
Theorem
4.2
applies in this situation and the only nonobvious hypothesis
to
verify is the
a
priori
Loo-estimate
(4.12)
on
u.
One way
is
to
argue
as
follows (a second way is described later). Choose any
t,
>
0
and use the
inequality
vt
2
dzAv
-
(B
+
1)”
+
A
to
show that
m(t)
=
min{v(z,
s);
2:
E
2,
to
5
s
5
t}
>
o
for all
t
>
to
where the solution exists. Now this estimate can be used
in the first equation in
(5.1)
to
obtain the Lm-estimate on
u
[observe that
-uv2
+
Bv
=
v(B
-
uv)
5
v(B
-
m(t)u)]. Details can be found in Hollis,
Martin, Pierre [9]. Thus Theorem
4.2
applies
to
the Brusselator.
A
more
careful use of these techniques shows further
that
each solution
to
(5.1)
is
uniformly bounded,
see
[9]:
SUP
114.7
t)llm
+
11v(.,
t)lloo
<
00.
t>o
In fact the work of BCnilan and Labani
[5]
establishes the existence
of
a
constant
C,
independent of the initial conditions
u,
and
v,,
such that
lim SUP
II4.j
t)lloo
+
IIv(.,
t)lloo
I
c.
t+m
In particular, this shows there
is
a
compact attractor for the solutions
of
(5.1).
Instead of assuming Loo-estimate
(4.12)
in Theorem
4.2,
we could have
more generally assumed that the nonlinear term in the first equation is
bounded above by
a
linear
function of
u
and
v
as
in the Brusselator. Indeed,
by Proposition
4.1,
we know that LP-norms of
u
and
v
on
QT
are essentially
equivalent (the term
IIuIIP,cT
can be dispensed with if the boundary condi-
tions are of the same type for
u
and
u).
Next, we can use
a
Gronwall’s type
inequality
to
obtain an LP-bound on
u.
For
instance, for the Brusselator
with
8,u
=
a,v
=
0,
we have
Nonlinear Reaction-Diffusion Systems
377
which implies by
(4.11)
that
Il~(t)llP
I
CI(t)
+
C2(t)llu(t)llp,t
Setting
g(t)
=
Ilu(t)llF,
this can be written
t
P
g(t)
I
Cl(t)
+
C2(t)
[ltg(s)ds]l’p}
I
2’Ci(t)
+
2’C2(t)L
g(s)ds,
which
is
a linear Gronwall inequality. Solving this inequality bounds
llullp,t
for
t
<
00.
In particular this method yields global existence in
any dimension
for
systems
like
the following studied in [23]:
{
ut
-
dlAu
=
w
-
uv
vt
-
d2Av
=
w
-
uv
on
R
x
(0,
M)
Wt
-
d3Aw
=
uv
-
w
&-av-aw=o
an
-
an
-
an
on
aR
x
(0,
m).
All these “LP-techniques” can also be extended to
m
x
m
systems. Let
us
describe the kind
of
assumptions that are needed for these methods to
apply. Consider
uf
-
d;Au’
=
fi(u)
on
R
x
(0,
T)
?&=(I
on
aR
x
(0,
T)
where
u
=
(u’,
. .
.
,urn)
and
d;
>
0.
Assume
f
=
(ti)
is
locally Lipschitz
continuous and quasi-positive (see
(1.1)).
Assume moreover that
there exists
a
m
x
m
triangular and invertible
matrix
P
with
nonnegative entries,
C
>
0
and
6
E
Rm
such that
VrERm,
r20,
Pf(r)<Cr+b
(5.3)
the growth
off
is
at most polynomial.
Then (5.2) has a global classical solution
for
all
u,,
v,
€
Lm(R)
with
u,,
v,
2
0
(see
[19]
for
a
proof). The linear upper bound in
(5.3)
can even be weak-
ened to some polynomial upper bound with small degree depending on the
dimension
(see
again
[19]).
I
where the usual order in
Rm
is
used, and
(5.4)

378
R.
H.
Martin
and
M.
Pierre
This method also covers the case when reaction terms satisfy some
“Liap unov- typ e” condition such
as
(5.5)
VH(r)
*
f(r)
5
CH(r)
+
b
where
H(r)
=
CEl
H;(r;)
with
H;
:
[O,m)
+
[0,00)
convex, regular and
Hi(r;)
+
00
as
r;
+
00.
Indeed, it
is
easy to check that if
u
is
a
nonnegative
solution of (5.2), then
vi
=
Hi(u1)
is
a subsolution
of
vf
-
d;Av’
5
H,!(u’)f;(u),
i
=
1,.
. .
,
m
Then (5.5) appears for system
(5.6)
as
one of the
linear
relations (5.3).
Since the method carries over to nonnegative subsolutions (and not only
solutions), global existence will also be obtained for
m
x
m
systems satisfying
m
conditions like (5.5) with a
triangular structure.
All of these ideas are
combined in
[19]
where details and several examples are given. Note that
uniform Loo-bounds in time can also be obtained in that framework (see
Finally we mention some similar results in [12] with
R
=
RN.
It
is
also
POI
).
proved that if
only one
balance relation holds in an
m
x
m
system like
m
C
fi(u)
=
0
i=
1
(5.7)
and if each
fi
has less than quadratic growth, then global existence follows
from direct
LO3
estimates on
Vu.
1.6
Limits
of
the Method and
Open
Problems
Although many systems can be treated by the above method, it
is
not suf-
ficient to understand all the systems for which the two main properties of
positivity and preservation
or
dissipativity of mass hold. We will list here a
few difficulties that cannot be overcome by above approach and we will illus-
trate them by significant open problems. It would be interesting to develop
new techniques to treat them
or
to provide good and significant negative
results like actual blow up in finite time. We will successively consider the
following questions
-
“Non-
t
riangu lar” sys terns
-
“Critical” boundary conditions
-
Nonlinear diffusions
-
Growth faster than polynomial
Nonlinear Reaction-Diffusion Systems
379
1.6.1
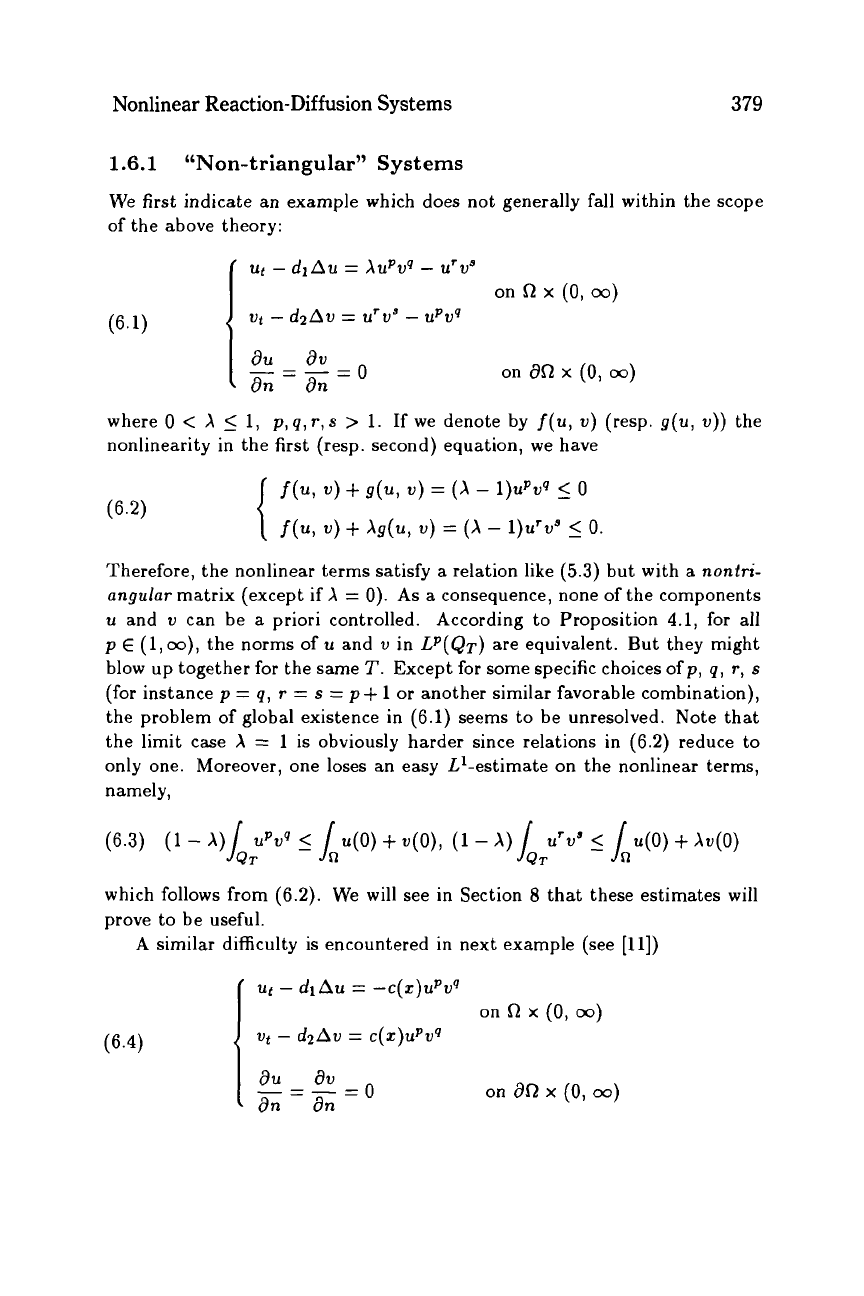
“Non-triangular” Systems
We first indicate an example which does not generally fall within the scope
of the above theory:
au
av
-=-
=o
an
an
on
aR
x
(0,
m)
where
0
<
X
5
1,
p,
q,
r,
s
>
1.
If
we denote by
f(u,
v)
(resp.
g(u,
v))
the
nonlinearity in the first (resp. second) equation, we have
f(l.6,
v)
+
g(u,
v)
=
(A
-
1)UPV‘
I
0
f(U,
v)
+
Xg(u,
v)
=
(A
-
1)U‘V’
5
0
{
Therefore, the nonlinear terms satisfy a relation like
(5.3)
but with a
nontri-
angular
matrix (except if
X
=
0).
As
a consequence, none of the components
u
and
v
can be
a
priori controlled. According to Proposition 4.1, for all
p
E
(1,
oo),
the norms of
u
and
v
in
LP(QT)
are equivalent. But they might
blow up together for the same
T.
Except for some specific choices ofp,
q,
r,
s
(for instance p
=
q,
r
=
s
=
p+
1
or
another similar favorable combination),
the problem
of
global existence in
(6.1)
seems to be unresolved. Note that
the limit case
X
=
1
is
obviously harder since relations in (6.2) reduce to
only one. Moreover, one loses an easy L’-estimate on the nonlinear terms,
namely,
which follows from (6.2).
We
will see in Section
8
that these estimates will
prove to be useful.
A similar difficulty
is
encountered in next example (see
[ll])
~t
-
dlAu
=
-c(z)uPvQ
on
R
x
(0,
00)
ut
-
d2Av
=
C(Z)UPVQ
(6.4)
au
av
I
%=an-
-0
on
8R
x
(0,
m)
