Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.

250
Benno Fuchssteiner
Indeed, these commutator relations are
a
simple consequence of the hereditary property of
a.
One should observe that the relation
[rn,Iim]
=
(m
+
p)Ii,+,
is equivalent to the fact
that
r,
+
(m
+
p)Iin+m
is
a
time-dependent symmetry group generator of
ut
=
Km(u).
A
Lie algebra consisting of
r’s
and
K’s
fulfilling (8.9) is called
a
hereditary algebra.
These
scaling symmetries exist for almost all popular soliton equations, and even in those cases
where
a
scaling symmetry
or
a
hereditary cannot be found one can nevertheless construct
a
suitable hereditary algebra. For example, in the KdV case
ro(u)
=
izuz
+
u is the scaling
symmetry, and for the mKdV and the potential sine-Gordon one finds
ro(u)
=
xu,
+
u.
From the invariance of the symmetry group generators one finds that the submanifold
(see for example
[19])
N
MN
=
{
u
1
there exists
on
such that
anIin
=
0)
(8.10)
is invariant under any of the flows ut
=
Iin(u),
in particular under
ut
=
Zirl(u).
This
manifold is called the manifold
of
N-soliton solutions.
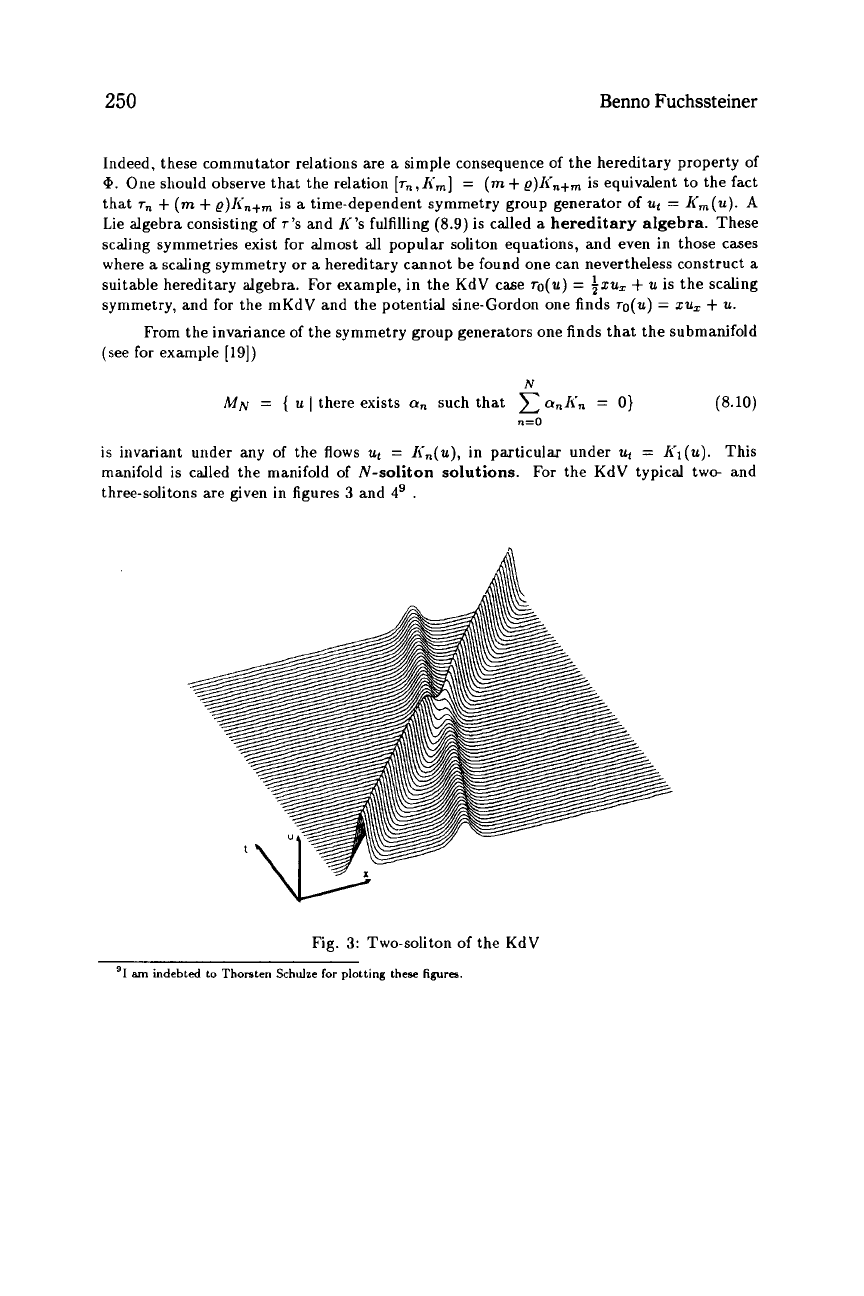
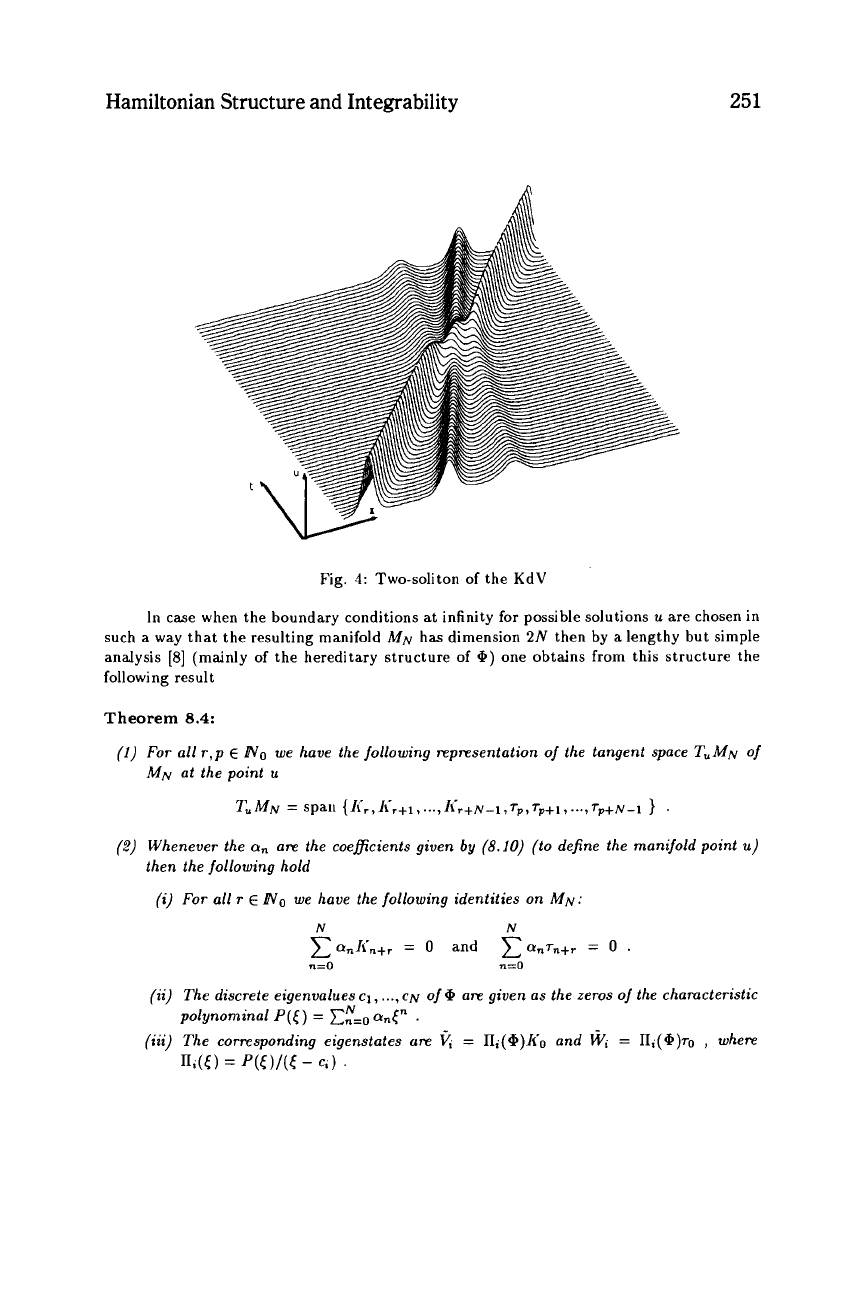
For the KdV typical two- and
three-solitons are given in figures
3
and
4’
.
n=O
Fig.
3:
Two-soliton of the KdV
’1
am
indebted to Thornten Schulze
lor
plotting these
figures.
Hamiltonian Structure and Integrability
251
Fig.
4:
Two-soliton
of
the KdV
In
case when the boundary conditions at infinity
for
possible solutions
u
are chosen in
such
a
way that the resulting manifold
MN
has dimension
2N
then by
a
lengthy but simple
analysis
[8]
(mainly
of
the hereditary structure
of
a)
one obtains from this structure the
following result
Theorem
8.4:
(I)
For
all
r,p
E
NO
we have the following representation
of
the tangent space
T,MN
of
MN
at the point u
T,MN
=
spati
{lir,li,+l,.
..,
lir+N-1,rp,r~+l,...,Tp+N-~
}
.
(2)
Whenever the
a,
are
the coeficients given by
(8.10)
(to
define the manifold point u)
then the following hold
(i)
For
all
r
E
No
we have the following identities on
MN:
(ii) The discrete eigenvalues
c,,
...,
CN
of+
are
given
as
the zeros of the characteristic
(iii) The corresponding eigenstates
are
=
II,(@)Ko
and
W,
=
II,(@)To
,
where
polynominal
P(()
=
I,”=,
antn
.
K(C)
=
P(€)/(<
-
ct)
’

252
Benno
Fuchssteiner
As
a
direct consequence of Theorem
8.4
we obtain that the recursion operator
CP.
leaves the
tangent space
T,MN
of the reduced manifold invariant. Hence, the restriction
6
:=
@ired
of
CP
to
MN
is
a
linear operator on
a
finite dimensional space. This operator
6
has the
properties listed below in:
Observation
8.5:
(1)
6
is invertible and can
be
written as
6
=
&e;'
where
&,
o2
are a compatible pair
of
implectic opemtors.
(2)
The eigenvalues el,
...,
CN
of
6
are doubly degenemted.
(3)
Renorming the eigenstates
c,W,
leads
to
eigenstates
V,
and
W,
which are hamiltonian
vectorjelds w.r.1.
61
and
62.
(4)
The eigenstates
V,
and
W,
fulfil the commutator relations
(8.11)
These last two results show that the finite dimensional reductions, given by those members
of
the abelian symmetry group which is generated by the bi-hamiltonian formulation is, under
suitable boundary conditions at infinity, the same situation
as
we found in the completely
integrable finite dimensional case.
Since the eigenstates
l<,Wi
are hamiltonian vector fields and since they fulfill the
canonical commutator relations
(8.9),
their potentials can be interpreted as action/angle
variables
for
the flow induced by (1.1) on
MN.
Although all
our
considerations were of
a
purely algebraic nature we should remark
that in most cases which are relevant from the physical viewpoint the N-soliton solutions
(with vanishing boundary conditions at infinity) are those solutions which decompose into
N
single waves
for
t
-
foo
N
UN
2
Si(X
+
Cit
-k
9;)
.
i=l
This can be seen for the KdV from Figures
4
and
5.
By comparison with the asymptotic
data we get in these case we get a simple method for finding the eigenstates
of
the recursion
operator.
Observation
8.6:
Taking the partial derivatives of
UN
w.r.1. the asymptotic data
auN
@!!
and
-
8%
ac;
one obtains eigenstates of the recursion operator
6
for the eigenvalue
c;.
The function
auN/aq,
then is the vector field corresponding
to
the action variable
and
this is called the

Hamiltonian Structure and Integrability
253
interacting
soliton
171.
In
case of the KdV equation a plot of such quantities is easily obtained (see Figure
5)
Fig.
5:
Interacting soliton
of
the
KdV
A
corresponding conjugate eigenstate for the KdV is obtained
by
taking the derivative
of the field function
UN
with respect to the parameters given
by
an eigenvalue of the
recursion operator.
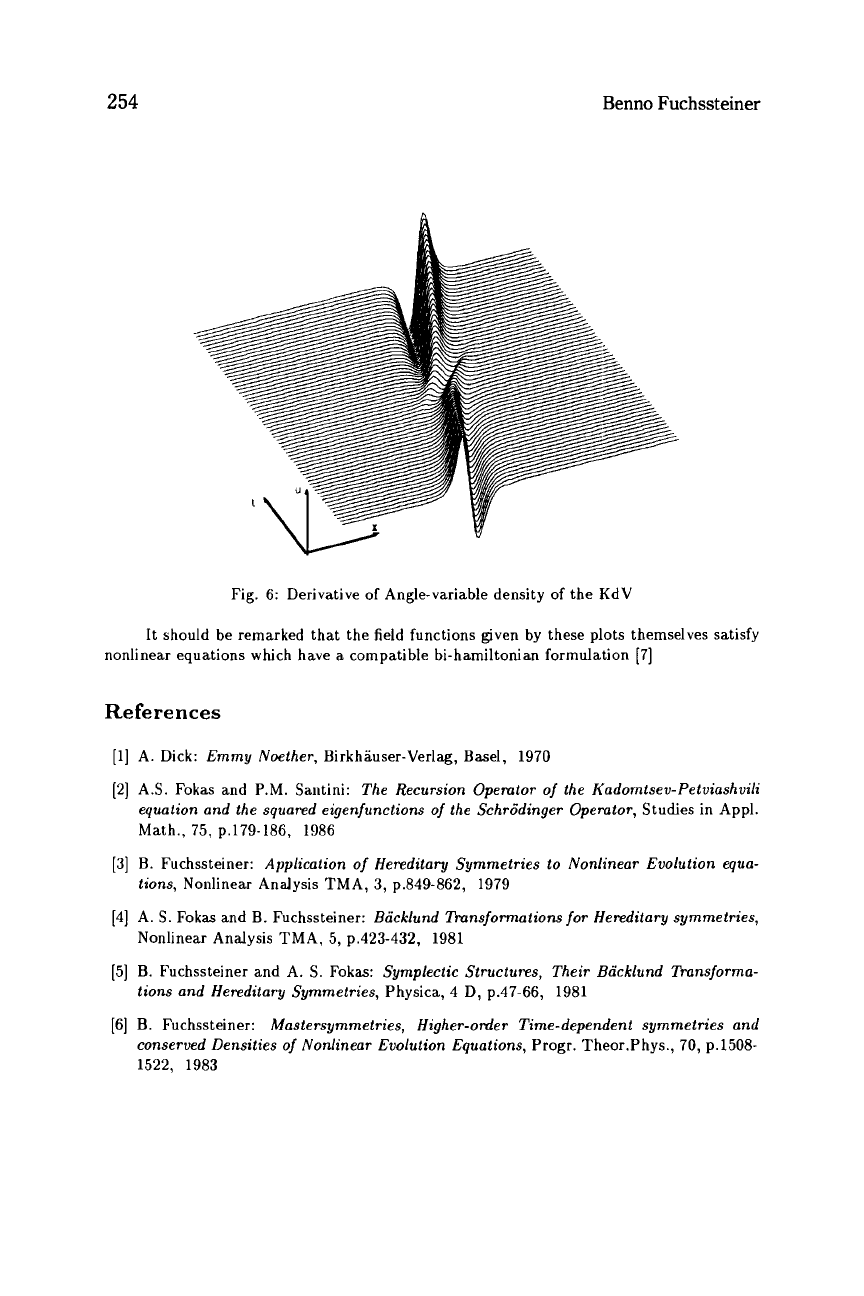
254
Benno Fuchssteiner
Fig. 6: Derivative
of
Angle-variable density of the KdV
It should be remarked that the field functions given by these
plots
themselves satisfy
nonlinear equations which have
a
compatible bi-hamiltonian formulation [7]
References
[l]
A.
Dick: Emmy Noether, Birkhauser-Verlag, Basel, 1970
(2)
AS. Fokas and P.M. Santini: The Recursion Operator of the Kadomtsev-Petviashvili
equation and the squared eigenfunctions of the Schrddinger Operator, Studies
in
Appl.
Math., 75, p.179-186, 1986
[3] B. Fuchssteiner: Application
of
Hereditary Symmetries
to
Nonlinear Evolution equa-
tions, Nonlinear Analysis TMA, 3, p.849-862,
1979
[4] A.
S.
Fokas and
B.
Fuchssteiner: Backlund Transformations for Hereditary symmetries,
Nonlinear Analysis TMA, 5, p.423-432, 1981
[5]
B.
Fuchssteiner and
A.
S.
Fokas: Symplectic Structures, Their Backlund Transforma-
tions and Hereditary Symmetries, Physica, 4 D, p.47-66, 1981
[6] B. Fuchssteiner: Mastersymmetries, Higher-order Time-dependent symmetries and
conserved Densities
of
Nonlinear Evolution Equations, Progr. Theor.Phys., 70, p.1508-
1522. 1983

Hamiltonian Structure and Integrability
255
[7]
B. Fuchssteiner:
Solitons in Interaction,
Progress
of
Theoretical Physics,
78, p.1022-
1050, 1987
[8]
B. Fuchssteiner and G. Oevel:
Geometry and action-angle variables
of
multisoliton
systems,
Reviews
in
Mathematical Physics,
1,
p.415-479, 1990
[9]
B. Fuchssteiner:
Compatibility in abstract algebraic Structures: Application
to
nonlin-
ear systems,
Paderborn, preprint,
1990
[lo]
1.M.Gelfand and
I.Y.
Dorfman: Funktsional’nyi Andiz
i
Ego
Prilozheniya,
Hamiltonian
Operators and Algebraic Structures related
to
them,
13, p.13-30, 1974
[I11
1.M.Gelfand and I.Y. Dorfman:
Funktsional’nyi Analiz i Ego Pnlozheniya,
The
Schouten bracket and Hamiltonian operators,
14, p.71-74, 1980
[
121
1.M.Gelfand and
I.Y.
Dorfman: Funktsional’nyi Analiz
i
Ego Prilozheniya,
Hamiltonian
Operators and Infinite-Dimensional Lie-Algebms,
15, p.23-40, 1981
[13]
D. J. Korteweg and G. De Vries:
On
the change of form
of
long waves advancing in
a
rectangular canal, and
a
new type
of
long stationary waves,
Philos.Mag.Ser.
5, 39,
p.422-443, 1895
[14]
F. Magri:
A simple model
of
the integrable Hamiltonian equation,
J.Math.Phys.,
19,
p.1156-1162, 1978
[15]
F. Magri:
A Geometrical Approach
to
the Nonlinear Solvable equations,
Nonlinear
Evolution equations and Dynamical Systems
(M.
Boiti,
F.
Pempinelli, G. Soliani eds.)
Springer Verlag,
p.233-263, 1980
(161
J.
Marsden:
Applications
of
Global
Analysis in Mathematical Physics,
Publish
or
Per-
ish, Inc.,
2,
Boston,
1970
(17)
E.
Nelson:
Tensor Analysis,
Princeton University Press, Princeton NJ,
1967
[
181
E.
Noether:
Invariante Variationsprobleme,
Nachr.v.d. Gesellschaft d.Wiss. zu
Gottingen,
p.235-257, 1918
[I91
S.P. Novikov,
S.
V. Manakov,
L.
P.
Pitaevskii and V.
E.
Zakharov:
Theory
of
Solitons,
The Inverse Scattering Method,
Consultants Bureau, New York-London,
1984
[20]
P.J. Olver:
Evolution Equations possessing infinitely many symmetries,
J.Math.Phys.,
18, 1977 p.1212-1215,
[21]
C.
Rebbi and G. Soliani:
Solitons and Particles,
World Scientific, Singapore,
1984
[22]
P.M. Santini and A.S.
Fokas:
Recursion Operators and Bi-Hamiltonian Structures
of
2+1
dimensional systems,
in: Topics in Soliton Theory and Exactly solvable Nonlinear
equations
(
eds: M. Ablowitz, B. Fuchssteiner, M. Kruskal) World Scientific Publ.,
Singapore,
1987 p.1-19,

256
Benno Fuchssteiner
[23] P.M. Santini and A.S. Fokas:
Recursion Operators and Bi-Hamiltonian structures in
Multidimensions.
I,
Cornrnun.
Math. Phys., 115, p.375-419,
1988
(241 P.M. Santini, A.S. Fokas:
Recursion Operators and Bi-Hamiltonian structures an Mul-
tidimensions.
Il,
Cornm. Math. Phys., 116, p.449-474, 1988
[25]
B.
Schutz:
Geometrical Methods
of
Mathematical Physics,
Cambridge University Press,
Cambridge-London-La Rochelle-New York-Melbourne-Sidney, 1980
[26]
S.
Yarnamuro:
Differential Calculus in Topological Linear Spaces,
374, Springer Verlag,
Berlin- Heidelberg-New York, 1974
[27]
S.
Yamarnuro:
A
theory
of
differentiation in locally convez spaces,
AMS, Providence,
Rhode Island, 1979

Symmetric
Chaos
Greg
King and Ian Stewart
Nonlinear Systems Laboratory
Mat
hematics
Inst
i
t
Ute
University
of
Warwick,
Coventry
CV4
7AL,
England
Abstract
Chaos is the occurrence of highly irregular behaviour in
a
deter-
ministic dynamical system. It is well known that both ordered and
chaotic states can be obtained in such systems by varying parameters.
In contrast we here consider circumstances in which aspects of both
order and chaos may be present in the same behaviour and
at
the
same parameter values. Namely, in
a
dynamical system with symme-
try, chaotic attractors may themselves possess
a
degree
of
symmetry.
The non-chaotic dynamics of symmetric systems has attracted in-
creasing attention of late, and the time appears to be ripe to extend
the investigation into the chaotic regime.
We describe some experimental situations in which this type of
behaviour appears to be implicated, including patterned turbulence
in fluids and nonlinear oscillations in electronic circuits. We describe
sample results from various numerical experiments, to illustrate some
of the phenomena that can occur, and describe some simple but fun-
damental mathematical results that go some way towards explaining
them. The field has many open questions, some of which are indi-
cated.
1
Introduction
Recent years have seen
a
tremendous flowering of the theory of deter-
ministic chaos: the occurrence in non-random dynamics of highly ir-
regular and apparently stochastic behaviour: see CvitanoviE
[13],
De-
Nonlinear Equations in the Applied Sciences
257
Copyright
0
1992
by
Academic Press, Inc.
All
rights
of
reproduction
in
any
form reserved.
ISBN 0-12-056752-0

258
Greg
King
and
Ian
Stewart
vaney [14], Guckenheimer and .Holmes [22]. Another development
of
some interest has been the growing recognition of symmetry-breaking
in equivariant (symmetrical) dynamical systems
as
a
mechanism for
pattern-formation, Golubitsky
et
al.
[19]. The aim of this paper is
to describe recent attempts
to
combine the two ideas by considering
chaotic dynamics in equivariant dynamical systems, thereby creating
a
framework for the study of patterned chaos. Our treatment is not
exhaustive: material not discussed here can be found in Crowe
et
al.
[12], Franjioni
et
al.
[17], Milnor [27], and Piiia and Cantoral [29].
As motivation we have selected the phenomenon of turbulent Tay-
lor vortices in the Taylor-Couette system (section 2). We choose this
not because it has been rigorously explained
-
indeed the resolution
of
its paradoxical features reported here and due to Golubitsky re-
mains conjectural
-
but because it embodies the precise combination
of symmetry and chaos that we aim to capture. General background
on symmetry in dynamics is sketched in section
3.
In section
4
we
exemplify some
of
the phenomena that can occur in
a
series
of
nu-
merical experiments: the cubic logistic map on the line; mappings of
the plane equivariant under the dihedral group
DJ;
and mappings of
the 2-torus equivariant under an action of
Dq.
Section
5
collects together some simple but useful theorems from
the literature and folklore
of
the subject. The first addresses the issue
of
the occurrence of ‘interesting’ dynamics in equivariant diffeomor-
phisms
as
opposed to mappings; the second ‘explains’ the occurrence
of symmetry-increasing crises; and the third demonstrates that the
symmetries of strange attractors may differ from those possible for
fixed points. Section
6
enters more deeply into the mathematics
of symmetric chaos and sketches some recent results of Krupa and
Roberts
(251
on symmetry-locking in equivariant circle maps.
In section
7
we return to applications with
a
survey of several
experiments on symmetric chaos in electronic circuits: the Van der
Pol-Duffing oscillator with cubic
or
quintic characteristic, and sys-
tems
of
coupled identical oscillators. These systems exhibit many of
the types of behaviour predicted theoretically: in particular the oc-
currence of symmetry-increasing crises. The general issue of extract-
ing symmetries of strange attractors from time series is addressed

Symmetric
Chaos
259
in section
8;
and in section
9
we return to the motivating problem
of turbulent Taylor vortices to describe
a
plausible scenario for their
formation, due to Chossat
and
Golubitsky, and to discuss
a
possible
method for testing this scenario experimentally.
2
Patterned
Chaos
Interest in chaotic dynamics
of
symmetric systems arises from the
occurrence in experiments of ‘coherent structures’: dynamical states
that combine local chaos with global pattern. In this section we de-
scribe one example of this phenomenon, to provide
a
degree of mo-
tivation for the more theoretical work to be described below, whose
connections with applications are currently stimulating but specula-
tive. The classic example of what we have in mind is the formation
of
turbulent Taylor vortices in Taylor-Couette
flow,
see Fensterma-
cher
et
al.
[15].
The experimental apparatus comprises
two
coaxial
cylinders, with fluid confined between them,
see
Figure
1.
In early experiments the outer cylinder is fixed and the inner
one rotates; more recently both cylinders are free to rotate.
If
the
outer cylinder is held fixed and the inner cylinder is slowly speeded
up, then
a
variety of patterned flows is observed. For suitable gap
widths the ‘main sequence’ of bifurcations takes the following form:
1.
Couette flow.
Laminar
flow
depending only on the radial posi-
tion: featureless.
2.
Taylor vortices.
The flow stratifies into horizontal vortex lay-
ers, with the axial velocity components in neighbouring vortices
acting in opposite directions.
3. Wawy vortices.
The interface between neighbouring vortices
develops
a
wavelike structure, which travels azimuthally with-
out changing form.
4.
Modulated wavy vortices.
The wavy interface begins to oscillate
axially
as
well
as
rotating azimuthally.
