Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


190
A.
S.
Fokas
where
P*
are the usual projection operators in
k
and
Conversely, given inverse data
Tf,
equation
(4.12)
yields
po.
Then the potential
Q
can be reconstructed from
Q(z,
y)
=
-i
lim
kjpo(z,
y,
k)
Ikl+m
The first equation
in
(4.14)
follows from the large
k
asymptotics
of
(4.9),
while the second equation follows from the large
k
asymptotics
of
(4.12).
The above analysis shows that
Q
can be reconstructed from
knowledge of
T.
If
Q
evolves in time according to the N-wave equa-
tions
(4.1),
then it is easy to show that the time evolution of
T
is
given by
(4.15)
dT
-
=
ilCT
-
ikTC,
dt
T(1,
k,
0)
=
To(1,
k).
5
A
Cauchy Problem for the KPII Equation
The KP equation is given by
(qt
+
6qqz
+
qzzz)z
+
3u2qyy
=
0
(54
where
u2
=
fl.
The choice
u2
=
-1
is frequently refered to as KPI,
u2
=
1
as KPII. The KP equation plays the role in
2+ 1
that the KdV
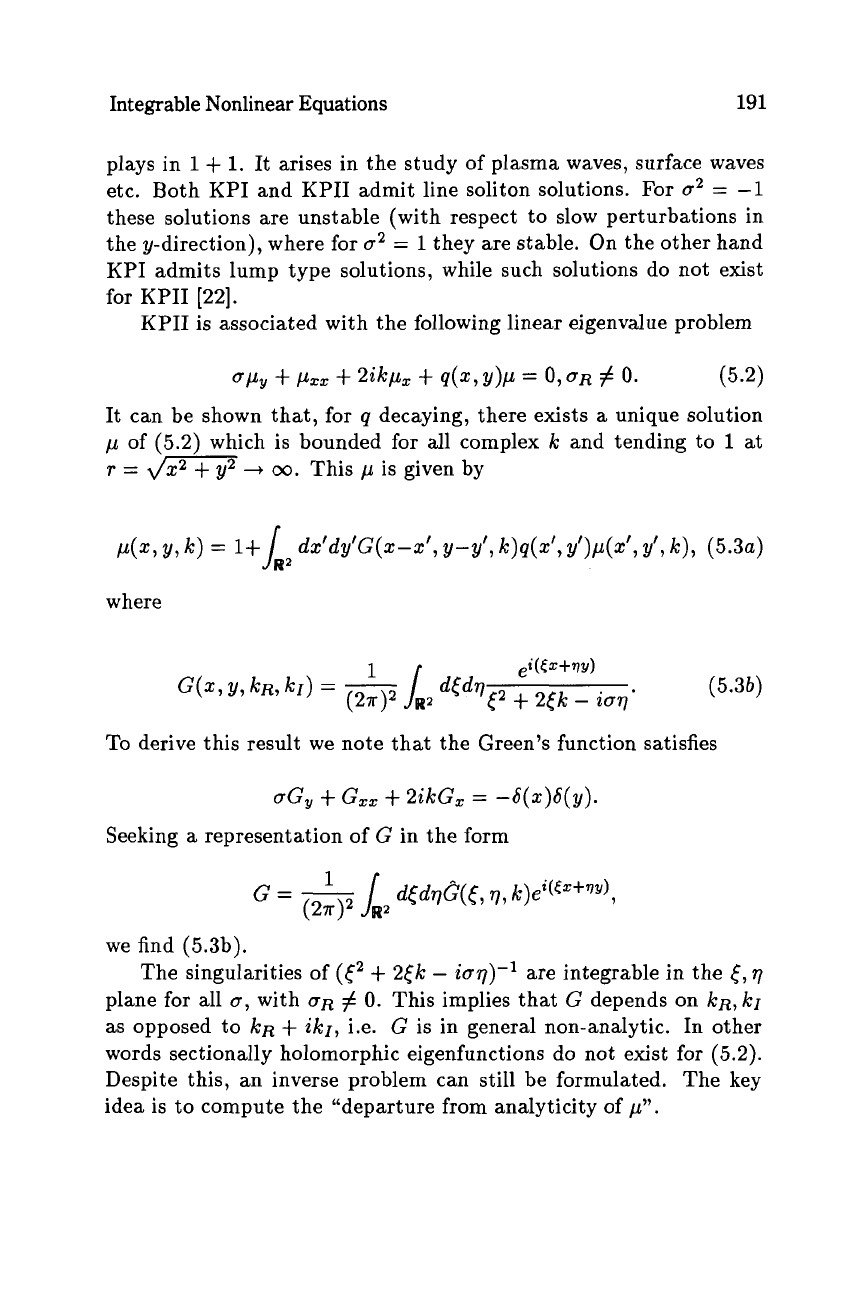
Integrable Nonlinear Equations
191
plays in
1
+
1.
It
arises in the study of plasma waves, surface waves
etc. Both KPI and KPII admit line soliton solutions.
For
u2
=
-1
these solutions are unstable (with respect to slow perturbations in
the y-direction), where for
c2
=
1
they
are
stable. On the other hand
KPI admits lump type solutions, while such solutions do not exist
for
KPII
[22].
KPII is associated with the following linear eigenvalue problem
upy
+
pxx
+
2ikpx
+
q(3,
Y)p
=
0,
OR
#
0.
(5.2)
It can be shown that, for
q
decaying, there exists
a
unique solution
p
of
(5.2)
which is bounded for
all
complex
k
and tending to
1
at
T
=
dm
+
00.
This
p
is given by
where
To derive this result
we
note that the Green’s function satisfies
uGY
+
GXX
+
2ikGx
=
--S(X)~(Y).
Seeking
a
representation of
G
in the form
we find
(5.3b).
The singularities of
(t2
+
2tk
-
iuq)-’
are integrable in the
t,
q
plane for all
u,
with
UR
#
0.
This implies that
G
depends on
kR, kz
as opposed to
kR
+
ikl,
i.e.
G
is in general non-analytic. In other
words sectionally holomorphic eigenfunctions do not exist for
(5.2).
Despite this, an inverse problem can still be formulated. The key
idea is to compute the “departure from analyticity of
p”.

192
A.
S.
Fokas
We will show that if
p
solves
(5.3)
then
where
fJz
..
fJI
kZ
ko
+
kR+-ki, ko
+
-
(kR
+
2-k1)
fJR
,p(x,
y,
kR, kI)
+
-2 (x
+
2%~)
k
fJR
(5.5)
and the
inverse data
T
is defined by
implies
Different-ating
(5.3)
w.r.t.
and using
(5.7)
we find
where
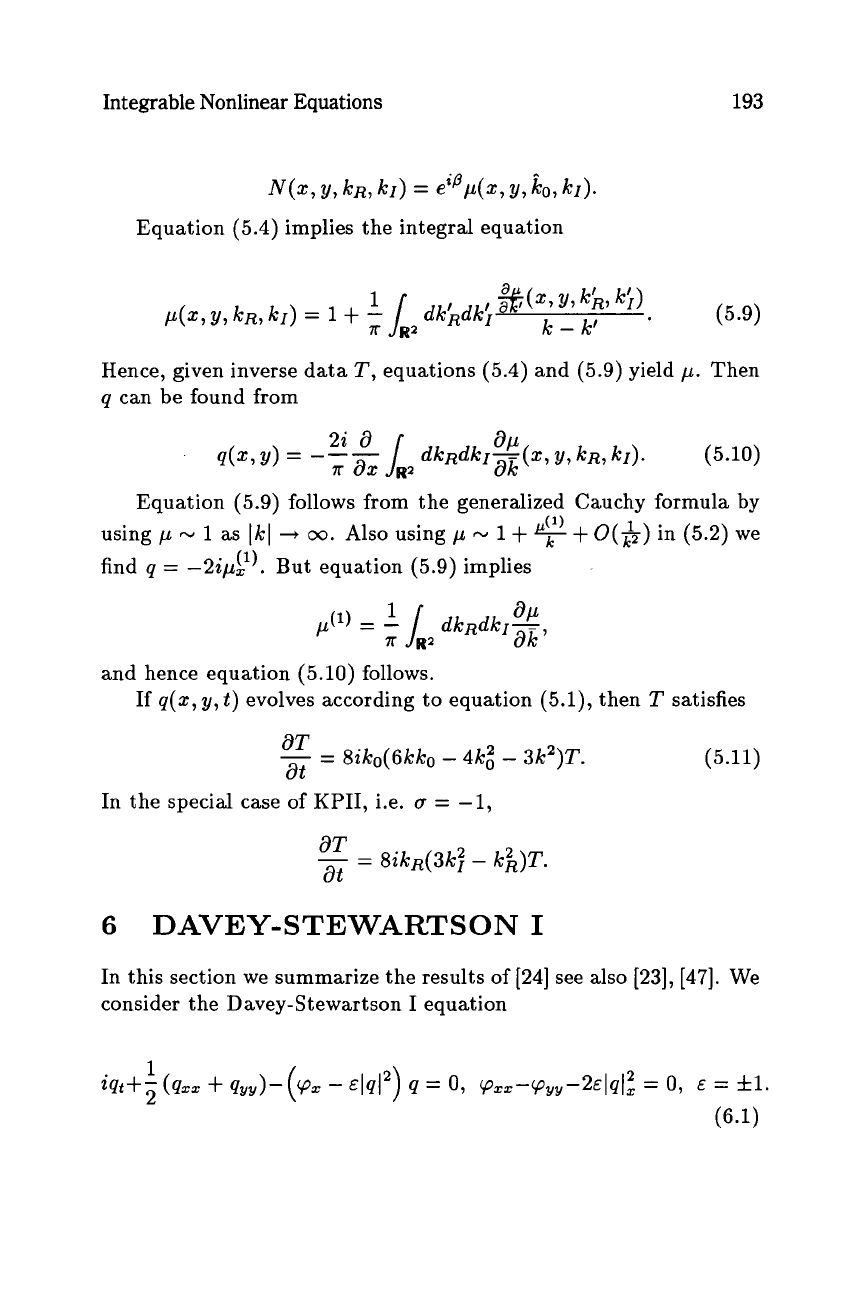
N
solves
N(x,
y,
kR, kl)
=
eiP(x9y7kR,kr)
f
i2
dx'dy'GqN.
Multiplying the above equation by
e-@
and using the symmetry
condition
Integrable Nonlinear Equations
193
N(x,
Y,
kR, kI)
=
ei’p(x,
Y,
h,
kI).
Equation
(5.4)
implies the integral equation
Hence, given inverse data
T,
equations
(5.4)
and
(5.9)
yield
p.
Then
q
can be found from
Equation
(5.9)
follows from the generalized Cauchy formula by
using
p
N
1
as
lkl
+
00.
Also using
p
N
1
+
+
+
O($)
in
(5.2)
we
find
q
=
-2ipC).
But equation
(5.9)
implies
(1)
and hence equation
(5.10)
follows.
If
q(x,
y,
t)
evolves according to equation
(5.1),
then
T
satisfies
-
8iko(6kko
-
4k:
-
3k2)T.
3T
at
--
(5.11)
In the special case
of
KPII,
i.e.
cr
=
-1,
aT
-
=
8ik~(3k;
-
ki)T.
at
6
DAVEY-STEWARTSON
I
In this section we summarize the results of
[24]
see also
[23], [47].
We
consider the Davey-Stewartson
I
equation

194
A.
S.
Fokas
This equation provides
a
two-dimensional generalization of the cel-
ebrated nonlinear Schrodinger equation and can be derived from
rather general asymptotic considerations.
Physical applications in-
clude plasma physics, nonlinear optics and water waves. In the above
q(x,y,t)
is the amplitude of
a
surface wave packet and
cp
is the ve-
locity potential of the mean flow interacting with the surface wave.
One assumes small amplitude, nearly monochromatic, nearly one-
dimensional waves. In the context of water waves equation
(6.1)
is
the shallow water limit of the Benney-Roskes equation, where one as-
sumes dominant surface tension;
E
=
-1
and
E
=
1
indicate focusing
and defocusing regimes respectively.
Introducing characteristic coordinates,
5
=
2
+
y,q
=
2
-
y,
equations
(6.1)
yield
where
We shall solve an initial-boundary value problem for the above sys-
tem, i.e. given initial data
q(t,
q,O)
and boundary data
ul(q,
t),
ug(<,
t),
we shall determine
q((,
7,t).
In particular we are interested in find-
ing the effect on the surface wave of imposing an external mean
flow
(ul(q,t),u2(t,t))
far upstream in the
(t,q)
plane. We assume
that
q(t,
q,
0),
q(q,
t),
u2(<,
t)
are decaying for large values of
6,q
and
bounded for all
t.
Equation (6.2a) is associated with the Lax equation

Integrable Nonlinear Equations
195
We let
!P
=
Mezp[ik(Jz
-
y)],
we use characteristic coordinates
and we define the analytic eigenfunction
M+
as the solution of the
Volterra integral equations
M+
satisfies (6.4a) and it is also analytic in the upper half k-complex
plane. Similarly if
M-
satisfies equations similar to those of (6.5),
with the integrals
in
M$,
MA
replaced by
-
J:,
and
-
Jtm
respec-
tively, it follows that
M-
is analytic in the lower half k-complex
plane. The eigenfunctions
M+,
M-
are related via the scattering
equations
where the scattering data
S(k,t)
is defined by
S(lc,l>
=
-
J
dtdqq((, q)e-"v-jktM-
22(t,
77,
k).
(6.7)
4?r
R2
Given
S,
the nonlocal
RH
problem (6.6) yields
M-,
then
q
can be
found from
q((,
q)
=
1
/
R2
dkdlS(k, !)eiea+ikfM-
ll(t,%q.
(6.8)
We note that equations (6.7), (6.8) can be thought of as
a
nonlin-
ear Fourier transform. These equations
are
basic for solving both an
initial value problem and an initial-boundary value problem for DSI.
In the case of an initial value problem the evolution of
S(k,l,t)
satis-
fies
iSt
+
(k2
+
12)S
=
0.
However, if the boundary conditions
~1,212

196
A.
S.
Fokas
are nonzero,
S
satisfies
a
more complicated equation:
Let
S([,
q,
t)
be the Fourier transform of
S(k,
1,
t),
then
9
satisfies
i3t+S,,+s7)7)+(7J2(E,t)
+
Ul(7,t))
3
=
0,
Given
q(t,
q,
0)
one first computes
S([,
7,O);
then, since
u1(q,
t),
u2([,t)
are given, equation (6.9) yields
S([,
7,t).
Then the
RH
prob-
lem (6.6) yields
M-,
and (6.8) yields
q([,
q,t).
In order to investigate
the structure
of
the solution
q([,
q,
t),
and in particular the behav-
ior
of
q([,q,t)
as
t
+.
00,
one needs to analyze equation (6.9). We
have achieved this, using the spectral theory of the time-dependent
Schrodinger equation. Indeed, separation of variables suggests that
the analysis
of
(6.9) is intimately related to the analysis
of
i!Pt
+
!Pzs
+
U(Z,t)!P
=
0,
U€R.
(6.10)
In particular the long time behavior
of
S
depends only on the dis-
crete spectrum of (6.10). Suppose that
~1,212
contain
L,
M
discrete
eigenfunctions respectively, corresponding to eigenvalues
Xj,
pj,
i.e.,
~1(q,t)
:
5(777t)7
Xj,
j
=
1,*.*,L
~(t,
t)
:
Xj(t,
t),
(6.11)
Pj,
j
=
1,
*
*
'7
Then
It
is quite interesting that
for
any initial-boundary conditions,
the scattering data become degenerate as
t
---f
00.
Then the
RH
problem (6.6) degenerates to
a
system
of
linear algebraic equations.
The asymptotic value of
q
can be calculated in closed form provided
that
Xj,
Yj
can be found in closed form.
For
example, suppose
u1,
u2

Integrable Nonlinear Equations
197
are reflectionless, then
Xj,
Yj
also satisfy linear algebraic equations:
In
u1(q,
t)
is given by
L
ul(q,
t)
=
-28,
C
tfe-';"+"*t)
qq,
t),
(6.13)
j=1
then the
Y;s
solve the linear algebraic system
Similarly if
uz(6,
t)
is given by
then
In the above equations
t;,
m;,
A;,
pi643
and
XiR,
piR&+.
Using (6.12),
where
Xj,
Yj
are given by (6.14), (6.16) and solving the inverse
problem in closed form we find that
q
is given by,
MJ
i=l,j=l
Q
=
2
X
Xi((,
t)Y3(~7
t)zij(t,
7,
t>*
(6.17)
Z;j
solves
(6.18)
and the matrix
A
is defined by

198
A.
S.
Fokas
A
+
p(I+
CT1
[(I
+
C€)-
1
p
*T
]
,
(6.19)
where superscript
T
denotes the transpose of
a
matrix, and the ma-
trix
p
can be found from initial data
dtdrl%t,
rl,
0)XX
O)Yj*(rl7
0).
(6.20)
We call the above solution an
(M,L)
dromion. In the special case
that
u1,
u2
are time independent, then
Xjz
=
pjz
=
0
and the above
solution degenerates to an
(M,
L)
breather. The solutions obtained
by Boiti et
al
correspond to certain choices
of
p.
The following elegant formulae are valid,
1qI2
=
48&
In
det(1-
&A),
(6.21)
where the matrix
A
is defined by (6.19). Furthermore
E
+
k2
d(dqlqI2
=
4
In
det(1-
~pp+),
(6.22)
where
(p+)ij
=
(p*)ji.
The
(1,l)
dromion
is
given by
Thus if
u1(q,
t)
=
2Xi/cosh2X~(Q-fj) and
u2((,
t)
=
2&/COSh2PR(i-
f)
then
q((,
7,
t)
is
given by (6.23) with
p
uniquely determined from
q((,
Q,
0).
The velocity of
q
in the
(5,~)
directions is given by (-2~1, -2X1),

Integrable Nonlinear Equations
199
therefore it is completely determined by the velocities of u2,ul re-
spectively.
The initial data, i.e.
p,
only affect the motion of the
maximum of
q.
The (2,2) dromion corresponds to
L
=
M
=
2.
As
t
-,
foo,
q(q,
t)
consists of two one-dimensional solitons trav-
eling with speeds -2X11, -2X21. Similarly
u2((,
t)
consists of two
one-dimensional solitons traveling with speeds.
-2~11, -2~21.
By
analyzing the solution
q((,q,t)
as
t
+
foo
we find that
q
con-
sists of 4 localized entities
q;,
i,j
=
1,2 each travelling with velocity
(-2p;1,-2Xj1) (see Fig.
1.1-1.3).
However, in contrast to the one
dimensional case,
q$
#
q;,
i.e. the two-dimensional dromions do
not
retain their form upon interaction (unless pl2
=
p21
=
0).
Thus it
appears that in addition to the exchange of energy between the mean
flow and the surface waves, the localized lumps on the surface can
also exchange energy among themselves. The complete investigation
of the asymptotic behavior of the
(L,M)
dromion is given in [47].
Several other exact solutions of the
DS
equation are also analyzed in
We have also analyzed the ability of the dromions to be driven by
the boundaries. In particular we have considered the case where the
motion of each boundary is given by
a
single soliton, which changes
velocity at
t
=
to.
Let
w;,
wf
and
v;,
vf
be the initial and final speeds
associated with the motion of
u~(q,t)
and uz((,t) respectively. We
have first shown that
as
t
+
00
the dromion follows the motion
of
the boundaries. Furthermore if
E
denotes the energy of the corre-
sponding dromion generated by the this motion of the boundaries
then
1471
-
vw
Ej
-
E;
=
41n
(6.24)
wi
-w
where
V
=
(
4CLR
)
x,
W
=
(e)
x,
and
2X;,2&
are the max-
imum amplitutes of the solitons describing the motion of u1,u2 re-
spectively. Thus if the motion of the boundaries is not uniform the
dromions radiate energy. Similar results can be obtained when the
boundaries move in
a
more complicated way.
