Alligood K., Sauer T., Yorke J.A. Chaos: An Introduction to Dynamical Systems
Подождите немного. Документ загружается.


B IFURCATIONS
Describing saddle-node and period-doubling bifurcations for arbitrary fam-
ilies of two-dimensional maps is complicated by the fact that fixed and periodic
points can have both contracting and expanding directions. Although the pre-
vious example is clearly a special case, the property of having one eigenvalue
with absolute value smaller than 1 at all points (or, analogously, one eigenvalue
with absolute value greater than 1 at all points) is one that holds for a large and
important class of maps. For such maps, the types of bifurcations that can occur
are limited to those seen in one-dimensional maps.
Definition 11.12 Let f be a smooth map of ⺢
2
, and let Df(v)bethe
Jacobian matrix of partial derivatives of f with respect to the v coordinates
evaluated at a point v 僆 ⺢
2
. We say f is area-contracting if | det Df(v)| ⬍ 1 for
all v 僆 ⺢
2
. The map f is area-preserving if | det Df(v)| ⫽ 1 for all v 僆 ⺢
2
.
It is a fact from advanced calculus that the number | det Df(v)| determines
thechangeinareaeffectedbythemapf near the point v.Infact,ifS is a region
in ⺢
2
,then
Area(f(S)) ⫽
S
| det Df(v)|dv.
See (Fitzpatrick, 1995).
Area-contracting maps represent an important class of dynamical systems.
Included in this class are time-T maps of dissipative systems of differential equa-
tions. An example is the time-2
map of the forced, damped pendulum with
equation of motion
¨
x ⫹ c
˙
x ⫹ sin x ⫽ b cos t. We saw in Example 9.5 that with
damping c ⬎ 0, the Jacobian determinant is e
⫺2
c
⬍ 1 for each x and
˙
x.
✎ E XERCISE T11.9
For which values of a and b is the H
´
enon map f(x, y) ⫽ (a ⫺ x
2
⫹ by, x)
area-contracting?
Let p be a periodic orbit of period k. We use the phrase “eigenvalues of p”to
refer to the eigenvalues of D
v
f
k
(p). For maps of the plane, D
v
f
k
(p) has eigenvalues
e
1
and e
2
. The area-contracting hypothesis implies |e
1
e
2
| ⬍ 1. In particular, at
least one of 兵e
1
,e
2
其 must be inside the unit circle in the complex plane. The
unit circle is the set of complex numbers u ⫹ iv with modulus u
2
⫹ v
2
⫽ 1.
Thus the area-contracting hypothesis restricts the types of periodic orbits that
can occur. There are only two types of hyperbolic orbits: attractors or sinks (both
eigenvalues inside the unit circle) and saddles (one eigenvalue greater than ⫹1or
470

11.6 BIFURCATIONS IN P LANE M APS:AREA-PRESERVING C ASE
smaller than ⫺1). In Chapter 12, we need to distinguish between regular saddles
(one eigenvalue greater than ⫹1) and flip saddles (one eigenvalue less than ⫺1).
Notice that the unstable orbits are all saddles, regular or flip. There are no repellers
for area-contracting maps.
Similarly, the types of nonhyperbolic orbits are restricted by the area-
contracting hypothesis. There are only two types: orbits with one eigenvalue equal
to ⫹1, and orbits with one eigenvalue equal to ⫺1. As in the one-dimensional
case, the most frequently seen bifurcations are saddle-nodes and period-doublings.
The difference in the two-dimensional area-contracting case is that what were
paths of repellers in the one-dimensional case are replaced by paths of saddles,
flip or regular, here. We discuss why the saddle-node and period-doubling bifur-
cations are those typically seen for families of one-dimensional maps and families
of area-contracting maps of the plane in Chapter 12.
➮ COMPUTER EXPERIMENT 11.1
Plot the period-6
attracting orbit of the driven double-well Duffing oscil-
lator
¨
x ⫹ 0.1
˙
x ⫺ x ⫹ x
3
⫽
sin t for
⫽ 5.9. Next, increase
and locate the two
period-doubling bifurcations that occur for 5.9 ⬍
⬍ 6.
11.6 BIFURCATIONS IN PLANE MAPS:
A
REA-PRESERVING CASE
In previous sections we saw that bifurcations of fixed points or periodic points can
occur only at parameter values for which the derivative or an eigenvalue of the
orbit has absolute value one. For one-dimensional families, saddle nodes or period-
doubling bifurcations can occur when the derivative is 1 or ⫺1, respectively. For
families of maps of two or more (real) variables, there can be eigenvalues of
absolute value (magnitude) 1 which are not real numbers.
✎ E XERCISE T11.10
Let f be a map of ⺢
n
, and let p be a fixed point of f.
(a) Show that if a ⫹ bi is an eigenvalue of Df(p), then the complex
conjugate a ⫺ bi is also an eigenvalue.
471
B IFURCATIONS
(b) Show that a bifurcation of a period-k orbit from a fixed point can
only occur at a bifurcation orbit that has an eigenvalue that is a kth root of
unity.
In fact, a bifurcation of period-k orbits from a fixed point can only occur
when a primitive kth root of unity is an eigenvalue. In families of one-dimensional
maps this means k ⫽ 1ork ⫽ 2. Bifurcations of higher-period orbits are similarly
ruled out for families of area-contracting maps of the plane. If, however, we
drop the area-contracting hypothesis, then bifurcations of higher-period orbits
(k ⬎ 2) can occur for families of two-dimensional maps. Unless there are special
symmetries or restrictions on the Jacobian (as in the case of area-preserving maps),
such bifurcations are rare compared to saddle nodes or period doublings. As we
discuss in Chapter 12, the latter two bifurcations are “generic”, in the sense that
most one-parameter families possess only these bifurcations.
To investigate how and when bifurcations of higher periods occur, we begin
with a family of two-dimensional linear maps.
E XAMPLE 11.13
Let f
a
(x, y) ⫽ (
a
2
x ⫺
a
3
2
y,
a
3
2
x ⫹
a
2
y), for a ⬎ 0, and x, y in ⺢. When
a ⫽ 1, the map rotates all nonzero vectors through an angle of
3. The origin is
fixed. When the eigenvalues of a fixed point all lie on the unit circle, it is called an
elliptic fixed point. The origin is elliptic for a ⫽ 1. Although an elliptic fixed point
of a linear map is not attracting, it is stable, meaning that for each neighborhood
N
, there is a (perhaps smaller) neighborhood N
␦
such that all orbits beginning
in N
␦
remain in N
. Orbits that start close stay close. For nonlinear maps, elliptic
fixed points may or may not be stable.
It is worthwhile here to think of a “path” e(a) of eigenvalues (parametrized
by a); specifically, e(a) ⫽ a(
1
2
⫹ i
3
2
), which for 0 ⬍ a ⬍ 1 begins inside the unit
circle and crosses the unit circle when a ⫽ 1 at a sixth root of unity. Another
path of eigenvalues consists of the complex conjugate of e(a), for each a ⬎ 0. For
0 ⬍ a ⬍ 1, the origin is a sink; for a ⬎ 1, the origin is a source; and at a ⫽ 1,
the origin is an elliptic point around which all other points rotate on invariant
circles through an angle of
3. In the last case, all other points are period-six
points.
For a one-parameter family f
a
of nonlinear planar maps, we know that the
eigenvalues of Df
a
are the key to detecting changes of stability that might result
in bifurcations. To locate bifurcations of period-k orbits along a path of fixed
472

11.6 BIFURCATIONS IN P LANE M APS:AREA-PRESERVING C ASE
points, we look for points at which a path e(a) of eigenvalues crosses the unit
circle at a kth root of unity. If the path crosses the unit circle with nonzero speed
(if the derivative de da is nonzero), then a family of invariant closed curves will
bifurcate from the path of fixed points. The family of closed curves may all occur
at one parameter value, such as the invariant circles in the previous linear case, or
they may occur in a path that emanates from the bifurcation parameter through
either smaller or larger parameter values. We do not discuss the dynamics that
occur on the invariant closed curves except to say that near the bifurcation point,
on each closed curve, points rotate near the rate given by the Jacobian at the
bifurcation orbit.
In Example 11.14, we investigate a bifurcation in the H
´
enon family which
produces a family of invariant closed curves as we pass from area-contracting to
area-expanding maps.
E XAMPLE 11.14
Let h
b
(x, y) ⫽ (⫺.75 ⫺ x
2
⫹ by, x). Recall that ⫺b is the Jacobian deter-
minant: h is area-contracting or area-expanding according to whether |b| is less
than or greater than 1. For b ⫽⫺1, there is a fixed point at (⫺1 2, ⫺1 2) with
eigenvalues e ⫽
1
2
⫾
3
2
i, (two sixth-roots of unity). Figure 11.16 shows a neigh-
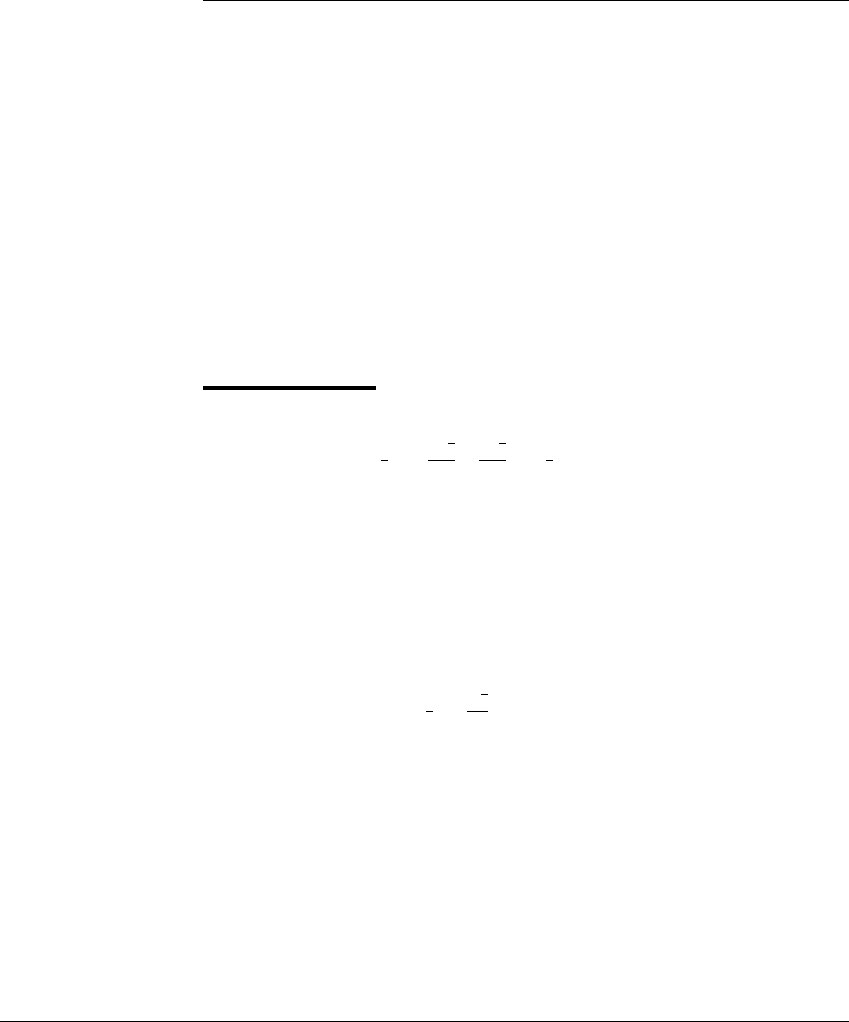
borhood of this fixed point (or its continuation) as b varies from ⫺.9to⫺1.1.
The fixed point goes from sink (Figure 11.16(a)) to source (Figure 11.16(b)). In
particular, Figure 11.16(c) shows invariant curves encircling the fixed point at
b ⫽⫺1. Notice that the crossing stable and unstable manifolds of a nearby fixed
point saddle form the boundary of the “elliptic” behavior at the original fixed
point. There are no invariant closed curves for |b| ⫽ 1. (See Exercise T11.11
below.)
✎ E XERCISE T11.11
Show that an invertible map of ⺢
2
which is either area-contracting or
area-expanding can have no invariant closed curves (except points).
Families of area-preserving planar maps (see Defn. 11.12) can possess an
extremely rich bifurcation structure. We still see periodic saddle orbits in these
maps, but no sinks or sources. The stable orbits must be elliptic: Both eigenvalues
are on the unit circle.
473
B IFURCATIONS
(a) (b)
(c)
Figure 11.16 The continuation of two fixed points in the H
´
enon family.
Various orbits of the map h
b
(x, y) ⫽ (⫺.75 ⫺ x
2
⫹ by, x) are shown for (x, y)in
[⫺2.5, 5.0] ⫻ [⫺2.5, 5.0]. (a) At b ⫽⫺0.9, the map is area-contracting and there
is an attracting fixed point. The stable and unstable manifolds of a nearby saddle
fixed point are depicted. One branch of the unstable manifold spirals into the
attractor. (b) At b ⫽⫺1.1, the map is area-expanding and there is a repelling fixed
point (the continuation of the elliptic point at this parameter). Now the stable
manifold of the saddle is shown to spiral out of the repeller. (c) At the in-between
value b ⫽⫺1.0, the map is area-preserving and there is an elliptic fixed point (the
continuation of the attractor at this parameter). The stable and unstable manifolds
of the saddle now cross in infinitely many homoclinic points.
474

11.6 BIFURCATIONS IN P LANE M APS:AREA-PRESERVING C ASE
✎ E XERCISE T11.12
Show that there are no hyperbolic periodic or fixed sinks or sources for
area-preserving maps of the plane.
We investigate the H
´
enon family again—this time fixing b at ⫺1and
varying a. Each map in the resulting family is an area-preserving map.
E XAMPLE 11.15
Let h
a
(x, y) ⫽ (a ⫺ x
2
⫺ y, x). Verify that for each a, the plane map h
a
is
area-preserving. For a ⬍⫺1 there are no fixed points. When a ⫽⫺1, a fixed point
appears at (⫺1, ⫺1). This orbit has a double eigenvalue of ⫹1. For a ⬎⫺1, there
are two fixed points: one that is unstable (a saddle) and one that begins as a stable
elliptic orbit for a near ⫺1. We follow the orbits along the second, stable path.
As a increases slightly beyond ⫺1, eigenvalues of these orbits are constrained
to the unit circle in the complex plane. Since for any fixed parameter the two
eigenvalues must be complex conjugates, the two paths of eigenvalues move (with
increasing a) around the unit circle. One moves along the top (imaginary part
positive), and the other moves along the bottom (imaginary part negative) as
shown in Figure 11.17. When a ⫽ 3, the two paths of eigenvalues join at ⫺1,
where there is a period-doubling bifurcation. What type of behavior do you expect
near the elliptic fixed point as a increases from ⫺1to3?
Our final example of this section is called the standard map. Like the
H
´
enon family, it has been studied extensively as a prototype—in this case,
as a model for understanding nonlinear phenomena in area-preserving maps.
While Poincar
´
e maps for dissipative systems of differential equations are area-
contracting, Poincar
´
e maps for conservative systems are area-preserving.
E XAMPLE 11.16
(Standard map.) Let
S
a
(x, y) ⫽ (x ⫹ y(mod2
),y⫹ a sin(x ⫹ y)(mod2
)), (11.7)
for x 僆 [⫺
,
], y 僆 [⫺
,
]. Notice that the standard map S
a
is periodic with
period 2
in both variables. That is, S
a
(x ⫹ 2
,y) ⫽ S
a
(x, y)andS
a
(x, y ⫹ 2
) ⫽
S
a
(x, y). Therefore, like the cat map of Challenge 2, only the values of x and y
modulo 2
are important, and we can consider the phase space to be a torus.
475
B IFURCATIONS
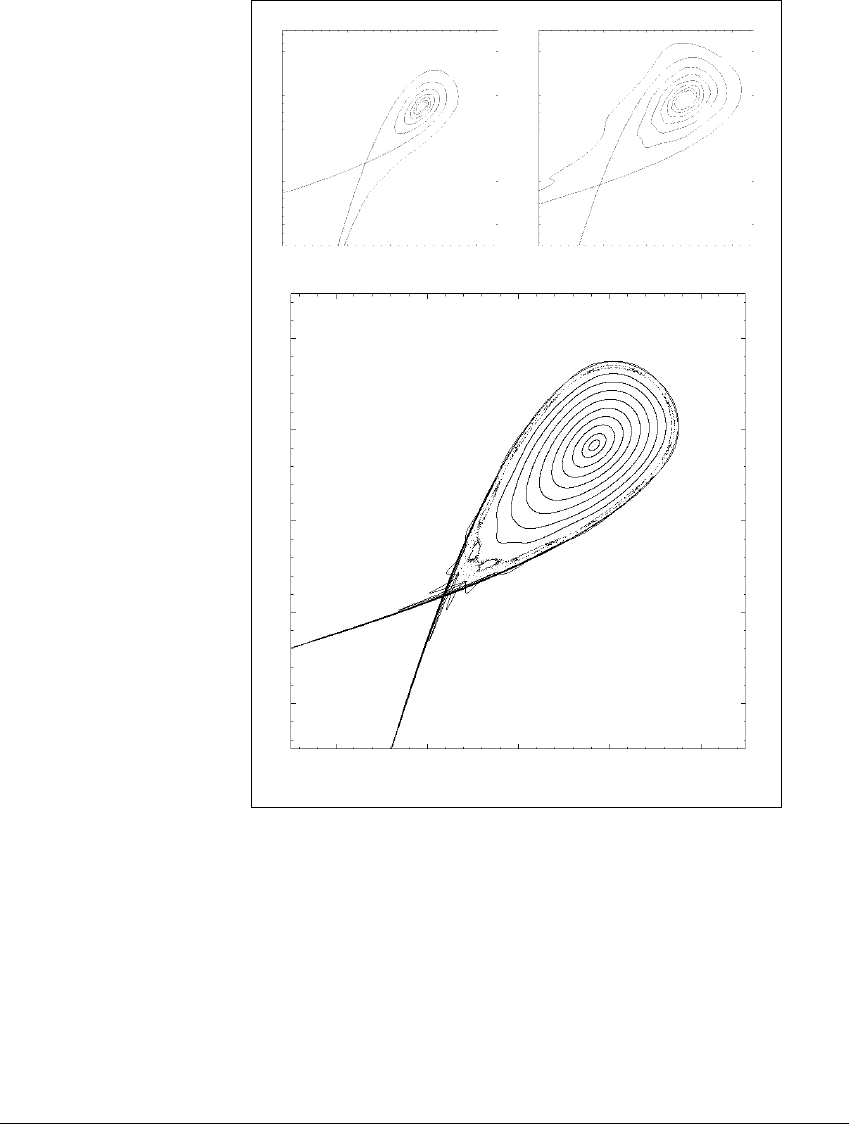
e
1
e
2
a = 3
a = -1
Figure 11.17 Complex eigenvalues for a family of elliptic fixed points.
Paths of eigenvalues in the complex plane for the continuation of an elliptic fixed
point of the area-preserving H
´
enon family h(a, x, y) ⫽ (a ⫺ x
2
⫺ y, x) are depicted
schematically. The elliptic point is born at a ⫽⫺1, at which point both eigenvalues
are ⫹1. Then the paths split and move around the unit circle as complex conjugates.
The paths join at a period-doubling bifurcation when a ⫽ 3. There the fixed point
loses stability, becoming a saddle, as one path of eigenvalues moves outside the unit
circle.
✎ E XERCISE T11.13
Let S
a
denote the standard map. Show that det(DS
a
(x, y)) ⫽ 1. Therefore,
S
a
is a one-parameter family of area-preserving maps.
When the parameter a is zero, the torus is filled with invariant closed curves
(one for each y value). In Figure 11.18, both axes are [⫺
,
], so that one can
imagine the right and left boundaries to be glued together, and the same for the
top and bottom boundaries, to form the torus. The center of the square is the
origin, which is a fixed point for the standard map.
The closed curves in Figure 11.18(a) are called KAM curves. KAM curves
figure importantly in understanding stability within area-preserving maps. This
stability analysis is the subject of KAM theory, a field whose major advances are
due to the three mathematicians A. Kolmogorov, V. Arnold, and J. Moser. On
each of these curves, all points move with the same rate of rotation, called the
rotation number. The rotation number of a circle increases with y.
As the parameter a is increased from 0, other closed curves form that do
not wrap all the way around the torus. These KAM curves appear as oval-shaped
476
11.6 BIFURCATIONS IN P LANE M APS:AREA-PRESERVING C ASE
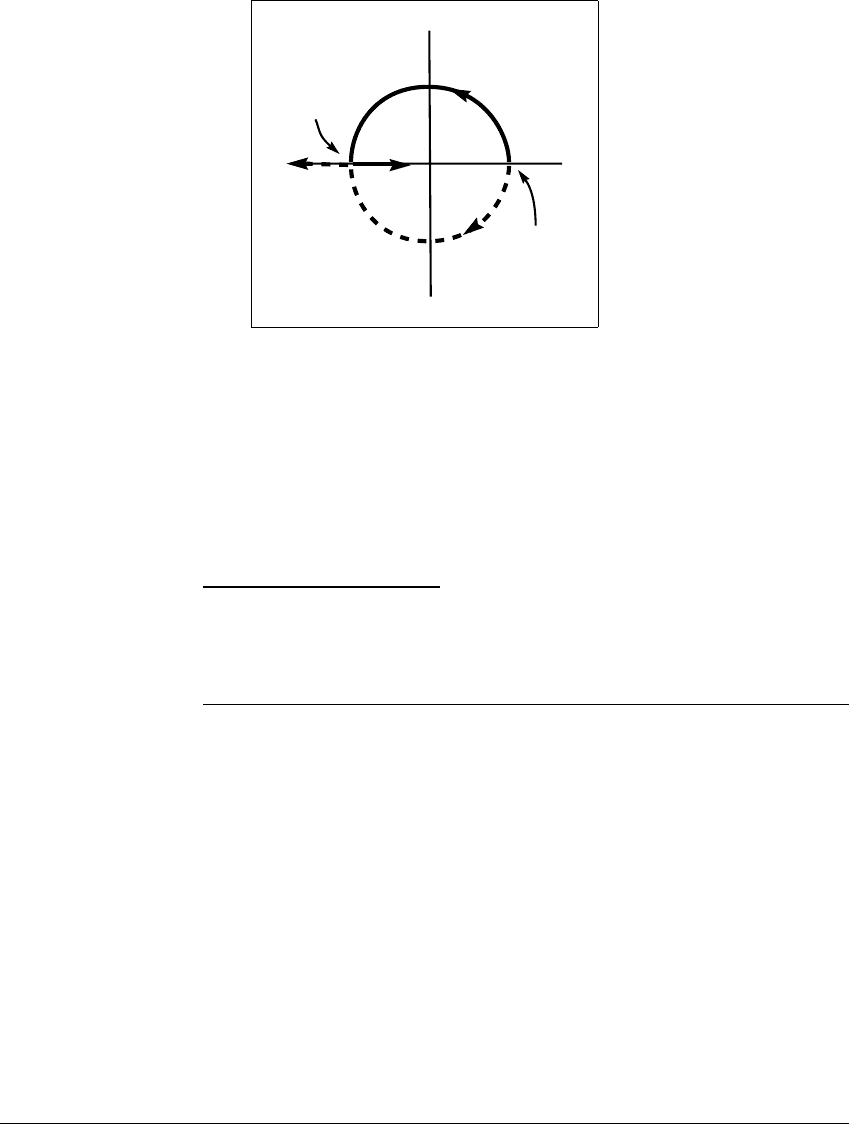
(a) (b)
(c) (d)
(e) (f)
Figure 11.18 Standard maps at selected parameters.
Typical orbits of the standard family of maps S
a
(x, y) ⫽ (x ⫹ y mod 2
, y ⫹ a sin(x ⫹
y)mod2
) are shown for (x, y)in[⫺
,
] ⫻ [⫺
,
] and parameter values (a) a ⫽
0(b)a ⫽ 0.3(c)a ⫽ 0.6(d)a ⫽ 0.9(e)a ⫽ 1.2and(f)a ⫽ 7.0. In all but (f),
several orbits are plotted. In (f), one orbit is shown.
477

B IFURCATIONS
islands in Figure 11.18(b)–(e). Points on the closed curves are constrained to
map to the same closed curve. Each island in Figure 11.18(b)–(e) was made by
plotting a single orbit, which fills the curve densely. The KAM curves eventually
give way to irregular orbits. The last KAM curve that wraps around the torus can
be seen in the lower half of Figure 11.18(d). It disappears at a ⬇ 0.97.
✎ E XERCISE T11.14
Let S
a
denote the standard map.
(a) Show that for a ⬎ 0, the origin (0, 0) is a saddle fixed point.
(b) For what values of a is (
, 0) an elliptic fixed point?
As a increases from zero, the invariant circles near y ⫽ 0 break up. We
see the stable and unstable manifolds of the saddle (0, 0) forming the boundary
between the closed curves surrounding the elliptic point and the closed curves
encircling the cylinder. A further increase in a shows regions of chaotic behavior
developing around these stable and unstable manifolds. New elliptic orbits come
into existence and disappear as chaotic regions expand to fill an increasingly
larger space. Figure 11.18(f), at a ⫽ 7.0, shows no regions of stability. Only one
orbit is plotted.
➮ COMPUTER EXPERIMENT 11.2
The standard map can be made area-contracting by altering one term.
Define
S
a,b
(x, y) ⫽ (x ⫹ y(mod2
),by⫹ a sin(x ⫹ y)(mod2
)). (11.8)
Investigate S
a,b
for a ⫽ 4.0andb ⫽ 0.98. How many attracting periodic orbits
can you find? (More than 100 exist for periods ⱕ 5.)
11.7 BIFURCATIONS IN DIFFERENTIAL
EQUATIONS
The main tool for analyzing the stability of periodic solutions of differential
equations is the Poincar
´
e map, which we first defined in Chapter 2. Let v be a
point in ⺢
n
, let f be a map defined on ⺢
n
, and let
␥
be a periodic orbit of the
478
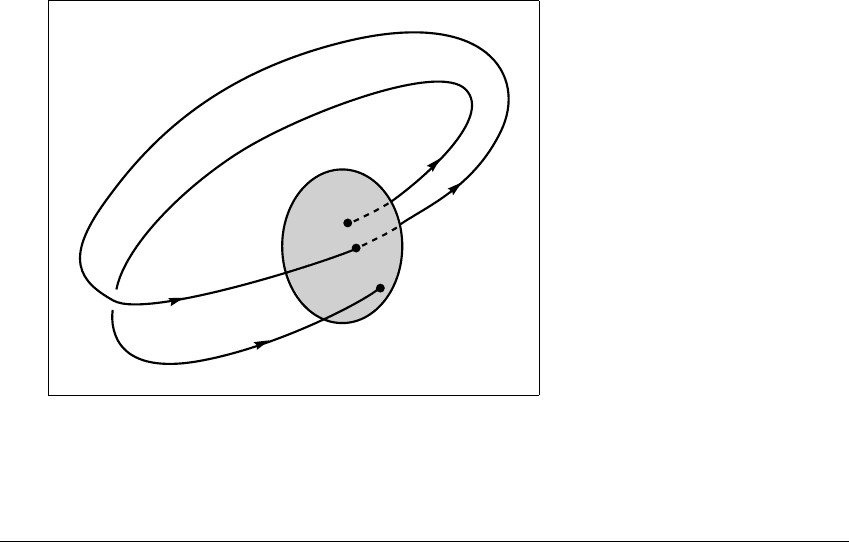
11.7 BIFURCATIONS IN DIFFERENTIAL E QUATIONS
autonomous differential equation
˙v ⫽ f(v). (11.9)
For a point v
0
on
␥
, the Poincar
´
emapT is defined on an n ⫺ 1 dimensional
disk D transverse to
␥
at v
0
. Figure 11.19 shows a periodic orbit for a flow of
⺢
3
with a two-dimensional disk D. If v
1
is a point sufficiently close to v
0
on D,
then the solution through v
1
will follow close to
␥
until it intersects D again (the
first return to D) at a point v
2
. This fact follows from continuous dependence
on initial conditions, Theorem 7.16. Let T(v
1
) ⫽ v
2
. (We may have to restrict
the domain of T to a smaller disk centered at v
0
in order to have the solution
hit D upon first return.) For a periodic orbit of a planar differential equation, the
cross-sectional disk is a line segment transverse to the periodic orbit.
Notice that v
0
is a fixed point or periodic point of T if and only if v
0
is on
a periodic orbit of (11.9). Thus we can investigate the stability of periodic orbits
through a linear analysis of T, by looking at the eigenvalues of D
v
T(v
0
).
Definition 11.17 The eigenvalues of the (n ⫺ 1) ⫻ (n ⫺ 1) Jacobian
matrix D
v
T(v
0
) are called the (Floquet) multipliers of the periodic orbit
␥
.
D
v
2
= T(v
1
)
v
0
v
1
Figure 11.19 A Poincar
´
emap.
The Poincar
´
e map, called T here, is defined on a surface D which is transverse to
the flow direction on a periodic orbit. The point v
0
on the periodic orbit maps to
itself under the Poincar
´
e map, while v
1
maps to v
2
.
479
