Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

340

Table 3

is

the

data

on unaided distance vision of 7477 women aged

30-39 employed in Royal

Ordnance

factories in

Britain

from 1943

to

1946.

The

row variable is

the

right eye

grad

e

and

the

column variable is

the

left eye grade

with

the

categories ordered from

the

Best (1)

to

the

Worst

( 4).

The

vision

data

in Table 3 have

been

analyzed by

many

statisti-

cians, including

Stuart

(1955), Bishop

et

al. (1975, p. 284), McCullagh

(1978),

Goodman

(1979), Agresti (1983), Tomizawa (1985, 1993, 2009),

Miyamoto,

Ohtsuka

and

Tomizawa (2004), Tomizawa, Miyamoto

and

Ya-

mamoto

(2006), Tomizawa

and

Tahata

(2007),

and

Tahata,

Yamamoto,

Nagatani

and

Tomizawa (2009).

Table 4

is

the

data

on unaided distance vision of 3168 pupils comprising

nearly equal

number

of boys

and

girls aged 6-12

at

elementary schools in

Tokyo,

Japan,

examined in

June

1984.

The

data

in Table 4 have also been

analyzed by Tomizawa (1985), Miyamoto

et

al. (2004),

and

Tahata

and

Tomizawa (2006).

Table 5 is

the

data

on unaided distance vision of 4746

students

aged 18

to

about

25

including

about

10 percent women in Faculty of Science

and

Technology, Science University of Tokyo in

Japan

examined in April 1982.

The

data

in Table 5 have been analyzed by Tomizawa (1984, 1985)

and

Tahata

et

al. (2009).

The

data

in Table 6 represent

the

cross-classification of a sample of

individuals according

to

their

socioprofessional category in 1954

and

in

1962 (see Caussinus, 1965; Bishop

et

al., 1975, p. 298).

Tables 1

through

6 are

the

data

of square contingency tables having

the

same row

and

column classifications.

In

addition,

the

categories in each

of Tables 1

through

6 are ordered.

Many

observations concentrate on (or

near)

the

main

diagonal cells in

the

table. Therefore

the

row classification

tends

to

be

strongly associated

with

the

column classification, namely,

the

model of independence (i.e., null association) between

the

row

and

column

classifications does

not

hold. For those

data

we are interested in

whether

or

not

the

row value of

an

individual is symmetric

to

the

column value.

Many

models of

symmetry

and

asymmetry

have been proposed

by

many

statisticians; for instance, Bowker (1948), Caussinus (1965), Bishop

et

al.

(1975,

Chap. 8), McCullagh (1978),

Goodman

(1979), Agresti (1983, 2002),

Tomizawa (1993, 2009),

and

Tomizawa

and

Tahata

(2007). We omit here

the

details of models of

symmetry

or

asymmetry.

For

the

data

in Tables 1

through

6

we

are also interested

in

measuring

the

relative improvement in variation in predicting

the

value of

the

other

variable when

the

value of one variable is known, opposed

to

when

it

is

not

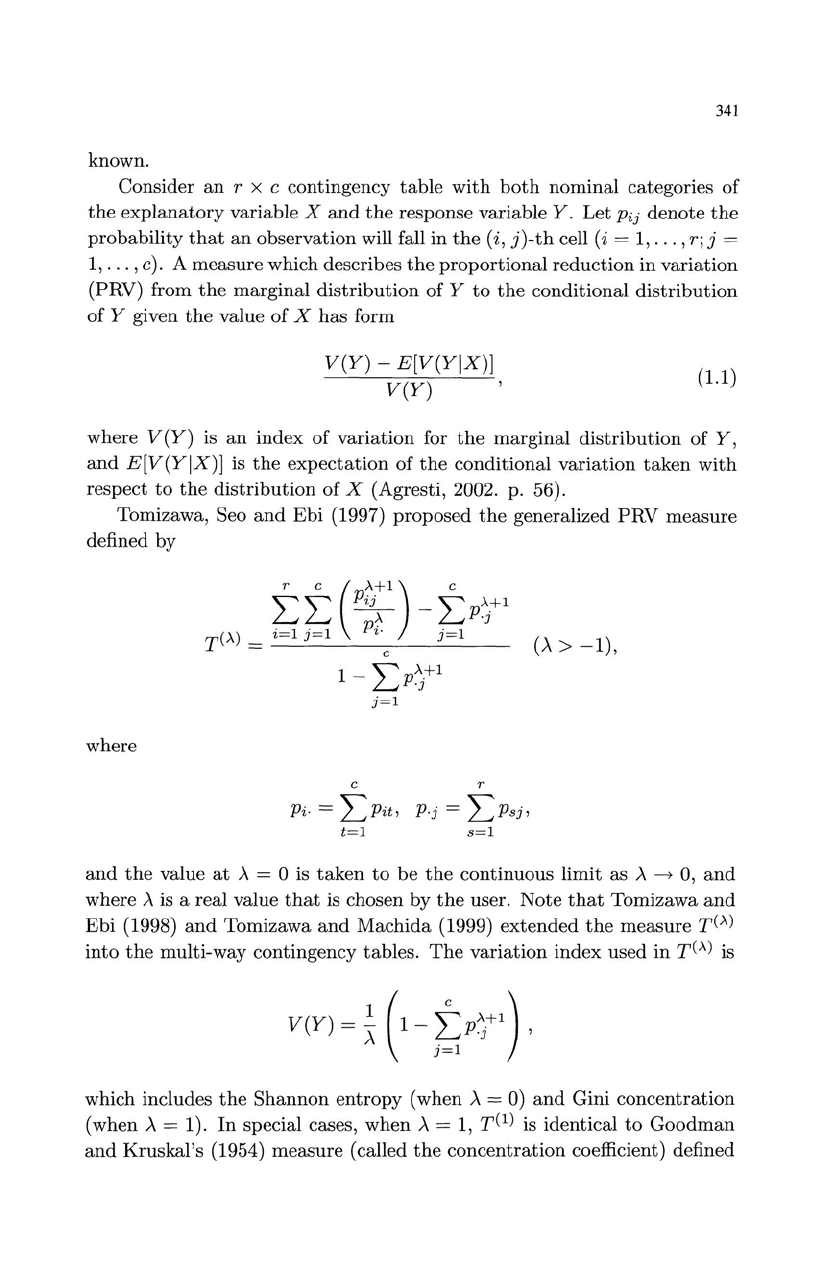
341

known.

Consider

an

r x c

contingency

table

with

both

nominal

categories

of

the

explanatory

variable

X

and

the

response

variable

Y.

Let

Pij

denote

the

probability

that

an

observation

will fall

in

the

(i,

j)-th

cell (i = 1,

...

,rj

j =

1,

...

,c). A

measure

which

describes

the

proportional

reduction

in

variation

(PRV)

from

the

marginal

distribution

of

Y

to

the

conditional

distribution

of

Y given

the

value

of

X

has

form

V(Y)

-

E[V(YIX)]

V(Y)

(1.1 )

where

V(Y)

is

an

index

of

variation

for

the

marginal

distribution

of

Y,

and

E[V(YIX)]

is

the

expectation

of

the

conditional

variation

taken

with

respect

to

the

distribution

of

X (Agresti, 2002. p. 56).

Tomizawa, Seo

and

Ebi

(1997)

proposed

the

generalized

PRY

measure

defined

by

(A>

-1),

where

c r

Pi·

=

LPit,

p.j =

LPSj,

t=l

s=l

and

the

value

at

A = 0 is

taken

to

be

the

continuous

limit

as A

-7

0,

and

where

A is a

real

value

that

is chosen

by

the

user.

Note

that

Tomizawa

and

Ebi

(1998)

and

Tomizawa

and

Machida

(1999)

extended

the

measure

T(A)

into

the

multi-way

contingency

tables.

The

variation

index

used

in

T(A)

is

V(Y)

=

~

(1

-

tp~+l)

,

J=l

which

includes

the

Shannon

entropy

(when

A = 0)

and

Gini

concentration

(when

A = 1).

In

special cases,

when

A = 1,

T(l)

is

identical

to

Goodman

and

Kruskal's

(1954)

measure

(called

the

concentration

coefficient) defined

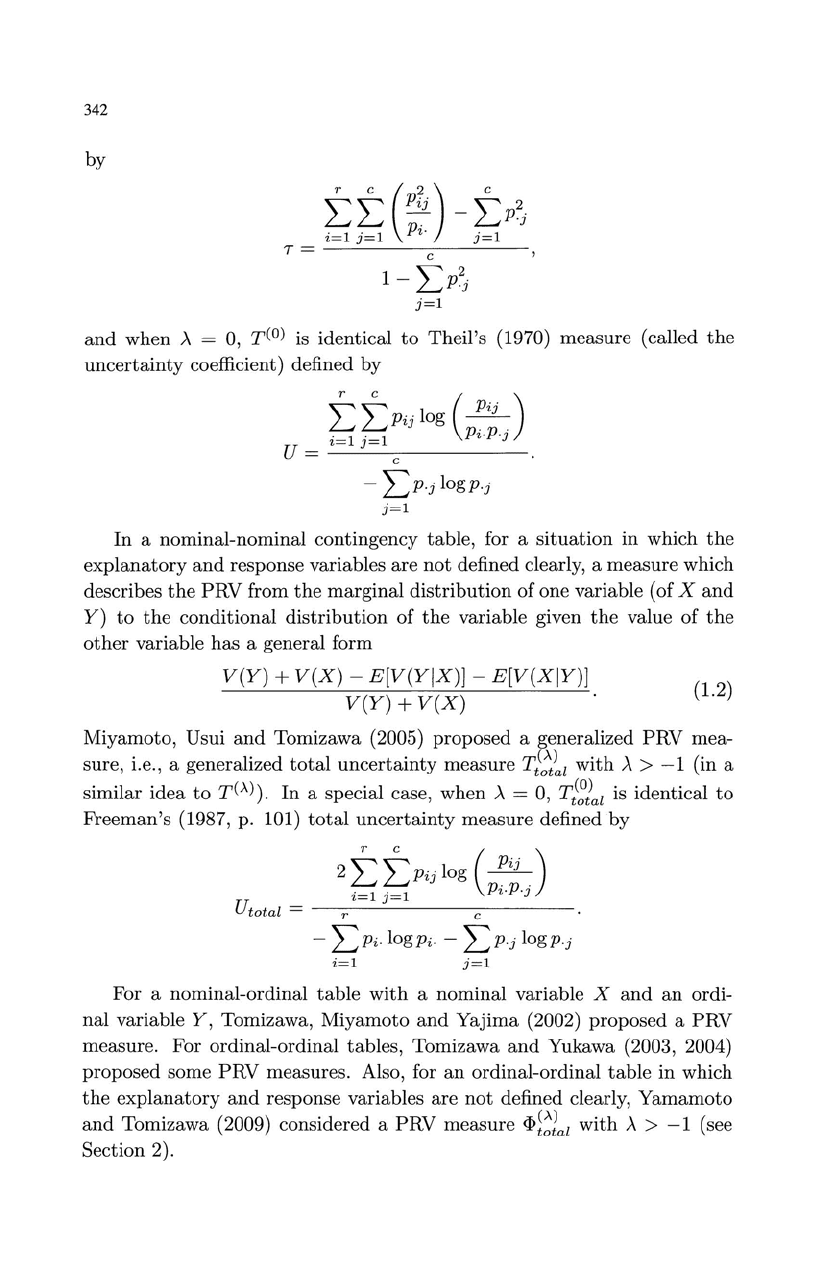
342

by

T=

and

when)..

=

0,

T(O)

is identical

to

Theil's

(1970)

measure

(called

the

uncertainty

coefficient) defined

by

t

tpij

log (

Pi

j

)

i=l

j=l

p"'P'J

U =

---'----c-----

-

LP.j

logp.j

j=l

In

a nominal-nominal contingency

table,

for a

situation

in

which

the

explanatory

and

response variables

are

not

defined clearly, a

measure

which

describes

the

PRY

from

the

marginal

distribution

of

one

variable (of X

and

Y)

to

the

conditional

distribution

of

the

variable given

the

value

of

the

other

variable

has

a general form

V(Y)

+

V(X)

-

E[V(YIX)]-

E[V(X/y)]

V(Y)

+

V(X)

(1.2)

Miyamoto, Usui

and

Tomizawa (2005)

proposed

a generalized

PRY

mea-

sure, i.e., a generalized

total

uncertainty

measure

Tt~~l

with)"

>

-1

(in a

similar

idea

to

TP.·»).

In

a special case,

when)..

= 0,

Tt~Ll

is identical

to

Freeman's

(1987, p. 101)

total

uncertainty

measure

defined

by

2 t t Pij log (

Pi

j

)

i=l

j=l p",p']

Utotal

=

~-rCC-------c-----

-

LPi.logpi.

-

LP.j

logp.j

i=l

j=l

For a nominal-ordinal

table with

a

nominal

variable X

and

an

ordi-

nal

variable Y, Tomizawa,

Miyamoto

and

Yajima

(2002)

proposed

a

PRY

measure.

For

ordinal-ordinal tables, Tomizawa

and

Yukawa (2003, 2004)

proposed

some

PRY

measures. Also, for

an

ordinal-ordinal

table

in

which

the

explanatory

and

response variables

are

not

defined clearly,

Yamamoto

and

Tomizawa (2009) considered a

PRY

measure

~~lal

with

)..

>

-1

(see

Section 2).

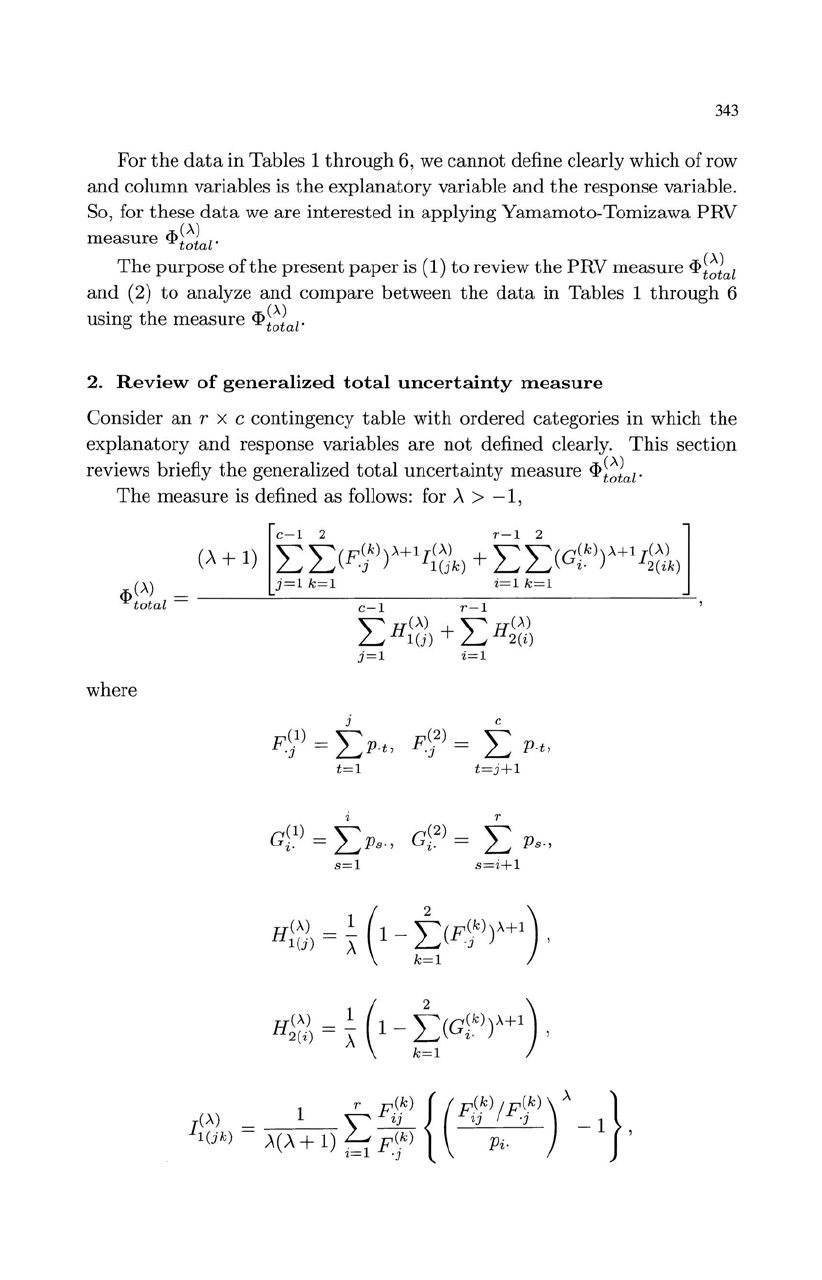
343

For

the

data

in Tables 1

through

6,

we

cannot

define clearly which of row

and

column variables is

the

explanatory

variable

and

the

response variable.

So, for these

data

we are interested in applying Yamamoto-Tomizawa PRY

(A)

measure

I]>

total'

The

purpose of

the

present

paper

is (1)

to

review

the

PRY measure

I]>~~L

and

(2)

to

analyze

and

compare between

the

data

in Tables 1

through

6

. h

,T..(A)

usmg t e measure

'l'total'

2.

Review

of

generalized

total

uncertainty

measure

Consider

an

r x c contingency

table

with

ordered categories in which

the

explanatory

and

response variables are not defined clearly.

This

section

reviews briefly

the

generalized

total

uncertainty

measure

I]>

~~L.

The

measure is defined as follows: for A >

-1,

~

~'J

l(Jk)

~

2'

2(2k)

(

A

+

1)

[~~(F(k))A+1

J(A)

+

~

~(dk))A+1

J(A)

1

I]>(A) _

j=l

k= l i= l k= l

total

-

c-1

r - 1 '

where

L

H~~])

+ L

H~~)

j=l

i=l

j c

F.~1)

=

LP.t,

F.j2)

= L p·t,

t= l t=j + 1

i r

C

(l)

'"

C(2)

=

'"

i·

=

~Ps.,

2'

~

Ps·

,

s=l

s=i+1

H(A)

=

~

(1

_

~(F(k))A

+

1)

l(J)

A

~'J

'

k=l

H(A)

=

~

(1

_

~(dk))A+1)

2(2) A

~

2'

,

k=l

1

r

F(k)

F(k)jF(k)

(A) =

~

2J

'J

_ 1

{

A}

J'Uk)

'\(H

1)

~

Flk) (

p,)

,

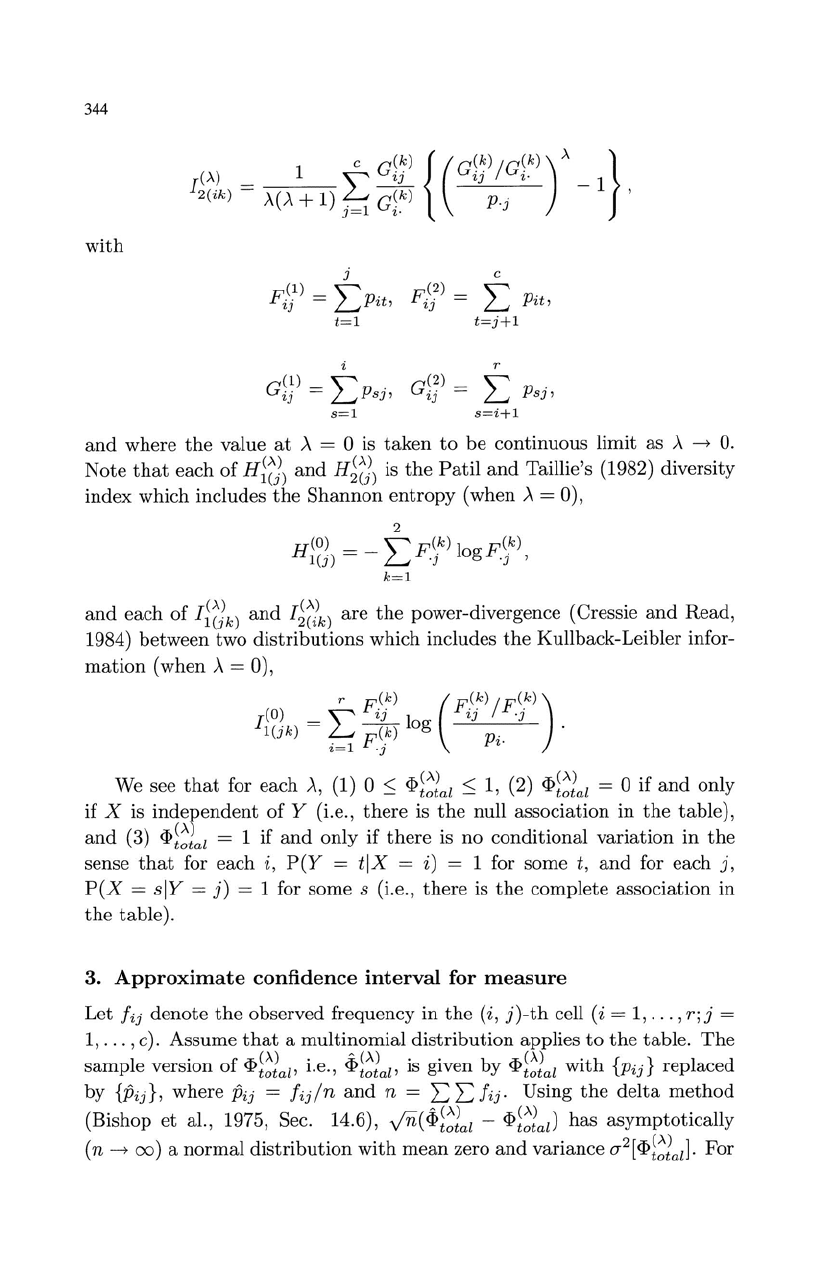
344

with

j

c

FS)

=

LPit,

F(2)

=

2J

L

Pit,

t=1

t=j+l

i

r

aU)

=

LPsj,

0<2)

=

'J

L

Psj,

s=1

s=i+l

and

where

the

value

at

A = 0 is

taken

to

be

continuous

limit

as

A

---->

O.

Note

that

each of

Hi~])

and

H~~])

is

the

Patil

and

Taillie's (1982)

diversity

index

which includes

the

Shannon

entropy

(when A = 0),

2

Hi~J)

= - L

F.~k)

log

F.~k),

k=1

and

each

of

Ji~]k)

and

J~~L)

are

the

power-divergence (Cressie

and

Read,

1984)

between

two

distributions

which includes

the

Kullback-Leibler infor-

mation

(when

A = 0),

r F(k)

(F(k)/F(k))

J

(O)

'"

'J

1

'J

.J

l(jk)

=

~

-----u0

og

. .

i=1

F.

j

p,.

We see

that

for

each

A,

(1) 0 S

~~L

s

1,

(2)

~~L

= 0 if

and

only

if

X is

independent

of

Y (i.e.,

there

is

the

null

association

in

the

table),

and

(3)

~~L

= 1

if

and

only

if

there

is

no

conditional

variation

in

the

sense

that

for

each

i,

P(Y

=

tlX

= i) = 1 for some t,

and

for

each

j,

P(X

=

slY

=

j)

= 1 for

some

s (i.e.,

there

is

the

complete

association

in

the

table).

3.

Approximate

confidence

interval

for

measure

Let

Iij

denote

the

observed

frequency in

the

(i,

j)-th

cell (i = 1,

...

,

r;

j =

1,

...

,c).

Assume

that

a

multinomial

distribution

applies

to

the

table.

The

1

. f

",(A)

.

.i.(A)

. . b

",(A)

. h { } 1 d

samp

e verSlOn 0

'¥

total'

1.e.,

'¥

total>

IS

gIven y

'¥

total

WIt

Pij

rep

ace

by

{Pij},

where

Pij

=

Iij

/

nand

n = L L

Iij.

Using

the

delta

method

(Bishop

et

al., 1975, Sec. 14.6),

Vn(~~LI

-

~~L)

has

asymptotically

(n

---->

(0) a

normal

distribution

with

mean

zero

and

variance

(72

[

~~iatl.

For

345

the

detail of

(72[~~L],

see Yamamoto

and

Tomizawa (2009). Therefore

we

can

obtain

an

approximate

confidence interval for

~~ial

using

the

estimated

approximate

standard

error

o-[~~Ll/Vn

for

~~~ial'

where

o-2[~~iall

denote

(72

[

~~all

with

{Pij} replaced by {Pij}.

4.

Analysis

of

data

We shall analyze

the

data

in Tables 1

through

6 using

the

total

uncertainty

measure

~~ial'

Table 7 gives

the

value of

estimated

measure

~~~L

and

the

approximate

95% confidence interval for

the

measure

~~L

applied

to

the

data

in Tables 1

through

6.

We see from Table 7

that

the

confidence interval for

~~L

applied

to

the

data

in Table 1 includes zero for all

A.

Therefore

this

would indicate

that

there

is

a

structure

of independence, i.e., null association, between

the

daily

temperature

at

Nagasaki City in 2001

and

in 2002. Namely, when we

know

the

temperature

of a

day

in 2001 (in 2002),

the

knowledge would not

be useful for predicting

the

temperature

of

the

day

in 2002 (in 2001).

We also see from Table 7

that

for

the

data

of Merino ewes in Table 2

the

values of

estimated

measure

~~~Ll

are close

to

zero, however,

the

confidence

intervals for

~~ial

do not include zero. Therefore these would indicate

that

the

number

of lambs

born

in 1953 may

be

somewhat associated

with

the

number

of lambs

born

in 1952. Namely, when

we

know

the

number

of

lambs

born

in 1952 (in 1953),

the

knowledge

may

be

somewhat useful for

predicting

the

number

of lambs

born

in 1953 (in 1952).

Moreover we see from Table 7

that

for

three

kinds of vision

data

in

Tables

3,

4

and

5,

the

values of

estimated

measure

~~~L

are

greater

than

zero

and

the

confidence intervals for

~~ial

do not include zero. Therefore

for each of vision

data

in Tables

3,

4

and

5,

the

right eye

grade

for

an

individual is strongly associated

with

the

left eye grade for

the

individual.

Namely, when

we

know

the

grade of one eye (of right eye

and

left eye) for

an

individual,

the

knowledge would

be

useful for predicting

the

grade of

the

other

eye for

the

individual.

In

addition, we see from Table 7

that

(1)

the

value of

estimated

measure

~~~L

and

the

values in confidence interval for

~~ial

applied

to

the

vision

data

of pupils in Table 4 are

greater

than

the

corresponding values of

them

applied

to

the

vision

data

of women in Table 3,

and

(2)

the

values of

them

applied

to

the

vision

data

of

students

in Table 5 are

greater

than

the

corresponding values of

them

applied

to

the

vision

data

of pupils in Table

346

4.

Thus,

when

we

want

to

predict

the

grade

of

one

eye for

an

individual

by

obtaining

the

knowledge

of

the

grade

of

the

other

eye for

the

individual,

(1)

the

knowledge would

be

useful for

the

data

of

pupils

in

Table

4

rather

than

for

the

data

of

women

in

Table 3,

and

also (2)

the

knowledge would

be

useful for

the

data

of

students

in Table 5

rather

than

for

the

data

of

pupils

in

Table 4.

We see from Table 7

that

for example,

when

A = 1,

the

value of esti-

mated

measure

<l>~!Ll

is 0.650 for

the

data

of

students

in

Table

5.

Thus,

when

we

predict

the

grade

of one eye for a

student

by

obtaining

the

knowl-

edge

of

the

grade

of

the

other

eye for

the

student,

the

prediction

becomes

65%

better

when

we know

the

information

than

when

we

do

not

know

the

information. Similarly, for

the

vision

data

of

pupils

in

Table 4,

the

predic-

tion

becomes 53%

better

when

we know

the

information

than

when

we

do

not

know

the

information. Also, for

the

vision

data

of women

in

Table

3,

the

prediction

becomes 44%

better.

We see

further

from Table 7

that

for

the

data

of

socioprofessional

status

in Table 6,

the

value

of

estimated

measure

<l>~~lal

are

greater

than

zero

and

the

confidence intervals for

~~L

do

not

include zero.

In

addition,

the

value

of

<l>~~L

applied

to

the

data

in

Table 6 is

greater

than

any

other

value

of

<l>~~ial

applied

to

the

data

in

Tables 1

through

5.

Therefore, for

the

data

of

socioprofessional

status

in Table 6,

the

socioprofessional

status

in

1962 for

an

individual is

strongly

associated

with

the

status

in

1954 for

the

individual. Namely,

when

we

know

the

socioprofessional

status

in

1954 (in

1962) for

an

individual,

the

knowledge would

be

useful for

predicting

the

status

in 1962 (in 1954) for

the

individual. We see from Table 7

that

for

example,

when

A = 1,

the

value

of

estimated

measure

<l>~!~al

is 0.699 for

the

data

of

socioprofessional

status

in

Table

6.

Thus,

we

want

to

predict

the

socioprofessional

status

in

one

year

of

1954

and

1962 for

an

individual

by

obtaining

the

knowledge

of

the

status

in

the

other

year

for

the

individual,

the

prediction

becomes

about

70%

better

when

we know

the

information

than

when

we

do

not

know

the

information.

5.

Remarks

For a two-way contingency

table

with

the

explanatory

variable X

and

the

response variable

Y,

the

PRY

measure

of

form (1.1) including, e.g.,

TCA),

T

and

U, would

be

useful for seeing

what

degree

the

relative improvement

in

variation

for

predicting

the

value

of

response variable Y

when

we know

the

value

of

explanatory

variable X is

toward

the

perfect

prediction

(i.e.,

347

when

the

measure equals 1).

For a two-way contingency

table

in

which

the

explanatory

and

response

variables

are

not

defined clearly,

the

PRY

measure

of

form (1.2) including,

e.g.,

Tt~~~l'

Utotal

and

~~ial'

would

be

useful for seeing

what

degree

the

rel-

ative improvement in variation for

predicting

the

value

of

one variable when

we know

the

value

of

the

other

variable is toward

the

perfect prediction.

Yamamoto

and

Tomizawa (2009) applied

the

total

uncertainty

measure

~~ial

to

the

data

of cross-classification of

father's

and

his son's occupa-

tional

status

in

Denmark,

in

British

and

in

Japan

(though

the

details are

omitted

here).

When

we

want

to

predict

the

son's

occupational

status

for

a

pair

of

father

and

his son by

obtaining

the

knowledge

of

his

father's

occu-

pational

status

for

the

pair,

and

conversely we

want

to

predict

the

father's

occupational

status

for

the

pair

by

obtaining

the

knowledge

of

his son's

occupational

status

for

th

e pair, we

ar

e

interested

in

what

degree

the

pre-

diction becomes

better

when we know

the

information for one

of

the

pair

than

when we do

not

know

the

information. In such a case,

the

PRY

mea-

sure as

~~L

would

be

useful for measuring

the

degree

of

the

proportional

reduction

in variation.

Note

that

(1)

the

measures T()') ,

T,

U,

TL~~1

and

Utotal

are

usually used

when

the

row

and

column classifications have

both

nominal categories, (2)

the

measure

~~L

is used when

those

have

both

ordered

categories,

and

(3)

the

PRY

measure proposed

by

Tomizawa

et

al. (2002) is used when one

of

row

and

column classifications

has

the

nominal category

and

the

other

has

the

ordered category.

6.

Conclusions

The

present

paper

has

analyzed several categorical

data

and

compared

the

degree

of

PRY

betwe

en

them

using

the

total

uncertainty

measure

~~L·

In

the

present

paper

we have seen

that

when

we

want

to

predict

the

value

of

one variable by knowing

the

value

of

the

other

variable,

the

prediction

based

on

the

information would

be

useful for

the

unaided

distance vision

data

and

for

the

data

of

socioprofessional

status

rath

er

than

for

the

data

of

temperatures

in

two years

and

for

th

e

data

of

numbers

of

lambs

born

in

consecutive years.

7.

Discussion

The

data

in Table 8,

taken

from

Everitt

(1992, p. 56) show

the

frequencies

obtained

when 284 consecutive admissions

to

a

psy

c

hiatric

hospital

are

348

classified

with

respect

to

social class

and

diagnosis.

Everitt

examined

what

degree

the

knowledge

of

a

patient's

social class is useful for

predicting

his

diagnostic

Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

Подождите немного. Документ загружается.