Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics
Подождите немного. Документ загружается.


This page intentionally left blankThis page intentionally left blank

Quantum
Bio-Informatics
IV
eds. L.
Accardi,
W.
Freudenberg
and
M.
Ohya
© 2011
World
Scientific
Publishing
Co.
(pp.
311-319)
DISCRETE
APPROXIMATION
TO
OPERATORS
IN
WHITE
NOISE
ANALYSIS
SI SI
Faculty
of
Information
Science and Technology
Aichi
Prefectural University
Aichi
Prefecture, Japan
2000 AMS
Classification:
60H40
In
this
paper
we
discuss
how
to
approximate
white
noise
functionals
and
the
oper-
ators
in
white
noise
analysis
by
using
variables
depending
on
discrete
parameter.
We
discuss
to understand
the
basic
idea
and
real
meaning
of
approximation
of
operators.
1.
Introduction
Main
aim
of
this
report
is
to
discuss a
method
of
approximation
of
white
noise functional
by
using a
system
of
variables
with
discrete
parameter.
Then,
we
naturally
proceed
to
the
approximation
of
operators.
When
we discuss
approximation
of
nonlinear
functionals of
white
noise
we
meet
a crucial difficulty. Namely,
to
come
to
some
nonlinear
functionals
of
13(t)'s we
are
naturally
required
to
have
the
so-called renormalization.
Thus,
we shall provide a general
theory
of
renormalization
of
white
noise
functionals.
First,
we shall
take
a
system
{Xn'
n E
Z}
of
standard
Gaussian
random
variables
and
their
functions.
We
then
come
to
approximation
of
white
noise functional
cp(
13
(t), t E
R}
by
those
of
Xn's.
In
this
course, we
can
see reasons
why
renormalization
is necessary for some
white
noise functionals. So
this
approximation
that
we discuss
in
this
note
is very
much
different from
the
approximation
of
ordinary
functions.
The
last
section is
devoted
to
the
approximation
of
operators
acting
on
the
space
of
white
noise functionals.
311
312
2.
Analysis
of
white
noise
functionals
We wish
to
discuss functionals rp(B(t), t E R), noting
that
{B(t)
, t E
R}
is
a
system
of idealized elemental
random
variables.
First,
we form basic
functionals of
B(t)'s,
that
is polynomials in
B(t)'s.
Consider a system
A = algebra generated by
the
system
{I,
B(t),
t E
R}.
Proposition
2.1.
A forms a graded algebra, that is
i) A is an algebra.
ii) A =
L
~=o
An,
(algebraic direct
sum)
where
An
= {homogeneous polynomials of degree n}
iii)
An·
Am
=
{fn(B)gm(B);f
n
E
An
,gm
E
Am}.
Remark
2.1.
B(t)
=
B(t,
w),
wE
n(fl),
B
is
Brownian motion. For every
w,
B(t,
w)
is
defined as a generalized function of t.
But
smeared variables
B(~),~
E
E,E
being a nuclear space, are well defined as
ordinary
random
variables.
Remark
2.2.
The
sigma field generated by
{B(t),
t E
R}
is
understood
to
be
equa
l
to
the
sigma field B generated by
{B(~),~
E
E}.
It
is known
that
00
(L2)
==
L
2
(n,B,fl)
=
EB'H
n (Fock space)
n=O
In
particular,
HI
is
spanned
by
B(~)
,
~
E E
and
we have
As
an
extension of
the
isometry
we
have
Also
it
is shown
that
(1)
can
be
extended
to
'Hi-
I)
~
K(-I)(R),
(1)
(2)
where
K(
-1)
(R) is
the
Sobolev space
of
degree
-lover
Rl.
Since
K(
-1)
(R)
contains
delta
function
btU
, we see
that
B(t)
is a well defined
member
of
H
(-
I)
1 .
313
In
this
line
the
orthogonalization
A~
of
the
sub-algebras
An leads us
to
define a space
H~
-n)
of
generalized
white
noise functionals
of
degree
nand
finally come
to
the
space
of
generalized
white
noise functionals :
(L2)- =
EBcn1{~-n).
3.
Discrete
parameter
case
We
restrict
time
parameter
to
[0,1].
The
linear space
spanned
by
(E,
~n)"s
~n
being
a
base
of
L2([0, 1]) is
the
same
as
the
space
spanned
by
the
(E,
Xn,k) where
= n
lln
=
[k
- 1
~]
Xn,k
XLl.
k
,
k
2n'
2
n
.
Write
X;:
=
2~
(E,
Xn,k)'
Then
X;:'s
are
independent
identically
N(O,
l)-distributed,
n =
0,1,2""
; 1
:s;
k
:s;
2n.
Obviously
B(X;:, n = 0,
1,2,'"
; 1
:s;
k
:s;
2n)
= B = V
Bn
n
where
Bn
=
B(X;:,
1
:s;
k
:s;
2n).
We have
n
Theorem
3.1.
The space
(L;J
is increasing
in
n and inductive limit
of
(L;J is equal to (L2).
4.
Operators
:
from
discrete
to
continuous
form
We first
approximate
a
Brownian
motion
by
Levy's
construction
(see
[7])
which is fitting
to
realize
an
approximation.
That
is,
we
should
take
an
independent
system
{Ll.jnB}, which
approximates
Brownian
motion
when
k
{llk}
is
getting
finer.
Thus,
we
take
{Ll.}!}
as a basic
system
for
the
random
variables in
the
k
followings.
We
are
now going
to
give
the
interpretation
why
we
take
Frechet deriv-
ative
to
define 8
t
=
a:(t)'
In
fact, we define
at
as
a limit (in
the
sense
to
be
prescribed
below)
of
at,
where
{Xn}
is
independent
identically
N(O,
l)-distributed.
To see
the
idea
we
simply
note
in
the
followings.

314
1)
In
the
discrete
parameter
case let X =
(X
1
,X
2
,···),
Xi's
be
inde-
pendent
identically
and
N(O,
I)-distributed.
The
partial
derivative
a!L
f(X)
is defined
to
be
8
-8
f(x1,x2,···)1
-x··
Xn
x J - J
(3)
We wish
to
use analogous technique.
2)
Since
.6,nB
.
.6,~
-t
{t}
===?
~n
-t
B(t).
k
(4)
and
since
the
S-transform
of
B(t)
is
~(t),
we
can
use a
counter
part
of
(3). Namely, for
cp(B)
= cp(B(t), t E R1) first
take
8~~t)
(Scp)(~)
and
apply
S-l.
This
is expressible
as
8 =
~
=
S-1_
8
_(S
)
tCP
8B(t)
8~(t)
cp,
(5)
where
atct)
is
the
Frechet derivative.
Formally
we
follow this, however we need some
interpretations
to
have
(5)
understood
correctly.
Coming
back
to
(3),
the
partial
derivative
a~n
means
a derivative
with
respect
to
X
n
.
This
fact
should
correspond
to
a
variation
of
the
random
vari-
able
X
n
,
for which
we
recongnize
the
variation
within
the
world
of
random
function.
This
is difficult
to
be
justified. Having overcome
this
difficulty,
the
definition (5) is acceptable. In
the
expression (5),
the
Frechet deriva-
tive is
understood
to
be
a derivative
obtained
by
measuring
infinitesimal
variations in all possible direction.
The
idea
of
understanding
the
partial
derivative
with
respect
to
the
random
variable
B(t)
is
the
same
as
in
the
discrete case.
Concerning
the
definition of
the
partial
derivative 8
t
,
we
have
to
note
a crucial difference form
that
in
the
discrete case.
For
a~n'
the
variable
Xn
is a
standard
guage, i.e.
Xn
has
unit
variance.
On
the
other
hand
B(t)
is
an
infinitesimal
random
variable, formally
speaking
it
has
variance
it.
Such difference is
absorbed
by
the
S-transform.
Nevertheless, we
must
be
careful
when
8
t
is
approximated
by
a~n
in
the
discrete case.
The
examples which
are
now
in
order
illustrate
this
fact.
We
note
that
the
Frechet derivative lets
the
degree of homogeneous
functionals decrease
by
1, which
means
8
t
is
an
annihilation
operator.
It

315
acts in such a way
that
the
kernel function
F(
Ul,
...
,un
) associated
to
cp
E Hn becomes
The
S-transform
acting on (L2)- is expressible as
(Scp)(~)
=
C(~)(e(x,O,
cp(x))
since
e(-'·
) E (L2)+.
Set F =
{(Scp)(~);
cp
E (L
2
)-,
~
E
E}.
Theorem
4.1.
The vector space F can
be
topologiz
ed
to
be
a Repoducing
Kernel
Hilbert Space with kernel
C(~
-
7)),
(~,
7))
E E x E
and
F~(L2)-.
Example
4.1.
If
j
is
continuous,
then
at J
j(u)B(u)dU
=
j(t).
In
particular,
atB(s)
=
o(t
- s), where 0
is
the
delta
function.
This
is
the
analogue of
Example
4.2.
at :
B(s)n
:=
(n
-
1)
:
B(t)n-l
:
o(t
- s).
Note
that
0 (t -
s)
follows in
the
above expression.
Example
4.3.
For
the
Gauss kernel
cp
=
Nee!
B(U)2du,
(6)
Thus,
atcp
= 2c : B(t)cp
:,
(7)
which comes from
the
variation of
S-transform
o
o~U(O
=
2c~(t)U(O,
(8)
(See
the
literature
6
).
Taking S- l-transform,
we
obtain
(7).
316
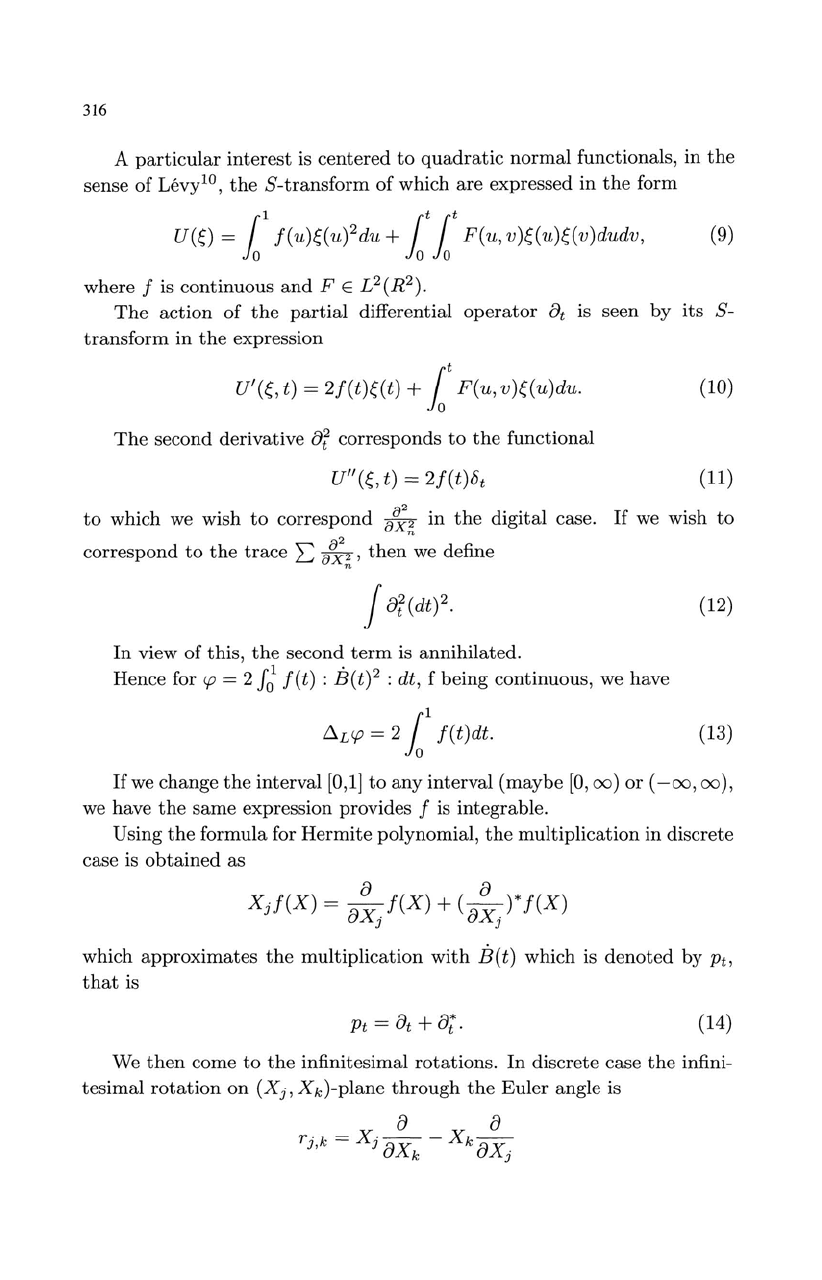
A
particular
interest
is centered
to
quadratic
normal
functionals,
in
the
sense
of
LevylO,
the
S-transform
of
which
are
expressed
in
the
form
U(O
=
10
1
f(U)~(U)2du+
1o
t
1o
t
F(u,v)~(u)~(v)dudv,
(9)
where f is continuous
and
F E L2(R2).
The
action
of
the
partial
differential
operator
at
is seen by
its
S-
transform
in
the
expression
UI(~,
t) =
2f(t)~(t)
+
10
t
F(u,
v)~(u)du.
(10)
The
second derivative
a;
corresponds
to
the
functional
U/I(~,
t) =
2f(t)8
t
(11)
to
which
we
wish
to
correspond
8~2
in
the
digital case.
If
we wish
to
correspond
to
the
trac
e
z=
8~~
,
then
nwe define
(12)
In
view of this,
the
second
term
is annihilated.
Hence for
<p
= 2
f01
f(t)
: B(t)2 : dt, f being continuous, we have
l1
L
<p
= 210
1
f(t)dt.
(13)
If
we
change
the
interval
[0,1]
to
any
interval (maybe
[0
,
00)
or
(-00,00),
we have
the
same
expression provides f is integrable.
Using
the
formula for
Hermite
polynomial,
the
multiplication in discrete
case is
obtained
as
Xjf(X)
=
o~f(X)
+
(o~)*f(X)
J J
which
approximates
the
multiplication
with
B(t) which is
denoted
by Pt,
that
is
(14)
We
th
en come
to
the
infinitesimal rotations.
In
discrete case
the
infini-
tesimal
rotation
on
(Xj,
Xk)-plane
through
the
Euler
angle is
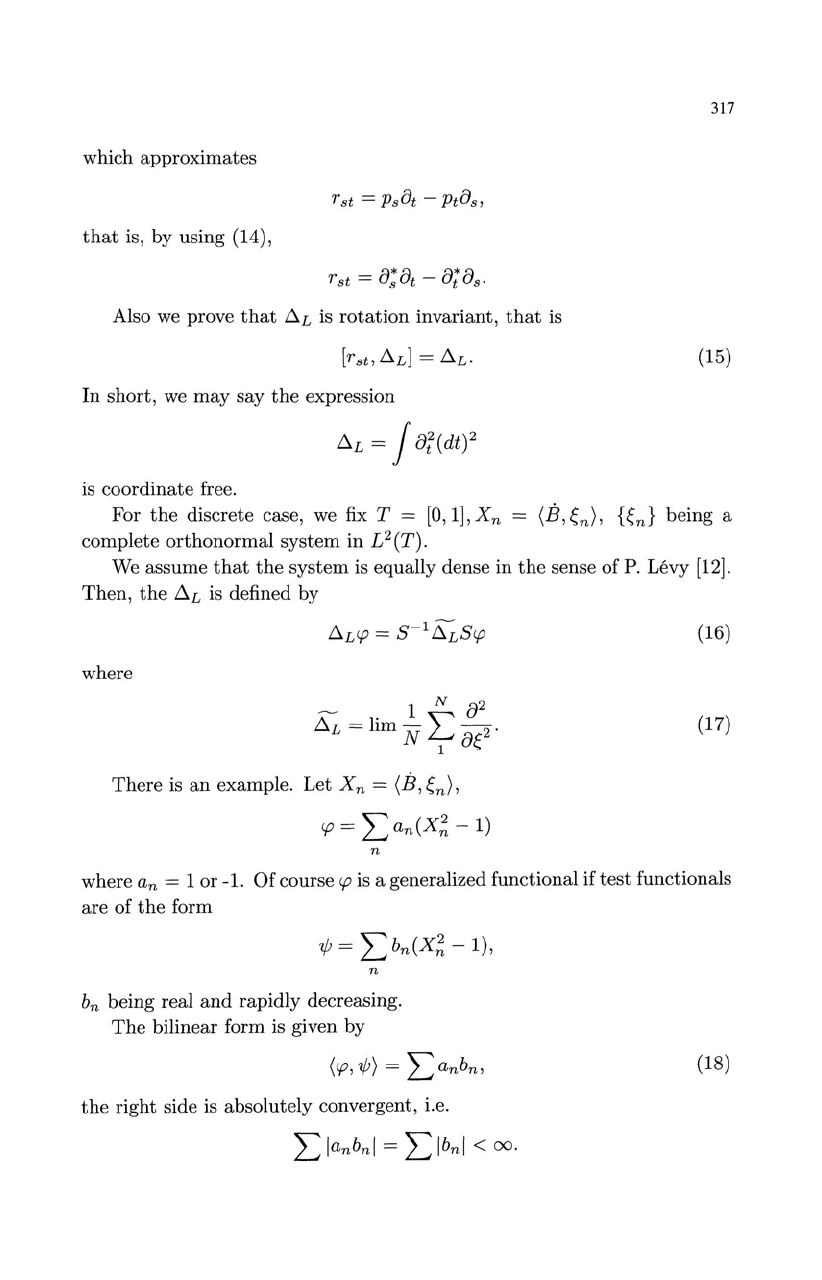
317
which
approximates
that
is, by using (14),
Also
we
prove
that
6.£
is
rotation
invariant,
that
is
(15)
In
short,
we
may
say
the
expression
6.£
= J &;(dt)2
is
coordinate
free.
For
the
discrete case, we fix T =
[O,I],X
n
(B,(n),
{(n}
being a
complete
orthonormal
system
in
L
2
(T).
We assume
that
the
system
is equally dense in
the
sense
of
P. Levy
[12].
Then,
the
6.£
is defined by
(16)
where
_ 1 N
&2
6.£
= lim N
~
&e'
(17)
There
is
an
example. Let
Xn
=
(B,(n),
cp
= I>n(X'; -
1)
n
where an = 1
or
-1.
Of
course
cp
is a generalized functional if
test
functionals
are
of
the
form
n
b
n
being real
and
rapidly
decreasing.
The
bilinear form is given by
(18)
the
right side is absolutely convergent, i.e.

318
And
_
IN
6.L<P
= lim N
Lan
1
exists for periodic
an.
The
limit changes by
rotation.
Thus,
we see
that
there
is a difference between
the
discrete case
and
the
continuous case such
that
the
Laplacian
in
the
discrete case is
not
coordinate
free
although
the
Laplacian in
the
continuous case is
coordinate
free.
Acknowledgement
The
author
wishes
to
express
her
deep
gratitude
to
the
organizers for
the
invitation
to
International
conference
QBIC
2010, held
in
Tokyo University
of Science.
References
1.
L.
Accardi
and
V. Bogachevet,
Th
e
Ornstein
Uhlenb
eck
Process
and
the
Dirichlet
Form
associated
to
Levy
Laplacian,
N. 193
Volterra
Center,
2004.
2.
T.
Hida,
Canonical
representations
of
Gaussian
processes
and
their
applica-
tions. Mem.
ColI. Sci. Univc.
Kyoto
, 34 (1960), 109-155.
3.
T.
Hida
and
N. Ikeda, Analysis
on
Hilbert
space
with
reproducing
kernel
arising from
multiple
Wiener
integral.
Proc,
5th
Berkeley Symp.
on
Math.
Statistics
and
Probability. vol.
2,
(1967) 117-143.
4.
T.
Hida, Analysis
of
Brownian
functionals.
Carleton
Math.
Lecture
Not
es no.
13,
Carleton
University, 1975.
5.
T.
Hida,
Theory
of
Probability.
Floundation
and
Developments
.
Kyouritsu
Pub.
Co.
in
Japanese
2010.
6.
T.
Hida
and
Si Si,
An
innovation
approach
to
random
fields.
Application
of
white
noise theory. World Scientific
Pub.
Co. 2004.
7.
T.
Hida
and
Si Si,
Lectures
on
white
noise
function
als. World. Sci.
Pub.
Co.
2008.
8. T . Hida,
Theory
of
Probability.
Floundation
and
Dev
elopments.
Kyouritsu
Pub.
Co.
in
Japanese
2008.
9.
P. Levy,
Processus
stochastiques
et
mouvement
brownien.
Gauthier-Villars.
1948. 2eme ed.
with
supplement
1965.
10.
P. Levy,
Problemes
concrets
d'analyse
fonctionnelle.
Gauthier-Villars
. 1951.
11.
J.
Mikusinski,
On
the
square
of
the
Dirac
delta-distribution.
Bulletin
de
l
'Aca
demi
e Polonaise des Sciences. Ser.
math,
astro
et
Phys.
14 (1966), 511-
513.
12.
Si Si
and
T.
Hida, Some
aspects
of
quadratic
generalized
white
noise func-
tionals.
Proc.
QBlC08
held
at
Tokyo Univ.
of
Science. 2008,
QP-PQ,
Vol,
XXIV
2009,
World
Scientific
Publ.
Co., 184-191

319
13. Si Si,
An
aspect
of
quadratic
Hida
distributions
in
th
e re
alization
of a
duality
betwe
en Ga
ussian
a
nd
Poisson noises.
IDAQP
11
(2008) 109-118.
14.
Si Si,
Introduction
to
Hida
distributions
.
World
Sci.
Pub.
Co. 2010.
to
a
ppear
