Voit J. The Statistical Mechanics of Financial Markets

Подождите немного. Документ загружается.

3.2 Bachelier’s “Th´eorie de la Sp´eculation” 29

• Unlike modern bond futures, Bachelier’s futures do not include an obliga-

tion of delivery of the bond at maturity. Only the price diﬀerence of the

underlying bond is settled in cash, as would be done today with, e.g., index

futures. The advantage of buying the future, compared to an investment

into the bond, then is that only price changes must be settled. The impor-

tant investment in the bond upfront can thus be avoided, and the leverage

of the returns is much higher.

• The expiry date is the last trading day of the month. The price of the fu-

tures is ﬁxed on entering the contract, cf. Sect. 2.3.2, and the long position

acquires all rights deriving from the underlying bond, including interest.

• The long position receives the interest payments (coupons) from the fu-

tures.

• Bachelier’s futures can be extended beyond their maturity (expiry) date,

to the end of the following month, by paying a prolongation fee K.This

is not possible on present day futures. It conveys some option character to

Bachelier’s futures because its holder can decide to honor the contract at

a later stage where the market may be more favorable to him.

Market Hypotheses

Bachelier makes a series of assumptions on his markets which have become

standard in the theory of ﬁnancial markets. He postulates that, at any given

instant of time, the market (i.e., the ensemble of traders) is neither bullish nor

bearish, i.e., does not believe either in rising or in falling prices, a hausse or

baisse of the market. (Notice that the individual traders may well have their

opinion on the direction of a market movement.) This is, in essence, what has

become the hypothesis of “eﬃcient and complete” markets. In particular:

• Successive price movements are statistically independent.

• In a perfect market, all information available from the past to the present

time, is completely accounted for by the present price.

• In an eﬃcient market, the same hypothesis is made, but small irregularities

are allowed, so long as they are smaller than applicable transaction costs.

• In a complete market, there are both buyers and sellers at any quoted price.

They necessarily have opposite opinions about future price movements, and

therefore on the average, the market does not believe in a net movement.

The Regular Part of the Price of Bachelier’s Futures

Let us assume that there are no ﬂuctuations in the market. The price of the

futures F is then completely governed by the price movements of the underly-

ing security S which is shown in Fig. 3.1. Due to the accumulation of interest,

the value, and therefore the price, of the bond increases linearly in time by

Z = 75c over three months. When the coupon is detached from the bond at

times t

i+1

− t

= 3m), the value of the bond decreases instantaneously

30 3. Random Walks in Finance and Physics

i+1

100.00

100.75

Fig. 3.1. Deterministic part of the spot price evolution of the underlying French

government bond. t

denotes the time where a 75c coupon is detached from the

bond

by Z. The movement of the futures price is more dramatic, reﬂecting only

price changes, but reproduces the basic pattern of Fig. 3.1. In the absence

of prolongation fees (K = 0), immediately after the payment of interest at

some t

, the value of the futures contract is zero. Due to the accumulation of

interest on the underlying bond, the futures price then increases linearly in

time to 75c, immediately before t

i+1

F (t)=S(t) −S(t



i+1

− t



(t − t

)fort

≤ t ≤ t

i+1

. (3.1)

This is because at maturity, the price diﬀerence accumulated on the underly-

ing bond is settled between the long and short positions. Immediately after

the maturity date, the value of the futures falls to zero again as shown as

the solid line in Fig. 3.2. The holder of the futures receives the interest pay-

ment of the underlying bond. Notice the leverage on the price variations of

the futures. The bond price varies by 0.75% each time a coupon is detached

while the futures varies by 100% because the interest payment is 0.75% of

the bond value, but makes up the entire value of the futures. With a ﬁnite

prolongation fee K, price movement will be less pronounced. In the extreme

case where K = Z/3, the value of the futures contract at maturity, after

one month, will be equal to the initial investment for carrying it on, i.e., K.

It will then jump up by K due to the cost of prolongation, etc. This is the

3.2 Bachelier’s “Th´eorie de la Sp´eculation” 31

i+1

Fig. 3.2. Deterministic part of the evolution of the futures price F for three diﬀerent

prolongation fees: K =0(solid line), K = Z (dotted line), and 0 <K<Z(dashed

line)

dotted line in Fig. 3.2. For intermediate Z>K>0, the futures price will

vary as represented by the dashed lines in Fig. 3.2: the value is K<Z/3

immediately after interest payment, from where it increases linearly to Z/3

at the ﬁrst maturity date, jumps by another K and increases to 2Z/3 at the

second maturity date, etc., to t

i+1

where interest is paid, and the value falls

back to K.

The important observation of Bachelier now is that all prices on any

given line F(t) [or S(t)] are equivalent. As long as the price evolution is

deterministic, the return an investor gets from buying the futures (or bond)

at any given time is the same, provided the price is on the applicable curve

F (t)[orS(t)]. The returns are the same because the slope is independent

of time. For a given K, all prices on one given curve represent the true, or

fundamental (in modern terms), value of the asset. For a given prolongation

cost K the drift of the true futures price is

dF (t)

dS(t)

−

i+1

− t

)

, (3.2)

between two maturity dates.

If now ﬂuctuations are added, and the current spot price of the futures is

F (t), the true, or fundamental value of the futures a time t + T from now, is

F (t + T )=F (t)+

T, (3.3)

32 3. Random Walks in Finance and Physics

provided no maturity date occurs during t. The eﬀect of a maturity date can

be included as described above, and a similar relation holds for the funda-

mental price of the bond. Of course, there is no guarantee that the quoted

price at t + T will be equal to

F (t + T ).

3.2.2 Probabilities in Stock Market Operations

Bachelier distinguishes two kinds of probabilities, a “mathematical” and a

“speculative” probability. The mathematical probability can be calculated

and refers to a game of chance, like throwing dice. The speculative prob-

ability may not be appropriately termed “probability”, but perhaps better

“expectation”, because it depends on future events. It is a subjective opinion,

and the two partners in a ﬁnancial transaction necessarily have opposite ex-

pectations (in a complete market: necessarily always exactly opposite) about

those future events which can inﬂuence the value of the asset transacted.

The probabilities discussed here, of course, refer to the mathematical

probabilities. Notice, however, that the (grand-) public opinion about stock

markets, where the idea of a random walk does not seem to be deeply rooted,

sticks more to the speculative probability. Also for speculators and active

traders, the future expectations may be more important than the mathemat-

ical probability of a certain price movement happening. The mathematical

probabilities refer to idealized markets where no easy proﬁt is possible. On the

other hand, fortunes are made and lost on the correctness of the speculative

expectations. It is important to keep these distinctions in mind.

Martingales

In Sect. 3.2.1, we considered the deterministic part of the price movements

both of the French government bond, and of its futures. There is a net return

from these assets because the bond generates interest. Between the cash ﬂow

dates, there is a constant drift in the (regular part of) the asset prices and

most likely, there will also be a ﬁnite drift if ﬂuctuations are included. Such

drifts are present in most real markets, cf. Figs. 1.1 and 1.2. Consequently,

Bachelier’s basic hypothesis on complete markets, viz. that on the average,

the agents in a complete market are neither bullish nor bearish, i.e., neither

believe in rising nor in falling prices, Sect. 3.2.1, must be modiﬁed to account

for these drifts which, of course, generate net positive expectations for the

future movements.

The modiﬁed statement then is that, up to the drift dF/dt,resp.dS/dt,

the market does not expect a net change of the true, or fundamental, prices.

(Bachelier takes the artiﬁcial case K = Z/3, i.e., the dotted lines in Fig. 3.2,

to formalize this idea.) However, deviations of a certain amplitude y,where

y = S(t) − S(0) or F (t) − F (0), occur with probabilities p(y), which satisfy



∞

−∞

p(y)dy = 1 (3.4)

3.2 Bachelier’s “Th´eorie de la Sp´eculation” 33

for all t. The expected proﬁt from an investment is then

E(y) ≡y =



∞

−∞

yp(y)dy>0solongas

⎧

⎨

⎩

> 0

Z>3K.

(3.5)

[The notation E(y) for an expectation value is more common in mathematics

and econometrics, while physicists often prefer y.] Such an investment is

not a fair game of chance because it has a positive expectation. However, for

fair game of chance : E(y)=0. (3.6)

This condition, the vanishing of the expected proﬁt of a speculator, is fulﬁlled

in Bachelier’s problem only if Z =3K,orifdS/dt or dF/dt is either zero or

subtracted out. Then a modiﬁed price law between the maturity dates

x(t)=y(t) −

t or x(t)=y(t) −

t, (3.7)

where t is set to zero at the maturity times (nt

for the bond and nt

/3for

the futures), must be used. This law fulﬁlls the fair game condition

E(x) ≡x =0. (3.8)

With these prices corrected for the deterministic changes in fundamental

value, the expected excess proﬁt of a speculator now vanishes. A clear sepa-

ration of the regular, or deterministic price movement, contained in the drift

term, and of the ﬂuctuations, has been achieved. Equation (3.8) emphasizes

that there is no easy proﬁt possible due to the fair game condition (3.6). Now

it is possible to attempt a statistical description of the ﬂuctuation process.

x(t) describes a drift-free time series. This is what is called, in the modern

theory of stochastic processes [32], a martingale, or a martingale stochastic

process, i.e., E(x) = 0, or more precisely (in discrete time)

E(x

t+1

− x

t−1

t−2

,...,x

)=0, (3.9)

where E(x

t+1

−x

,...) is the expectation value formed with the conditional

probability p(x

t+1

− x

,...)ofx

t+1

− x

, conditioned on the observations

t−1

t−2

,...,x

. One may also say that y(t), the stochastic process (time

series) followed by the bond price or any other ﬁnancial data, is an equivalent

martingale process. An equivalent martingale process is a stochastic process

which is obtained from a martingale stochastic process by a simple change of

the drift term, cf. (3.7).

The equivalent martingale hypothesis is equivalent to that of a perfect

and complete market, and approximately equivalent to that of an eﬃcient

and complete market.

34 3. Random Walks in Finance and Physics

Distribution of Probabilities of Prices

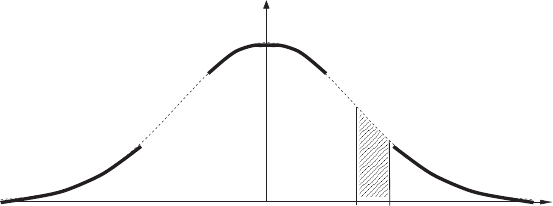
What can we say about the probability density p(x, t) of a price change of a

certain amplitude x, at some time t in the future? In attempting to answer

this question, Bachelier gave a rather complete, though sometimes slightly

inaccurate, formulation of a theory of the random walk, ﬁve years before

Einstein’s seminal paper [4].

From now on, we will assume that the price S(t) itself follows a martingale

process, or that all eﬀects of nonzero drifts have been incorporated correctly.

The general shape of the probability distribution at some time t in the future

is shown in Fig. 3.3. Here, p(x

,t)dx

is the probability of a price change

≤ x ≤ x

+dx

at time t. In a ﬁrst appoximation, the complete market

hypothesis requires the distribution to be symmetric with respect to x =0,

and the fair game condition, i.e., the assumption of a martingale process,

requires the maximum to be at x = 0 at any t, and to have a quadratic

variation for suﬃciently small x. Also, it must decrease suﬃciently quickly

for x →±∞to make p(x, t) normalizable. Strictly speaking, since the price

of a bond cannot become negative, p(x, t)=0forx<−S(0), but this eﬀect

is negligible in practice so long as ﬂuctuations are small compared to the

bond price.

The Chapman–Kolmogorov–Smoluchowski Equation Bachelier then

tries to derive p(x, t) from the law of multiplication of probabilities. If

p(x

)dx

is the probability of a price change x

≤ x ≤ x

+dx

at time

,andp(x

− x

)dx

is the probability of a change x

− x

in t

,the

joint probability for having a change to x

at t

and to x

at t

+ t

p(x

)p(x

−x

)dx

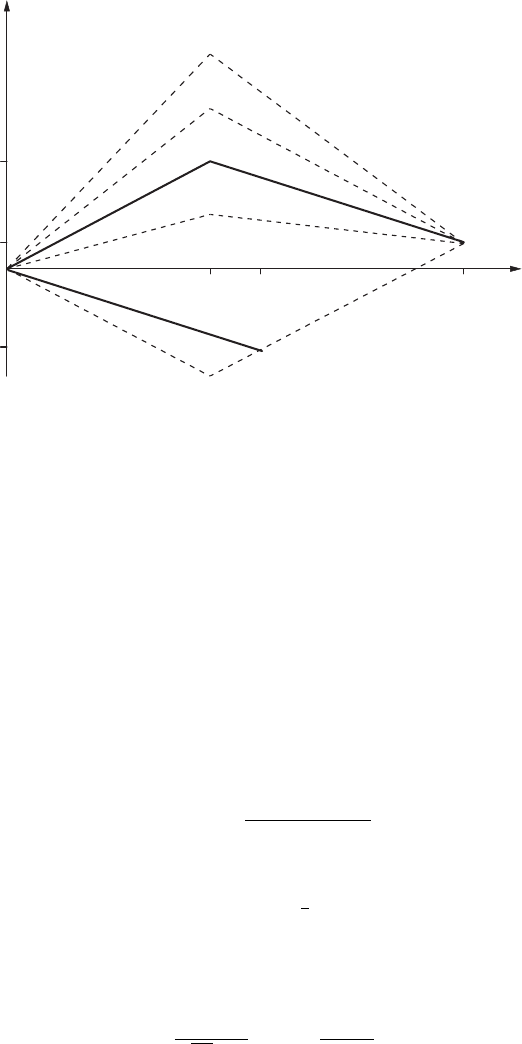
. These paths are shown as solid lines in Fig. 3.4.

Then, the probability to have a change of x

at t

+ t

, independent of the

intermediate values, is

p(x

+ t

)dx





+∞

−∞

p(x

)p(x

− x

)dx



. (3.10)

+dx

p(x)

Fig. 3.3. General shape of the probability density function p(x, t) of a price change

x at some time t in the future

3.2 Bachelier’s “Th´eorie de la Sp´eculation” 35

-x

Fig. 3.4. Multiplication of probabilities in the (x, t)-plane. Strictly speaking, only

the probabilities at t

, t

,andt

are used. For clarity, they have been connected

by straight “paths”. To derive the Chapman–Kolmogorov–Smoluchowski equation,

one must integrate over all values of x at t

. A few such paths a shown as dashed

lines

This equation is known in physics and mathematics as the Chapman–

Kolmogorov–Smoluchowski (CKS) equation, and was rederived there some

decades after Bachelier. It is a convolution equation for the probabilities

of statistically independent random processes (resp. Markov processes more

generally).

Bachelier solves this equation by the Gaussian normal distribution

p(x, t)=p

(t)exp



−πp

(t)x



. (3.11)

Inserting this into CKS (3.10) gives the condition

+ t

)

)+p

)

(3.12)

which in turn determines the time evolution of p(t)as

(t)=H/

√

t (3.13)

with a constant H. The substitution σ

= t/2πH

then gives the normal

form of the Gaussian

p(x, t)=

√

2πσ(t)

exp



−

2σ

(t)



. (3.14)

36 3. Random Walks in Finance and Physics

= 2

= 1

= 0.5

Fig. 3.5. The Gaussian distribution for three diﬀerent values of the standard de-

viation σ, i.e., three diﬀerent times t ∝ σ

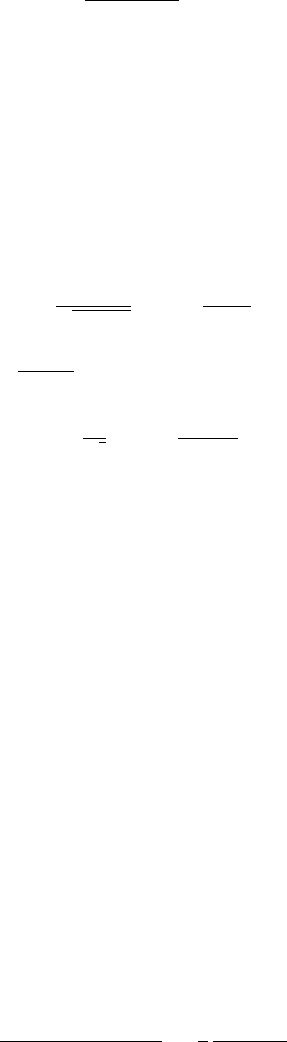
Its shape, for three diﬀerent values of σ, i.e., time, is shown in Fig. 3.5. The

following facts are important [set x

= 0 in Fig. 3.5 if you are interested in

changes or x

= S(0) if you are interested in absolute prices]: (i) for t =0,we

have σ = 0, and this corresponds to p(x)=δ(x), i.e., certain knowledge of

the price at present (not shown in Fig. 3.5); (ii) the peak of the distribution,

and its mean do not change with time, reﬂecting the martingale property;

(iii) the distribution function broadens slowly, only with σ ∝

√

t.Thisfact

(and eventual deviations thereof, of real markets) is of practical importance

since it excludes big price movements over moderately long time intervals.

An important problem is, however, that Bachelier did not recognize that

his “solution” to (3.10) is not the only solution, and in fact a rather special

one. Fortunately enough, Bachelier approached his problem along several

diﬀerent routes. He obtained the same solution (3.14) for two more special

problems, where it was both correct and unique. One was the solution of the

random walk, the other the formulation of a “diﬀusion law” for price changes.

The Random Walk A discrete model for asset price changes would consider

two mutually exclusive events A (happening with a probability p), and B

(with a probability q =1− p). These events can be thought to represent

price changes by ±x

, in one time step. Then the probability of observing,

in m events, α realizations of A and m − α realizations of B is given by the

binomial distribution

3.2 Bachelier’s “Th´eorie de la Sp´eculation” 37

A,B

(α, m −α)=

α!(m − α)!

(1 − p)

m−α

. (3.15)

One may now ask:

1. Which α maximizes p(α, m−α)atﬁxedm and p? The answer is α = mp,

and thus m − α = mq. In a ﬁnancial interpretation, this gives the most

likely price change after m time steps, e.g., trading days x

max

= m(p −

q)x

. A ﬁnite diﬀerence p − q would represent a drift in the market (in

this argument, one is not restricted to martingale processes).

2. What is the distribution function of price changes? The complete expres-

sion for general α, p, m has been derived by Bachelier [6]. It simpliﬁes,

however, in the limit m →∞, α →∞with h = α − mp ﬁnite, to

p(h)=

√

2πmpq

exp



−

2mpq



. (3.16)

3. For p = q =1/2, ﬁnally, and setting h → x, m = t/∆t with ∆t the unit

time step, and H =



2∆t/π, the Gaussian distribution

p(x)=

√

exp



−

πH



(3.17)

of (3.11)–(3.14) is recovered. In this limit of large m, one has passed from

discrete time and discrete price movements to continuous variables.

This is the ﬁrst formulation of the random walk, or equivalently of the theory

of Brownian motion, or of the “Einstein–Wiener stochastic process”.

Other quantities of interest, such as the probability for a price change

contained in a window, P (0 ≤ x(t) ≤ X), the expected width X of the

distribution of price changes, P (−X ≤ x(t) ≤ X)=1/2, or of the expected

proﬁt associated with a ﬁnancial instrument whose payoﬀ is x if x>0, and

zero if x<0 (i.e., an investment in options), have been derived by simple

integration [6].

The Diﬀusion Law Yet another derivation can be done via the diﬀusion

equation. For this purpose, assume that prices are discretized ...,S

n−2

n−1

n+1

n+2

,..., and that at some time t in the future, these prices are

realized with probabilities ...,p

n−2

n−1

n+1

n+2

,.... Then, one may

ask for the evolution of these probabilities with time. Speciﬁcally, what is the

probability p



of having S

at a time step ∆t after t? If we assume that a price

change S

→ S

n±1

must take place during ∆t, we ﬁnd p



=(p

n−1

+ p

n+1

)/2

because the price S

can either be reached by a downward move from S

n+1

occurring with a probability p

n+1

/2, or by an upward move from S

n−1

with

a probability p

n−1

/2. The change in probability of a price S

during the time

step ∆t is then

∆p

= p



− p

n+1

− 2p

+ p

n−1

→

∂

p(S, t)

∂S

(∆S)

(3.18)

38 3. Random Walks in Finance and Physics

if the limit of continuous prices and time is taken. On the other hand,

∆p

→

∂p(S, t)

∂t

∆t (3.19)

in the same limit, and therefore

∂

∂S

−

∂p

∂t

=0. (3.20)

p(S, t) therefore satisﬁes a diﬀusion equation, and the Gaussian distribution is

obtained for special initial conditions. These conditions p(S, 0) = δ[S −S(0)],

i.e., knowledge of the price at time t =0,applyhere.

Bachelier realized that (3.20) is Fourier’s equation, and that consequently,

one may think of a diﬀusion process, or of radiation of probability through a

price level.

These considerations are equally valid for Bachelier’s bonds and for his

futures. As has been discussed above, both prices diﬀer by their drift coeﬃ-

cients, and by an oﬀset corresponding to the nominal value of the bond, but

not in their ﬂuctuations. Therefore, the equivalent martingale processes for

both assets are the same, and the description of their ﬂuctuations achieved

here is valid for both of them. We will see later that the same model, with

only minor modiﬁcations, became the standard model for ﬁnancial markets.

Bachelier solved many other important problems in the theory of random

walks, always motivated by ﬁnancial questions. He calculated prices for simple

and exotic options, and solved the ﬁrst passage problem (the probability that

a certain price S, or price change x, is reached for the ﬁrst time at time t

in the future). He also solved the problem of diﬀusion with an absorbing

barrier – this corresponds to hedging of options with futures, and vice versa,

and the corresponding probability distribution is shown in Fig. 3.6. Here, one

requires that losses larger than a threshold, −x

, have zero probability. In

p(x)

-x

Fig. 3.6. Probability distribution for hedging of options with futures, equivalent

to diﬀusion with an absorbing barrier