Tsoulfanidis N. Measurement and detection of radiation

Подождите немного. Документ загружается.

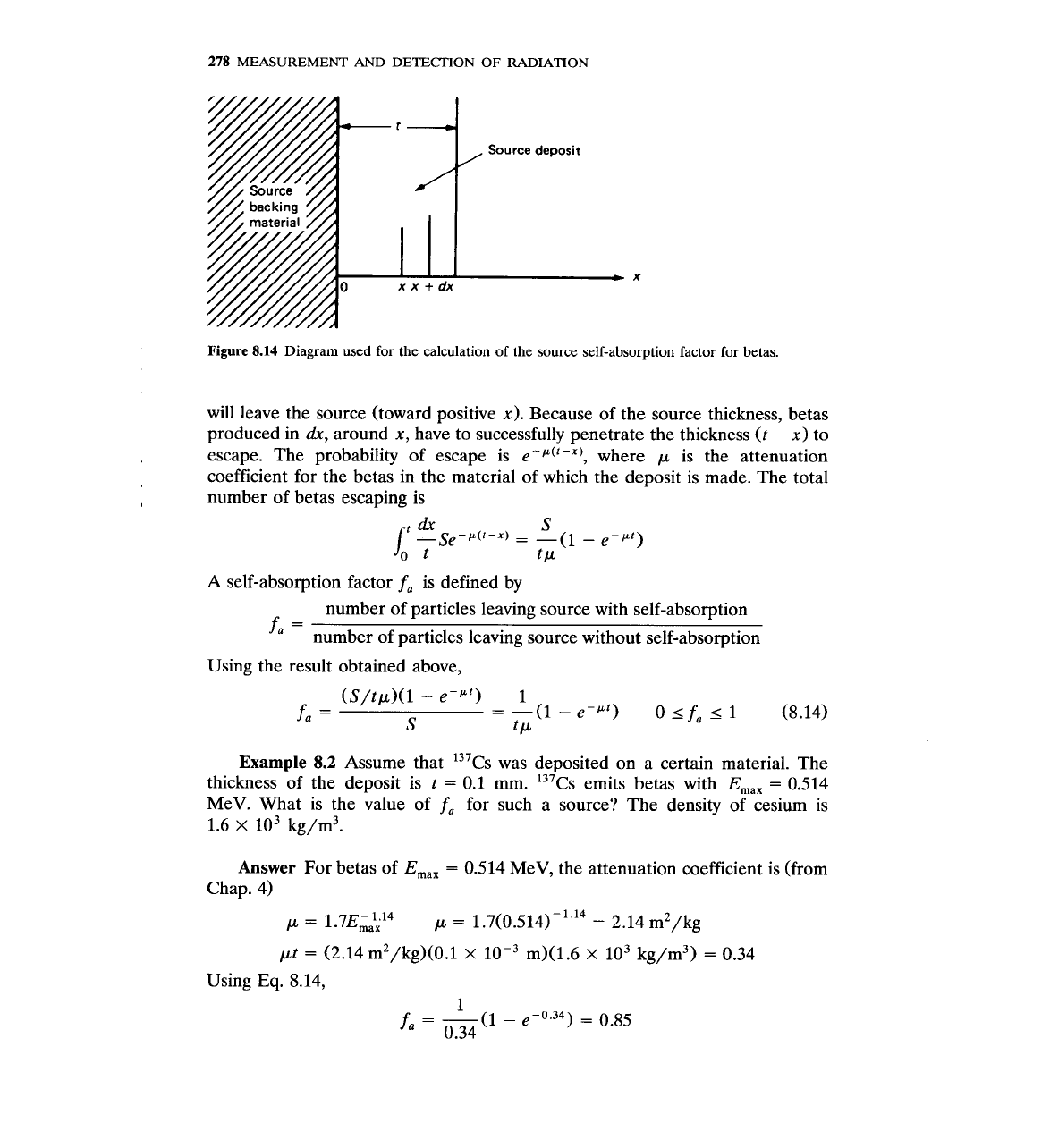
278

MEASUREMENT

AND

DETECTION

RADIATION

Source

deposit

)

xx+dx

Figure

8.14

Diagram used for the calculation of the source self-absorption factor for betas.

will leave the source (toward positive x). Because of the source thickness, betas

produced in

around x, have to successfully penetrate the thickness (t

x) to

escape. The probability of escape is e-"('-"), where

is the attenuation

coefficient for the betas in the material of which the deposit is made. The total

number of betas escaping is

self-absorption factor

is defined by

number of particles leaving source with self-absorption

number of particles leaving source without self-absorption

Using the result obtained above,

Example

8.2

Assume that '37~s was deposited on a certain material. The

thickness of the deposit is t

0.1 mm. 137~s emits betas with

Emax

0.514

MeV. What is the value of

for such a source? The density of cesium is

1.6

lo3

kg/m3.

Answer

For betas of

Emax

0.514 MeV, the attenuation coefficient is (from

Chap. 4)

II.

1.7E-'.I4

max

1.7(0.514)-~'~~

2.14 m2/kg

(2.14 m2/kg)(0.1

mK1.6

lo3

kg/m3)

0.34

Using

Eq.

8.14,

RELATIVE

AND

ABSOLUTE

MEASUREMENTS

279

Therefore, only

percent of the betas escape this source. Or, if this effect is

not taken into account, the source strength will have an error of 15 percent.t

the source emits monoenergetic charged particles, essentially all the

particles leave the source deposit as long as

where

range of the

particles. In practice, the sources for monoenergetic charged particles are such

that

in which case,

Then the only effect of the source deposit is

an energy loss for the particles that traverse it (see also Chap.

13).

8.3.2

Source Backscattering Factor

(f,)

source cannot be placed in midair. It is always deposited on a material that is

called

source backing

source suppoll.

The source backing is usually a very thin

material, but no matter how thin, it may backscatter particles emitted in a

direction away from the detector (Fig. 8.15). To understand the effect of

backscattering, assume that the solid angle

Fig.

8.15

lop2.

Also

assume that all the particles entering the detector are counted, self-absorption is

zero, and there is no other medium that might absorb or scatter the particles

except the source backing.

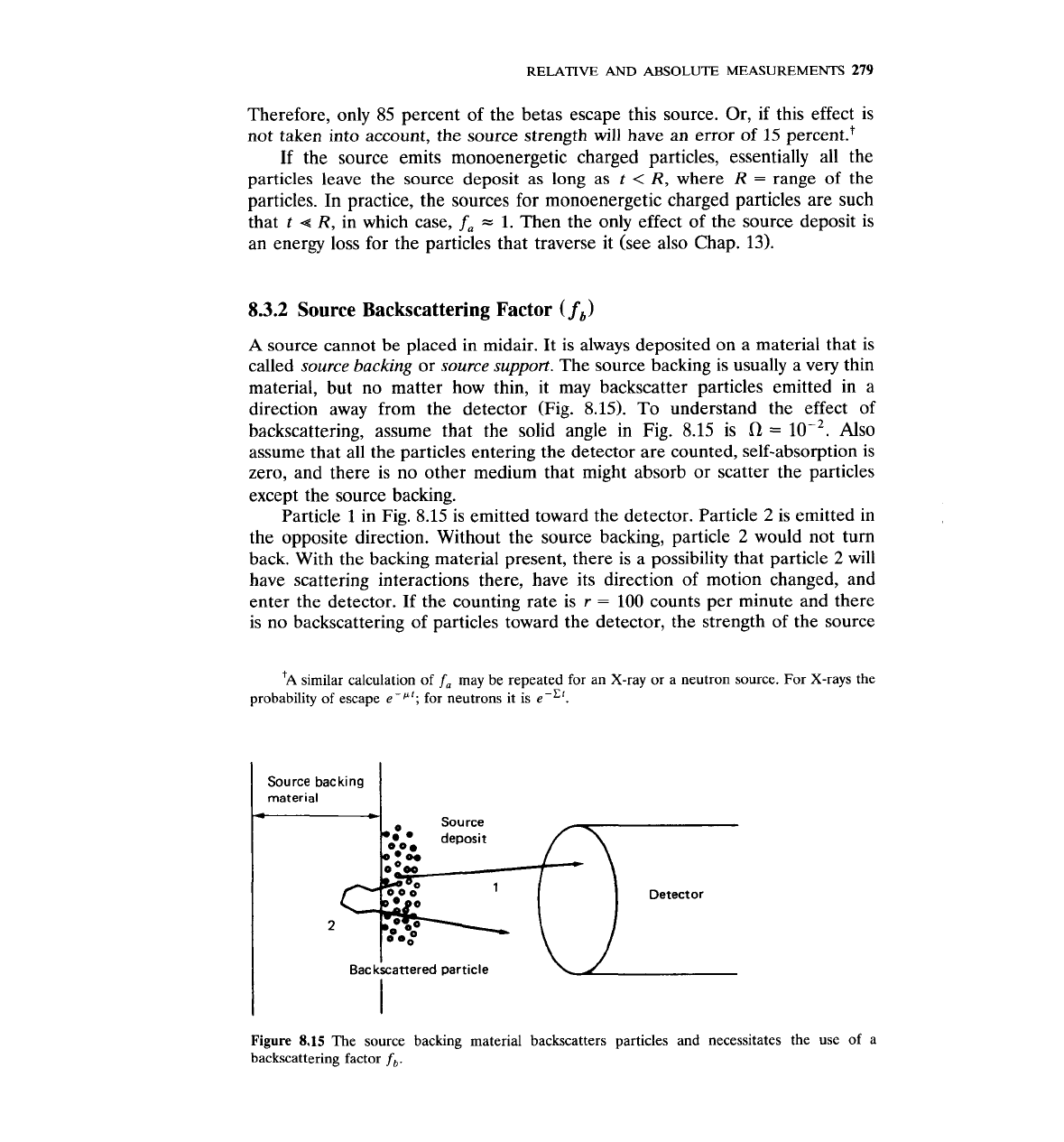
Particle

in Fig. 8.15 is emitted toward the detector. Particle

is emitted in

the opposite direction. Without the source backing, particle

would not turn

back. With the backing material present, there is a possibility that particle

will

have scattering interactions there, have its direction of motion changed, and

enter the detector. If the counting rate is

100 counts per minute and there

is no backscattering of particles toward the detector, the strength of the source

similar calculation of

may be repeated for an X-ray or a neutron source. For X-rays the

probability of escape

e-!-";

for neutrons it is

e-".

Source backing

material

Source

I?:.:

deposit

Backscanered particle

Figure

8.15

The source backing material backscatters particles and necessitates the use of a

backscattering factor

f,.

280

MEASUREMENT

AND

DETECTION

RADIATION

will be correctly determined as

100

s=-=--

10-~

10,000

part ./min

If the source backing backscatters

percent of the particles, the counting rate

will become

105

counts per minute, even though it is still the same source as

before. If source backscattering is not taken into account, the source strength

will be erroneously determined as

To correct properly for this effect, a source backscattering factor

(fb)

defined by

number of particles counted with source backing

(8.15)

number of particles counted without source backing

From the definition it is obvious that

2>fb21

In the example discussed above,

1.05,

and the correct strength of the

source is

105

s=-=

10,000

part ./min

lop2

1.05

The backscattering factor is important, in most cases, only for charged

particles. It depends on three variables:

Thickness

(b)

of the backing material

Particle kinetic energy

(T)

Atomic number of the backing material

(Z)

The dependence of

on thickness

is shown in Fig.

8.16.

which should be expected. For large thicknesses,

reaches a saturation

Figure

8.16

(a)

The backscattering factor

as a function of thickness

of the backing material.

(b)

The saturation backscattering factor as a function of the atomic number

of the backing material.

RELATIVE

AND

ABSOLUTE MEASUREMENTS

281

value, which should also be expected. Since charged particles have a definite

range, there is a maximum distance they can travel in the backing material, be

backscattered, and traverse the material again in the opposite direction. There-

fore an upper limit for that thickness is

R/2,

where

is the range of the

particles. Experiments have shown that

The dependence of the saturation backscattering factor of electrons on the

kinetic energy and the atomic number of the backing material is given by the

following empirical

equati~n,"?'~ based on a least-squares fit of experimental

results:

where the constants

for

1,2,.

. .

have these values:

Figure 8.17 shows the change of fb(sat) versus kinetic energy

for four

elements.

backscattering correction should be applied to alpha counting in

27r

counters (Fig. 8.8b). It has been determined13-l5 that the number of backscat-

0.05 0.1

Electron energy, MeV

Figure

8.17

Backscattering factor as a

function of energy for C,

Al,

Cu, and Au.

Curves were obtained using

Eq. 8.16.

282

MEASUREMENT

AND

DETECTION

RADIATION

tered alphas is between

and

percent, depending on the energy of the alphas,

the uniformity of the source, and the atomic number of the material forming the

base of the counter.

Correction for source backscattering is accomplished in two ways:

extremely thin backing material is used for which

In general, a

low2 material is used, e.g., plastic, if possible.

thick backing material is used, for which the saturation backscattering

factor should be employed for correction of the data.

For accurate results, the backscattering factor should be measured for the

actual geometry of the experiment.

8.4 DETECTOR EFFECTS

The detector may affect the measurement in two ways. First, if the source is

located outside the detector (which is usually the case), the particles may be

scattered or absorbed by the detector window. Second, some particles may enter

the detector and not produce a signal, or they may produce a signal lower than

the discriminator threshold.

8.4.1 Scattering and Absorption Due to the Window of the Detector

In most measurements the source is located outside the detector (Fig. 8.18). The

radiation must penetrate the detector window to have a chance to be counted.

Interactions between the radiation and the material of which the detector wall is

made may scatter and/or absorb particles. This is particularly important for

low-energy

particles.

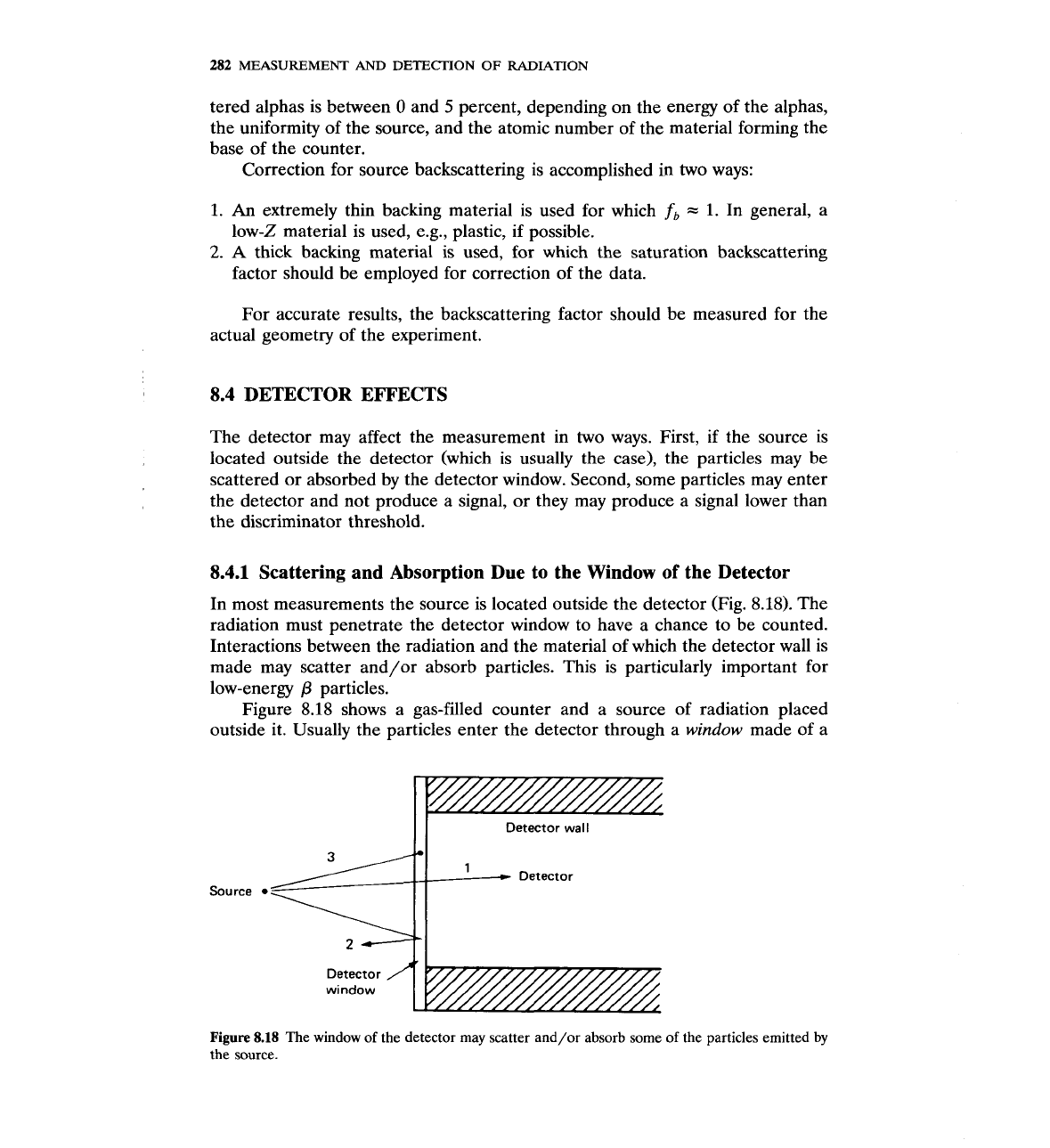
Figure 8.18 shows a gas-filled counter and a source of radiation placed

outside it. Usually the particles enter the detector through a

window

made of a

Detector wall

Detector

window

Figure

8.18

The window of the detector may scatter and/or absorb some of the particles emitted by

the source.

RELATIVE

AND

ABSOLUTE

MEASUREMENTS

283

very thin material (such as glass, mica, or thin metal). Looking at Fig. 8.18, most

of the particles, like particle

traverse the window and enter the counter. But,

there is a possibility that a particle, like particle

may be scattered at the

window and never enter the counter. Or, it may be absorbed by the material of

the window (particle 3).

In the case of scintillation counters, the window consists of the material that

covers the scintillator and makes it light-tight. In some applications the source

and the scintillator are placed in a light-tight chamber, thus eliminating the

effects of a window.

In semiconductor detectors, the window consists of the metallic layer

covering the front face of the detector. That layer is extremely thin, but may still

affect measurements of alphas and heavier charged particles because of energy

loss there.

There is no direct way to correct for the effect of the window. Commercial

detectors are made with very thin windows, but the investigator should examine

the importance of the window effect for the particular measurement performed.

If there is a need for an energy-loss correction, it is applied separately to the

energy spectrum. If, however, there is a need to correct for the number of

particles stopped by the window, that correction is incorporated into the

detector efficiency.

8.4.2

Detector Efficiency

(

)

It is not certain that a particle will be counted when it enters a detector. It may,

depending on the type and energy of the particle and type and size of detector,

go through without having an interaction (particle

in Fig.

8.19);

it may produce

a signal so small it is impossible to record with the available electronic instru-

ments (particle 3); or, it may be prevented from entering the detector by the

window (particle

4).

In Fig. 8.19, the particle with the best chance of being

detected is particle

No interaction-No pulse

Source

Figure

8.19

Particles detected are those that interact inside the detector and produce a pulse higher

than the discriminator level.

284

MEASUREMENT

AND

DETECTION

RADIATION

The quantity that gives the fraction of particles being detected is called the

detector eficiency

given by

number of particles recorded per unit time

number of particles impinging upon the detector per unit time

(8.17)

The detector efficiency depends upon1

Density and size of detector material

Type and energy of radiation

Electronics

Effect of density and size of detector material.

The efficiency of a detector will

increase if the probability of an interaction between the incident radiation and

the material of which the detector is made increases. That probability increases

with detector size. But larger size is of limited usefulness because the back-

ground increases proportionally with the size of the detector, and because in

some cases it is practically impossible to make large detectors. (Semiconductor

detectors are a prime example.)

The probability of interaction per unit distance traveled is proportional to

the density of the material. The density of solids and liquids is about a thousand

times greater than the density of gases at normal pressure and temperature.

Therefore, other things being equal, detectors made of solid or liquid material

are more efficient than those using gas.

Effect of type

and

energy of radiation.

Charged particles moving through matter

will always have Coulomb interactions with the electrons and nuclei of that

medium. Since the probability of interaction is almost a certainty, the efficiency

for charged particles will be close to 100 percent. Indeed, detectors for charged

particles have an efficiency that is practically 100 percent, regardless of their

size or the density of the material of which they are made. For charged particles,

the detector efficiency is practically independent of particle energy except for

very low energies, when the particles may be stopped by the detector window.

Charged particles have a definite range. Therefore, it is possible to make a

detector with a length

such that all the particles will stop and deposit their

energy in the counter. Obviously, the length

should be greater than

where

is the range of the particles in the material of which the detector is made.

Photons and neutrons traversing a medium show an exponential attenuation

(see Chap.

4),

which means that there is always a nonzero probability for a

photon or a neutron to traverse any thickness of material without an interaction.

As a result of this property, detectors for photons or neutrons have efficiency

less than 100 percent regardless of detector size and energy of the particle.

'1n gamma spectroscopy, several other efficiencies are being used in addition to this one (see

Chap.

12).

RELATIVE

AND

ABSOLUTE

MEASUREMENTS

285

Effect

electronics.

The electronics of a detector affects the counter efficiency

indirectly. If a particle interacts in the detector and produces a signal, that

particle

will

be recorded only if the signal is recorded. The signal will be

registered if it is higher than the discriminator level, which is, of course,

determined by the electronic noise of the counting system. Thus, the counting

efficiency may increase if the level of electronic noise is decreased.

As an example, consider a counting system with electronic noise such that

the discriminator level is at

mV. In this case, only pulses higher than

mV will

be counted; therefore, particles that produce pulses lower than

mV will not be

recorded. Assume next that the preamplifier or the amplifier or both are

replaced by quieter ones, and the new noise level is such that the discriminator

level can be set at 0.8 mV. Now, pulses as low as 0.8 mV will be registered, more

particles will be recorded, and hence the efficiency of the counting system

increases.

If electronics is included in the discussion, it is the efficiency of the system

(detector plus electronics) that is considered rather than the efficiency of the

counter.

8.4.3

Determination of Detector Efficiency

The efficiency of a detector can be determined either by measurement or by

calculation. Many methods have been used for the measurement of detection

efficiency,16-l8 but the simplest and probably the most accurate is the method of

using a calibrated source, i.e., a source of known strength. In Fig. 8.19, assume

that the source is a monoenergetic point isotropic source emitting

particles

per second. If the true net counting rate is

counts per second, the solid angle

fl,

and the efficiency is

the equation giving the efficiency is

where

=fa

..-

is a combination of all the correction factors that may have

to be applied to the results. Note that the correction factors and the efficiency

depend on the energy of the particle.

Accurate absolute measurements rely on measured rather than calculated

efficiencies. Nevertheless, an efficiency calculation is instructive because it

brings forward the parameters that are important for this concept. For this

reason, two cases of efficiency calculation for a photon detector are presented

below.

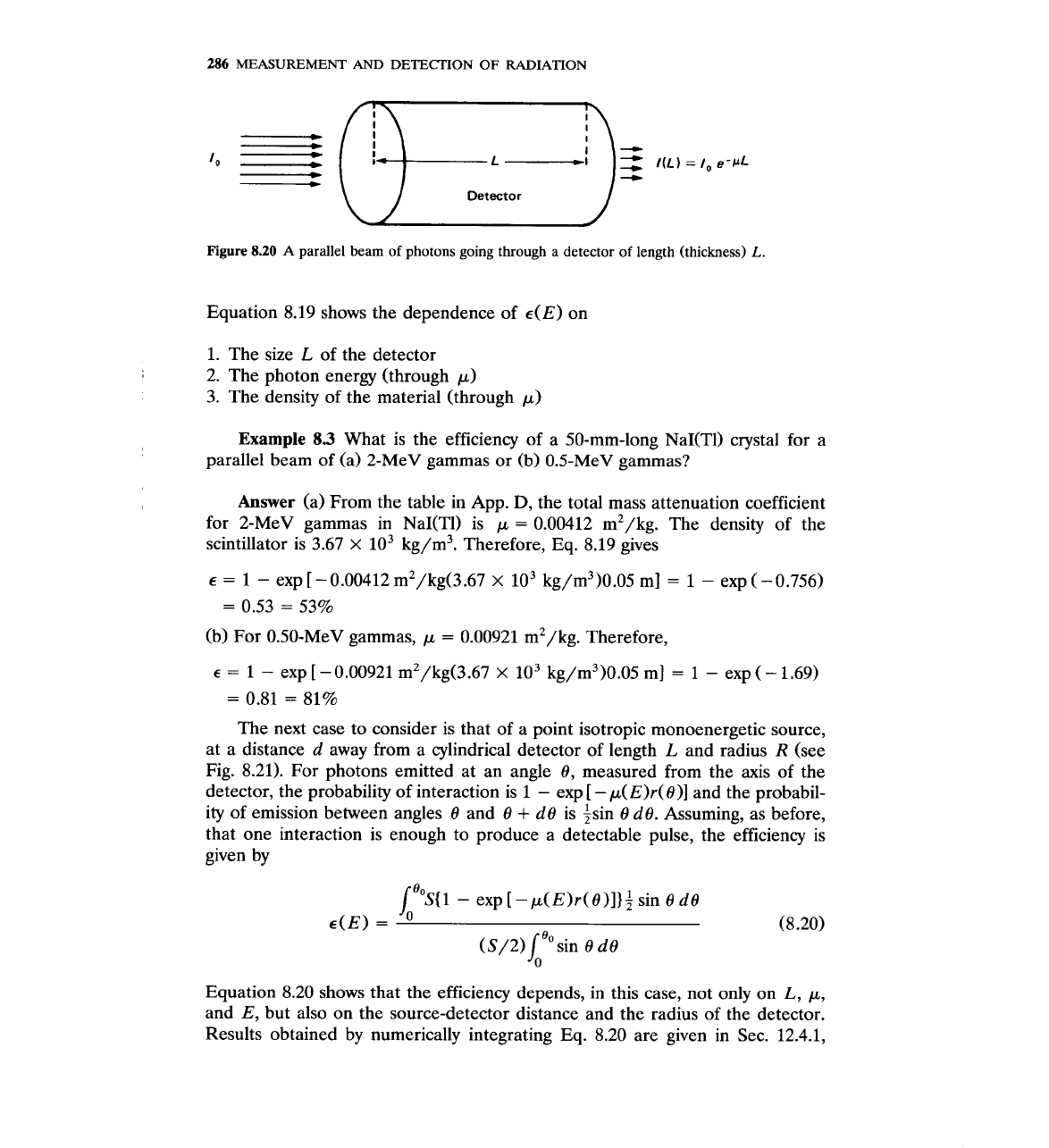
Consider first a parallel beam of photons of energy

impinging upon a

detector of thickness

(Fig. 8.20). The probability that a photon will have at

least one interaction in the detector is

eC'"(E)L, where

p(E)

is the total

linear attenuation coefficient of photons with energy

in the material of which

the detector is made. If one interaction is enough to produce a detectable pulse,

the efficiency is

E(~)

e-~(E)L

(8.19)

286

MEASUREMENT

AND

DETECTION

RADIATION

Figure

8.20

parallel beam of photons going through a detector of length (thickness)

Equation 8.19 shows the dependence of E(E) on

The size

of the detector

2. The photon energy (through p)

3. The density of the material (through p)

Example

8.3

What is the efficiency of a 50-mm-long NaI(T1) crystal for a

parallel beam of (a)

2-MeV

gammas or (b)

0.5-MeV

gammas?

Answer

(a) From the table in App.

the total mass attenuation coefficient

for 2-MeV gammas in NaI(T1) is

0.00412 m2/kg. The density of the

scintillator is 3.67

lo3

kg/m3. Therefore, Eq. 8.19 gives

exp

[

-0.00412 m2/kg(3.67

lo3

kg/m3)0.05 ml

exp

(

-0.756)

0.53

53%

(b) For 0.50-MeV gammas,

0.00921 m2/kg. Therefore,

exp

[

-0.00921 m2/kg(3.67

lo3

kg/m3)0.05 m]

exp

(

1.69)

0.81

81%

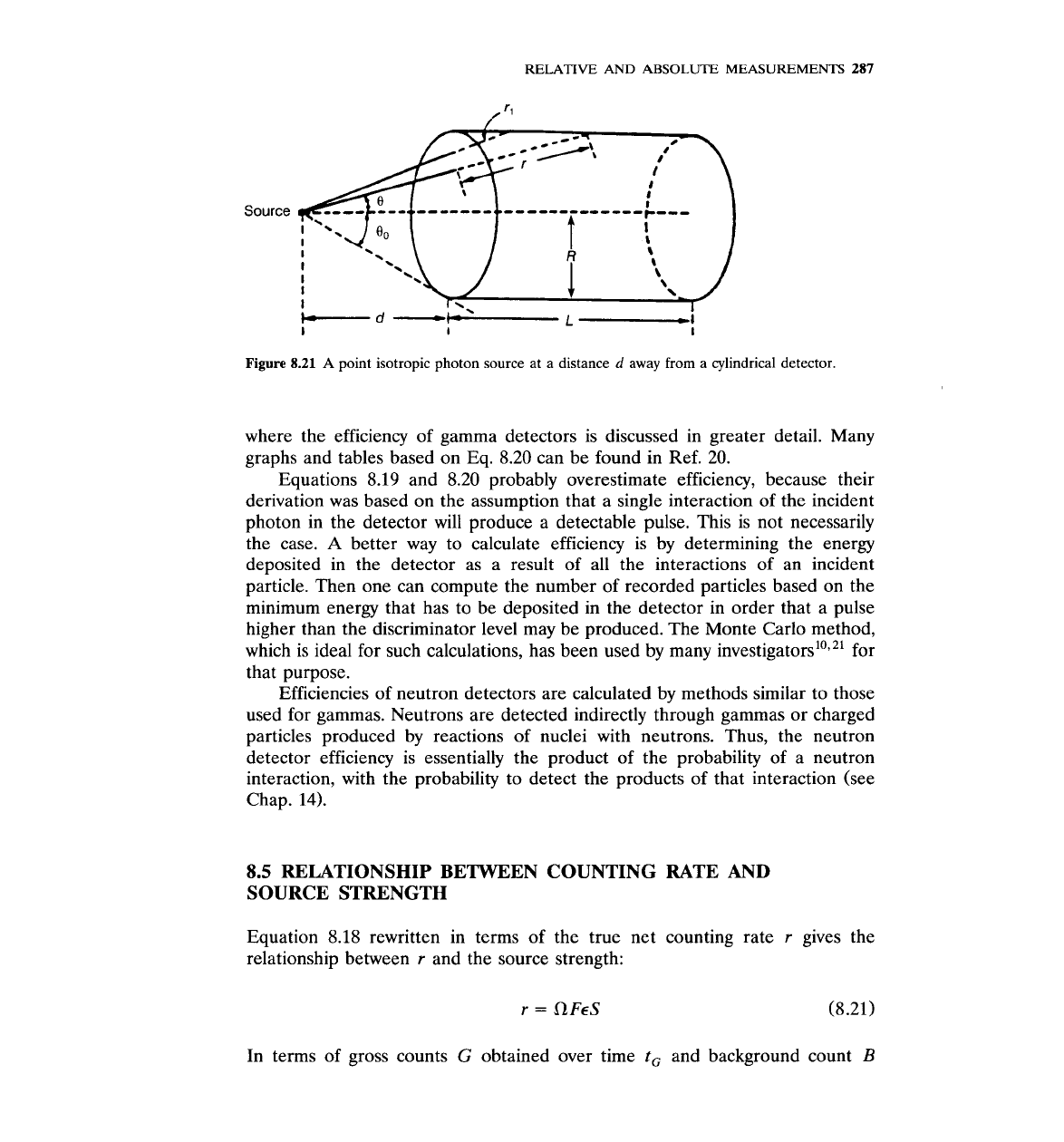
The next case to consider is that of a point isotropic monoenergetic source,

at a distance d away from a cylindrical detector of length

and radius

(see

Fig. 8.21). For photons emitted at an angle 8, measured from the axis of the

detector, the probability of interaction is

exp [-p(E)r(8)] and the probabil-

ity of emission between angles 8 and 8

d8 is isin 8 do. Assuming, as before,

that one interaction is enough to produce a detectable pulse, the efficiency is

given by

/oss{l

exp [-p(~)r(e)]}i sin 8 dB

E(E)

(8.20)

(s/~)/'o sin 8 dB

Equation

8.20

shows that the efficiency depends, in this case, not only on

and E, but also on the source-detector distance and the radius of the detector.

Results obtained by numerically integrating Eq. 8.20 are given in Sec. 12.4.1,

RELATIVE

AND

ABSOLUTE

MEASUREMENTS

287

Figure

8.21

point isotropic photon source at a distance

away from a cylindrical detector.

where the efficiency of gamma detectors is discussed in greater detail. Many

graphs and tables based on Eq. 8.20 can be found in Ref. 20.

Equations 8.19 and 8.20 probably overestimate efficiency, because their

derivation was based on the assumption that a single interaction of the incident

photon in the detector will produce a detectable pulse. This is not necessarily

the case.

better way to calculate efficiency is by determining the energy

deposited in the detector as a result of all the interactions of an incident

particle. Then one can compute the number of recorded particles based on the

minimum energy that has to be deposited in the detector in order that a pulse

higher than the discriminator level may be produced. The Monte

Carlo method,

which is ideal for such calculations, has been used by many

investigator^'^^^^

for

that purpose.

Efficiencies of neutron detectors are calculated by methods similar to those

used for gammas. Neutrons are detected indirectly through gammas or charged

particles produced by reactions of nuclei with neutrons. Thus, the neutron

detector efficiency is essentially the product of the probability of a neutron

interaction, with the probability to detect the products of that interaction (see

Chap.

14).

8.5

RELATIONSHIP BETWEEN COUNTING RATE

AND

SOURCE STRENGTH

Equation 8.18 rewritten in terms of the true net counting rate

gives the

relationship between

and the source strength:

In terms of gross counts

obtained over time

and background count