Troyan V., Kiselev Y. Statistical Methods of Geophysical Data Processing

Подождите немного. Документ загружается.

Basic concepts of the probability theory 73

In the ﬁrst case ξ(t) is called as a random process, in the second case it is called

as a random sequence. An example of the random process can be a graphic pre-

sentation of a seismic trace and an example of a random sequence can be digital

records of a seismic trace. Let’s consider a random function ξ(t) and we shall as-

sume, that for its study is yielded n independent trials, for example, the seismic

traces from n identical explosions is ﬁled. In each trial the realization of the random

function x

(t), x

(t), . . . , x

(t) is obtained. Let’s ﬁx an instant t = t

. If we were

interested with properties of ξ(t

) only in an instant t

, then for a continuous ran-

dom variable ξ

= ξ(t

) a complete description is reduced to the density function

f(x

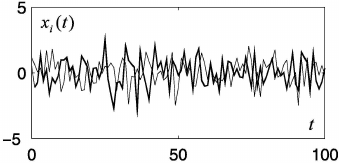
). An example of two realizations of random process is given in Fig. 1.44.

For more detailed description of a random function we shall choose two points t

Fig. 1.44 An example of two realizations of the random process.

and t

. The ordinates, relevant to them, ξ(t

) and ξ(t

) will be random variables ξ

and ξ

, which are completely characterised by a two-dimensional density function

f(x

, x

, t

). The random function is speciﬁed, if the multivariate density func-

tion f(x

, x

, . . . , x

, t

, . . . , t

) is given. Though such description of a random

function is complete, in practice, usually, only the ﬁrst two moments are considered.

The ﬁrst moment

= M[ξ(t

)] = hξ(t

is the mathematical expectation of the ordinate of the random function at an arbi-

trary time t

. Omitting an index 1 at t, we shall write

(t) = M[ξ(t)] = hξ(t)i.

The function m

(t) is not random and is completely determined by a distribution

law f (x/t):

(t) =

∞

−∞

xf(x/t)dx.

The central moments of the second order.

(1) Variance of a random function ξ(t) at ﬁxed timing t:

D[ξ(t)] = M[(ξ(t) − m

(t))

] = h(ξ(t) − m

(t))

74 STATISTICAL METHODS OF GEOPHYSICAL DATA PROCESSING

(2) Covariance moment of the random functions ξ(t

) and ξ(t

R(t

, t

) = cov(ξ(t

), ξ(t

)) = M[(ξ(t

) − m

))

× (ξ(t

) − m

))] = h(ξ(t

) − m

))(ξ(t

) − m

))i.

The function R(t

, t

) in the theory of random functions is called a correlation

function or an autocorrelation function. Writing down the explicit expression for

the mathematical expectation, we shall obtain

D[ξ(t)] =

∞

−∞

(x(t) − m

(t))

f(x/t)dx,

R(t

, t

) =

∞

−∞

(x(t

) − m

))

× (x(t

) − m

))f(x

, x

, t

)dx

A branch of the theory of random functions operating only the moments of

the ﬁrst two orders is called as the correlation theory of random functions. For any

random process ξ(t) the time average (on parameter t) is determined by the formula

hξ(t)i

= lim

T →∞

(1/2T )

−T

ξ(t)dt,

if this limit exists. As a result hξ(t)i

is a random quantity.

1.10.1 Properties of random functions

For the case of the stationary random function all multivariate distribution laws

depend only on a cross location of instants t

, t

, . . . , t

, i.e. for the stationarity of

a random function at arbitrary n should be fulﬁlled the next relation

f(x

, . . . , x

, . . . , t

) = f(x

, . . . , x

+ t

, . . . , t

+ t

were t

is an arbitrary number. In the speciﬁc case at n = 1 and n = 2, assuming

= −t

, for stationary functions we shall have accordingly

f(x

) = f(x

/0) = f(x

)

and

f(x

, x

, t

) = f(x

, x

/0, τ ),

where τ = t

− t

, i.e. the one-dimensional distribution law of an ordinate of a

random function does not depend on an instant of time, for which this ordinate is

Basic concepts of the probability theory 75

chosen, and the two-dimensional law depends only on a diﬀerence of instants. Using

expressions for distribution laws of stationary random functions, we shall obtain

(t) =

∞

−∞

xf(x)dx = const,

D[ξ(t)] =

∞

−∞

(x(t) − m

(t))

f(x)dx = const,

R(t

, t

) =

∞

−∞

(x(t

) − m

))(x(t

) − m

))

× f (x

, x

, t

)dx

= R(t

− t

) = R(τ).

The conditions m

= const, D[ξ(t)] = const and R(t

, t

) = R(t

− t

) = R(τ) are

the necessary conditions of the stationarity, but not the suﬃcient conditions, for

they can be carried out, but, beginning at some n, the distribution law will cease to

satisfy to a condition f(x

, . . . , x

, . . . , t

) = f (x

, . . . , x

+ t

, . . . , t

+ t

so the random function ξ(t) will be non-stationary. However for the solution of

practical problems frequently it is restricted to the application of the correlation

theory, therefore A.Ya. Khinchin has entered concept the stationarity in wide sense.

The random function is called stationary in the wide sense, if its mathematical

expectation and variance are constant, and the correlation function depends only

on a diﬀerence of instants, for which the ordinates of a random function are taken.

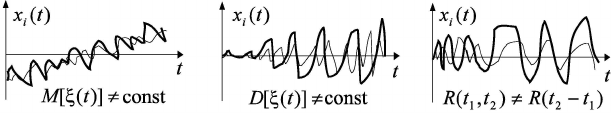
The examples non-stationary in the wide sense of random functions are submitted

on Fig. 1.45.

Fig. 1.45 Examples of non-stationary processes.

The normal distribution law is used most frequently at the study of the random

functions. The total characteristic of the normal random process are the mathe-

matical expectation and correlation function. The values of ordinates of a normal

random function in arbitrary instants t

, t

, . . . , t

are completely described by the

mathematical expectation and covariance matrix

= R(t

− t

If the probability properties of a random function in the subsequent time interval

are completely determined by the value of ordinate of this function in a given instant

76 STATISTICAL METHODS OF GEOPHYSICAL DATA PROCESSING

and do not depend on its values at the previous moments, such random function is

called Markovian, otherwise the random function is called non-Markovian.

The stationary random process ξ(t) satisﬁes to the ergodic hypothesis, if with

the probability 1 the time average is equal to the assembly average (on a set of

observations)

P [hξ(t)i

= M [ξ(t)]] = 1,

always supposing that these averages exist. The application of an ergodic hypothesis

enables to spot the statistical properties of the process on one member function,

that is very important for the practical applications.

1.10.2 Properties of the correlation function

(1) Autocorrelation function is an even function:

R(τ) = R(−τ).

(2) Ordinate of a real autocorrelation function does not exceed a variance of a

random function:

R(0) ≥ R(τ).

(3) Multiplication of [ξ(t) −m

(t)] on real function z(t) gives a following inequality

z(t

)z(t

)R(t

− t

)dt

≥ 0.

At suﬃciently great value of an interval τ = t

− t

the diversion of an ordi-

nate of a random function from its mathematical expectation in an instant t

becomes practically independent from the value of this deviation in an instant

. In this case the function R(τ ), giving value of connection between ξ(t

) and

ξ(t

), at τ → ∞ will tend to zero. Most frequently used approximations of

autocorrelation functions are submitted in a Fig. 1.46.

(4) The adding to a random function of a nonrandom quantity or a nonrandom

function does not change a value of the correlation function. Let us

η(t) = ξ(t) + ϕ(t),

where ϕ(t) is a nonrandom function. Taking into account

(t) = m

(t) + ϕ(t),

we obtain

, t

) = M[(η(t

) − m

)) × (η(t

) − m

))]

= M[(ξ(t

) − m

))(ξ(t

) − m

))] = R

, t

Basic concepts of the probability theory 77

Fig. 1.46 Examples of approximating functions for the autocorrelation function (σ = 0.5, α = 1.0,

β = 4.0).

1.10.3 Action of the linear operator on a random function

An linear homogeneous operator L is called the operator which preserves linear

operations

(1) L[cξ(t)] = cL[ξ(t)], where c is a constant.

(2) L[ξ

(t) + ξ

(t)] = Lξ

(t) + Lξ

(t).

Let us ξ(t) is a random function with the mathematical expectation m

(t) and

the correlation function R

, t

). Let us η(t) is one more correlation function

η(t) = L[ξ(t)].

To ﬁnd the mathematical expectation

(t) = M[L[ξ(t)]] = L[M[ξ(t)]] = Lm

(t)

and the correlation function

, t

) = M[(η(t

) − m

))(η(t

) − m

))]

= M{(L[ξ(t

)] − L[m

)])(L[ξ(t

)] − L[m

)])}

= L

M[(ξ(t

) − m

))(ξ(t

) − m

))]

= L

, t

1.10.4 Cross correlation function

Let us consider a system of two random functions ξ(t), η(t) and to deﬁne a cross

correlation function as following

ξη

, t

) = M[(ξ(t

) − m

))(η(t

) − m

))].

78 STATISTICAL METHODS OF GEOPHYSICAL DATA PROCESSING

In a case of the real functions we obtain

ξη

, t

)= R

ηξ

, t

and for the stationary random functions:

ξη

(τ)= R

ηξ

(−τ).

According to the deﬁnition of the correlation function we can write

ξη

, t

) =

∞

−∞

(x(t

) − m

))(y(t

) − m

))

× f

ξη

(x, y/t

, t

)dxdy.

Using the Cauchy-Bunyakovskii inequality we can write





∞

−∞

(x(t

) − m

))(y(t

) − m

))f(x, y/t

, t

)dxdy





≤

∞

−∞

(x(t

) − m

))

f(x, y/t

, t

)dxdy

∞

−∞

(y(t

) − m

))

f(x, y/t

, t

)dxdy,

whence follows

ξη

, t

)] ≤

, t

and

ξη

(τ) ≤

for the stationary case. Thus, for non-dimensional cross correlation function

ξη

, t

) =

ξη

, t

)

, t

)

, t

)

the following inequalities are carried out

−1 ≤ r

ξη

, t

) ≤ 1.

1.10.5 Wiener–Khinchin theorem and power spectrum

In the most of applications the function ξ(t), describing the stationary random

process, is a real function with the following properties:

Basic concepts of the probability theory 79

(1) There are the ﬁnite values of quantities

h|ξ|i

= lim

T →∞

(1/2T )

−T

|x(t)|dt

and

h|ξ|

= lim

T →∞

(1/2T )

−T

|x(t)|

dt.

A quantity h|ξ|

is called the average power.

(2) In each ﬁnite interval the function ξ(t) is a function with a constrained variation.

The correlation function (with time dependence) for a real random function ξ(t)

is deﬁned by the formula

(τ) = lim

T →∞

−T

ξ(t)ξ(t + τ)dt.

This limit exists always, when exists h|ξ|

. The function R

(τ) is real at real ξ(t).

For an ergodic random processes the function R

(τ) with probability 1 coincides

with the correlation function R

(τ) on a set of observations.

The cross correlation function (with time dependence) for two real functions

ξ(t), η(t) is deﬁned by the formula

ξη

(τ) = lim

T →∞

−T

ξ(t)η(t + τ )dt.

This limit exists always, when exists h|ξ|

, h|η|

. The function R

ξη

(τ) is real

at real ξ(t), η(t). A spectral density R

(ω) for a function ξ(t) and a cross spectral

density R

ξη

(ω) for a pair of functions ξ(t) and η(t) are determined with the help of

Wiener–Khinchin relations (the Wiener–Khinchin theorem):

(ω) =

∞

−∞

(τ) exp{−iωτ}dτ = 2

∞

(τ) cos ωτdτ,

(τ) =

2π

∞

−∞

(ω) exp{iωτ }dω =

∞

(ω) cos ωτdω,

ξη

(ω) =

∞

−∞

ξη

(τ) exp{−iωτ}dτ,

ξη

(τ) =

2π

∞

−∞

ξη

(ω) exp{iωτ}dω,

80 STATISTICAL METHODS OF GEOPHYSICAL DATA PROCESSING

where both integrals with cosine in the right hand sides of two ﬁrst relations are

applicable only in case of real R

(τ). For the real process ξ(t) the spectral density

(ω) is an even function

(ω) = R

(−ω).

Assuming τ = 0, it is possible to get an expression for a variance of the process

through the spectral density

= R

(0) =

2π

∞

−∞

(ω)dω.

Let’s consider three examples of an evaluation of the spectral density.

Example 1.21. Let the correlation function is approximated by the expression (see

Fig. 1.47)

(τ) = σ

exp{−α|τ|}, (1.81)

Then its spectrum is given by the expression

(ω) = σ

∞

−∞

exp{−iωτ − α|τ |}dτ

= σ

∞

exp{−(iω + α)τ}dτ +

−∞

exp{(α − iω)τ}dτ

= σ



α + iω

α − iω



= 2σ

+ ω

. (1.82)

Fig. 1.47 Graphic representation: (a) for correlation function (1.81), (b) for spectrum of the

correlation function (1.82), (σ = 2, α = 1).

Example 1.22. The correlation function reads as

(τ) = σ

exp{−α|τ|}cos ω

τ.

Taking into account that

cos ω

τ =

(exp{iω

τ} + exp{−iω

τ}),

Basic concepts of the probability theory 81

let evaluate its spectral density:

(ω) =

∞

−∞

exp{−iωτ −α|τ| + iω

τ}dτ

∞

−∞

exp{−iωτ −α|τ| −iω

τ}dτ

= σ

(ω − ω

)

+ α

(ω + ω

)

+ α



Example 1.23. The correlation function is given by the formula

(τ) = σ

exp{−α

}cos ω

τ.

Expressing cos ω

τ through the exponentials, we shall have for the spectral density

(ω) =

∞

−∞

exp{−α

+ iω

τ − iωτ}dτ

∞

−∞

exp{−α

− iω

τ − iωτ}dτ

Each of integrals can be reduced to the Poisson integral, if an exponent in the

integrand results in the form



ατ + i

2α

∓ i

2α



4α

(ω ± ω

)

Evaluating these integrals we shall obtain

(ω) =

√

2α



exp



−(ω + ω

)

4α



+ exp



−(ω − ω

)

4α



1.10.6 Deﬁnition of estimations of the characteristics of random

variables

Let a realization of a random process is registered in the digital form with a step of

discreteness ∆t — x(t

), j = 1, . . . , m. The estimate of the mathematical expecta-

tion is determined by the formula

ˆm

) =

i=1

An estimate for the correlation function is given by the formula

, t

) =

n − 1

i=1

[(x

) − ˆm

))(x

) − ˆm

))],

82 STATISTICAL METHODS OF GEOPHYSICAL DATA PROCESSING

, t

) =

n − 1

i=1

) −

n − 1

In a case of a stationary ergodic random processes an estimate of the mathe-

matical expectation is written as:

ˆm

j=1

x(t

or, change over integral, we shall obtain

ˆm

x(t)dt.

For the autocorrelation function the estimates in the discrete and integral forms,

taking into account the accepted assumptions, can be written accordingly as follows:

(τ) =

m − l − 1

m−l

j=1

(x(t

) − ˆm

)(x(t

+ τ) − ˆm

where τ = ∆tl = t

, and

(τ) =

T − τ

T −τ

[x(t) − ˆm

][x(t + τ ) − ˆm

]dt.