Tarek Ahmed. Reservoir engineering handbook

Подождите немного. Документ загружается.

258 Reservoir Engineering Handbook

0.01 0.05 0.1 0.2 0.5 1 2 5 10 20 30 40 50

60 70 80 90 95 98 99 99.8 99.9 99.99

0.010.050.10.20.525102030406070809095989999.9899.999.99 1

Figure 4-29. Probability-log scale.

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 258

Solution

Step 1. Arrange the entire permeability data in a descending order and

calculate % of thickness with greater permeability as shown

below:

md ft h with greater k % of h with greater k

435 2 0 0

254 2 2 3.6

196 2 4 7.1

172 3 6 10.7

166 2 9 16.1

158 2 11 19.6

153 2 13 23.2

147 2 15 26.8

128 1 17 30.4

105 2 18 32.1

100 1 20 35.7

91 2.5 21 37.5

90 2.5 23.5 42.0

83 2 26 46.4

72 1.5 28 50

62 2 29.5 52.7

49 6.5 31.5 56.3

44 2 38 67.9

40 2 40 71.4

35 2 42 75.0

32 2 44 78.6

30 2 46 82.1

28 2 48 85.7

27 2 50 89.3

20 2 52 92.9

9 2 54 96.4

Total = 56¢

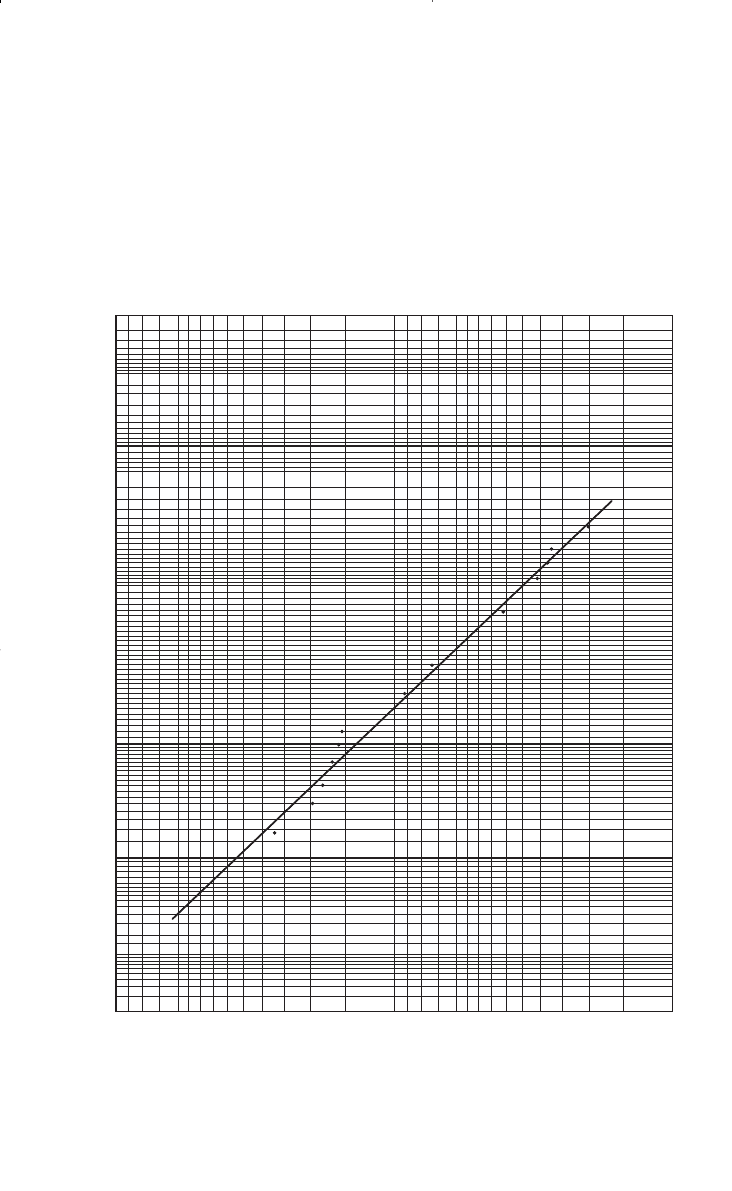
Step 2. Plot the permeability versus % of thickness with greater k on a

log-probability scale as shown in Figure 4-30 and read

= 68 md

84.1

= 29.5

Fundamentals of Rock Properties 259

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 259

260 Reservoir Engineering Handbook

0.01 0.05 0.1 0.2 0.5 1 2 5 10 20 30 40 50

60 70 80 90 95 98 99 99.8 99.9 99.99

0.010.050.10.20.525102030406070809095989999.899.999.99 1

% of Thickness with Greater k

Permeability, k

Figure 4-30. % of h vs. k.

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 260

Step 3. Calculate V by applying Equation 4-70.

It should be noted that if all the permeabilities are equal, the numerator

or Equation 4-70 would be zero, and the V would also be zero. This

would be the case for a completely homogeneous system. The Dykstra-

Parsons method is commonly referred to as a Permeability Ordering

Technique.

In water flooding calculations, it is frequently desired to divide the

reservoir into layers that have equal thickness and different permeability.

The log-probability scale can be used in this case to assign the perme-

ability scale into equal percent increments and to read the corresponding

permeability at the midpoint of each interval.

Example 4-19

Using the data given in Example 4-18, determine the average layer

permeability for a 10-layered system, assuming a uniform porosity.

Solution

Using the Dykstra-Parsons’s log-probability plot as shown in Figure 4-

30, determine the permeability for the 10-layered system as follows:

Layer % Probability k, md

1 5 265

2 15 160

3 25 120

435 94

545 76

655 60

765 49

875 39

985 29

10 95 18

Although permeability and porosity are not related in a strict technical

sense, they should correlate in rock of similar lithology and pore size distri-

V =

68 29 5

057

Fundamentals of Rock Properties 261

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 261

bution. In many cases, the logarithm of permeability versus porosity plots

is frequently made and the best straight line is drawn through the points.

Lorenz Coefficient L

Schmalz and Rahme (1950) introduced a single parameter that

describes the degree of heterogeneity within a pay zone section. The term

is called Lorenz coefficient and varies between zero, for a completely

homogeneous system, to one for a completely heterogeneous system.

The following steps summarize the methodology of calculating Lorenz

coefficient:

Step 1. Arrange all the available permeability values in a descending

order.

Step 2. Calculate the cumulative permeability capacity Skh and cumula-

tive volume capacity Sfh.

Step 3. Normalize both cumulative capacities such that each cumulative

capacity ranges from 0 to 1.

Step 4. Plot the normalized cumulative permeability capacity versus the

normalized cumulative volume capacity on a Cartesian scale.

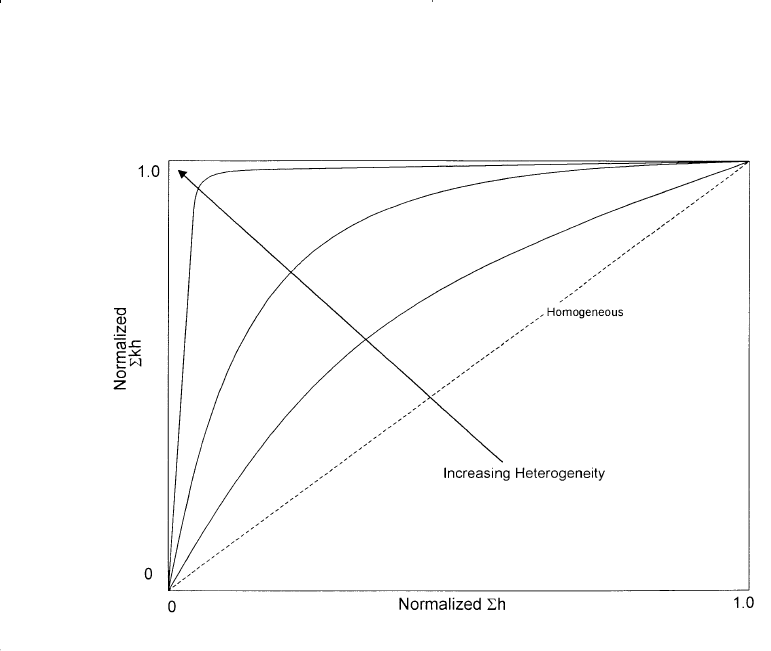
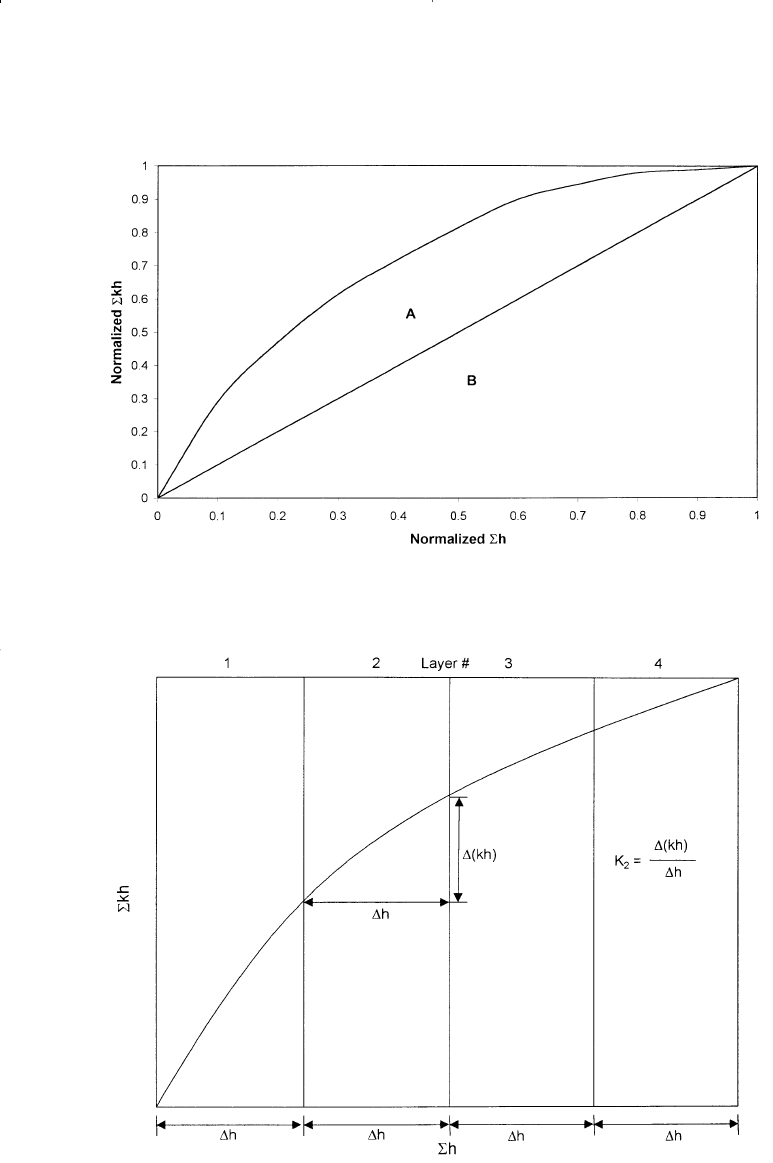
Figure 4-31 shows an illustration of the flow capacity distribution. A

completely uniform system would have all permeabilities equal, and a

plot of the normalized Skh versus Sfh would be a straight line. Figure

4-31 indicates that as the degree of contrast between high and low values

of permeability increases the plot exhibits greater concavity toward the

upper left corner. This would indicate more heterogeneity, i.e., the severi-

ty of deviation from a straight line is an indication of the degree of het-

erogeneity. The plot can be used to describe the reservoir heterogeneity

quantitatively by calculating the Lorenz coefficient. The coefficient is

defined by the following expression:

Area above the straight line

Area below the straight line

= (4 - 71)

262 Reservoir Engineering Handbook

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 262

where the Lorenz coefficient L can vary between 0 and 1.

0 = completely homogeneous

1 = completely heterogeneous

Figure 4-32 shows the relation of the permeability variation V and

Lorenz coefficient L for log-normal permeability distributions as pro-

posed by Warren and Price (1961). This relationship can be expressed

mathematically by the following two expressions:

Lorenz coefficient in terms of permeability variation:

L = 0.0116356 + 0.339794V + 1.066405V

- 0.3852407V

(4-72)

Permeability variation in terms of Lorenz coefficient:

V =-5.05971(10

-4

) + 1.747525L - 1.468855 L

+ 0.701023 L

(4-73)

Fundamentals of Rock Properties 263

Figure 4-31. Normalized flow capacity.

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 263

The above two expressions are applicable between 0 < L < 1 and 0 <

V < 1.

Example 4-20

Using the data given in Example 4-18, calculate the Lorenz coefficient

assuming a uniform porosity.

Solution

Step 1. Tabulate the permeability data in a descending order and calculate

the normalized Skh and Sh as shown below:

264 Reservoir Engineering Handbook

.2 .4 .6 .8 1.0

1.0

Variation, V

Lorenz Coefficient

Figure 4-32. Correlation of Lorenz coefficient and permeability variation.

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 264

k, md h, ft kh Skh Skh/5646.5 Sh Sh/56

435 2 870 870 0.154 2 0.036

254 2 508 1378 0.244 4 0.071

196 2 392 1770 0.313 6 0.107

172 3 516 2286 0.405 9 0.161

166 2 332 2618 0.464 11 0.196

158 2 316 2934 0.520 13 0.232

153 2 306 3240 0.574 15 0.268

147 2 294 3534 0.626 17 0.304

128 1 128 3662 0.649 18 0.321

105 2 210 3872 0.686 20 0.357

100 1 100 3972 0.703 21 0.375

91 2.5 227.5 4199.5 0.744 23.5 0.420

90 2.5 225 4424.5 0.784 26 0.464

83 2 166 4590.5 0.813 28 0.50

72 1.5 108 4698.5 0.832 29.5 0.527

62 2 124 4822.5 0.854 31.5 0.563

49 6.5 294 5116.5 0.906 38.0 0.679

44 2 88 5204.5 0.922 40.0 0.714

40 2 80 5284.5 0.936 42 0.750

35 2 70 5354.4 0.948 44 0.786

32 2 64 5418.5 0.960 46 0.821

30 2 60 5478.5 0.970 48 0.857

28 2 56 5534.5 0.980 50 0.893

27 2 54 5588.5 0.990 52 0.929

20 2 40 5628.5 0.997 54 0.964

9 2 18 5646.5 1.000 56 1.000

Step 2. Plot the normalized capacities on a Cartesian scale as shown in

Figure 4-33.

Step 3. Calculate the Lorenz coefficient by dividing the area above the

straight line (area A) by the area under the straight line (area B)

to give:

L = 0.42

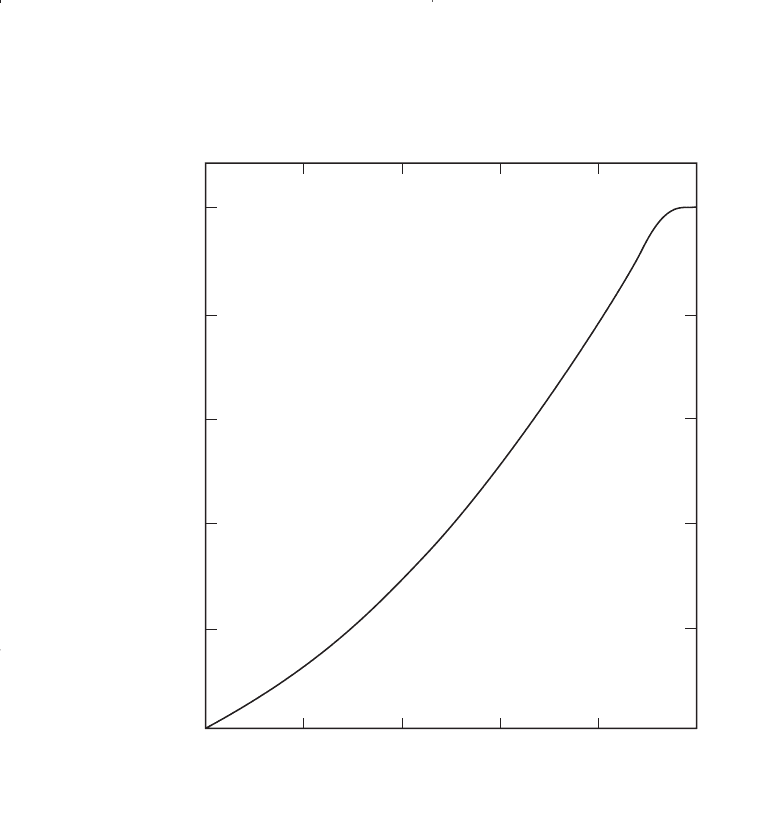
A plot of the cumulative permeability capacity Skh versus Sh (without

normalization) is commonly constructed, as shown in Figure 4-34, and

used to assign average permeability values for a selected number of

reservoir layers. If the intervals of the thickness are chosen, as shown in

Figure 4-34, then the average values of permeability for each thickness

interval (layer) can be calculated by dividing the incremental (kh) by the

incremental thickness.

Fundamentals of Rock Properties 265

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 265

266 Reservoir Engineering Handbook

Figure 4-33. Normalized flow capacity for Example 4-20.

Figure 4-34. Cumulative permeability capacity vs. cumulative thickness.

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 266

It should be noted that it is not necessary that equal thickness sections

be chosen. They may be selected at irregular increments as desired.

There are also some advantages of selecting layer properties so that each

layer has the same permeability thickness product.

Example 4-21

Using the data given in Example 4-18, calculate the average perme-

ability for a 10-layered system reservoir. Compare the results with those

of the Dykstra-Parsons method.

Solution

Step 1. Using the calculated values of Skh and Sh of Example 4-20, plot

Skh versus Sh on a Cartesian coordinate as shown in Figure 4-35.

Step 2. Divide the x-axis into 10 equal segments*, each with 5.6 ft.

Step 3. Calculate the average permeability k

–

for each interval, to give:

Layer k

–

from Dykstra-Parsons, Example 4-19

1 289 265

2 196.4 160

3 142.9 120

4 107.1 94

5 83.9 76

6 67.9 60

7 44.6 49

8 35.7 39

9 32.1 29

10 17.2 18

The permeability sequencing (ordering) methods of zonation do not

consider the physical location of the rocks with the vertical column. All

the data are considered to be a statistical sampling, which will describe

the statistical distribution of permeability, porosity, and thickness within

the reservoir. All the values of equal permeability are presumed to be in

communication with each other.

Fundamentals of Rock Properties 267

*It should be noted that the 56 feet do not equal the reservoir net thickness. It essentially

represents the cumulative thickness of the core samples.

Reservoir Eng Hndbk Ch 04b 2001-10-24 09:46 Page 267