Tarek Ahmed. Reservoir engineering handbook

Подождите немного. Документ загружается.

The gas viscosity is not commonly measured in the laboratory because

it can be estimated precisely from empirical correlations. Like all inten-

sive properties, viscosity of a natural gas is completely described by the

following function:

= (p,T,y

)

where m

= the viscosity of the gas phase. The above relationship simply

states that the viscosity is a function of pressure, temperature, and com-

position. Many of the widely used gas viscosity correlations may be

viewed as modifications of that expression.

METHODS OF CALCULATING THE VISCOSITY OF

NATURAL GASES

Two popular methods that are commonly used in the petroleum indus-

try are the:

• Carr-Kobayashi-Burrows Correlation Method

• Lee-Gonzalez-Eakin Method

The Carr-Kobayashi-Burrows Correlation Method

Carr, Kobayashi, and Burrows (1954) developed graphical correlations

for estimating the viscosity of natural gas as a function of temperature,

pressure, and gas gravity. The computational procedure of applying the

proposed correlations is summarized in the following steps:

Step 1. Calculate the pseudo-critical pressure, pseudo-critical tempera-

ture, and apparent molecular weight from the specific gravity or

the composition of the natural gas. Corrections to these pseudo-

critical properties for the presence of the nonhydrocarbon gases

(CO

, N

, and H

S) should be made if they are present in concen-

trations greater than 5 mole percent.

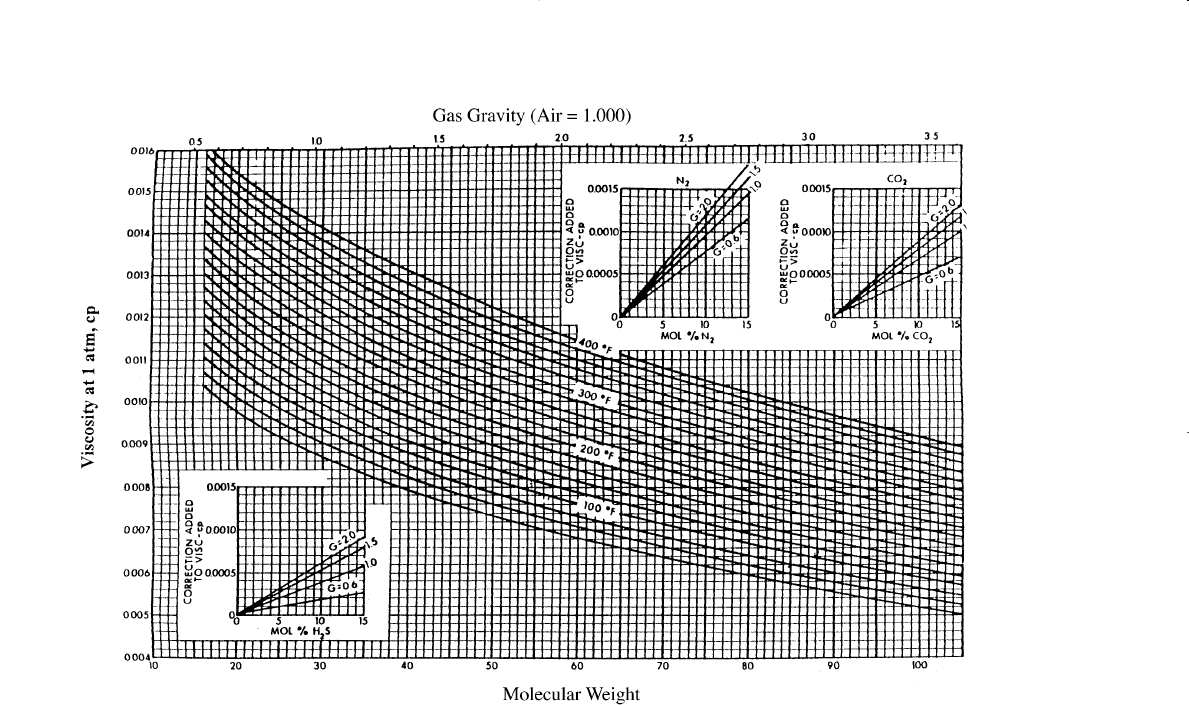
Step 2. Obtain the viscosity of the natural gas at one atmosphere and the

temperature of interest from Figure 2-5. This viscosity, as denoted

by m

, must be corrected for the presence of nonhydrocarbon

components by using the inserts of Figure 2-5. The nonhydrocar-

bon fractions tend to increase the viscosity of the gas phase. The

effect of nonhydrocarbon components on the viscosity of the nat-

68 Reservoir Engineering Handbook

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 68

Reservoir-Fluid Properties 69

Figure 2-5. Carr’s atmospheric gas viscosity correlation. (Permission to publish by the Society of Petroleum Engineers of AIME. Copy-

right SPE-AIME.)

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 69

ural gas can be expressed mathematically by the following rela-

tionships:

= (m

)

uncorrected

+ (Dm)

(2-57)

where m

= “corrected” gas viscosity at one atmospheric

pressure and reservoir temperature, cp

(Dm)

= viscosity corrections due to the presence of N

(Dm)

= viscosity corrections due to the presence of CO

(Dm)

= viscosity corrections due to the presence of H

)

uncorrected

= uncorrected gas viscosity, cp

Step 3. Calculate the pseudo-reduced pressure and temperature.

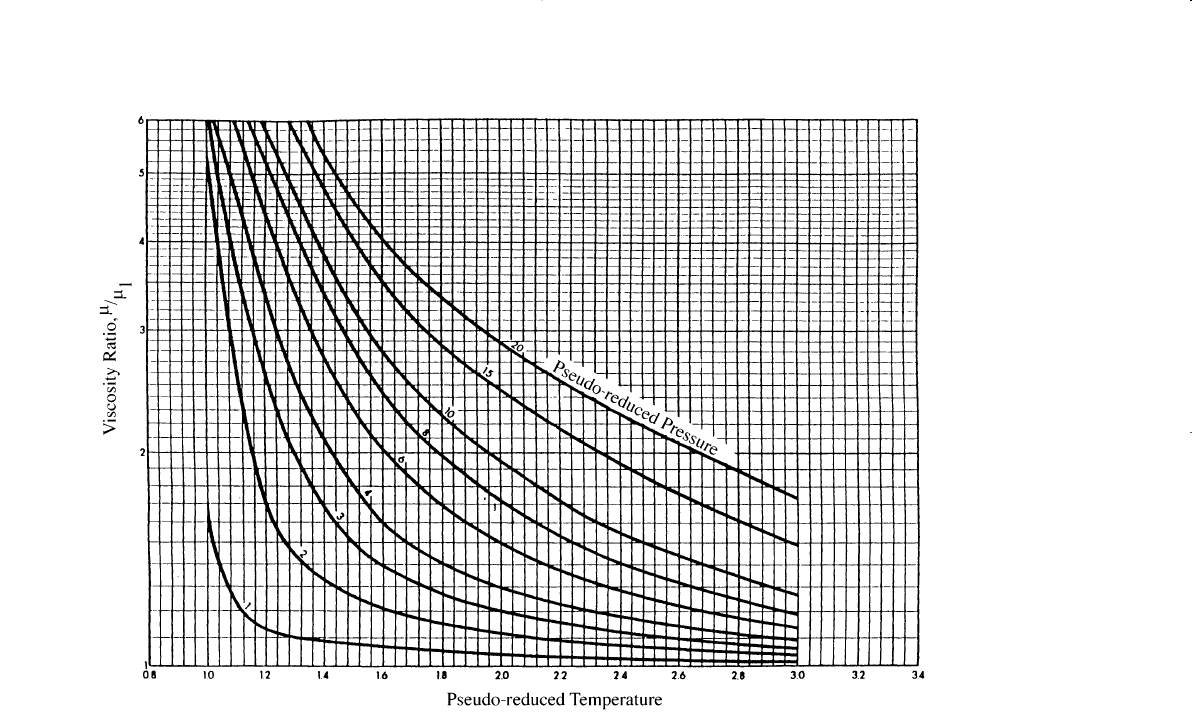
Step 4. From the pseudo-reduced temperature and pressure, obtain the

viscosity ratio (m

) from Figure 2-6. The term m

represents the

viscosity of the gas at the required conditions.

Step 5. The gas viscosity, m

, at the pressure and temperature of interest

is calculated by multiplying the viscosity at one atmosphere and

system temperature, m

, by the viscosity ratio.

The following examples illustrate the use of the proposed graphical

correlations:

Example 2-13

Using the data given in Example 2-12, calculate the viscosity of the gas.

Solution

Step 1. Calculate the apparent molecular weight of the gas:

= (0.72) (28.96) = 20.85

Step 2. Determine the viscosity of the gas at 1 atm and 140°F from Fig-

ure 2-5:

= 0.0113

70 Reservoir Engineering Handbook

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 70

Reservoir-Fluid Properties 71

Figure 2-6. Carr’s viscosity ratio correlation. (Permission to publish by the Society of Petroleum Engineers of AIME. Copyright SPE-

AIME.)

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 71

Step 3. Calculate p

and T

= 2.99

= 1.52

Step 4. Determine the viscosity rates from Figure 2-6:

Step 5. Solve for the viscosity of the natural gas:

Standing (1977) proposed a convenient mathematical expression for cal-

culating the viscosity of the natural gas at atmospheric pressure and reser-

voir temperature, m

. Standing also presented equations for describing the

effects of N

, CO

, and H

S on m

. The proposed relationships are:

where:

where m

= viscosity of the gas at atmospheric pressure and

reservoir temperature, cp

T = reservoir temperature, °R

= gas gravity

, y

= mole fraction of N

, CO

, and H

S, respectively

Dempsey (1965) expressed the viscosity ratio m

by the following

relationship:

()

[

8.49

(

)

( ) + 3.73

(

)

]

log

(2 - 61)

( ) [ . ( )log( ) . ( )]Dm g

NN g

8 48 10 9 59 10=+

(2 - 60)

()

Dm =

(

)

(

)

[]

908 10

624 10

log

uncorrected

()

= [1.709 (

2.062

] (T 460)

+ 8.118 ( ) 6.15 (

) ( )

10 log (2 - 59)

uncorrected

CO H S N

()

() ()

(2 - 58)

222

mmm

DDD

= ( ) = (1.5) (0.0113) = 0.01695 cp

= 1.5

72 Reservoir Engineering Handbook

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 72

where T

= pseudo-reduced temperature of the gas mixture, °R

= pseudo-reduced pressure of the gas mixture, psia

. . . a

= coefficients of the equations are given below:

=-2.46211820 a

=-7.93385648 (10

-1

)

= 2.970547414 a

= 1.39643306

=-2.86264054 (10

-1

=-1.49144925 (10

-1

)

= 8.05420522 (10

-3

= 4.41015512 (10

-3

)

= 2.80860949 a

= 8.39387178 (10

-2

)

=-3.49803305 a

=-1.86408848 (10

-1

)

= 3.60373020 (10

-1

= 2.03367881 (10

-2

)

=-1.044324 (10

-2

=-6.09579263 (10

-4

)

The Lee-Gonzalez-Eakin Method

Lee, Gonzalez, and Eakin (1966) presented a semi-empirical relation-

ship for calculating the viscosity of natural gases. The authors expressed

the gas viscosity in terms of the reservoir temperature, gas density, and

the molecular weight of the gas. Their proposed equation is given by:

where

Y = 2.4 - 0.2 X (2 - 66)

X = 3.5 +

986

+ 0.01 M

(2 - 65)

(9.4 + 0.02 M ) T

209 + 19 M + T

15.

(2 - 64)

=KX

62 4

exp

(2 - 63)

(

pr pr

12 13

=a +a

(a + a p

(

)

01 2 4

(2(2 - 62)

Reservoir-Fluid Properties 73

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 73

= gas density at reservoir pressure and temperature, lb/ft

T = reservoir temperature, °R

= apparent molecular weight of the gas mixture

The proposed correlation can predict viscosity values with a standard

deviation of 2.7% and a maximum deviation of 8.99%. The correlation is

less accurate for gases with higher specific gravities. The authors pointed

out that the method cannot be used for sour gases.

Example 2-14

Rework Example 2-13 and calculate the gas viscosity by using the

Lee-Gonzalez-Eakin method.

Step 1. Calculate the gas density from Equation 2-16:

Step 2. Solve for the parameters K, X, and Y by using Equations 2-64,

2-65, and 2-66, respectively:

Step 3. Calculate the viscosity from Equation 2-63:

PROPERTIES OF CRUDE OIL SYSTEMS

Petroleum (an equivalent term is crude oil) is a complex mixture con-

sisting predominantly of hydrocarbons and containing sulfur, nitrogen,

oxygen, and helium as minor constituents. The physical and chemical

properties of crude oils vary considerably and are dependent on the con-

1.33

= (119.72) 5.35

8.3

62.4

= 0.0173 cp

exp

[9.4 + 0.02 (20.85)]

(600)

209 + 19 (20.85) + 600

= 119.72

X = 3.5 +

986

600

+ 0.01 (20.85) = 5.35

Y = 2.4 0.2 (5.35) = 1.33

1.5

(2000) (20.85)

(10.73) (600) (0.78)

= 8.3 lb/ft

74 Reservoir Engineering Handbook

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 74

centration of the various types of hydrocarbons and minor constituents

present.

An accurate description of physical properties of crude oils is of a con-

siderable importance in the fields of both applied and theoretical science

and especially in the solution of petroleum reservoir engineering prob-

lems. Physical properties of primary interest in petroleum engineering

studies include:

• Fluid gravity

• Specific gravity of the solution gas

• Gas solubility

• Bubble-point pressure

• Oil formation volume factor

• Isothermal compressibility coefficient of undersaturated crude oils

• Oil density

• Total formation volume factor

• Crude oil viscosity

• Surface tension

Data on most of these fluid properties are usually determined by labo-

ratory experiments performed on samples of actual reservoir fluids. In

the absence of experimentally measured properties of crude oils, it is

necessary for the petroleum engineer to determine the properties from

empirically derived correlations.

Crude Oil Gravity

The crude oil density is defined as the mass of a unit volume of the

crude at a specified pressure and temperature. It is usually expressed in

pounds per cubic foot. The specific gravity of a crude oil is defined as the

ratio of the density of the oil to that of water. Both densities are measured

at 60°F and atmospheric pressure:

where g

= specific gravity of the oil

= density of the crude oil, lb/ft

= density of the water, lb/ft

= (2 - 67)

Reservoir-Fluid Properties 75

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 75

It should be pointed out that the liquid specific gravity is dimension-

less, but traditionally is given the units 60°/60° to emphasize the fact that

both densities are measured at standard conditions. The density of the

water is approximately 62.4 lb/ft

, or:

Although the density and specific gravity are used extensively in the

petroleum industry, the API gravity is the preferred gravity scale. This

gravity scale is precisely related to the specific gravity by the following

expression:

The API gravities of crude oils usually range from 47° API for the

lighter crude oils to 10° API for the heavier asphaltic crude oils.

Example 2-15

Calculate the specific gravity and the API gravity of a crude oil system

with a measured density of 53 lb/ft

at standard conditions.

Solution

Step 1. Calculate the specific gravity from Equation 2-67:

Step 2. Solve for the API gravity:

Specific Gravity of the Solution Gas

The specific gravity of the solution gas g

is described by the weighted

average of the specific gravities of the separated gas from each separator.

API = = API

141 5

0 849

131 5 35 2

..-∞

62 4

0 849

∞-API =

141 5

131 5

(2 - 68)

62 4

60 60

,/∞∞

76 Reservoir Engineering Handbook

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 76

This weighted-average approach is based on the separator gas-oil ratio,

or:

where n = number of separators

sep

= separator gas-oil ratio, scf/STB

sep

= separator gas gravity

= gas-oil ratio from the stock tank, scf/ STB

= gas gravity from the stock tank

Example 2-16

Separator tests were conducted on a crude oil sample. Results of the

test in terms of the separator gas-oil ration and specific gravity of the

separated gas are given below:

Separator Pressure Temperature Gas-Oil Ratio Gas Specific

# psig °F scf/STB Gravity

Primary 660 150 724 0.743

Intermediate 75 110 202 0.956

Stock tank 0 60 58 1.296

Calculate the specific gravity of the separated gas.

Solution

Estimate the specific gravity of the solution by using Equation 2-69:

Gas Solubility

The gas solubility R

is defined as the number of standard cubic feet of

gas which will dissolve in one stock-tank barrel of crude oil at certain

()(.)()(.)()(.)

724 0 743 202 0 956 58 1 296

724 202 58

0 819

sep i sep i st st

sep i st

()()

()

(2 - 69)

Reservoir-Fluid Properties 77

Reservoir Eng Hndbk Ch 02a 2001-10-24 09:23 Page 77