Stone M., Goldbart P. Mathematics for Physics: A Guided Tour for Graduate Students
Подождите немного. Документ загружается.

8.3 Bessel functions 285
60 40 20 0 20 40 60
60
40
20
0
20
40
60
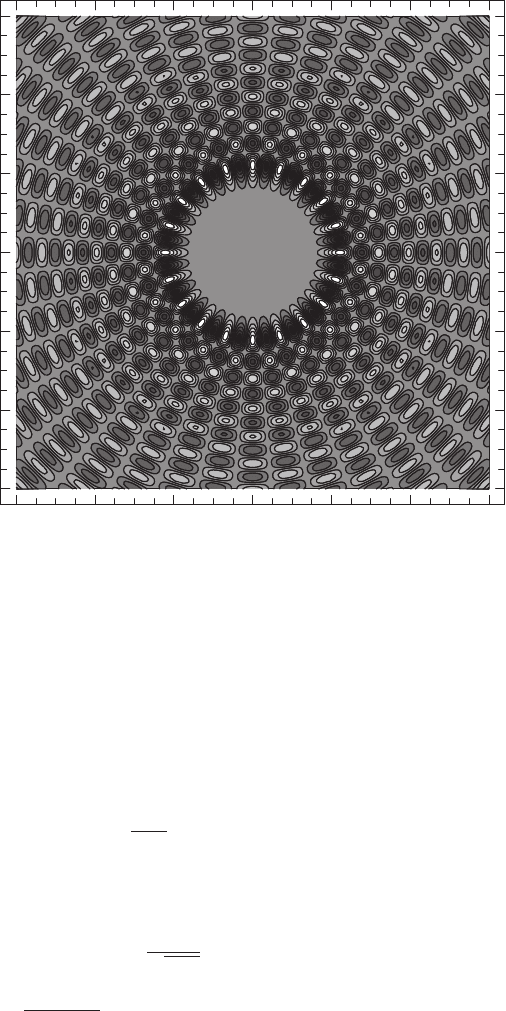
Figure 8.12 The hole appearing in Figure 8.11 is visible in the real part of ψ
k,20
(rθ) =
e
i20θ
J
20
(kr).
is proportional to |ψ|
2
,soψ itself decreases as r
−1/2
. In contrast to the classical particle
density, the quantum mechanical wavefunction amplitude remains finite at the caustic –
the “geometric optics” infinity being tempered by diffraction effects.
Exercise 8.3: The WKB (Wentzel–Kramers–Brillouin) approximation to a solution of
the Schrödinger equation
−
d
2
ψ
dx
2
+ V (x)ψ (x) = Eψ(x)
sets
ψ(x) ≈
1
√
κ(x)
exp
±i
x
a
κ(ξ)dξ
,
where κ(x) =
√
E − V (x), and a is some conveniently chosen constant. This form of
the approximation is valid in classically allowed regions, where κ is real, and away
from “turning points” where κ goes to zero. In a classically forbidden region, where κ is
imaginary, the solutions should decay exponentially. The connection rule that matches
286 8 Special functions
the standing wave in the classically allowed region onto the decaying solution is
1
2
√
|κ(x)|
exp
−
!
!
!
!
x
a
κ(ξ)dξ
!
!
!
!
→
1
√
κ(x)
cos
!
!
!
!
x
a
κ(ξ)dξ
!
!
!
!
−
π
4
,
where a is the classical turning point. (The connection is safely made only in the direction
of the arrow. This is because a small error in the phase of the cosine will introduce a
small admixture of the growing solution, which will eventually swamp the decaying
solution.)
Show that setting y(r) = r
−1/2
ψ(r) in Bessel’s equation
−
d
2
y
dr
2
−
1
r
dy
dr
+
l
2
y
r
2
= k
2
y
reduces it to Schrödinger form
−
d
2
ψ
dr
2
+
(l
2
− 1/4)
r
2
ψ = k
2
ψ.
From this show that a WKB approximation to y(r) is
y (r) ≈
1
(r
2
− b
2
)
1/4
exp
±ik
r
b
ρ
2
− b
2
ρ
dρ
, r b
=
1
√
x(r)
exp{±i[kx(r) − lθ(r)]},
where kb =
l
2
− 1/4 ≈ l, and x(r) and θ(r) were defined in connection with (8.102).
Deduce that the expressions (8.102) are WKB approximations and are therefore accurate
once we are away from the classical turning point at r = b ≡ r
min
.
8.3.2 Orthogonality and completeness
We can write the equation obeyed by J
n
(kr) in Sturm–Liouville form. We have
1
r
d
dr
r
dy
dr
+
k
2
−
m
2
r
2
y = 0. (8.103)
Comparison with the standard Sturm–Liouville equation shows that the weight function,
w(r),isr, and the eigenvalues are k
2
.
From Lagrange’s identity we obtain
(k
2
1
− k
2
2
)
R
0
J
m
(k
1
r)J
m
(k
2
r)rdr
= R
k
2
J
m
(k
1
R)J
m
(k
2
R) − k
1
J
m
(k
2
R)J
m
(k
1
R)
. (8.104)

8.3 Bessel functions 287
We have no contribution from the origin on the right-hand side because all J
m
Bessel
functions except J
0
vanish there, whilst J
0
(0) = 0. For each m we get a set of orthog-
onal functions, J
m
(k
n
x), provided the k
n
R are chosen to be roots of J
m
(k
n
R) = 0or
J
m
(k
n
R) = 0.
We can find the normalization constants by differentiating (8.104) with respect to k
1
and then setting k
1
= k
2
in the result. We find
R
0
7
J
m
(kr)
8
2
rdr =
1
2
R
2
7
J
m
(kR)
8
2
+
1 −
m
2
k
2
R
2
7
J
m
(kR)
8
2
,
=
1
2
R
2
7
[J
n
(kR)]
2
− J
n−1
(kR)J
n+1
(kR)
8
. (8.105)
(The second equality follows on applying the recurrence relations for the J
n
(kr), and
provides an expression that is perhaps easier to remember.) For Dirichlet boundary
conditions we will require k
n
R to be a zero of J
m
, and so we have
R
0
7
J
m
(kr)
8
2
rdr =
1
2
R
2
7
J
m
(kR)
8
2
. (8.106)
For Neumann boundary conditions we require k
n
R to be a zero of J
m
. In this case
R
0
7
J
m
(kr)
8
2
rdr =
1
2
R
2
1 −
m
2
k
2
R
2
7
J
m
(kR)
8
2
. (8.107)
Example: Harmonic function in a cylinder. We wish to solve ∇
2
V = 0 within a cylinder
of height L and radius a (Figure 8.13). The voltage is prescribed on the upper surface
of the cylinder: V (r, θ, L) = U (r, θ). We are told that V = 0 on all other parts of the
boundary.
The general solution of Laplace’s equation will be a sum of terms such as
sinh(kz)
cosh(kz)
×
J
m
(kr)
N
m
(kr)
×
sin(mθ)
cos(mθ)
, (8.108)
z
r
L
a
Figure 8.13 Cylinder geometry.

288 8 Special functions
where the braces indicate a choice of upper or lower functions. We must take only the
sinh(kz) terms because we know that V = 0atz = 0, and only the J
m
(kr) terms because
V is finite at r = 0. The k’s are also restricted by the boundary condition on the sides of
the cylinder to be such that J
m
(ka) = 0. We therefore expand the prescribed voltage as
U (r, θ) =
m,n
sinh(k
nm
L)J
m
(k
mn
r)
[
A
nm
sin(mθ) + B
nm
cos(mθ)
]
, (8.109)
and use the orthonormality of the trigonometric and Bessel function to find the
coefficients to be
A
nm
=
2cosech(k
nm
L)
πa
2
[J
m
(k
nm
a)]
2
2π
0
dθ
a
0
U (r, θ)J
m
(k
nm
r) sin(mθ)rdr, (8.110)
B
nm
=
2cosech(k
nm
L)
πa
2
[J
m
(k
nm
a)]
2
2π
0
dθ
a
0
U (r, θ)J
m
(k
nm
r) cos(mθ)rdr, m = 0, (8.111)
and
B
n0
=
1
2
2cosech(k
n0
L)
πa
2
[J
0
(k
n0
a)]
2
2π
0
dθ
a
0
U (r, θ)J
0
(k
n0
r) rdr. (8.112)
Then we fit the boundary data expansion to the general solution, and so find
V (r, θ, z) =
m,n
sinh(k
nm
z)J
m
(k
mn
r)
[
A
nm
sin(mθ) + B
nm
cos(mθ)
]
. (8.113)
Hankel transforms
When the radius, R, of the region in which we are performing our eigenfunction expansion
becomes infinite, the eigenvalue spectrum will become continuous, and the sum over
the discrete k
n
Bessel-function zeros must be replaced by an integral over k. By using
the asymptotic approximation
J
n
(kR) ∼
<
2
πkR
cos
kR −
1
2
nπ −
1
4
π
, (8.114)
we may estimate the normalization integral as
R
0
7
J
m
(kr)
8
2
rdr ∼
R
πk
+ O(1). (8.115)
We also find that the asymptotic density of Bessel zeros is
dn
dk
=
R
π
. (8.116)

8.3 Bessel functions 289
Putting these two results together shows that the continuous-spectrum orthogonality and
completeness relations are
∞
0
J
n
(kr)J
n
(k
r) rdr =
1
k
δ(k − k
), (8.117)
∞
0
J
n
(kr)J
n
(kr
) kdk =
1
r
δ(r − r
), (8.118)
respectively. These two equations establish that the Hankel transform (also called the
Fourier–Bessel transform) of a function f (r), which is defined by
F(k) =
∞
0
J
n
(kr)f (r)rdr, (8.119)
has as its inverse
f (r) =
∞
0
J
n
(kr)F(k)kdk. (8.120)
(See exercise 8.14 for an alternative derivation of the Hankel-transform pair.)
Some Hankel transform pairs:
∞
0
e
−ar
J
0
(kr) dr =
1
√
k
2
+ a
2
,
∞
0
J
0
(kr)
√
k
2
+ a
2
kdk =
e
−ar
r
. (8.121)
∞
0
cos(ar)J
0
(kr) dr =
0, k < a,
1/
√
k
2
− a
2
, k > a.
∞
a
J
0
(kr)
√
k
2
− a
2
kdk =
1
r
cos(ar). (8.122)
∞
0
sin(ar)J
0
(kr) dr =
1/
√
a
2
− k
2
, k < a,
0, k > a.
a
0
J
0
(kr)
√
a
2
− k
2
kdk =
1
r
sin(ar). (8.123)
Example: Weber’s disc problem. Consider a thin isolated conducting disc of radius a
lying on the xy-plane in R
3
. The disc is held at potential V
0
. We seek the potential V in
the entirety of R
3
, such that V → 0 at infinity.
It is easiest to first find V in the half-space z ≥ 0, and then extend the solution to z < 0
by symmetry. Because the problem is axisymmetric, we will make use of cylindrical
290 8 Special functions
polar coordinates with their origin at the centre of the disc. In the region z ≥ 0 the
potential V (r, z) obeys
∇
2
V (r, z) = 0, z > 0,
V (r, z) → 0 |z|→∞,
V (r,0) = V
0
, r < a,
∂V
∂z
!
!
!
!
z=0
= 0, r > a. (8.124)
This is a mixed boundary value problem. We have imposed Dirichlet boundary conditions
on r < a and Neumann boundary conditions for r > a.
We expand the axisymmetric solution of Laplace’s equation in terms of Bessel
functions as
V (r, z) =
∞
0
A(k)e
−k|z |
J
0
(kr) dk, (8.125)
and so require the unknown coeffcient function A(k) to obey
∞
0
A(k)J
0
(kr) dk = V
0
, r < a
∞
0
kA(k)J
0
(kr) dk = 0, r > a. (8.126)
No elementary algorithm for solving such a pair of dual integral equations exists. In this
case, however, some inspired guesswork helps. By integrating the first equation of the
transform pair (8.122) with respect to a, we discover that
∞
0
sin(ar)
r
J
0
(kr) dr =
π/2, k < a,
sin
−1
(a/k), k > a.
(8.127)
With this result in hand, we then observe that (8.123) tells us that the function
A(k) =
2V
0
sin(ka)
πk
(8.128)
satisfies both equations. Thus
V (r, z) =
2V
0
π
∞
0
e
−k|z |
sin(ka)J
0
(kr)
dk
k
. (8.129)
The potential on the plane z = 0 can be evaluated explicitly to be
V (r,0) =
V
0
, r < a,
(2V
0
/π) sin
−1
(a/r), r > a.
(8.130)

8.3 Bessel functions 291
The charge distribution on the disc can also be found as
σ(r) =
∂V
∂z
!
!
!
!
z=0
−
−
∂V
∂z
!
!
!
!
z=0
+
=
4V
0
π
∞
0
sin(ak)J
0
dk
=
4V
0
π
√
a
2
− r
2
, r < a. (8.131)
8.3.3 Modified Bessel functions
When k is real the Bessel function J
n
(kr) and the Neumann N
n
(kr) function oscillate at
large distance. When k is purely imaginary, it is convenient to combine them so as to
have functions that grow or decay exponentially. These combinations are the modified
Bessel functions I
n
(kr) and K
n
(kr).
These functions are initially defined for non-integer ν by
I
ν
(x) = i
−ν
J
ν
(ix), (8.132)
K
ν
(x) =
π
2 sin νπ
[I
−ν
(x) − I
ν
(x)]. (8.133)
The factor of i
−ν
in the definition of I
ν
(x) is inserted to make I
ν
real. Our definition
of K
ν
(x) is that in Abramowitz and Stegun’s Handbook of Mathematical Functions.It
differs from that of Whittaker and Watson, who divide by tan νπ instead of sin νπ.
At short distance, and for ν>0,
I
ν
(x) =
x
2
ν
1
(ν + 1)
+···, (8.134)
K
ν
(x) =
1
2
(ν)
x
2
−ν
+··· (8.135)
When ν becomes an integer we must take limits, and in particular
I
0
(x) = 1 +
1
4
x
2
+···, (8.136)
K
0
(x) =−(ln x/2 + γ)+··· (8.137)
The large x asymptotic behaviour is
I
ν
(x) ∼
1
√
2πx
e
x
, x →∞, (8.138)
K
ν
(x) ∼
π
√
2x
e
−x
, x →∞. (8.139)
292 8 Special functions
From the expression for J
n
(x) as an integral, we have
I
n
(x) =
1
2π
2π
0
e
inθ
e
x cos θ
dθ =
1
π
π
0
cos(nθ)e
x cos θ
dθ (8.140)
for integer n. When n is not an integer we still have an expression for I
ν
(x) as an integral,
but now it is
I
ν
(x) =
1
π
π
0
cos(νθ)e
x cos θ
dθ −
sin νπ
π
∞
0
e
−x cosh t−νt
dt. (8.141)
Here we need |arg x| <π/2 for the second integral to converge. The origin of the “extra”
infinite integral must remain a mystery until we learn how to use complex integral
methods for solving differential equations. From the definition of K
ν
(x) in terms of I
ν
we find
K
ν
(x) =
∞
0
e
−x cosh t
cosh(νt) dt, |arg x| <π/2. (8.142)
Physics illustration: Light propagation in optical fibres. Consider the propagation of
light of frequency ω
0
down a straight section of optical fibre. Typical fibres are made
of two materials: an outer layer, or cladding, with refractive index n
2
, and an inner core
with refractive index n
1
> n
2
. The core of a fibre used for communication is usually
less than 10 µm in diameter.
We will treat the light field E as a scalar. (This is not a particularly good approximation
for real fibres, but the complications due to the vector character of the electromagnetic
field are considerable.) We suppose that E obeys
∂
2
E
∂x
2
+
∂
2
E
∂y
2
+
∂
2
E
∂z
2
−
n
2
(x, y)
c
2
∂
2
E
∂t
2
= 0. (8.143)
Here n(x , y) is the refractive index of the fibre, which is assumed to lie along the z-axis.
We set
E(x, y, z, t) = ψ(x, y, z)e
ik
0
z−iω
0
t
(8.144)
where k
0
= ω
0
/c. The amplitude ψ is a (relatively) slowly varying envelope function.
Plugging into the wave equation we find that
∂
2
ψ
∂x
2
+
∂
2
ψ
∂y
2
+
∂
2
ψ
∂z
2
+ 2ik
0
∂ψ
∂z
+
n
2
(x, y)
c
2
ω
2
0
− k
2
0
ψ = 0. (8.145)
Because ψ is slowly varying, we neglect the second derivative of ψ with respect to z,
and this becomes
2ik
0
∂ψ
∂z
=−
∂
2
∂x
2
+
∂
2
∂y
2
ψ + k
2
0
1 − n
2
(x, y)
ψ, (8.146)

8.3 Bessel functions 293
which is the two-dimensional time-dependent Schrödinger equation, but with t replaced
by z/2k
0
, where z is the distance down the fibre. The wave modes that will be trapped
and guided by the fibre will be those corresponding to bound states of the axisymmetric
potential
V (x, y) = k
2
0
(1 − n
2
(r)). (8.147)
If these bound states have (negative) “energy” E
n
, then ψ ∝ e
−iE
n
z/2k
0
, and so the actual
wavenumber for frequency ω
0
is
k = k
0
− E
n
/2k
0
. (8.148)
In order to have a unique propagation velocity for signals on the fibre, it is therefore
necessary that the potential support one, and only one, bound state.
If
n(r) = n
1
, r < a,
= n
2
, r > a, (8.149)
then the bound state solutions will be of the form
ψ(r, θ) =
e
inθ
e
iβz
J
n
(κr), r < a,
Ae
inθ
e
iβz
K
n
(γ r), r > a,
(8.150)
where
κ
2
= (n
2
1
k
2
0
− β
2
), (8.151)
γ
2
= (β
2
− n
2
2
k
2
0
). (8.152)
To ensure that we have a solution decaying away from the core, we need β to be such
that both κ and γ are real. We therefore require
n
2
1
>
β
2
k
2
0
> n
2
2
. (8.153)
At the interface both ψ and its radial derivative must be continuous, and so we will have
a solution only if β is such that
κ
J
n
(κa)
J
n
(κa)
= γ
K
n
(γ a)
K
n
(γ a)
.
This Schrödinger approximation to the wave equation has other applications. It is called
the paraxial approximation.
294 8 Special functions
8.3.4 Spherical Bessel functions
Consider the wave equation
∇
2
−
1
c
2
∂
2
∂t
2
ϕ(r, θ , φ, t) = 0 (8.154)
in spherical polar coordinates. To apply separation of variables, we set
ϕ = e
iωt
Y
m
l
(θ, φ)χ(r), (8.155)
and find that
d
2
χ
dr
2
+
2
r
dχ
dr
−
l(l + 1)
r
2
χ +
ω
2
c
2
χ = 0. (8.156)
Substitute χ = r
−1/2
R(r) and we have
d
2
R
dr
2
+
1
r
dR
dr
+
ω
2
c
2
−
(l +
1
2
)
2
r
2
R = 0. (8.157)
This is Bessel’s equation with ν
2
→ (l +
1
2
)
2
. Therefore the general solution is
R = AJ
l+
1
2
(
kr
)
+ BJ
−l−
1
2
(
kr
)
, (8.158)
where k =|ω|/c. Now inspection of the series definition of the J
ν
reveals that
J
1
2
(x) =
<
2
πx
sin x, (8.159)
J
−
1
2
(x) =
<
2
πx
cos x, (8.160)
so these Bessel functions are actually elementary functions. This is true of all Bessel
functions of half-integer order, ν =±1/2, ±3/2, ... We define the spherical Bessel
functions by
2
j
l
(x) =
<
π
2x
J
l+
1
2
(x), (8.161)
n
l
(x) = (−1)
l+1
<
π
2x
J
−(l+
1
2
)
(x). (8.162)
2
We are using the definitions from Schiff’s Quantum Mechanics.
