Stacey F.D., Davis P.M. Physics of the Earth

Подождите немного. Документ загружается.

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C10.3D

–

147

– [135–148] 13.3.2008 10:35AM

(Eq. (10.27)) and rheological properties are very

sensitive to it. For materials such as minerals,

steady creep is observed only if T/T

is reasonably

high and at lower temperatures brittle failure

occurs instead. In the Earth the distinction is

apparent in the distribution of earthquakes,

which are restricted to relatively cool regions.

From a study of the thermal structure of the litho-

sphere at the point of subduction, McKenzie et al.

(2005) reached the conclusion that earthquakes

occur only where the temperature is below

600 8C. They interpreted this as the temperature

distinguishing brittle failure from creep. From

a detailed study of Californian earthquakes,

Bonner et al. (2003) concluded that the limit is

nearer to 400 8C. Taking the solidus temperature

of the uppermost mantle as T

 1400 K, we can

refer to a homologous temperature, T/T

¼0.5

to 0.6, as the critical condition distinguishing

seismic from aseismic behaviour. Although T

is not well defined, because the different miner-

als have different melting points, we take this

condition as an approximate guide to the varia-

tion in homologous temperature throughout

the mantle. T/T

< 0.5 only near to the surface

and in subducting slabs in the upper mantle

and not at all in the lower mantle. Implications

for lower mantle temperatures are considered in

Section 19.5.

10.7 Frequency dependent

elasticity and the dispersion

of body waves

Consider the special case of a seismic pulse

that starts as a  function, a square pulse of

infinitesimal duration. Its Fourier spectrum is

initially white, that is, wave components of all

frequencies have the same energy per unit fre-

quency interval. Crests of all of the component

waves coincide at the initiating  function, but

cancel elsewhere. But this cancellation fails

as soon as the mix of components changes by

selective removal of high frequencies. If we

make the assumption that all frequencies

travel at the same speed, then the coinciding

crests stay together as a peak of the pulse. But

it does not remain as a sharp  function; it

spreads out symmetrically to both earlier and

later times. The peak would arrive at any point

down the path at the time expected from the

wave speed, so half of the pulse arrives faster

than the wave speed. Worse, since there is no

sharp onset of the pulse, it begins to arrive

even before it has been initiated. This viola-

tion of the principle of causality demonstrates

that there is an erroneous assumption: wave

components of different frequencies cannot

travel at the same speed. A frequency-dependent

attenuation coefficient necessarily implies also

frequency-dependent speed or wave dispersion.

In the special case of frequency independent

attenuation, that is Q / !, there would be no

dispersion because all harmonic components of

a pulse would be similarly attenuated and it

would propagate undeformed, but with dimini-

shing amplitude.

The problem of attenuation-related disper-

sion is discussed by Aki and Richards (2002) and

the mathematical theory, based on the require-

ment of causality, is reviewed by Brennan and

Smylie (1981). Most discussions emphasize the

approximation of constant Q, that is Q independ-

ent of frequency, which yields a relationship

between the phase speeds, v, at two frequencies

and the Q of the medium,

vðf

¼ 1 þ



: (10:29)

This is a valuable result, showing that, for values

of Q observed for the Earth, the dispersion is

slight but not insignificant over the frequency

range of seismology. It is much more important

in comparing observations at seismic frequen-

cies with laboratory measurements at MHz or

GHz frequencies. In any case, Eq. (10.29) assumes

constant Q, and so is only a rough approximation

to the real Earth situation.

A more general treatment requires solution

of an integral involving the attenuation coeffi-

cient ( in Eq. (10.20)). The variation in phase

speed, v, with frequency, !

/2p, can be obtained

for an arbitrary variation of  with frequency,

using alternative expressions that relate v to

speed c at infinite frequency (Brennan and

Smylie, 1981),

10.7 FREQUENCY DEPENDENCE AND DISPERSION 147

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C10.3D

–

148

– [135–148] 13.3.2008 10:35AM

vð!



1

ð!Þ

 !



ð!Þ

 !

d!; (10:30)

where pv indicates the Cauchy principal value of

the integral and care is required in handling the

singularities. The first version of this integral is

the Hilbert transform of (!). Provided (!)is

known over a range extending well beyond the

frequency range of immediate interest, the

high-frequency limits of the integrals present

no problem because we are interested only in

the differences in v between finite values of !

and so subtract integrals from one another. A

simple qualitative argument shows what result

to expect. If  is independent of !, corresponding

to Q / ! or f, then the integrals in Eq. (10.30) are

independent of !

and there is no dispersion. In

this case we can see that all of the Fourier com-

ponents of the  function pulse considered above

are similarly attenuated. The pulse propagates

with diminishing amplitude but no change in

shape and no dispersion.

Now we can apply a simple amendment to

Eq. (10.29) to allow for frequency dependent Q.

If we write

Q / f

(10:31)

and consider an average of Q

1

over a moderate

frequency range of interest, then the equation

vðf

 1 þ

ð1  "Þ



1

i (10:32)

satisfies the two conditions, " ¼0 (Eq. (10.29)) and

" ¼1 (no dispersion). Equation (10.32) agrees with

laboratory data on diverse materials, including

plastics, metals, minerals and rocks, but it is

an empirical approximation, justified by the

fact that it is intermediate between the exact

solutions of Eq. (10.30) for " ¼0and" ¼1. Over

the frequency range from body waves to free

oscillations of the Earth, " 1/3 appears to be a

reasonable approximation, so that dispersion is

only about 2/3 as strong as in Eq. (10.29).

Linear anelastic mechanisms are relaxation

phenomena, so that, after a disturbance to any

element of material, causing an immediate elastic

response, there is a further, delayed, anelastic

response, which is an exponential relaxation

towards an equilibrium state. Thermally activated

movements of crystal dislocations past potential

barriers and the redistribution of heat between

compressed or dilated crystals with different

thermodynamic properties are examples of pro-

cesses that cause such relaxation. It follows

that the elastic modulus has a higher value at

high frequencies, which do not allow time for

the relaxation to occur, than at lower frequencies.

These are causes of a difference between relaxed

and unrelaxed moduli, considered in the special

case of composite materials in Section 10.4.

The variation of elastic modulus with fre-

quency is twice as strong as the variation in

phase speed, which depends upon the square

root of elastic modulus. In some analyses what

is here referred to as the elastic modulus is con-

sidered to be the real part of a complex modulus,

with the imaginary part representing strain that

is p/2 out of phase with stress. The real compo-

nent, or modulus, is the gradient of the axis of a

hysteresis loop, as in Fig. 10.3, and experiments

confirm that the modulus increases with fre-

quency. If the phase lag between strain and

stress is  then Q

1

¼tan , which is the ratio

of imaginary t o real components of t he modulus

(not to be confused with the logarithmic decre-

ment (Eq. (10.21)).

Since body waves have a slight positive dis-

persion (phase speed increasing with frequency),

group speed is slightly greater than phase speed

(see Eqs. (16.49) to (16.51)). Thus, at any particu-

lar frequency,

u ¼ v= 1 

1  "



: (10:33)

However, u is less than the limiting phase speed

at high frequency, which corresponds to the

value of v for the completely unrelaxed modulus.

It should be emphasized that the theory of

body wave dispersion is valid only for linear

attenuation mechanisms that operate at large dis-

tances from earthquakes. As we have mentioned,

anelasticity may be non-linear within about

ten fault dimensions. We have referred also to

the observational problem that high frequency

waves are more readily scattered than low fre-

quencies and effects of scattering can be confused

with attenuation.

148 ELASTIC AND INELASTIC PROPERTIES

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

149

– [149–162] 13.3.2008 11:19AM

Deformation of the crust: rock mechanics

11.1 Preamble

The theory of elasticity in Chapter 10, even the

discussion of inelastic deformation, does not pro-

vide a description of the processes that led to the

geological features that we see in the surface

layers of the crust. We need a different theoretical

approach to the deformation of materials such as

crustal rocks that are granular, cool and at low

pressure. What determines how or why rocks

deform or break? How are the orientations of

faults related to the stresses that cause them?

How is crustal stress estimated? How is the safety

of mines and tunnels assessed? Can laboratory

observations of rock failure be extrapolated to

describe earthquakes? These are questions

addressed by the discipline of rock mechanics.

In its modern form it is used mainly by mining

engineers and the applied mathematicians who

work with them. Here it is applied to tectonics.

The mathematical methodology of the sub-

ject uses the tensor notation. This is given a

brief introduction in Section 10.3, in the discus-

sion of elasticity of crystals, and is extended in

this chapter. It is a convenient representation of

stress patterns involving superimposed com-

pression and shear, including rotation of refer-

ence axes, to identify principal stresses. The

most obvious and useful application is to the

criteria for mechanical failure.

Earthquakes are dramatic instances of failure

and most of them are shallow, by which we mean

that they are confined to the lithosphere, the cool

surface layer of the Earth. The exceptions occur in

the zones of subduction or convective downwel-

ling, where planes of earthquake foci trace the

penetration of the mantle by the cool lithospheric

material to depths as great as 700 km. They are

examples of mechanical failure, in which material

loses its coherence. It is essentially a phenomenon

of ‘cool’ material, at homologous temperatures

T/T

< 0.5, where T

is the melting point or

solidus temperature. Most of the mantle is at

higher temperatures and its convective defor-

mation, described as creep in Section 10.6,

occurs without sudden, localized failure.

Rock mechanics provides an interpretation of

different styles of faulting in terms of stress ori-

entations in the remote past as well as now.

Earthquake fault plane solutions (Section 14.4)

give the same information much more widely,

but only at the present time. Stresses in aseismic

areas are determined by application of rock

mechanics equations to borehole measure-

ments. All of these observations are combined

to produce stress maps, which now cover a large

fraction of the globe.

11.2 The tensor representation

of stress and strain

Stress can be represented in three dimensions as

a tensor. We consider an orthogonal coordinate

system (x

), written x

, being the Cartesian

(x,y,z), and identify three areas each with its nor-

mal aligned with one of the axes. Each area is

represented by a unit vector n

in the direction of

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

150

– [149–162] 13.3.2008 11:19AM

the normal and a magnitude A

. In the conven-

tion of continuum mechanics, stress is the force

per unit area exerted on material on the negative

side of the area by material on the positive side.

An area can be subjected to three independent

forces, one normal to it (in the direction of n

and two tangential. With three force compo-

nents acting on each of the three areas, a total

of nine stress components is possible. However,

as we shall see, only six are independent. A nor-

mal stress is a force, parallel to the area normal,

divided by the area on which it acts. It can be

either extensional or compressional depending

on whether the force is directed parallel or anti-

parallel to the area normal. In this convention an

extensional stress is positive but the opposite sign

convention is used in rock mechanics, in which

compressions predominate. The convention that

compression is positive is also used in treatments

of finite strain and high pressure equations of

state (Chapter 18). Recognizing the danger of con-

fusion, we use the continuum mechanics conven-

tion where this is standard and convert to the

rock mechanics convention for the discussion of

the Mohr circle in Section 11.4 and crustal

stresses in the following sections.

Shear stresses are the tangential forces per

unit area. A component of the stress tensor,

written 

, corr esponds to a f orce acting in

direction i on unit area of a plane with normal

j. Shear stress is positive if the force acts in the

positive i direction on t he positive side of the j

plane. With the corresponding Cartesian

notation,







: ð11:1Þ

In one dimension, strain is a fractional

change in length. In three dimensions, six

strains are possible, three length changes, in

the x

, x

and x

directions, denoted e

, e

and three shears e

, e

. The shear strain is

defined as half the angular change in the right

angle defined in the material by the x

, x

axes

prior to deformation. From this it is evident that

¼e

, and that there are only three independent

shear strains. This is mentioned in Section 10.3

and explained again below.

After application of a stress, the new positions

of material points in a body are given by the

displacement field u(x,y,z). Strains are gradients

in the displacement field. Consider a body sub-

jected to a stress that causes a displacement defor-

mation field u ¼(u

). Let the displacement in

the x

direction at two points a distance dx

apart

be u

and u

þdu

(Fig. 11.1). From a Taylor expan-

sion, u

ðx

þ dx

Þ¼u

ðx

Þþð@u

=@x

Þdx

...

we retain only linear terms du

¼ð@u

=@x

Þdx

that is we consider only linear or infinitesimal

elasticity theory, applicable to small strains. The

strain e

is the change in length, (qu

/@x

)dx

,per

unit original length dx

,thatis

¼ @u

=@x

: (11:2)

We treat shear strains in a similar manner.

Figure 11.2 shows the angle changes to what

are right angles in unstrained material. Shear

strain is

¼ 1=2ð

þ 

Þ¼1=2ð@u

=@x

þ @u

=@x

Þ: ð11:3Þ

If 

6¼

the body rotates, with rotation defined

as ð

 

Þ=2 ¼





We can generalize these results to three

dimensions,



; (11:4)

where i and j take on the values 1, 2 and 3.

Consider the volume dx

(Fig. 11.3), sub-

jected to shear stresses in the x

plane. The

tangential forces acting on each side from the

material external to the volume are 



, 

and 

. Then

the torque is 

.dx



.dx

(



)dx

. For the torque to be

δu

FIGURE 11.1 Variation of displacement u

in the

direction x

, giving the strain e

150 DEFORMATION OF THE CRUST: ROCK MECHANICS

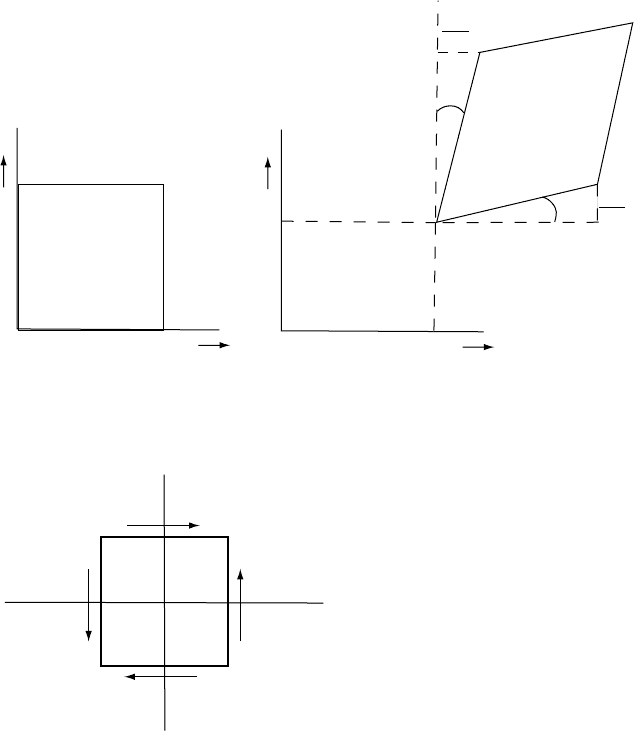
//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

151

– [149–162] 13.3.2008 11:19AM

independent of volume, dx

, 

¼

Similarly 

¼

and 

¼

. Generalizing,



¼

,and,becauseinisotropicmaterialshear

stress is proportional to the corresponding strain,

¼e

, with the result that only six elements of

the stress (strain) tensor are independent.

11.3 Hooke’s law in three

dimensions

For an isotropic material two independent mo-

duli are required and there are several ways of

choosing them (Sections 10.1 and 10.2). In

most geophysics these are bulk modulus, K, relat-

ing pressure to volume change, and rigidity mod-

ulus, , relating shear stress to change of shape

with constant volume. A different choice arises

from the tensor notation in which the three

dimensional version of Hooke’s law is expressed as



¼ lðe

þ e

Þþ2e

;



¼ lðe

þ e

Þþ2e

;



¼ lðe

þ e

Þþ2e

;



¼ 2e

;



¼ 2e

;



¼ 2e

;

(11:5)

where l and  are referred to as the Lam

e coef-

ficients but  is familiar as the shear modulus.

Equations (11.5) can be written in a convenient

shorthand as



¼ le

þ 2e

; (11:6)

where  is the Kroeneker delta for which



¼1if i ¼ j;



¼0if i 6¼ j:

(11:7)

e ¼e

þe

is the sum of fractional changes

in length in three directions which gives the rel-

ative volume change. The relationships to the

alternative isotropic moduli are here re-derived

by application of Eq. (11.6). Pressure is defined as

the average normal stress

, u

0, u

∂u

∂x

∂u

∂x

FIGURE 11.2 Displacements due to shear strain. Strains involve variations in displacements as a function of the

coordinate system.

–

FIGURE 11.3 Forces on the faces of an elementary

volume from a stress field 

11.3 HOOKE’S LAW IN THREE DIMENSIONS 151

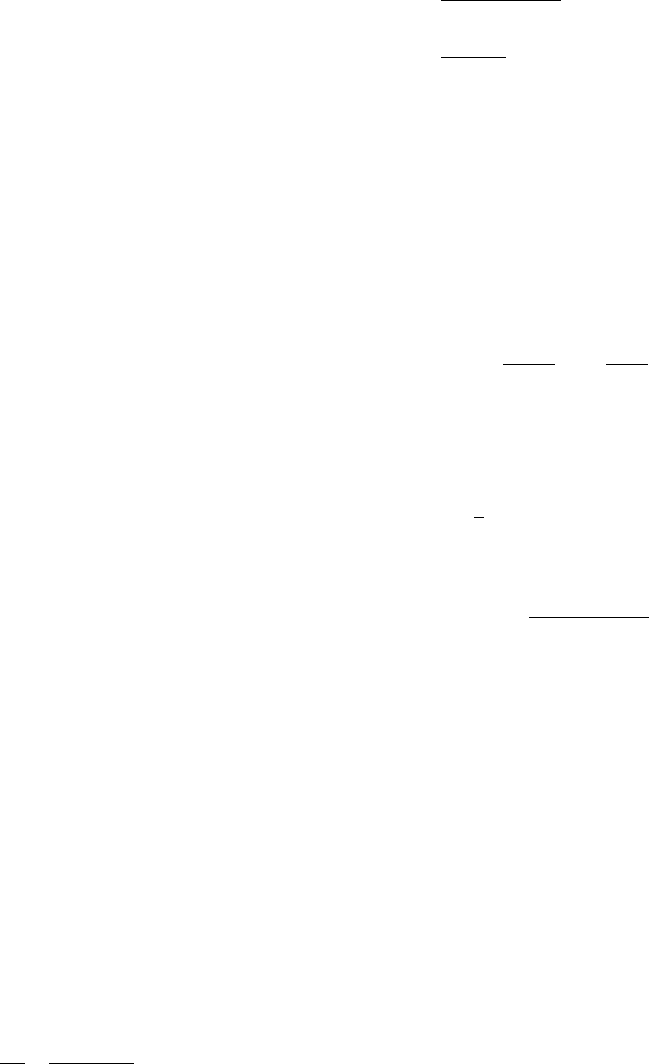
//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

152

– [149–162] 13.3.2008 11:19AM

p ¼P ¼ð

þ 

Þ=3; (11:8)

where P has compression positive, as used in

rock mechanics and interior stresses in the

Earth. Bulk modulus, K, the ratio of pressure to

volume change, is obtained by summing the first

three of Eqs. (11.5):

K ¼ P=e ¼ðl þ 2=3 Þ: (11:9)

Poisson’s ratio, , is not strictly a modulus, but

a dimensionless ratio. However, it is a useful

parameter that is always listed with elastic mod-

uli. Let uniaxial stress 

be applied in the x

direction to a rectangular block of elastic material

with the other sides free so that the other two

stress components are zero. The block expands

laterally if the body is compressed axially or will

contract laterally if it is extended. Poisson’s ratio

is the negative ratio of lateral to longitudinal

strains (a negative sign because the strains are of

opposite signs). Equations (11.5) become



¼ðl þ 2Þe

þ le

;

0 ¼le

þðl þ 2Þe

þ le

;

0 ¼le

þ le

þðl þ 2Þe

(11:10)

By symmetry, the lateral strains are equal, e

¼e

and Poisson’s ratio is

 ¼e

¼ l=ð2l þ 2Þ: (11:11)

An approximation that is sometimes used to

simplify rock mechanics equations is l ¼, giv-

ing  ¼1/4, referred to as a Poisson solid. While

the simplification may be justified where rough

solutions suffice, it underestimates Poisson’s

ratio for most solid materials, for which  ¼0.3

is a better approximation at low pressure, and it

increases systematically with pressure (for a rea-

son discussed in Section 18.8). For a fluid,  ¼0,

so that by Eq. (11.11) Poisson’s ratio is 0.5 and

this applies to the outer core and oceans.

Young’s modulus, E, is the ratio of longitudi-

nal stress to strain, with no lateral stress, 



¼0, as in the consideration of Poisson’s ratio

in Eq. (11.11).

E ¼



ð3l þ 2Þ

l þ 

: (11:12)

We can use Eqs. (11.11) and (11.12) to solve for the

Lam

e coefficients, l and , in terms of Young’s

modulus and Poisson’s ratio:

l ¼

E

ð1 þ Þð1  2Þ

;

 ¼

2ð1 þ Þ

(11:13)

If a linear stress 

produces a strain e

with

lateral strain prevented, then the first of

Eqs. (11.5) gives

 ¼ 

¼ðl þ 2Þ: (11:14)

This is the modulus controlling P-wave propaga-

tion in the Earth because the juxtaposition of

compressed and extended layers ensures that

¼e

¼0. The lateral stresses



¼ 

l þ 2





1  



(11:15)

are self-adjusted to make this so. A more useful

and familiar relationship for  is obtained by

substituting for l by Eq. (11.9):

 ¼ K þ

; (11:16)

and we note that

 ¼ðl þ 2Þ¼

ð1  ÞE

ð1 þ Þð1  2Þ

: (11:17)

These relationships are summarized in

Appendix D.

11.4 Tractions, principal stresses

and rotation of axes

The stress tensor can be thought of as a table of

coefficients that can be multiplied by an area

vector to give the forces on that area. Consider

an element of area of size A (Fig. 11.4) with direc-

tion cosines n

. The forces per unit area applied to

the material on the negative side of A by material

on the positive side are called the tractions and

are given by

j¼1



¼ 

(11:18)

using the Einstein summation rule for repeated

indices.

152 DEFORMATION OF THE CRUST: ROCK MECHANICS

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

153

– [149–162] 13.3.2008 11:19AM

Thus

¼ 

þ

;

¼ 

þ

;

¼ 

þ

(11:19)

For any stress field, 

,inanisotropicmaterial

it is possible to find three orthogonal unit areas

across which the tractions have no tangential com-

ponents, that is vector T is parallel to the area

vector n (Fig. 11.4(b)), which means that n is a

direction of principal stress. This requires the trac-

tion components to be proportional to the direc-

tion cosines of the areas. Let the constant

of proportionality be l, T

¼ln

. Substituting

Eq. (11.19) for T

in Eq. (11.18) and using the

Einstein summation rule, with the Kroenecker 

as in Eq. (11.7),



¼ l

;



 l

¼ 0:

To have a solution to this set of simultaneous

equations the determinant must be zero,

detð

 l

Þ¼ 

 l



¼ 0: (11:20)

Equation (11.20) is the characteristic equation

for an eigenvalue problem. In three dimensions

it is cubic in l,



 l 



l 



 l



¼ 0; (11:21)

which has three roots {l

, l

}, corresponding to

the three principal stresses. The corresponding

eigenvectors are the three unit (vector) areas

corresponding to each of l

, l

To find the eigenvector corresponding to l

for example, we require three equations in three

unknowns {n

}. Equation (11.21) comprises two

independent equations. The third is the ortho-

normal property of the unit area vectors

i¼1

¼ 1. Thus for l

the equations are

þ n

¼ 1;



þ 

¼ l

;



þ 

¼ l

(11:22)

An example in two dimensions

In two dimensions Eq. (11.22) reduces to

ð

 lÞ 



ð

 lÞ



¼ 0:

The characteristic equation becomes

ð

 lÞð

 lÞ

¼ 0;

 lð

þ 

Þþ



 

¼ 0;

(11:23)

the solution of which is

l ¼

ð

þ 



ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ð





þ

: (11:24)

Suppose that the stress field is a pure shear (



¼0). Then l ¼

Choose l

¼þ

. Solving for the eigen-

vector,

þ n

¼ 1;



¼ l

;

giving n

¼ n

; and therefore

¼ n

¼ 1=

ﬃﬃﬃ

Traction

Area

(b)

Area

(a)

FIGURE 11.4 Tractions are forces applied by material

on the positive side of an elementary area under stress.

Case (a) is the general one and if the vector sum of the

tractions is normal to the area, as in case (b), then the

normal is a direction of principal stress.

11.4 ROTATION OF THE STRESS TENSOR 153

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

154

– [149–162] 13.3.2008 11:19AM

As is obvious in this simple case the principal

stresses are a tension and compression equal to

the value of the shear stress and oriented at 458

to the shear direction.

Rotation of stress or strain tensors

Suppose that we wish to determine the compo-

nents of the stress tensor in a rotated coordinate

system x

relative to an unrotated system x

. The

traction force vector in the rotated system is

given by Eq. (11.19). The force components

resolved normal and tangential to the axial

planes of the rotated system give the new normal

and shear stresses, respectively. For example, in

two dimensions, if the axes are rotated anticlock-

wise by , the direction cosines of the area with

normal x

are n

¼½cos ; sin . From Eq. (11.19)

the tractions are T

¼ð

cos  þ 

sin Þ;

¼ð

cos  þ 

sin Þ. Stresses in the rotated

system, 

and 

, are found by resolving

the traction vector normal and parallel to the

area:



¼ðT

cos  þ T

sin Þ;



¼ðT

sin  þ T

cos Þ:

(11:25)

Inserting T

and T

we obtain



¼ 

cos

 þ 

sin

 þ 

sin 2;



 

sin 2 þ 

cos 2:

(11:26)

Repeating for the area with normal x

, i.e.,

 !

þ ;



¼ 

sin

 þ 

cos

  

sin 2:

(11:27)

By examining (11.26) and (11.27) we see that the

procedure for finding tractions in the rotated

system, then resolving them to normal and tan-

gential directions to find the stresses, can be

expressed in matrix form as the product of two

rotations,

cos  sin 

sin  cos 



; R

cos  sin 

sin  cos 



(11:28)

Then 

¼R

R

¼R R

, where T refers to the

transpose of a matrix.

Generalizing to three dimensions, we let R

cos(angle between x

and x



m¼1

n¼1



¼ RR

: (11:29)

The Mohr Circle

Consider a special case with the x

coordinate axes

so chosen that 

¼

¼0, that is 

and 

are

principal stresses, which we will denote by 

and



. After rotation by angle  , Eqs. (11.26) and

(11.27) may be rewritten as







þ 

 r cosð2Þ r sinð2Þ

r sinð2Þ



þ 

þ r cosð2Þ

;

(11:30)

where

r ¼



 

: (11:31)

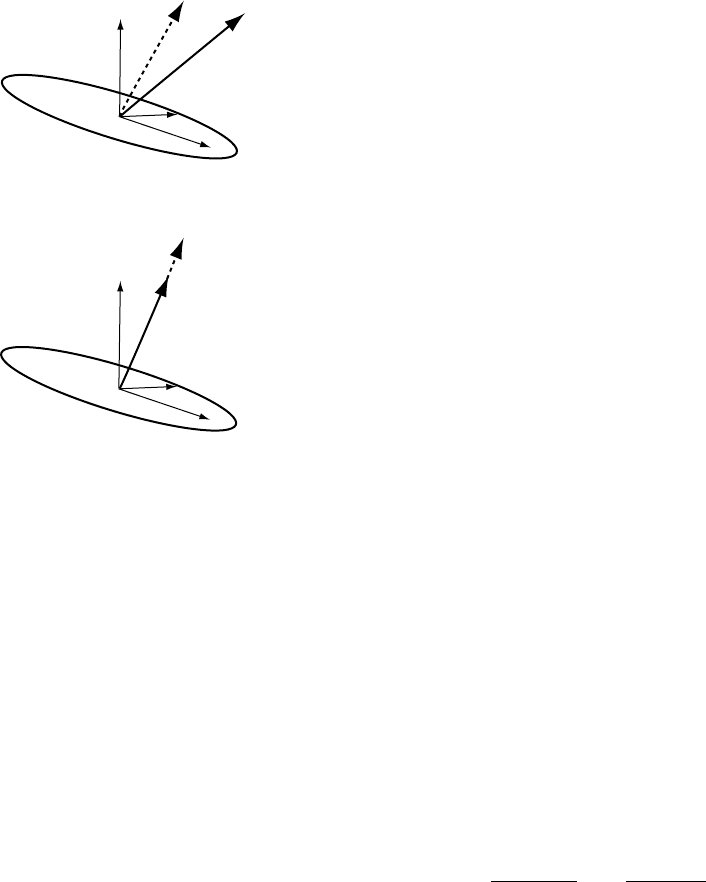
From Eq. (11.30) we see that the normal and

shear stresses can be represented by a circle dia-

gram (Fig. 11.5). The axes of the diagram are the

normal stress and shear stress components

(

, ). The centre of the circle is located at



þ 

; ¼ 0. The radius of the circle is r

(Eq. (11.31)). The stresses in a plane with its nor-

mal at angle  to the direction of the x

axis are

found by drawing the vector r from the point



þ 

; 0



at angle 2 to the 

axis. The 

axis component gives the shear in the rotated

system; the 

axis component gives one normal

stress; the 

axis component of negative r gives

the other normal stress.

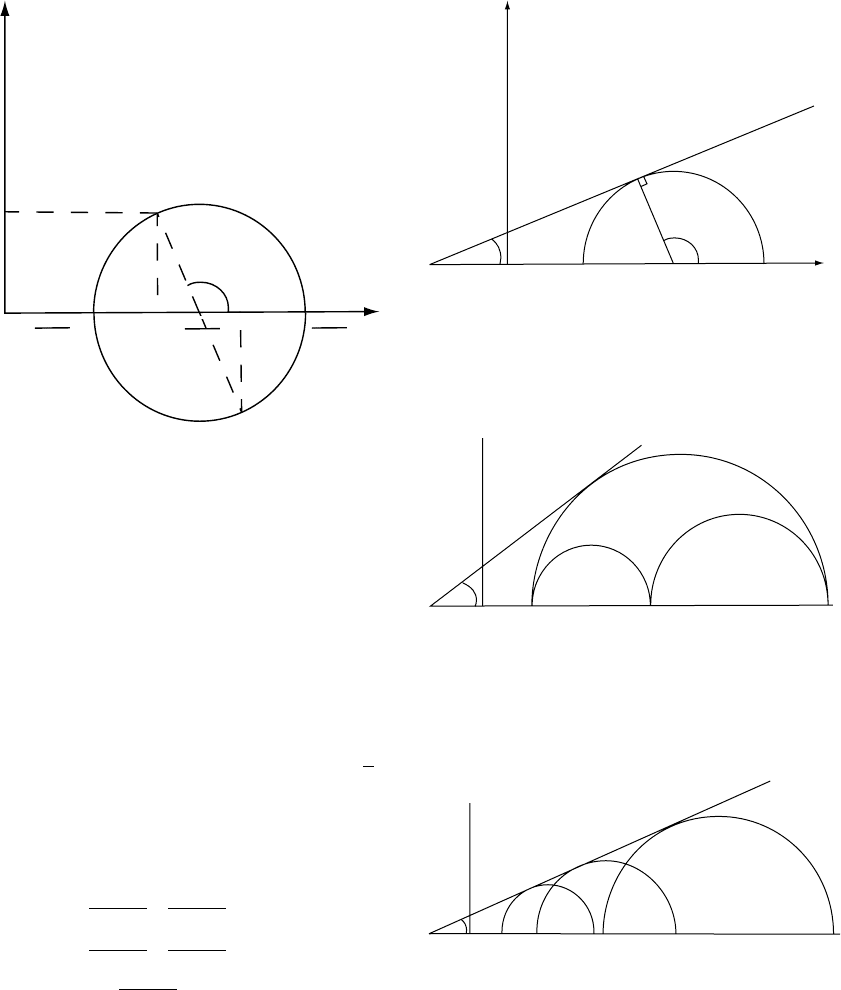
To convert the circle diagram (Fig. 11.5) from

the elastic (compressions negative ) to the rock

mechanics convention (compressions positive)

the normal stresses must be reversed in sign.

We rotate the circle diagram about the vertical

axis, and change the sign of r in Eq. (11.31) to

r ¼



 

(see Fig 11.6(a)). Then 

is the largest

(positive) compressive stress. The circle so

formed is called the Mohr circle (Fig. 11.6(a))

154 DEFORMATION OF THE CRUST: ROCK MECHANICS

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

155

– [149–162] 13.3.2008 11:19AM

after its inventor, O. Mohr. The intercepts of the

Mohr circle with the horizontal axis are the prin-

cipal stresses 

, 

. When the reference plane

rotates by an angle  in physical space, the cor-

responding point in the Mohr circle diagram

(Fig. 11.6(a)) moves around the circle by an

angle 2. From the Mohr circle we observe

that the maximum shear occurs at 2 ¼

,or

 ¼458, to the principal stress direction, and

has a value equal to half the difference in mag-

nitude of the maximum and minimum principal

stresses. From Eq. (11.30) we have



þ 



 

cosð2Þ;



þ 





 

cosð2Þ;



¼ ¼



 

sinð2Þ;

(11:32)

from which we can derive the relation



 

¼ð

 

Þcosð2Þ (11:33)

which we use in Chapter 13.

The three-dimensional case involves three

principal stresses, 

, 

. Let 

>

.We

can apply the Mohr circle analysis on each plane

containing two of the principal stresses, i.e.,

{

,

}, {

,

}, {

,

}, which results in three

Mohr circles as in Fig. 11.6(b). Then each circle

–

–r

2θ

σ'

FIGURE 11.5 Shear stress (vertical axis) plotted against

normal stresses (horizontal axis). Shear stress in a plane

at any angle to the x-axis is obtained by the projection of

the r vector on the vertical axis. Normal stresses are

obtained by the projections of the r vector and negative r

vector on the horizontal axis.

2θ

FIGURE 11.6(a) Mohr circle. Special case where the

horizontal axis is the direction of greatest principal

stress. The sloping line gives the frictional strength.

Frictional failure occurs when the Mohr circle intersects

the friction line.

FIGURE 11.6(b) Mohr circle for three dimensions. Each

circle represents a plane containing two of the principal

stresses. The failure line occurs in the plane with the

greatest shear stress.

FIGURE 11.6(c) Increasing pressure causes stability

against frictional failure by moving the centres of Mohr

circles to the right, which means greater shears must be

developed for their radii to reach the failure line.

11.4 ROTATION OF THE STRESS TENSOR 155

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C11.3D

–

156

– [149–162] 13.3.2008 11:19AM

describes stress components on a plane lying

between the principal stress pair. We see that

in Fig. 11.6(b) the maximum shear occurs in the

plane containing the intermediate principal

stress and bisects the angle between the greatest

and least principal stresses.

11.5 Crustal stress and faulting

Since any stress pattern can be resolved into three

principal stresses, 

, 

, and deep in the Earth

these are all large compared with the differences

between them, it is often convenient to identify the

average as a hydrostatic pressure, as in Eq. (11.8),

recalling that p ¼(

þ

)/3, and we refer to

the differences as deviatoric stresses. These are

generally small enough to be treated by the linear

(infinitesimal) elasticity theory, allowing a simple

superposition on hydrostatic compression, even

when the response to hydrostatic pressure is

strong enough to require analysis by a finite strain

theory, as in Chapter 18. Except near to the sur-

face, the hydrostatic pressure, P, is approximated

by the lithostatic or overburden pressure,

P 

g dz; (11:34)

which can be used as one of the equations needed

for complete specification of the stress pattern. In

common usage this is a positive quantity, but for

substitution in the standard equations of elastic-

ity theory, in which extension is positive, pres-

sure is taken as the negative quantity P.Then

the tectonic stresses causing deformation, the

deviatoric stresses, are obtained from the total

stresses by subtracting the pressure, p:



¼ 

 p

¼ 

þ P

: (11:35)

Faults are often planes of weakness estab-

lished by repeated movements, but their original

causes were tectonic stresses in directions that

determined the fault orientations. Tectonic

movements, ultimately driven by mantle con-

vection, persist in the same directions for many

millions of years, so that faults reflect the driving

forces that caused them, even where established

planes of weakness may now have a strong influ-

ence. Similarly, faults that are no longer active

indicate the orientations of stresses that moved

them many years ago. This is the reason for

interest in the classification of faults. They are

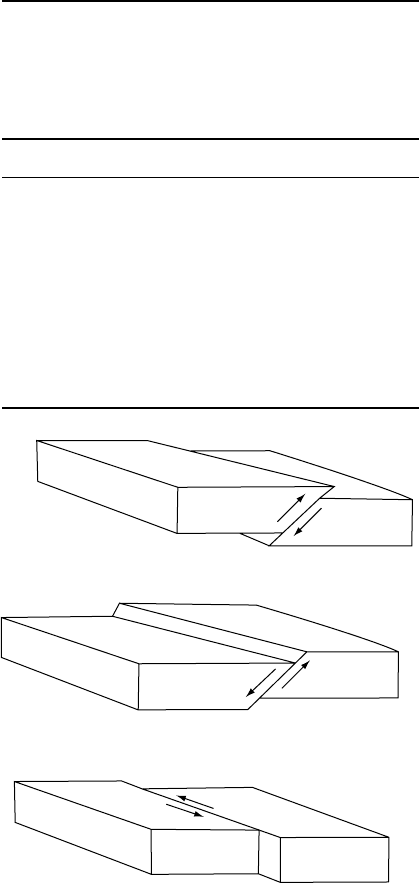
of three types, illustrated in Fig. 11.7, corres-

ponding to different stress orientations, as clas-

sified by Anderson (1905). The Anderson faulting

criteria are summarized in Table 11.1.

Reverse fault

Normal fault

Strike-slip fault

FIGURE 11.7 Different fault types.

Table 11.1 Anderson faulting criteria.



, 

, are maximum, intermediate and

minimum principal stresses, respectively.

Note that ‘strike’ means the orientation of

the surface trace of the fault



Normal

fault

vertical horizontal

along

strike

horizontal

Strike slip

fault

horizontal

(angle to

strike)

vertical horizontal

Reverse

(thrust)

fault

horizontal horizontal

along

strike

vertical

156 DEFORMATION OF THE CRUST: ROCK MECHANICS