Simon Benninga. Financial Modelling 3-rd edition
Подождите немного. Документ загружается.


428 Chapter 16
16.4.2.2 Payoff Pattern from a Written Call
In options markets the purchaser of a call buys the call from a counter-
party who issues the call. In the jargon of options, the issuer of the call
is called the call writer. It is worthwhile to spend a few minutes consider-
ing the difference between the security bought by the call purchaser and
the call writer:
•
The call purchaser buys a security that gives the right to buy a share
of stock on or before date T for price X. The cost of this privilege is
the call price C
0
, which is paid at the time of the call purchase. Thus
the call purchaser has an initial negative cash fl ow (the purchase
price C
0
); however, his cash fl ow at date T is always nonnegative:
max (S
T
− X, 0).
•
The call writer gets C
0
at the date of the call purchase. In return
for this price, the writer of the call agrees to sell a share of the stock
for price X on or before date T. Notice that whereas the call pur-
chser has an option, the call writer has undertaken an obligation.
Furthermore, note that the cash fl ow pattern of the call writer is
opposite to that of the call purchaser: The writer’s initial cash fl ow
is positive (+C
0
), and her cash fl ow at date T is always nonpositive:
−max (S
T
− X, 0).
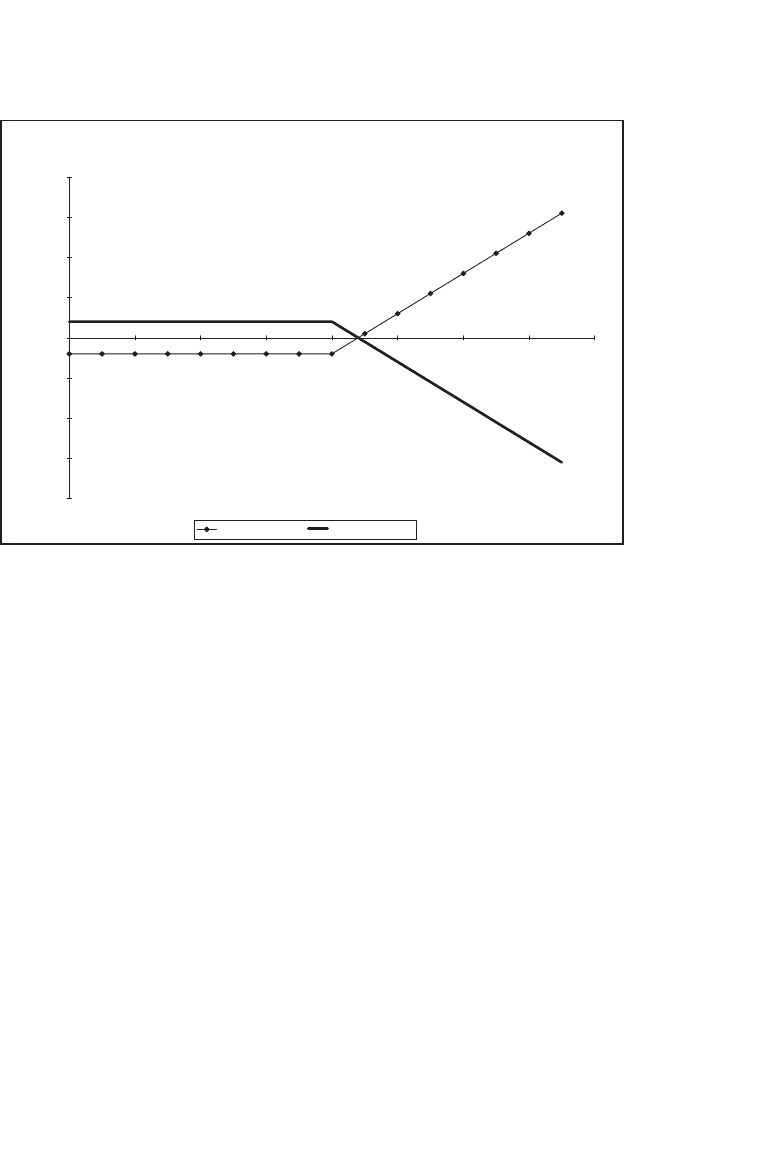
The profi t of a call writer is the opposite of that of the call purchaser.
For the case of the GP options,
Call writer’s profi t in September
=− −
=− −
=
+≤
−>
⎧
⎨
⎩
CSX
S
S
SS
T
T
T
TT
0
0
4040
440
44 40
max ( )
max ( )
,
,
if
if
Graphing the profi t patterns of the bought and the written call gives:
429 An Introduction to Options
16.4.3 Put Option Profi t Patterns
16.4.3.1 Payoff Pattern from a Purchased Put
If in July you bought one GP September 40 put for $2, then in September
you will exercise the put only if the market price of GP is lower than
$40. If we write the initial (July) put price as P
0
, we can write the profi t
function from the put in September as follows:
Put profi t in September =−−
=−−
=
−≤
−>
⎧
⎨
⎩
max ( )
max ( )
0
040 2
38 40
240
0
,
,
if
if
XS P
S
SS
S
T
T
TT
T
16.4.3.2 Payoff Pattern from a Written Put
The put writer obligates herself to purchase one share of GP stock on or
before date T for the put exercise price of X. For putting herself in this
invidious position, the writer of the put receives, at the time the put
is written, the put price P
0
. The payoff pattern from writing the GP
September 40 put is therefore
Call Option Profit Patterns
–40
–30
–20
–10
0
10
20
30
40
0 1020304050607080
Terminal stock price, S
T
($)
Profit ($)
Bought call Written call
430 Chapter 16
Put writer’s profit in September ,
,
=− −
=− −
PXS
S
T
T
0
0
2040
max ( )
max ( )
==
−+ ≤
>
⎧
⎨
⎩
38 40
240
SS
S
TT
T
if
if
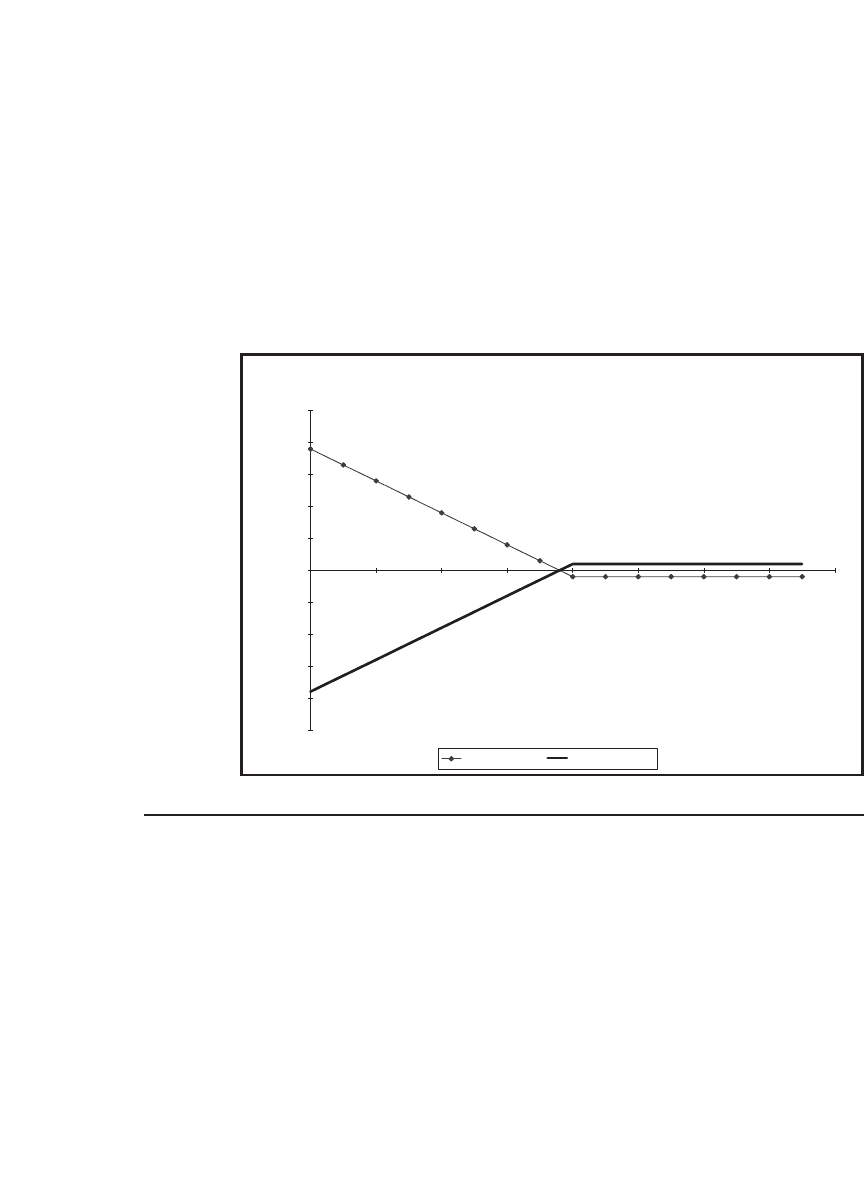
Graphing the profi t patterns of the bought and the written put gives the
following:
Put Option Profit Patterns
–50
–40
–30
–20
–10
0
10
20
30
40
50
0 1020304050607080
Terminal stock price, S
T
($)
Profit ($)
Bought put Written put
16.5 Option Strategies: Payoffs from Portfolios of Options and Stocks
There is some interest in graphing the combined profi t pattern from a
portfolio of options and stocks. These patterns give an indication of how
options can be used to change the payoff patterns of “standard” securities
such as stocks and bonds. Here are a few examples.
16.5.1 The Protective Put
Consider the following combination:
•
One share of stock, purchased for S
0
.
•
One put, purchased for P with exercise price X.
431 An Introduction to Options
This option strategy is often called a “protective put” strategy or “port-
folio insurance”; in Chapter 21 we return to this topic, exploring it
in much further detail. The payoff pattern of the protective put is
given by
Stock profi t + Put profi t
=−+ − −
=
−+−−
−−
⎧
⎨
⎩
=
−−
−
SS XS P
SSXSP
SSP
XS P
SS
TT
TT
T
T
00
00
00
00
0max ( ),
000
−
⎧
⎨
⎩
≤
>
≤
>P
SX
SX
SX
SX
T
T
T
T
if
if
if
if
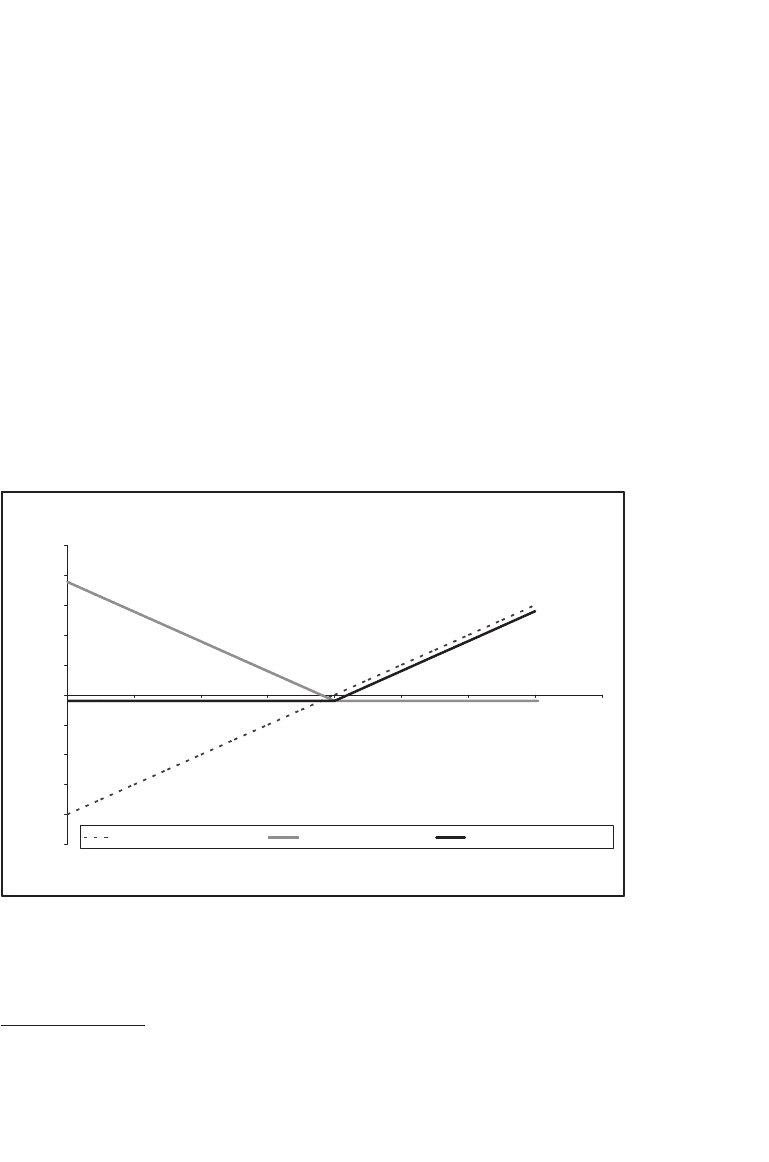
When applied to the GP example (that is, buying a share at $40 and a
put with X = $40 for $2) this strategy gives the following graph:
Protective Put Profit
–50
–40
–30
–20
–10
0
10
20
30
40
50
0 10203040506070
Terminal stock price, S
T
($)
Profit ($)
80
Terminal stock profit Terminal put profit Protective put profit
This pattern looks very much like the payoff patterns from a call.
6
6. In section 16.6 we prove and illustrate the put-call parity theorem. It follows from this
theorem that a call must be priced at a price C such that C = P + S
0
− Xe
−rT
. Thus, when
calls are correctly priced according to this theorem, the payoff from a put + stock
combination is the same as that from a call + bond combination.
432 Chapter 16
16.5.2 Spreads
Another combination involves buying and writing calls with different
exercise prices. When the bought call has a low exercise price and the
written call has a higher exercise price, the combination is called a bull
spread. As an example, suppose you bought a call (for $4) with an exer-
cise price of $40 and wrote a call (for $2) with an exercise price of $50.
This bull spread gives a profi t of
max ( ) [max ( ) ]SS
SS
S
TT
TT
T
−−− −−
=
−+
−−+=−
−−
40 0 4 50 0 2
42
40 4 2 42
40 4
,,
−−−−=
⎧
⎨
⎪
⎩
⎪
≤
≤≤
≥()S
S
S
S
T
T
T
T
50 2 8
40
40 50
50
if
if
if
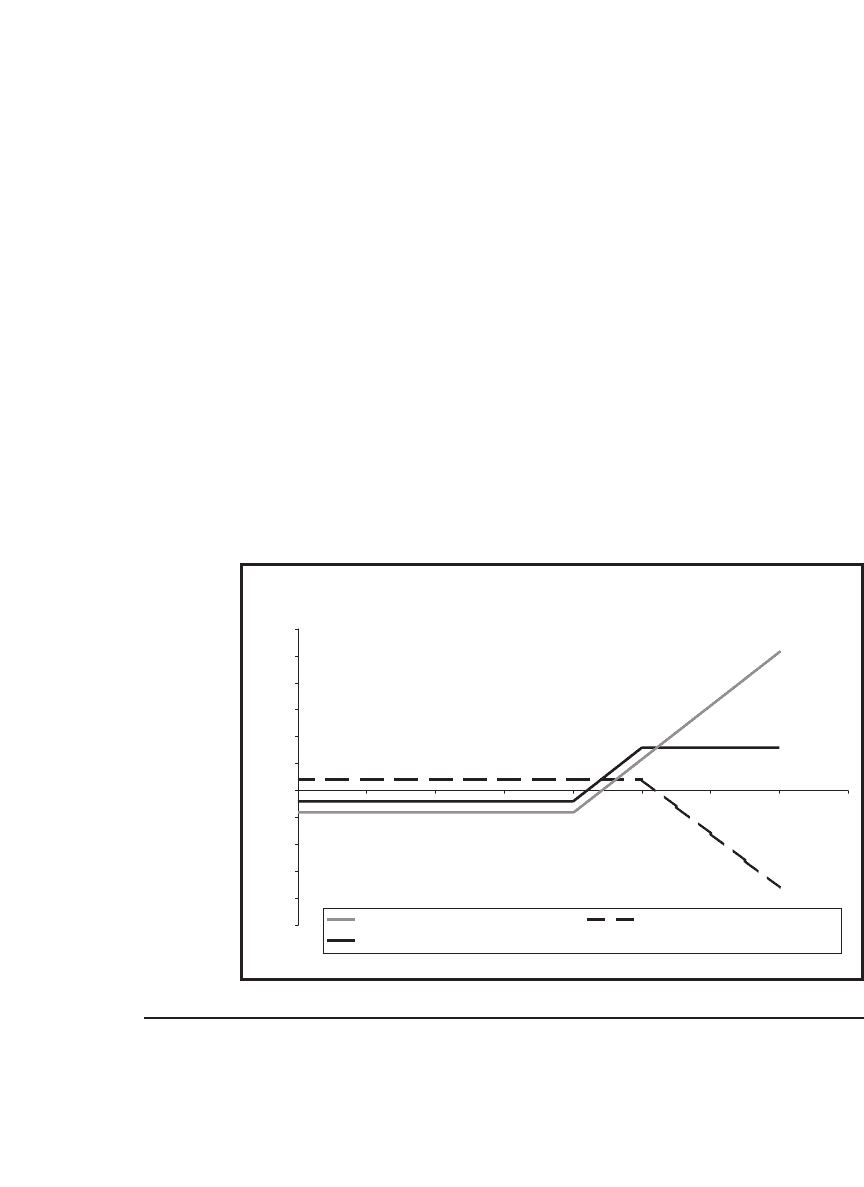
The following Excel graph shows each of the two calls and the resulting
spread profi t:
Bull Spread Profit Chart
–25
–20
–15
–10
–5
0
5
10
15
20
25
30
0 10203040506070
Terminal stock price, S
T
($)
Profit ($)
80
Terminal purchased call, X = 40 Terminal written call, X = 50
Terminal spread profit
16.6 Option Arbitrage Propositions
In succeeding chapters we price options given specifi c assumptions about
the probability distribution of the underlying asset (usually the stock) on
433 An Introduction to Options
which the option is written. However, there is much that can be learned
about the pricing of options without making these specifi c probability
assumptions. In this section we consider a number of these arbitrage
restrictions on option pricing. Our list is by no means exhaustive, and we
have concentrated on those propositions which provide insight into the
pricing of options or that will be used in later sections.
Throughout we assume that there is a single risk-free interest rate that
prices bonds; we also assume that this risk-free rate is continuously com-
pounded, so that the present value of a riskless security that pays off X
at time T is given by e
−rT
X.
proposition 1 Consider a call option written on a stock that pays no
dividends before the option’s expiration date T. Then the lower bound
on a call option price is given by
CSXe
rT
00
0≥−
−
max ( ),
Comment Before proving this proposition, it will be helpful to consider
its meaning: Suppose that the riskless interest rate is 10 percent, and
suppose we have an American call option with maturity T = 1/2 (i.e., the
expiration date of the option is one-half year from today) with X = 80
written on a stock whose current stock price S
0
= 83. A naive approach
to determining a lower bound on this option’s price would be to state
that it is worth at least $3, since it could be exercised immediately with
a profi t of $3. Proposition 1 shows that the option’s value is at least 83 −
e
−0.10
*
0.5
80 = 6.90. Furthermore, a careful examination of the following
proof will show that this fact does not depend on the option being an
American option—it is also true for a European option.
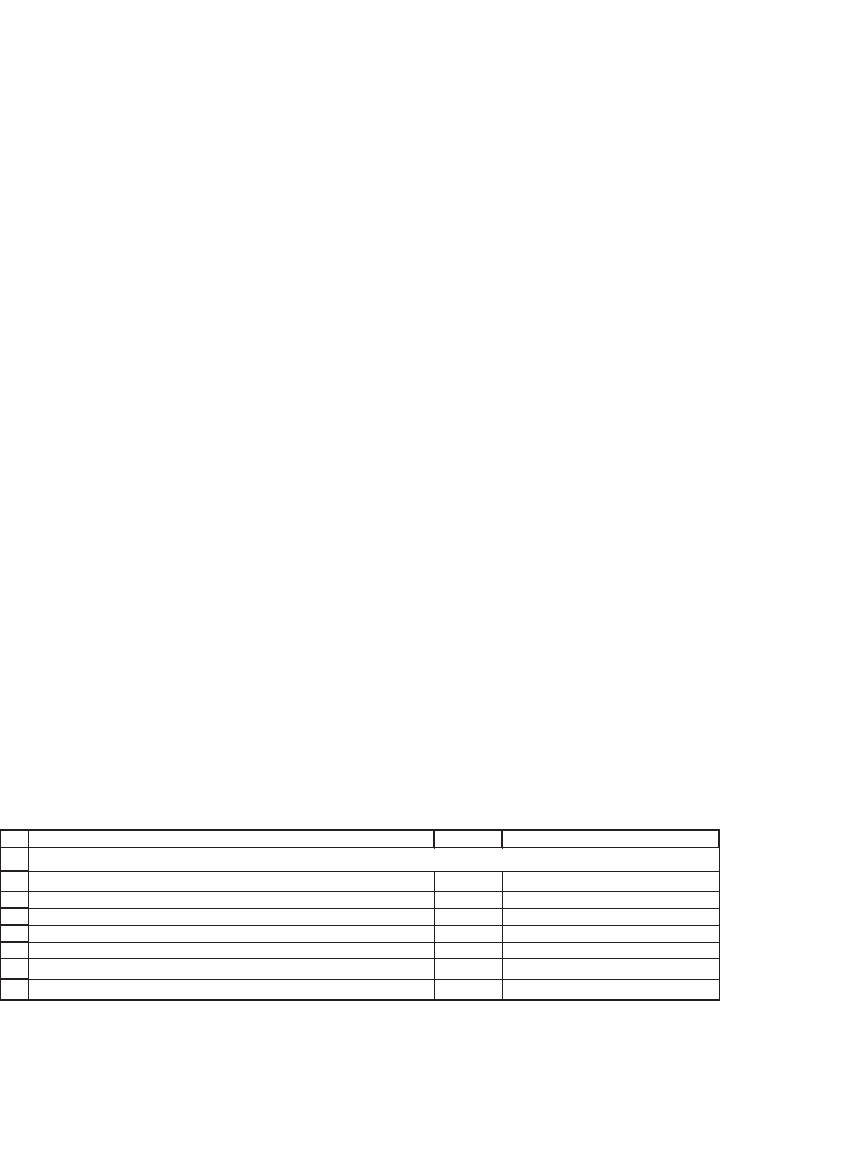
1
2
3
4
5
6
7
8
BA
Current stock price, S
0
83
Option time to maturity, T 0.5
Option exercise price, X 80
Interest rate, r 10%
Naive minimum option price, Max(S
0
-X,0)
3<
--
=MAX(B2-B4,0)
Proposition 1 lower bound on option price, Max(S
0
- Exp(-rT)X,0)
6.902 <
--
=MAX(B2-EXP(-B5*B3)*B4,0)
Proposition 1--Higher Lower Bounds for Call Prices
C
Proof Standard arbitrage proofs are built on the consideration of the
cash fl ows from a particular strategy. In this case the strategy is the
following:
434 Chapter 16
At time 0 (today):
•
Buy one share of the stock.
•
Borrow the present value (PV) of the option exercise price X.
•
Write a call on the option.
At time T:
•
Exercise the option if it is profi table to do so.
•
Repay the borrowed funds.
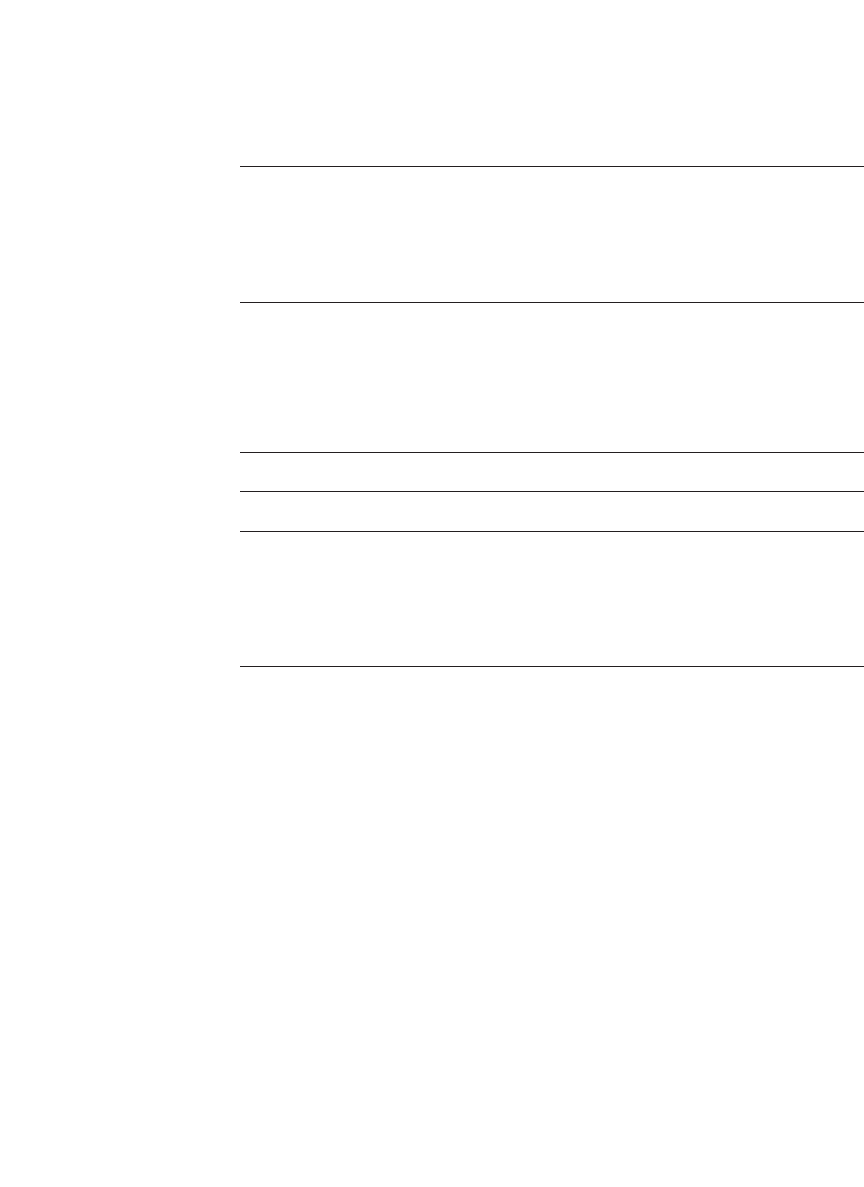
This strategy produces the following cash fl ow table:
Today
At Time T
Action Cash Flow
S
T
< XS
T
≥ X
Buy stock
−S
0
+S
T
+S
T
Borrow PV of X +Xe
−rT
−X −X
Write call
+C
0
0
−(S
T
− X)
Total
−S
0
+ Xe
−rT
+ C
0
S
T
− X ≤ 0
0
Note that at time T, the cash fl ow resulting from this option is either
negative (if the call is not exercised) or zero (when S
T
≥ X). Now a fi nan-
cial asset (in this case, the combination of purchasing a stock, borrowing
X, and writing a call) that has only nonpositive payoffs in the future must
have a positive initial cash fl ow; therefore,
CSXe CSXe
rT rT
00 00
0−+ > >−
−−
or
To fi nish the proof, we note that in no case can the value of a call be less
than zero. Thus we have that C
0
≥ max (S
0
− Xe
−rT
, 0), which proves the
proposition.
Proposition 1 has an immediate and very interesting consequence: In
many cases the early-exercise feature of an American call option is
worthless; therefore, an American call option can be valued as if it were
a European call. The precise conditions are the following:

435 An Introduction to Options
proposition 2 Consider an American call option written on a stock that
will not pay any dividends before the option’s expiration date T. Then it
is never optimal to exercise the option before its maturity.
Proof Suppose the holder of the option is considering exercising it
early, at some date t < T. The only reason to consider such early exercise
is that S
t
− X > 0, where S
t
is the price of the underlying stock at time t.
However, by Proposition 1 the market value of the option at time t
is at least S
t
− Xe
−r(T−t)
, where r is the risk-free rate of interest. Since
S
t
− Xe
−r(T−t)
> S
t
− X, it follows that the option’s holder is better off
selling the option in the market than exercising it.
Proposition 2 means that many American call options can be priced
as if they were European calls. Note that this statement is not true for
American puts, even if the underlying stock pays no dividends (we give
an example in Chapter 19).
proposition 3 (put bounds) The lower bound on the value of a put
option is
PXeS
rT
00
0≥−
−
max ( ),
Proof The proof of this proposition has the same form as the proof of
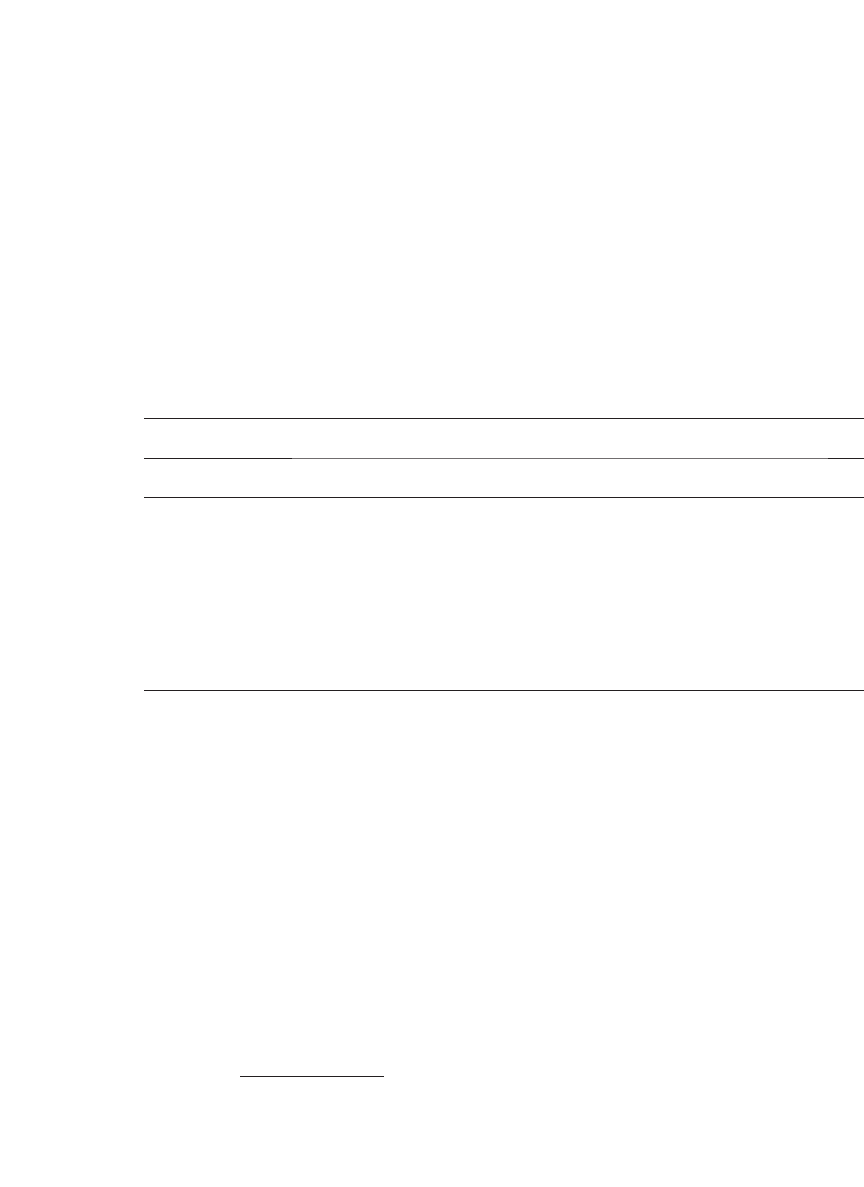
the previous theorem. We set up a table of strategies:
Today
At Time T
Action Cash Flow
S
T
< XS
T
≥ X
Short stock
+S
0
−S
T
−S
T
Lend PV of X −Xe
−rT
+X +X
Write put
+P
0
−(X − S
T
)
0
Total
P
0
+ S
0
− Xe
rT
0
X − S
T
≤ 0
Since the strategy has only negative or zero payoffs in the future, it must
have a positive cash fl ow today, so that we can conclude that
PXe S
rT
00
0−+≥
−
Combined with the fact that in no case can a put value be negative, this
proves the proposition.
436 Chapter 16
proposition 4 (put-call parity) Let C
0
be the price of a European call
with exercise price X written on a stock whose current price is S
0
. Let P
0
be the price of a European put on the same stock with the same exercise
price X. Suppose both put and call have exercise date T, and suppose
that the continuously compounded interest rate is r. Then,
CXe PS
rT
000
+=+
−
Proof The proof is similar in style to that of the two previous proposi-
tions. We consider a combination of the four assets (the put, the call, the
stock, and a bond), and show that the pricing relation must hold.
Today
At Time T
Action Cash Flow
S
T
< XS
T
≥ X
Buy call
−C
0
0
+S
T
− X
Buy a bond with payoff X
at time T
−Xe
−rT
XX
Write a put
+P
0
−(X − S
T
)
0
Short one share of the stock
+S
0
−S
T
−S
T
Total
−C
0
− Xe
−rT
+ P
0
+ S
0
00
Since the strategy has future payoffs that are zero no matter what
happens to the price of the stock, it follows that the initial cash fl ow of
the strategy must also be zero.
7
Therefore,
CXe PS
rT
000
0+−−=
−
which proves the proposition.
Put-call parity states that the stock price S
0
, the price of a call C
0
with
exercise price X and the price of a put P
0
with exercise price X, are
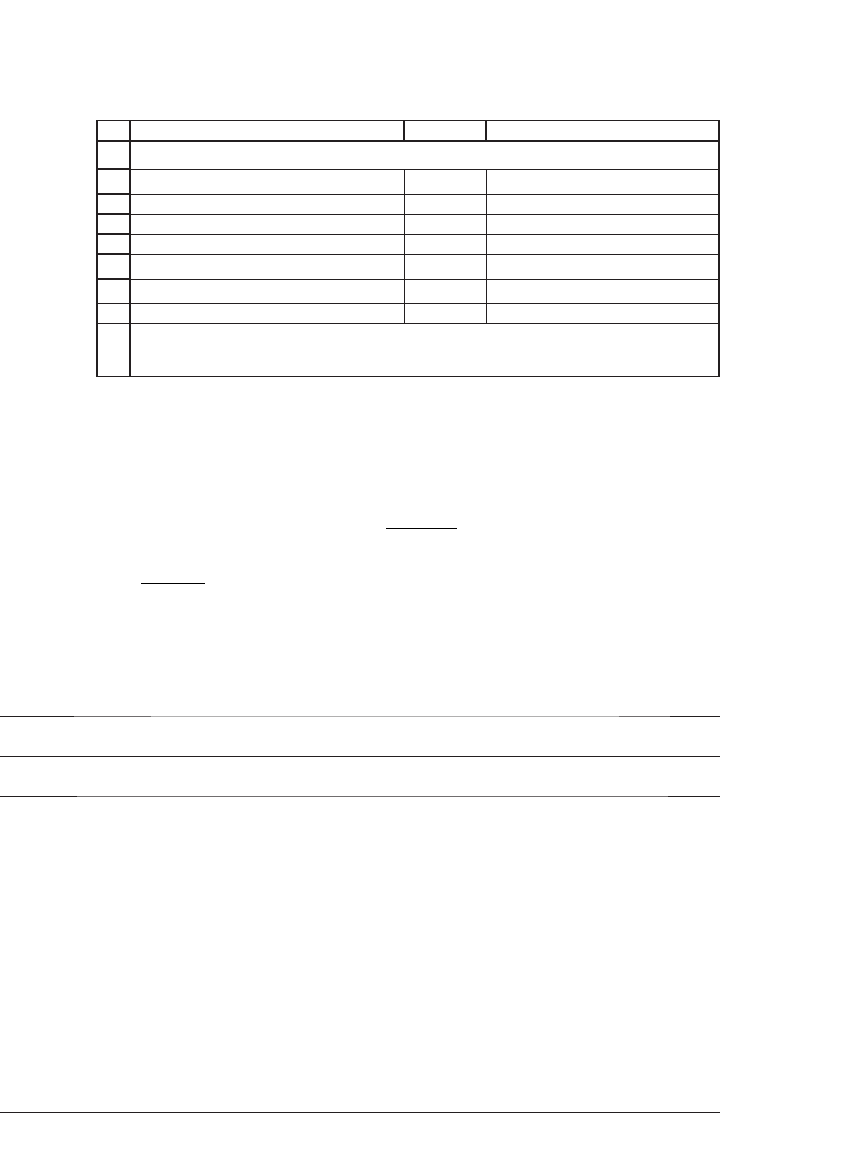
simultaneously determined with the interest rate r. Following is an illus-
tration which uses the call price C
0
, the option exercise price X, the
current stock price S
0
, and the interest rate r to compute the price of a
put with exercise price X and time to maturity T:
7. This is a fundamental fact of fi nance: If a fi nancial strategy has future payoffs that are
identically zero, then its current cost must also be zero. Likewise, if a fi nancial strategy
has future payoffs that are nonnegative, then its time-zero payoff must be negative
(that is, it must cost something).
437 An Introduction to Options
proposition 5 (call option price convexity) Consider three European
calls, all written on the same non-dividend-paying stock and with the
same expiration date T. We suppose that the exercise prices on the calls
are X
1
, X
2
, and X
3
, and denote the associated call prices by C
1
, C
2
, and
C
3
. We further assume that
X
XX
2
13
2
=
+
. Then,
C
CC
2
13
2
<
+
It follows that the call option price is a convex function of the exercise
price.
Proof To prove the proposition, we consider the following strategy:
At Time 0
At Time T
Action Cash Flow
S
T
< X
1
X
1
≤ S
T
< X
2
X
2
≤ S
T
< X
3
X
3
≤ S
T
Buy call with
exercise price
X
1
−C
1
0
S
T
− X
1
S
T
− X
1
S
T
− X
1
Buy call with
exercise price
X
3
−C
3
00 0
S
T
− X
3
Write two calls
with exercise
price X
2
+2C
2
00
−2(S
T
− X
2
) −2(S
T
− X
2
)
Total
2C
2
− C
1
− C
3
0
S
T
− X
1
≥ 02X
2
− X
1
− S
T
= X
3
− S
T
> 0
0
1
2
3
4
5
Option exercise price, X 60
Interest rate, r 10%
6
7
CBA
Current stock price, S
0
Option time to maturity, T 0.5
Call price, C
0
3
Put price, P
0
5.0738 <
--
=B6+B4*EXP(-B5*B3)-B2
Put-Call Parity
0
from the call price
terest rate r, the time to maturityT, and the ex e X.
55
8
This spreadsheet uses put-call parity to derive the put price P
9
C
0
, the in ercise pric
