Seely F.B. Analytical Mechanics for Engineers
Подождите немного. Документ загружается.

KINETICS
OF A TRANSLATING RIGID BODY 311
D,
is
assumed
to be
weightless
and frictionless.
Body
A
weighs
36
lb.,
B
weighs
60
lb.,
and C
weighs
120 lb.
The
coefficient of kinetic
friction
for A
and
the
plane
is
i,
and for B and
the
plane
it
is
i.
Find
(1)
the acceleration
of the
bodies,
(2)
the
tension,
T\,
in the
cord
between
A
and
B,
and
(3)
the
tension,
T
2
,
in the cord between
B and
C.
Solution. Each
body
has a
motion of translation.
Hence,
the
equations
of motion
for
a
translating
body
apply
to
each
body; namely,
(D
(2)
(3)
In
addition,
two
equations
involving
the coefficients
of friction for
bodies
A
and
B
are
needed, namely,
FA=INA,
(4)
(5)
Equation
(3)
is
not
needed in
determining
the
quantities
asked
for.
If the
action
lines of
NA
and
NB
were
desired,
however,
all
five
equations
would be
needed.
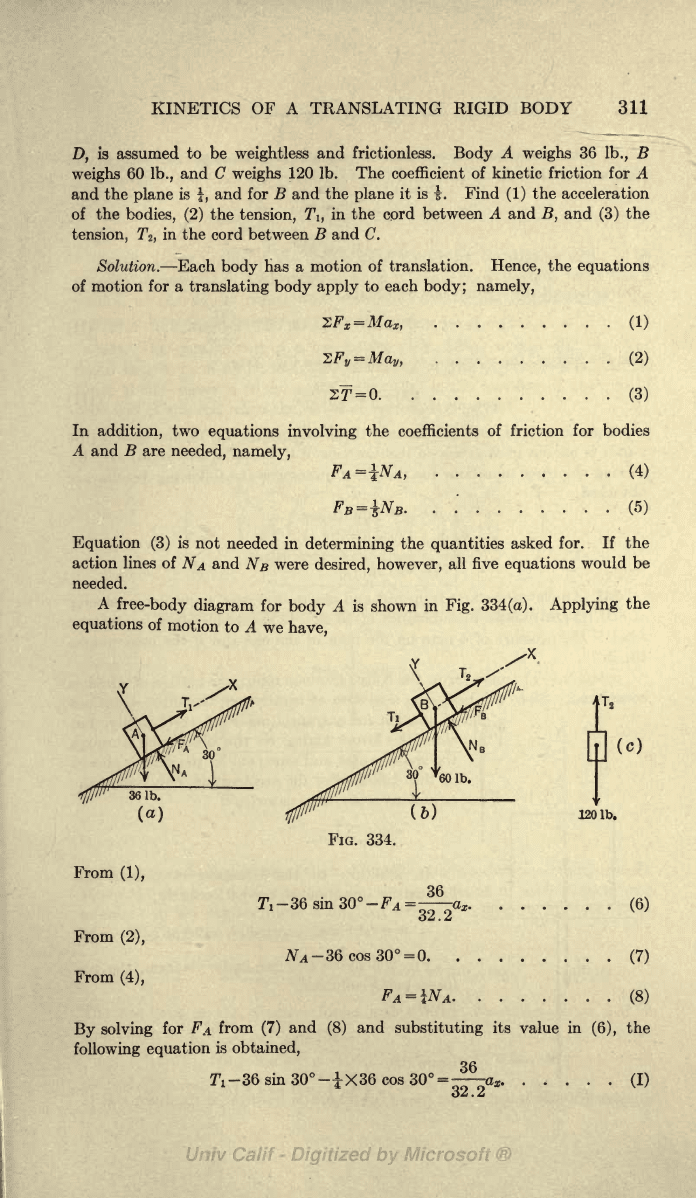
A
free-body diagram
for
body
A
is
shown
in
Fig.
334(a).
Applying
the
equations
of
motion
to A
we
have,
(c)
(6)
120
lb.
FIG.
334.
From
(1),
From
(2),
From
(4),
^-36sin30-F
A
=
a*.
AT
A
-36cos30=0.
(6)
(7)
(8)
By
solving
for
FA
from
(7)
and
(8)
and
substituting
its
value in
(6),
the
following
equation
is
obtained,
ri-36sin30-iX36cos30
=
(I)
312
FORCE, MASS,
AND ACCELERATION
In
like
manner,
by applying
the
equations
of
motion to
body
B,
the
following
equations
are obtained
(see
Fig. 334(6)
for
free-body
diagram):
Tt-Ti-FB-W
sin
30=
a*,
From the
last three
equations
we
obtain,
7
7
2
-7\-iX60
cos 30 -60
sin 30
(
60
32.2
ax
'
(ID
For the
body
C the
equation
of
motion
is
(see Fig.
334c),
32.2
(HI)
Equations
I, II,
and
III
contain
three
unknowns, 7\,
T
2
,
and a
x
or
a
(the
x-axis
is chosen
in each
case in the direction of
the
total
acceleration,
a).
By
solving
the three
equations
for the three unknowns
the
following
results are
obtained;
a
=
a
x
=
8.02ft./sec.
2
,
7*1
=
34.76
lb.,
7
1
2
=
90.1 lb.
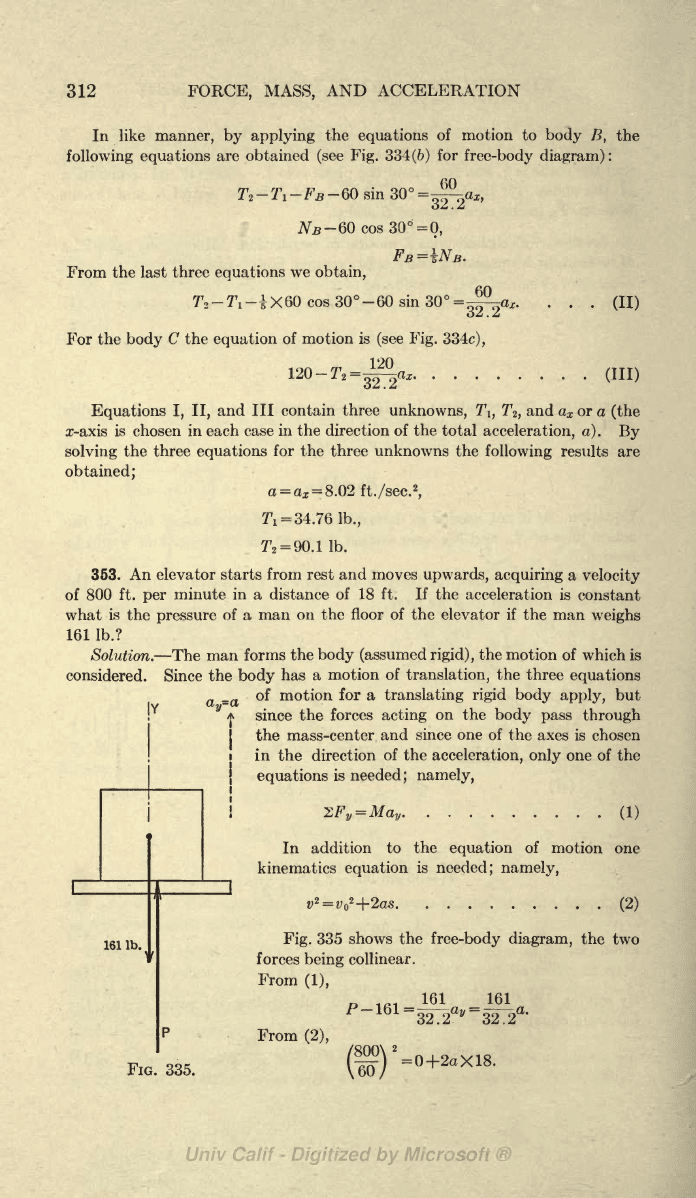
353.
An elevator starts
from
rest
and
moves
upwards,
acquiring
a
velocity
of 800
ft.
per
minute in a distance of 18 ft. If the
acceleration
is
constant
what
is
the
pressure
of a man
on the floor of the elevator if
the man
weighs
161 lb.?
Solution.
The
man
forms
the
body
(assumed
rigid),
the
motion
of
which
is
considered. Since
the
body
has
a
motion
of
translation,
the
three
equations
of motion
for a
translating rigid
body apply,
but
since
the forces
acting
on
the
body pass through
the mass-center
and
since one of
the
axes is
chosen
in
the direction
of the
acceleration,
only
one of
the
equations
is
needed;
namely,
|Y
t
161 lb.
In addition
to
the
equation
of
motion one
kinematics
equation
is
needed;
namely,
(2)
P
FIG.
335.
Fig.
335
shows the
free-body diagram,
the
two
forces
being
collinear.
From
(1),
P-161--
6
a
=
161
a
From
(2),

KINETICS
OF A
TRANSLATING
RIGID BODY
313
By
solving
the last
two
equations
the
following
results are
found,
a
=
4.93ft./sec.
2
,
P
=
185.7
Ib.
In the
above
problems
the
bodies have
rectilinear
translation
only.
The
parallel
or side
rod
of
a locomotive
when
running
on a
straight
track
has
a
motion
of
curvilinear
translation
with
reference
to the
engine
frame. Its
motion is considered
in
the
following
problem.
354. The
parallel
rod of a
locomotive
(Fig.
336a)
weighs
400
Ib.
The
crank
length,
r
t
,
is 15 in.
and the
radius,
r
2
,
of
the drivers is
3
ft.
If
the
speed
of
the
engine
is
50
miles
per
hour,
what
is the
reaction of
the
pin
at
each
end
of the
rod when
the
rod is
in its
lowest
position?
a)
=
9270
Ib.
C<

314
FORCE, MASS,
AND ACCELERATION
forces,
as shown
in
Fig.
336(6),
the
forces
will be in
equilibrium
(D'Alembert's.
principle)
.
It
will be observed
that the
forces form a
parallel
force
system.
The
equa-
tions of
equilibrium
for a
parallel
force
system
(Art. 49)
are,
(1)
(2)
Using
(1),
Using
(2),
Whence,
^1+^2-9270-400
=
0.
J
R
1
XJ-(9270+400)X-=0.
PROBLEMS
365.
A
man
who is
just
strong enough
to lift
a 150-lb.
weight
when
standing
on
the
ground
can
lift a 200-lb.
weight
from
the floor
of
an elevator when the
elevator
is
going
down with a certain
acceleration. What
is
the acceleration?
What
weight
can the
man
lift from
the
floor
of
the elevator
when
going up
with the same
acceleration?
Ans. a
=
8.05
ft.
/sec.
2
;
W
=
l20\b.
366. A 3-ton
cage
descending
a
shaft
with
a
speed
of 9
yd.
per
sec.
is
brought
to
rest
with a uniform
acceleration
in
a
distance
of
18
ft. What
is the
tension
in the cable
while the
cage
is
coming
to rest?
357.
The
dimensions
of
body
A
(Fig.
337)
are
3
ft.
by
2
ft.
by
4 ft.
and
its
weight
is 1000
Ib.
Assuming
that
the
body
will not
slip
on
the
carriage,
what
is
the
maximum
weight
that B
may
have without
causing
A to
tip
over
when
the acceleration
of the
carriage
is
8
ft.
per
sec.
2
?
The
pulley
D
is assumed
to be frictionless
and
weightless.
Ans.
201 Ib.
-D
-2^^
FIG. 337.
FIG.
338.
358.
A man
weighing
150
Ib.
leaves
his
room
by way
of a window which
is
50
ft. above
the
ground.
He
has
a
rope
that
is
long
enough
to reach
to
the
ground
but
it
can
support
a
force
only
of
125
Ib. What is
the
least
velocity
with
which
he
can reach the
ground?
KINETICS
OF
A ROTATING
RIGID BODY
315
369. Two
strings pass
over
a smooth
drum. On one side of
the
drum
the
strings
are attached
to a 50-lb.
weight;
on
the other side
one
string
is
attached
to a 40-lb.
weight
and
the other
string
to a
30-lb.
weight.
Find the accelera-
tion of the
weights
and the tension
in each of the
strings
during
motion.
Ans.
^30
=
25
lb.;
7
1
40
=
33.3
lb.;
a
=
5.37
ft./sec.
2
360. Steam is
shut off when
a train
running
at a
speed
of 30 miles
per
hour
reaches
a
0.4
per
cent
down-grade.
What
will be the
velocity
of the
train
after
100 sec.
if the train
resistance is 10
lb.
per
ton?
361. A small
car
(Fig.
338)
with its load
weighs
800 lb. and the center of
gravity, G,
of
the total
weight
is
5 ft.
from
the
track.
A
force
P of
120 lb. is
applied
to the
car
as shown.
Neglecting
the
friction
on the
track,
find the acceleration of
the car and the reactions
of the
track
on
each
pair
of wheels.
What
would be the reactions
on
the wheels if the force
P
acted
through
the
point
(7?
362.
A
door is
hung
on
a
track as shown
in
Fig.
339.
The
coefficient of friction
for
each
of
the shoes
(A
and
B)
and
the
track is
i
The door
weighs
FlG-
339
-
300
lb. What
force P is
required
to
give
the door an acceleration
of
4 ft.
per
sec.
2
? Find the
reactions of
the
shoes
on
the
track. How
far
will
the door
travel
in
2
sec.?
Ans.
P
=
112
lb.
ROTATION
146. Kinetics of a
Rotating
Rigid
Body.
If
a
rigid
body
is
acted
on
by
an unbalanced
force
system
that
produces
a
motion
of
rotation of the
body,
the
resulting
linear
accelerations
of
the
various
particles
of the
body
are
not
the
same,
but
vary directly
as
the distances of the
particles
from
the
axis of rotation
(Art. 133).
The
angular
velocities and accelerations of all the
particles,
how-
ever,
are the
same,
at
any
instant,
and
the
linear
acceleration
of
any
(and
every) particle
in
the
body
may
be
expressed
in
terms
of the common
angular
velocity,
,
and the
angular
acceleration,
a.
Thus,
in
Fig.
340
is
represented
a
physical
body
acted on
by
the
external
forces
P,
W,
and the reaction
(not
shown)
of the
axis at
0,
which
cause
the
body
to
rotate
with
an
angular
acceleration,
a,
about
an axis
through
0. Each
particle
of the
body
moves
on
a
cir-
cular
path
and the
linear
acceleration
of
any
particle
in the
body

316
FORCE,
MASS,
AND
ACCELERATION
has
two
components;
a
tangential
component,
a
t
,
and a
normal
component,
a
n
,
(Art. 118)
such
that,
a,
=
ra,
directed
tangent
to the
path
of
the
particle,
and
v
2
a
n
=
r
co
2
=
,
directed normal to the
path
of
the
particle,
towards
the center
of rotation.
in
which
co
and
a are the
angular velocity
and
angular
acceleration,
respectively,
of
the
particle
(and
also of the
body)
at the
instant,
v
is the
linear
velocity
of the
particle
at the
instant,
and
r is
the distance
of
the
particle
from
the axis
of rotation. The
accel-
eration
components
for
several
particles
are shown
in
Fig.
340.
From the
components
of
the
acceleration of
any particle
of the
body, expressed above,
the
components
of the resultant force
(effective
force)
required
to
produce
these
acceleration
components
may
now
be
found.
Thus,
if
m denotes the
mass of
the
particle,
then
from
Newton's second
law,
ma
t
=mra is the
tangential component
of the effective
force,
and
ma
n
=
mru>
2
is the normal
component
of the effective
force,
as
shown
in
Fig.
341.
Resultant
of
the
Effective
Forces. The
resultant
of the effective
forces
for the
whole
body
may
now
be
found. As
already
noted

KINETICS
OF A ROTATING
RIGID BODY
317
(see
footnote
under
Art.
144),
the effective
forces
form
a
coplanar
system
in
the
plane
of motion
and,
as indicated
in
Fig. 341,
the force
system
is non-concurrent.
According
to Art.
28,
the
resultant of
such a
coplanar
system
is either
a
force or a
couple.
In
either
case
the resultant
may
be
determined
completely
from
the summa-
tion
of
the
components
of
the forces
along
each of two
rect-
angular
axes
and the summation
of the moments
of
the forces
about some
point
in
the
plane
of
the forces.
Thus,
let the two
axes be
the T- and TV-axes as
shown
in
Fig.
341,
where
the
TV-axis
is
drawn
through
the center of
rotation, 0,
and the mass-center
of
the
body.
Let each of the
two
components,
mra and
rarco
2
,
of the
effective
force for
any
particle
be resolved
parallel
to
the
N- and
T-
axes
respectively.
Let
6 be
the
angle
(measured
clockwise from
the
TV-axis)
between the
radius, r,
to the
particle
and
the TV-axis.
Then,
from
Fig.
341,
the
component
of the effective force
parallel
to the TV-axis
is,
wrco
2
cos 9
mra sin
6,
and the
component
of the effective force
parallel
to the T-axis
is,
mra cos 6
mrai
2
sin
6.
Hence,
The
algebraic
sum of the
components
of the effective forces
parallel
to the TV-axis
=
S
(
mrco
2
cos 6 mra sin
6)
=
co
2
Smr
cos
da'Lmr sin
6
=
-Mrco
2
-0.
In
the above
equation
Srar
cos 6
represents
the moment
of
the
body
with
respect
to the T-axis
and
hence
may
be
replaced
by
Mr,
and Srar
sin
6
represents
the moment of the
body
with
respect
to
the TV-axis and hence
is zero since the
TV-axis
passes
through
the
mass-center
of the
body.
Further,
the
negative
sign
indicates
that
Mrco
2
is directed
from the mass-center
towards
the
center of
rotation.
In
like
manner,
The
algebraic
sum of the
components
of the
effective
forces
parallel
to
the
T-axis
=
2
(mra
cos 6
mru
2
sin
6)
=
a1l
mr cos
6
co
2
2mr sin
6

318
FORCE, MASS,
AND
ACCELERATION
The moment of
the
effective
force for
any
particle
about
the
center of
rotation, 0,
is
mra r
since the
normal
component,
rarco
2
,
of
the
effective force for
each
particle
passes
through
the axis of
rotation and
has,
therefore,
no
moment about
the center
0.
Hence,
taking
moments
about
0:
The
algebraic
sum of
the
moments of
the
effective
forces with
respect
to the
axis of
rotation
=S(rara-r)
where
Io
denotes
the
moment of
inertia of
the
body
with
respect
to the
axis
of rotation.
Since the
sum of
the
components,
in
any direction,
of the forces
of a
system
is
equal
to
the
component
of
their resultant in
the same
direction,
and since
the sum of the
moments of
the forces about
any
point
is
equal
to the
moment
of
their
resultant about the
same
point,
the
above results
may
be
summarized as
follows:
The
component
of the resultant
of the
effective
forces
parallel
to the
JV-axis
=
Mrco
2
.
The
component
of
the resultant of the
effective
forces
parallel
to
the
T-axis
=
Mra.
The
moment of the resultant
of the
effective
forces
about the
center
of rotation
=
/o.
If the
resultant
of
the effective forces
is a
force,
all of
the
above
expressions
are
required
to determine
it
completely. If,
however,
the resultant
is a
couple,
the first
two
expressions
reduce
to
zero,
in which
case
loa
expresses
the moment
of
the resultant
couple,
as will
be discussed
in
greater
detail later in
this article.
Relation
between
Effective
Forces and
External Forces.
The
relation
between
the resultant
of the effective forces for the whole
body
and the
resultant
of
the external forces which act
on
the
body
may
now
be found.
Since
the effective force
for a
particle
is the
resultant
of
all the forces
(both
internal
and
external)
which
act
on
the
particle,
the effective
forces for all the
particles
will
include
all of
the
internal forces
(exerted by
the
particles
among
them-
selves)
and
all of the external
forces.
Hence,
the
summation
of
the
Af-components
of
the effective
forces is
the
same
as
the
summa-

KINETICS
OF A
ROTATING
RIGID
BODY
319
tion of
the
N-components
of
the internal
forces
plus
the
summation
of the
AT-components
of
the external
forces,
and a similar
statement
applies
to the
^-components.
Therefore,
if F
denotes
a
force
and
T
the
moment of a
force,
the
relation
between the above
expressions
and the forces
acting
on the
particles
is
expressed by
the
following equations:
External
+
(
2F
n
)
Internal
(2F
t
)
External Internal
=
(2
To)
External
+(2
TO)
Internal
=
/0.
But the
algebraic
sum of the
components,
in
any
direction,
of
the internal
forces
and the
algebraic
sum of the
moments,
about
the axis of
rotation,
of the internal forces
are
zero,
since the
internal
forces
occur
in
pairs
of
equal,
collinear,
and
opposite
forces
(Newton's
third
law). Therefore,
the
resultant
of
the
effective forces
for the whole
body
and
the
resultant of
the
exter-
nal
forces,
only,
are
identical
(D'Alernbert's
principle).
Therefore,
in
the
above
equations,
the
expressions
which involve
the internal
forces
drop
out since as
just
stated,
Internal
=
0, (^F
t
)
Internal
=
0, (27o)
Internal
=
0.
Hence,
if F
is
now used
to denote an
external
force
only,
and T
is
used to
denote the
moment of an external
force,
the
equations
which
express
the
relations between
(1)
the external
forces,
(2)
the kinetic
properties
of the
body,
and
(3)
the
change
of motion
(the
equations
of
motion
for a
rotating rigid
body)
are,
It
will
be
noted
that
rco
2
and
ra
are the
normal
and
tangential
accelerations, respectively,
of
the mass-center
of the
body,
and
hence the first
two
of
the
above
equations may
be
written
ZF
n
=
Ma
n
and
2^,
=
Ma*.
Further,
if
the
body
rotates about an axis
through
the
mass-
center,
that
is,
if the
points
and G
coincide,
then the
right-

320
FORCE,
MASS,
AND
ACCELERATION
hand
members of the first
two
equations
are
equal
to
zero since
r
is
equal
to
zero,
and therefore
the
algebraic
sums of the
components
of the
external forces
in the
two
directions are
equal
to
zero.
Hence,
if a
rigid
body
rotates about an axis
through
its
mass-
center,
the
equation
of
motion
for the
body
is,
in which
S7
7
is the
algebraic
sum of the moments of
the external
forces
acting
on the
body
with
respect
to an axis
passing
through
the mass-center
(axis
of
rotation)
and
7
is
the moment
of
inertia
of the
body
with
respect
to the
same axis.
ILLUSTRATIVE
PROBLEMS
363.
The rod BCE
(Fig.
342a)
is made to oscillate
by
means
of
the
crank
A D
and
link
DC.
The
members
are
connected
by
smooth
pins
at
B, C,
and D.
The
rod BCE has a constant cross-section
and
weighs
16.1 Ib. In the
position
shown its
angular
velocity, co,
is 60
r.p.m.
and
its
angular
acceleration,
a,
is 40
rad./sec.
2
(see
Art. 134
for method of
finding
to
and
a).
Find the
pressure,
P,
of link
DC at
C
and
the
reaction,
R,
of the
pin
at
B.
FIG.
342.
.
Solution.
The
rod rotates about
the
center B. The
equations
of motion
for the
rod
are,
2/^=M?o>
2
(1)
(2)
(3)
