Roy K.K. Potential theory in applied geophysics

Подождите немного. Документ загружается.

6.1 Introduction 129

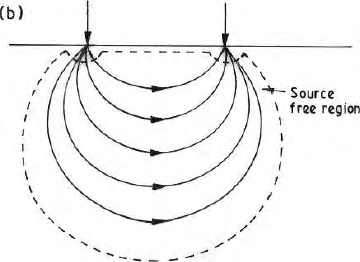
Fig. 6.2b. Source free region in a point source generated bipole ﬁeld

expressed in farad/meter. In magnetostatics, the magnetic induction is con-

nected to the magnetic ﬁeld through a scalar µ (5.13) i.e., the magnetic per-

meability. It is expressed in henry per meter. In direct current ﬂow ﬁeld, the

current density, i.e., the current per unit area is connected to the electric ﬁeld

through a scalar σ (6.9), the electrical conductivity. It is ex p ressed in mho/m

or Siemen. These scalars become tensors for an anisotropic medium. These

three equations in conjunction with the Maxwell’s (12.62 to 12.66) equations

form the basis of electromagnetic theory.

In direct current ﬂow ﬁeld, the physical property, we try to measure is elec-

trical conductivity or electrical resistivity. Electrical resistivity is reciprocal of

electrical conductivity. Of all the physical properties of the earth, measured

by geophysicists, electrical conductivity is the most sensitive parameter. A

little perturbation, in a medium through which current ﬂows, can change the

value of the electrical conductivity by several order of magnitude. The ratio

of the extreme values of resistivity or conductivity is of the order of 10

more.

The boundary conditions in direct curre nt ﬂow ﬁeld are (i) potential must

be continuous across the boundary i.e., φ

= φ

and (ii) normal component of

the current density must be continuous across the boundary,i.e., J

= J

The boundary value problems also must satisfy (i) Dirichlet (ii) Neumann or

(iii) mixed, Robin or Cauchy’s boundar y conditions.

Since this book includes elaborate treatments on direct current potential

and ﬁeld theory in Chap. 7 and Chap. 8 only a very few points, in preliminary

level, are discussed in this chapter. Application of direct current methods in

geophysical exploration is beyond the scope of this b ook and are available

in Alpin et al (1966), Keller and Frieschknecht (1966), Bhattacharya and

Patra (1968), Koefoed (1979), Zhdanov and Keller (1994). Advanced theories

however are given in Chaps. 8 and 15. Some treatments are also available in

Chaps. 9 and 11.

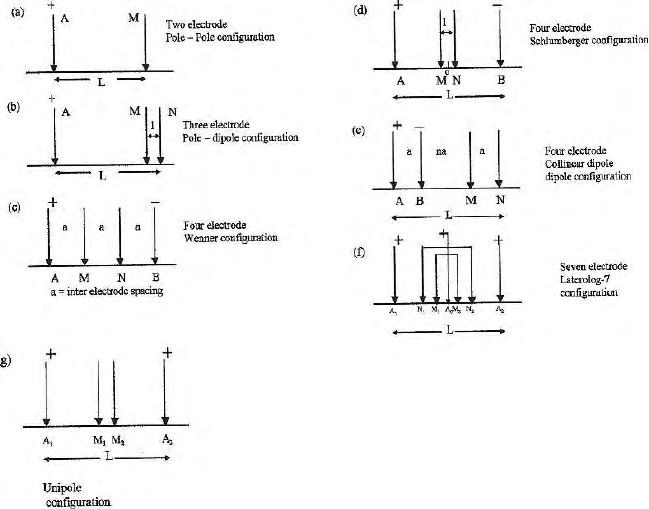
130 6 Direct Current Flow Field

Fig. 6.3. (a) Two electrode conﬁguration (normal log arrangement) with one cur-

rent electrode A and one potential electrode B; the other current electrode for return

current path and the other potential electrode are far away from this electrode set

up; (b) Three electrode conﬁguration (Lateral log arrangement) with one current

and two closely spaced potential electrodes, the other return current electrode is

placed far away from this set up; ( c) Four electrode conﬁguration (Wenner elec-

trode arrangement); A and B are two current electrodes and M and N are potential

electrodes; these electrodes are equidistant from one another; (d) Four electrode con-

ﬁguration (Schlumberger electrode arrangement), A and B are current electrodes,

closely spaced M and N are potential electrodes; (e) Four electrode conﬁguration

(Collinear dipole-dipole conﬁguration) with current dipole AB may have wide sep-

aration from potential dipole MN; (f) Seven electrode conﬁguration (Latero log

arrangement) with central focussing current electrode, two bucking current elec-

trodea A

and A

; two pairs of potential electrodes M

and M

; the return

current electrode is far away from this set up; (g) Four electrode conﬁguration

(Unipole method); here two current electrodes are sources for current focussing; two

closely spaced potential electrodes are used to measure pure anomaly; return current

electrode is far away from this set up

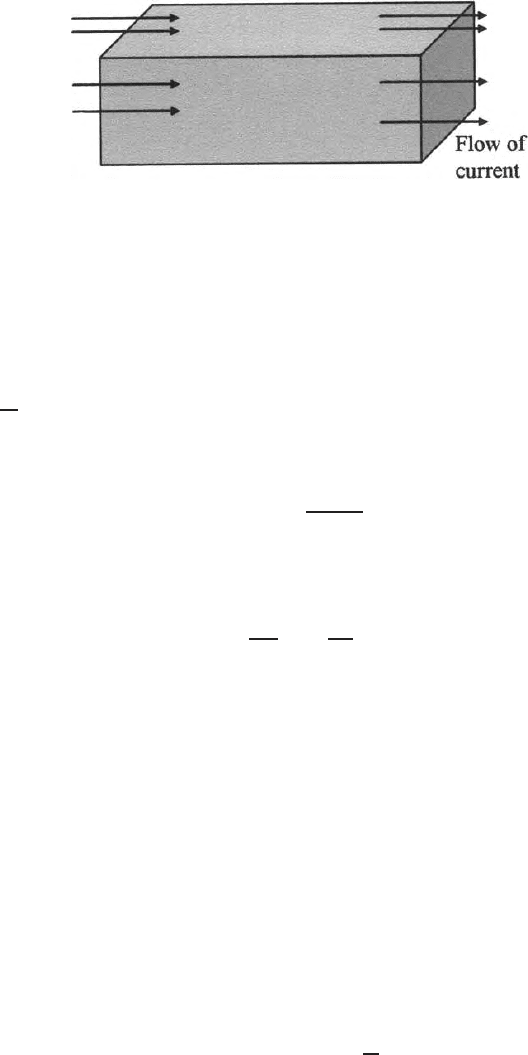
6.3 Diﬀerential form of the Ohm’s Law 131

Fig. 6.4. Flow of direct current through two opposite faces of a rectangular

parallelepiped

6.2 Direct Current Flow

In direct current ﬂow ﬁeld, the ﬂow of current is stationary. Let a constant

current I ﬂows through a homogeneous and isotropic medium.

We know that current I can be taken as the rate of ﬂow of charge i.e.

. At any point in the medium, I cannot be deﬁned but J can be deﬁned.

A small area ∆S is chosen normal to the ﬂow of current. The amount of

current ﬂows through the face in a time ∆t is given by (Fig. 6.4)

∆I = q

∆S.∆l

∆t

(6.1)

where ∆l is the distance traveled by the charges and q

is the volume density

of charge. From (6.1) we get

∆I

∆S

∆l

∆t

(6.2)

⇒



J=q

v (6.3)

where



J is the current density and v is the velocity. The expression for the

current is given by





J.n.ds (6.4)

where n is the normal vector.

6.3 Diﬀerential form of the Ohm’s Law

Ohm’s law is deﬁned as temperature remaining same the potential generated

between the two points of a conductor has direct proportionality with the

current ﬂowing through the ground.

So I = (φ

− φ

)

(6.5)

132 6 Direct Current Flow Field

where I is the current ﬂowing through this medium φ

and φ

are the potentials

at two points in the medium and R, the constant of proportionately is the

resistance oﬀered by the ground. Here

R = ρ

(6.6)

where ρ is the speciﬁc resistivity, l is the length and A is the cross sectional

area for the ﬂow of current. Speciﬁc resistivity of a medium is deﬁned as

the r esistivity oﬀered by the two opposite faces of an unit cube. The unit

of resistance is Ohm. The unit of resistivity is Ohm-meter. The reciprocal

of resistance is conductance (C). Its unit is mho. The reciprocal of resistiv-

ity is conductivity (σ). Its unit is mho/meter. From (6.5 and 6.6), we can

write

C = σ

= σ

∆S

∆l

. (6.7)

Here ∆I =

∆S

∆I

. (−∆φ) .σ (6.8)

⇒

∆I

∆S

= −

∆φ

∆l

.σ

⇒



J=σ



E. (6.9)

6.4 E q uat i on of Continuity

Since, the stationary electric ﬁelds are conservative, the electric ﬁeld is

expressed as the gradient of a scalar p otential (Φ) i.e.,

E=−∇Φ. (6.10)

Combining equation (6.9) with equation (6.10), we get

J=−σ∇Φ. (6.11)

Applying the principle of conservation of charge over a volume, which states

that charges can b e neither created nor destroyed, although equal amount of

positive and negative charges may be simultaneously created. From (6.8) we

can write

I =





→

n.ds (6.12)

and this outward ﬂow of positive charge must be balanced by a decrease of

positive charge (or an increase of negative charge) within a closed surface. If

the charge inside a clos ed surface is denoted by q

, then the rate of decrease

is – dq

/dt and the principle of conservation of charge requires

6.5 Anisotropy in Electrical Conductivity 133

I =





J.n.ds = −

. (6.13)

The negative sign indicates the direction of the current ﬂow. The (6.13) is

the continuity equation. By changing the surface integral to a volume integral

using divergence theorem, we get



J.ds =



vol

(∇.J) dv. (6.14)

Writing q



qdv,whereq

is the volume density of charge, we can write



vol

(∇.J) dv = −



vol

dv (6.15)

where q

is volume charge density. For outﬂow of current through a volume,

the derivative can be written as a partial derivative and the (6.15) becomes



vol

(∇.J) dv =



vol

−

∂q

∂t

dv (6.16)

Since, the expression is true for any volume, however small, it is true for an

incremental volume,

(∇.J) ∆v = −

∂q

∂t

∆v (6.17)

and the point form of the continuity is

(∇.J) = −

∂q

∂t

. (6.18)

∇.J=

∂q

∂t

δ (x) δ (y) δ (z) . (6.19)

6.5 Anisotropy in Electrical Conductivity

For an homogen eous and isotropic medium, the current density J and the

electric ﬁeld E are assumed to be in the same plane. If the electric ﬁeld (E

)

and conductivity (σ

) are along the x-direction, then curre nt density along

the x direction is J

. In general, however, not only the ﬁeld E

but also

the ﬁelds E

and E

may give rise to current densities in the x-direction in an

anisotropic medium. If the additional, current densities are prop ortional to the

ﬁelds E

and E

,thenσ

and σ

are proportionality constants respectively.

The total current density in the x-direction is the sum of these three terms.

In general, therefore, we can write,

134 6 Direct Current Flow Field

= σ

+ σ

= σ

+ σ

(6.20)

= σ

+ σ

The conductivity of a medium (σ) must be a tensor of rank 2, which in Carte-

sian coordinates will h ave the nine comp onents:

σ =

⎛

⎝

⎞

⎠

. (6.21)

If the conductivity tensor is symmetric, the oﬀ-diagonal terms will have sym-

metrically equal values i.e., σ

= σ

, and so on. If two of the coordinate

directions ar e selected to lie in the direction of maximum and minimum con-

ductivity, (the principal directions of the conductivity tensor), the oﬀ-diagonal

terms will become zero and σ can be shown as a diagonal matrix.

σ =

⎛

⎝

0 σ

00σ

⎞

⎠

. (6.22)

In isotropic materials, the three principal values of conductivity are al l equal

and in eﬀect, conductivity becomes a scalar quantity. In isotropic materials,

the electric ﬁeld vector and the current density vector are collinear, i.e. cur-

rent ﬂow, is along the di rection of applied electric ﬁeld. In anisotropic media

(the equipotential surfaces are no longer normal to the direction of current

ﬂow). Her e three principal values of the conductivity tensor are not equal.

Coincidence of directions occur only when the electric ﬁeld is directed along

one of the principal directions of th e tensor conductivity.

6.6 Potential at a Point due to a Point Source

Potential at a point due to a point sour ce of current I at a distance ‘r’ and in

a homogeneous and isotropic medium of resistivity ρ can be derived from the

solution of Laplace equation in a spherical coordinate (see Chap. 7) as

φ =

4π

. (6.23)

Therefore in a homogeneous and isotropic medium, the current lines will be

radial and equipotential lines will b e circular Fig. 6.1. The nature of current

and equipotential lines due to (i) a source and a sink of strength +I and −I

(ii) two sources of strength +I and +I are shown in Figs. 6.5 and 6.6 respec-

tively. The nature of the current lines and ﬁeld lines for bipole and dipole

ﬁelds for +1 and −1 are respectively given in Figs. 6.5 and 6.7.

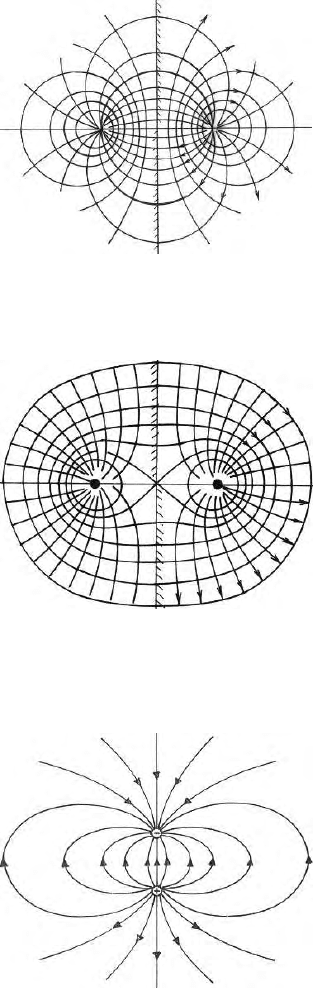
6.6 Potential at a Point due to a Point Source 135

Fig. 6.5. Current lines and equipotential lines due to a current source and a sink

Fig. 6.6. Field lines and equipotential lines due to two sources placed at a certain

distence

Fig. 6.7. Field lines and current lnes due to two closely spaced source and sink

136 6 Direct Current Flow Field

Potential at a point due to a point source at a distance r on the surface of

the earth is

φ =

Iρ

2π

(6.24)

since the solid angle subtended by a point c u rrent electrode on air earth

boundary is 2π. For Wenner electrode conﬁguration, the potential diﬀerence

between th e two potential electrodes M and N due to two current electrodes

placed at A and B is given by (Fig. 6.3 c and d)

∆φ =

Iρ

2π



−



−



−



(6.25)

Iρ

2π

where ‘a’ is the distance between the electrodes. Here for Wenner

conﬁguration AM = MN = NB = a. Therefore

ρ =2πa

∆φ

(6.26)

where 2πa is the geometric factor for Wenner conﬁguration. Similarly geo-

metric factors for all the electrode conﬁgurations cited, can be determined.

Numerical value of a geometric factor increases with electro de separation, the

farthest distance between the two active electrodes. For an inhomogeneous

medium, the resistivity ρ in (6.26) will change to ρ

, the apparent resistivity.

Apparent resistivity is deﬁned as the true resistivity of a ﬁctitious homogenous

medium when the response from an inhomogenous earth is same as that from

a ﬁctitious homogenous medium. For Schlumberger electrode conﬁguration

the expression for the apparent resistivity is

=(π/4)((L

− l

)/l)(∆Φ/I) (6.27)

where L, the distance between the two current electrodes, the distance b etween

two potential electrodes is l, I, the current ﬂowing through the medium and

∆Φ the potential diﬀerence measured between the two potential electrodes.

We deﬁned Geometric factor K =

2π

(

−

)

−

(

−

)

as the exact

geometic factor. We brought the idea of approximate geometric factors while

discussing the geometric factors for dc dipole conﬁgurations. It has a dimen-

sion of length for some cases. Geometric factor can b e variable in the case of

laterolog - 7 conﬁguration and can be negative for certain zones of parallel

dipole and wenner gamma or collinear dipole-dipole conﬁgurations. In general

geomertric factor is mostly an electrode separation dependent quantity.

6.7 Potential for Line Electrode Conﬁguration

Let us consider an inﬁnitely long line electrode through which a current I per

unit length is being sent through the half space (Parasnis 1965).

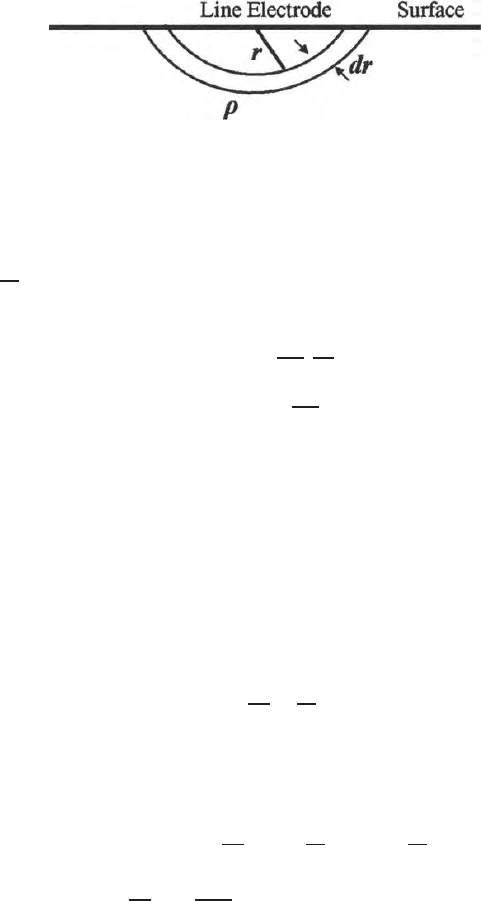
6.7 Potential for Line Electrode Conﬁguration 137

Fig. 6.8. Cylindrical annular space for a long line electrode

Figure 6.8 shows an annular semi cylin d er of unit length with the electrode

at the axis and having internal and external radii r and r + dr. If ρ is the

resistivity of the homog eneous ground; resistance of the annular cylindrical is

dR = ρ

πr

. Since current is passing out o f the cylindrical annular shell, the

potential drop across it is

dφ = −IdR = −

. (6.28)

Integrating φ = −

ln r + C. (6.29)

Therefore, potential at a po int due to a line electrode is a logarithmic p o ten-

tial. For φ =0atr=∞,wehaveC=C

∞

where C

∞

is an inﬁnite constant.

Therefore the potential will be inﬁnite at inﬁnite distances. If φ = 0 at r = 1,

then C = 0. Here potential will be positive for r less than 1 and negative for

r greater than 1. Potential will be −∞ as r →∞.Letr

and r

be the dis-

tances of an observation point from two inﬁnitely long line electrodes. These

electrodes are a source and a sink.

The potential from p o sitive and negative electrodes will b e of opposite

sign. If we choose C = C∞, the total potential at the point will be

φ =

Iρ

. (6.30)

The potential diﬀerence between the two points P1 and P2 in a ﬁeld created

by two line electrodes is given by (Fig. 6.9)

∆φ = φ

− φ

Iρ







− In





(6.31)

Iρ





(6.32)

since the inﬁnity constants cancel each other . The potential expressed in

(6.32) is ﬁnite at all the ﬁnite distances and tends to zero when both r

and r

tends to inﬁnity. Potentialis are also zero when r

, i.e., along

the vertical plane midway between the source and sink. Surface potential is

given by

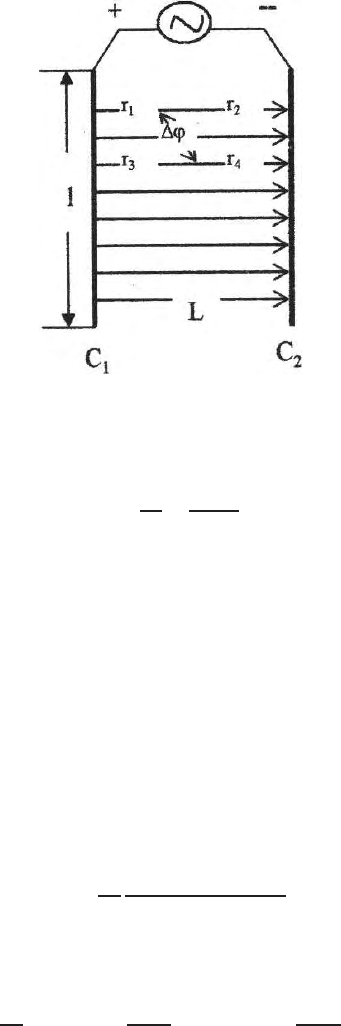
138 6 Direct Current Flow Field

Fig. 6.9. Shows the potential diﬀerence measured betw een the two points of obser-

vation P1 and P2 between a line source and a line sink C

and C

each of length l

and are planted on the ground at a distance L

φ =

Iρ

L −x

L + x

(6.33)

where 2L is the distance between the two electrodes and x is measured from

the midpoints of C

6.7.1 Potential due to a Finite Line Electro de

A line electrode of length 2b through which current I per unit length is being

supplied to a homogeneous ground of resistivity ρ (Fig.6.10).Wedetermine

the potential at a point P whose perpendicular distance from the electrode i s

x and which is situat ed on a proﬁle of measurement at a distance y from the

centre O of the electrode.

A small element of the line electrode, having a length dλ,atadistanceλ

from O can be treated to be a point electrode through which a current I dλ

is being propagated to the ground. Potential at P will then be

dφ =

Iρ

2π

dλ

+(λ −y)

}

1/2

. (6.34)

Integrating between the limits −b and +b, the potential of the entire line

electrode will be

φ =

Iρ

2π



sin h

−1



b −y



+sinh

−1



b + y



. (6.35)