Qiu X.G. (Ed.) High Temperature Superconductors

Подождите немного. Документ загружается.

130 High-temperature superconductors

43X

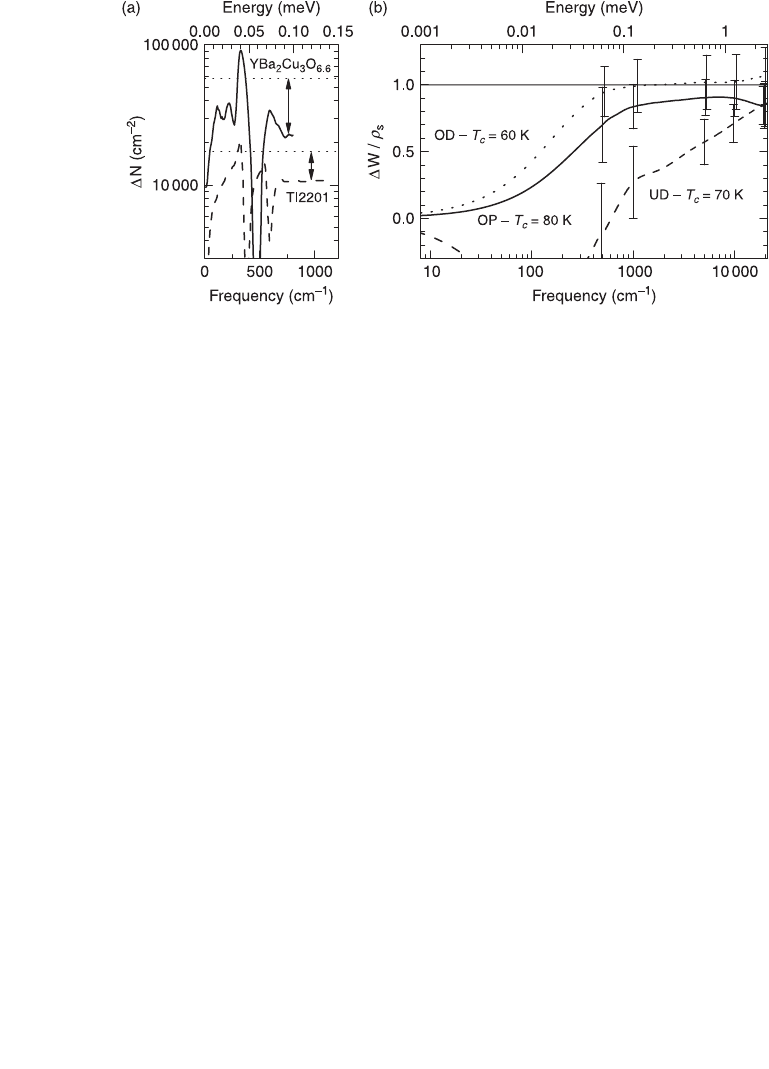
Fig. 3.14 shows the difference in the spectral weights of the normal and the

superconducting states normalized to the superﬂuid weight for Bi2212. In

optimally and overdoped Bi2212, the FGT sum rule is fulﬁlled and, at a ﬁrst

glance, variations in the kinetic energy are too small to be detected. The situation

is radically different in underdoped Bi2212. In this case, at 1 eV – a value

representative of the bandwidth – the FGT sum rule is still not satisﬁed. The fact

that ∆W is smaller than

implies that the kinetic energy decreases in the

superconducting state. However here, on the ab-plane, the gain in kinetic energy

is about 1 meV per Cu atom, much larger than the 0.25 meV/Cu condensation

energy measured by Loram et al. (2001). These results show that an electronic

mechanism, such as kinetic energy driven superconductivity (Hirsch, 2000), must

be considered.

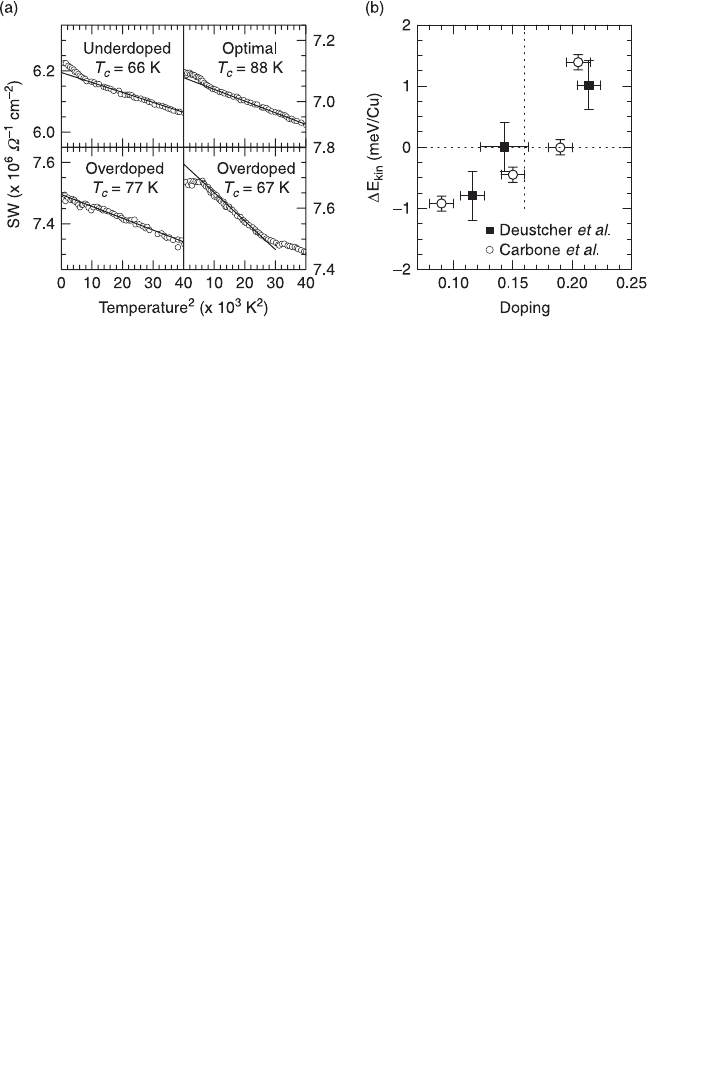
Another way to look at the kinetic energy change from the spectral weight

is shown in Fig. 3.15 (a) (Carbone et al., 2006). These ﬁgures show the total

spectral weight (including the

function in the superconducting state) integrated

from 0 to 10 000 cm

–1

for Bi2212 from underdoped to overdoped as a function of

the temperature squared. In all cases the spectral weight increases (almost as a T

behavior as shown by the straight line) in the normal state. This means that the

kinetic energy is decreasing with the temperature. Three different situations

3.14 (a) FGT sum rule violation along the c-axis of optimally doped

Tl2201 and underdoped YBCO shown by comparing the difference

between the normal and superconducting state charge density

(equivalent to the RSW) as a function of the upper cut-off frequency.

The dotted lines are the values for the superﬂuid weight obtained from

[adapted from Basov et al. (2001)]. (b) The difference between the

normal and superconducting state RSW as a function of the upper cut-

off frequency for Bi2212 ﬁlms from the underdoped to the overdoped

regime. The curves are normalized to the superﬂuid weight obtained

from

[adapted from Santander-Syro et al. (2004)].



Optical conductivity of high-temperature superconductors 131

43X

appear upon crossing the superconducting transition. In the underdoped

= 66 K) and optimally doped T

= 88 K the spectral weight increases faster

below T

. This corresponds to an extra decrease of kinetic energy as discussed

above. For the overdoped T

= 77 K sample, no change is seen at T

, meaning

neither gain nor loss of kinetic energy. Finally, the overdoped T

= 67 K shows a

spectral weight decreasing in the superconducting state. This is the ‘conventional’

BCS behavior of kinetic energy increasing below T

. This change of regime had

been remarked on a little earlier by Deutscher et al. (2005) as summarized in

Fig. 3.15 (b). Coming from the underdoped to overdoped the kinetic energy gain

at T

becomes a kinetic energy loss. This is an effect that must be taken into

account when describing superconductivity in cuprates. Deutscher and co-workers

propose a Bose-Einstein condensate to BCS regime crossover near optimal

doping, but this is still an open topic.

A brief, but necessary ﬁnal remark on the sum rule is that much ado was made

about the microscopic origins for the temperature T

evolution of the optical E

kin

in the normal state. It turned out to be a non sequitur (Norman et al., 2007). This

shape depends more on the choice of the cut-off frequency and is not reproducible

from sample to sample. However, the kinetic energy changes calculated from

optics in the formation of the superconducting condensate remain valid (Marsiglio

et al., 2008).

3.15 (a) The four panels show the spectral weight from 0 (including

the superﬂuid weight in the superconducting state) to 10 000 cm

–1

as a function of T

in Bi2212 at several dopings corresponding to the

indicated T

’s. The straight lines are guides to the eye and help to

visualize the changes in the RSW at T

[adapted from Carbone et al.

(2006)]. (b) The variation of the kinetic energy for several cuprates as a

function of doping [adapted from Deutscher et al. (2005)]. The vertical

dotted line indicates the position of optimal doping.

Panel (b) also includes data calculated from panel (a).



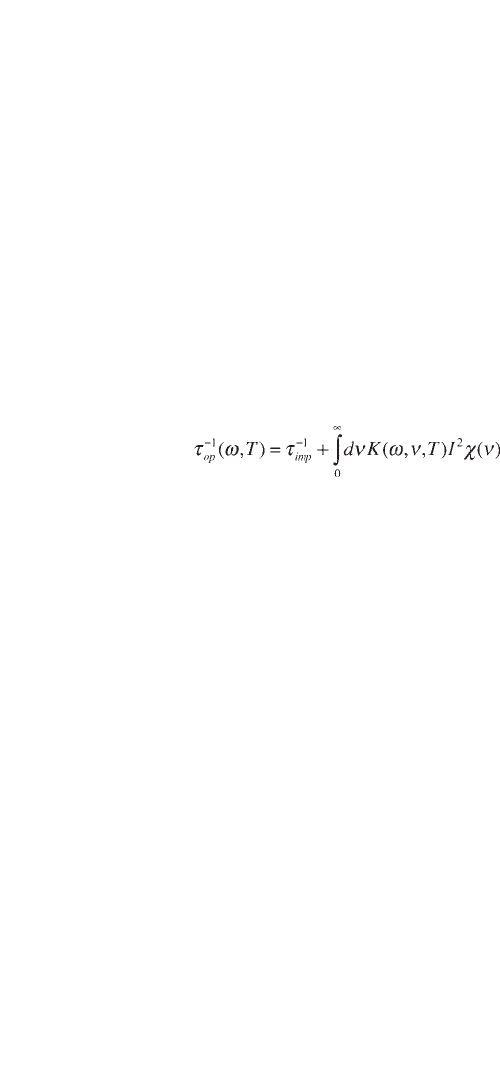
132 High-temperature superconductors

43X

3.5.3 The bosonic mode

The binding of a Cooper pair happens through the exchange of a boson by two

electrons. BCS theory leans on the isotope effect to assign a phonon to this boson.

It then uses an average value (e.g. the Debye frequency) for the phonon spectrum

to calculate all superconducting properties. Eliashberg theory extended the BCS

approach by introducing the full phonon spectrum

) into the calculations

(Carbotte, 1990). This function contains all the information pertinent to

superconductivity. Yet the justiﬁcation for the phonon exchange is based on the

isotope effect present in classical superconductors. In principle, any boson and its

generic I

(

) spectral response could lead to pairing.

In his review, Carbotte showed that the

) has been extensively and

successfully obtained for BCS superconductors by inverting tunneling data.

Farnworth and Timusk (1976) showed that the same function can be extracted

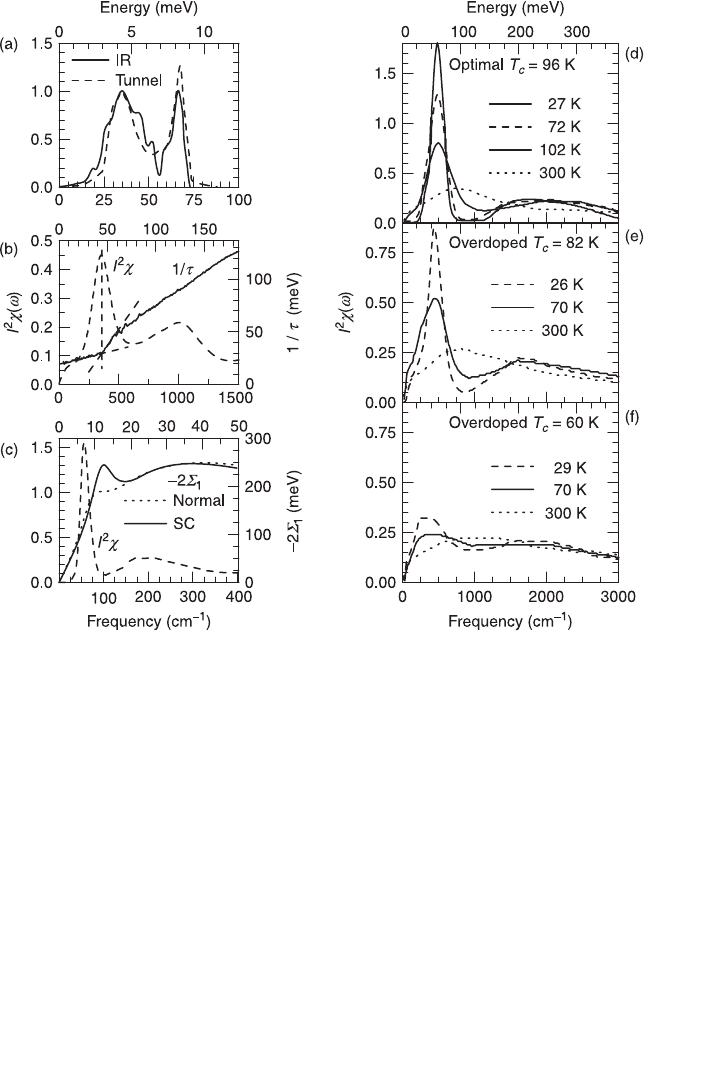
from the far-infrared response of superconducting Pb, as in Fig. 3.16 (a). This

possibility comes from the relation between the scattering rate and the bosonic

function proposed by Shulga et al. (1991):

[3.13]

where

–1

imp

is a dirty limit impurity scattering, and K(

,T) is a kernel that

depends on the physics of the system. It can be as simple as (

–

) for a metal at

zero temperature. Kernels for ﬁnite temperature, s-wave and d-wave are also

available (Schachinger et al., 2008). The ﬁrst attempts on solving Eq. 3.13 were

carried out by Carbotte et al. (1999) on YBCO. Their approach was based on

calculating the second derivative of 1/

(Marsiglio et al., 1988). Dordevic et al.

(2005) pushed the analysis further using inverse theory and obtained smoother

solutions for I

. These solutions however, often contain negative unphysical

regions. Schachinger et al. (2006) demonstrated that the use of maximum entropy

techniques results in a positive deﬁned I

Figure 3.16 (b) shows I

for optimally doped PCCO as well as the scattering

rate used in the inversion (Schachinger et al., 2008). This ﬁgure illustrates very

nicely that peaks in the bosonic spectral function correspond to a change in the slope

of the scattering rate. Even subtle changes as the one observed around 125 meV

produce marked features in I

. Note that the shoulder in 1/

, around 75 meV,

corresponds to a minimum rather than a peak in I

. Remembering that 1/

is related

to the imaginary part of the self-energy, panel (c) shows an interesting property of

the real part of the self-energy. The dashed curve is the I

function calculated for

Hg1201 at 29 K (Yang et al., 2009). The solid line is the real part of the optical self-

energy in the superconducting state, recalculated from the I

shown. The dotted

line uses the same I

to calculate the normal state response but, as it is in the normal

state, it requires a different kernel in Eq. 3.13. The peak around 100 meV in the

superconducting curve disappears above T

. In the superconducting state, this peak



Optical conductivity of high-temperature superconductors 133

43X

is located at

Ω

+ ∆, where

Ω

is the position of the sharp peak in the bosonic spectrum

and ∆ is the superconducting gap. This is a direct experimental way to determine the

presence of low frequency structures in the bosonic spectrum.

Panels (d) to (f) show how the bosonic function evolves with temperature and

doping in Bi2212 (Hwang et al., 2007). These ﬁgures indicate that the resonance

peak is built at the expense of spectral weight lost in the continuum and that it

survives in the normal state. The question is then, What are the underlying bosonic

excitations producing this spectrum?

3.16 Panel (a) shows the

) Eliashberg function obtained for Pb

from tunnel and infrared data [adapted from Farnworth and Timusk

(1976)]. Panel (b) compares the features observed in the scattering rate

of PCCO to the strong signatures in the bosonic function [adapted from

Schachinger et al. (2008)]. Panel (c) compares the bosonic function

to the mass enhancement factor in Hg1201 [adapted from Yang et al.

(2009)]. Panels (d) to (f) show the evolution of I

(

) in Bi2212 in the

optimal and overdoped regimes for different temperatures [adapted

from Hwang et al. (2007)].



134 High-temperature superconductors

43X

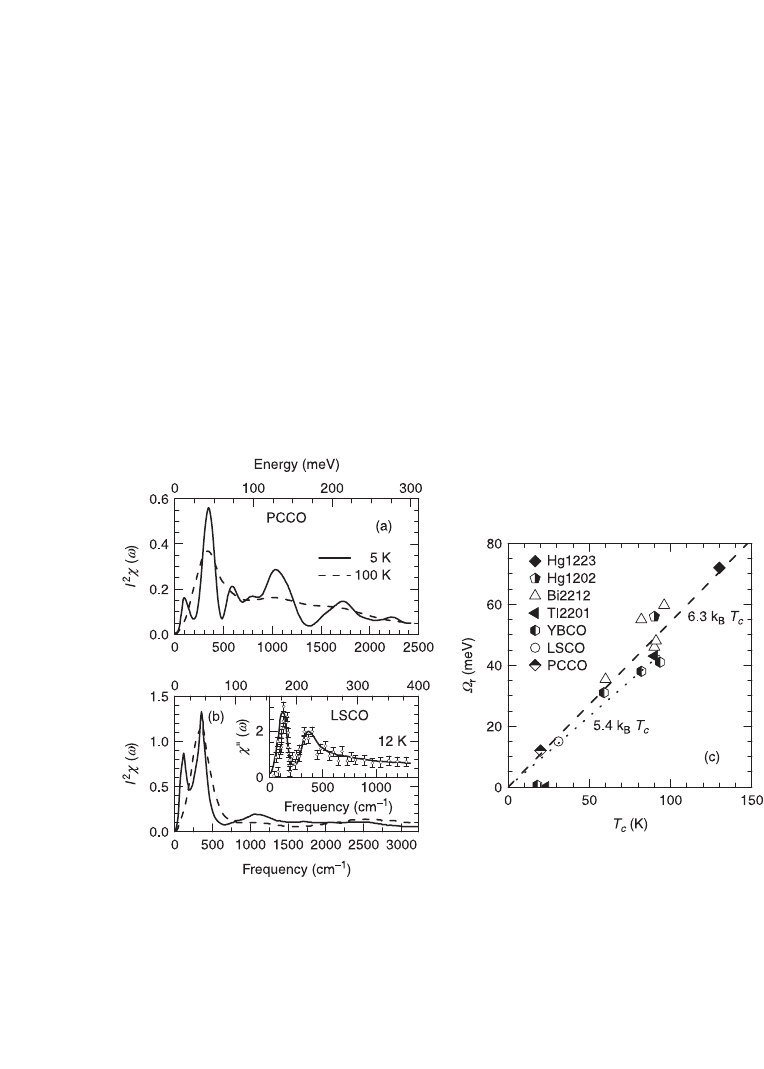
Figure 3.17 is a step in the direction of understanding the origins of the bosonic

spectrum. Panel (a) shows I

for optimally doped PCCO at a few temperatures

(Schachinger et al., 2008). Panel (b) shows the same function for LSCO (Hwang

et al., 2008b). In both cases there is a large peak around 40 meV at all temperatures.

But notice that another peak in the vicinity of 10 meV appears at low temperatures

in both cases. The inset of panel (b) shows neutron scattering data for LSCO

(Vignolle et al., 2007). This neutron spectrum contains the same two peaks

observed in optics. Panel (c), from Yang et al. (2009), summarizes the position of

the resonance peak seen in the optical I

for several compounds as a function of

. The ﬁrst thing we observe is that the position of the peak scales wonderfully

well with 6.3 k

(dashed line). But we also see that the points are not far from

the dotted line. This line is the position of the resonance mode seen in the inelastic

neutron scattering data. The correlation between the optical Eliashberg ‘glue’

function and neutron scattering is striking. It is very important to note that the

candidate for the ‘glue’ is not the resonance peak itself, as in some cases it

disappears before T

(Hwang et al., 2004a). The important part of the bosonic

3.17 Panel (a) shows the bosonic function for optimally doped PCCO

at different temperatures [adapted from Schachinger et al. (2008)].

Panel (b) shows the same function for LSCO [adapted from Hwang

et al. (2008)] and its inset shows the neutron resonance [adapted

from Vignolle et al. (2007)]. Panel (c) at the right shows the correlation

between the position of the IR bosonic peak and the critical

temperature. The dashed line is a best ﬁt through the IR data and the

dotted line is the behavior observed from neutron scattering data

[adapted from Yang et al. (2009)].



Optical conductivity of high-temperature superconductors 135

43X

spectrum is the continuum background. In fact the resonance peak spectral weight

comes from this continuum.

The correlation between the optical I

function and neutron data leaves no

doubt that, in cuprates, carriers are coupled to spin ﬂuctuations.

3.6 Future trends

Two events dominated the aftermath of the discovery of high-temperature

superconductivity. They were the discovery of superconductivity in MgB

and in

iron pnictides. Here I give a brief summary of the main ﬁndings in their optical

conductivity.

3.6.1 MgB

: two superconductors for the price of one

In 2001 Nagamatsu et al. (2001) blew away the record for T

in a non copper-

oxide when they found superconductivity at 39 K in MgB

. The physics behind

this material turned out be close to a conventional superconductor with optimized

parameters (Choi et al., 2002). Nevertheless, MgB

opened the door for the

understanding of multi-band superconductivity at high temperatures. Indeed, this

compound has two bands at the Fermi level and both go superconducting (Giubileo

et al., 2001; Liu et al., 2001). The ﬁrst important result coming out of the optical

conductivity in MgB

(Tu et al., 2001) was the observation that this material had

a reasonably well behaved Drude response and strong correlation effects were not

invited to the party. Guritanu et al. (2006) beautifully showed the consequences in

the reﬂectivity and optical conductivity of the existence of two bands at the Fermi

level. They found a large plasma edge shift depending on whether one probes the

3D character

band or the 2D character, higher electronic density,

band.

Although MgB

represented a breath of fresh air in the ﬁeld, the real shake on

superconductivity was yet to come.

3.6.2 Behind the iron curtain

The big turmoil in the ﬁeld came in 2006. In the pre-high-T

era, ‘common sense’

said that superconductivity and magnetism were mutually exclusive. Little did we

know that Hosono’s group would ﬁnd superconductivity in another non-copper

oxide material based, precisely, on iron (Kamihara et al., 2006) and arsenic

(Kamihara et al., 2008). These so-called pnictides have already reached critical

temperatures over 50 K (Liu et al., 2008) and have a phase diagram which seems

to be strongly driven by magnetism. A global picture of these materials is still not

achieved. They are deﬁnitely more complex than conventional superconductors,

but many successful theoretical predictions were made based on electronic

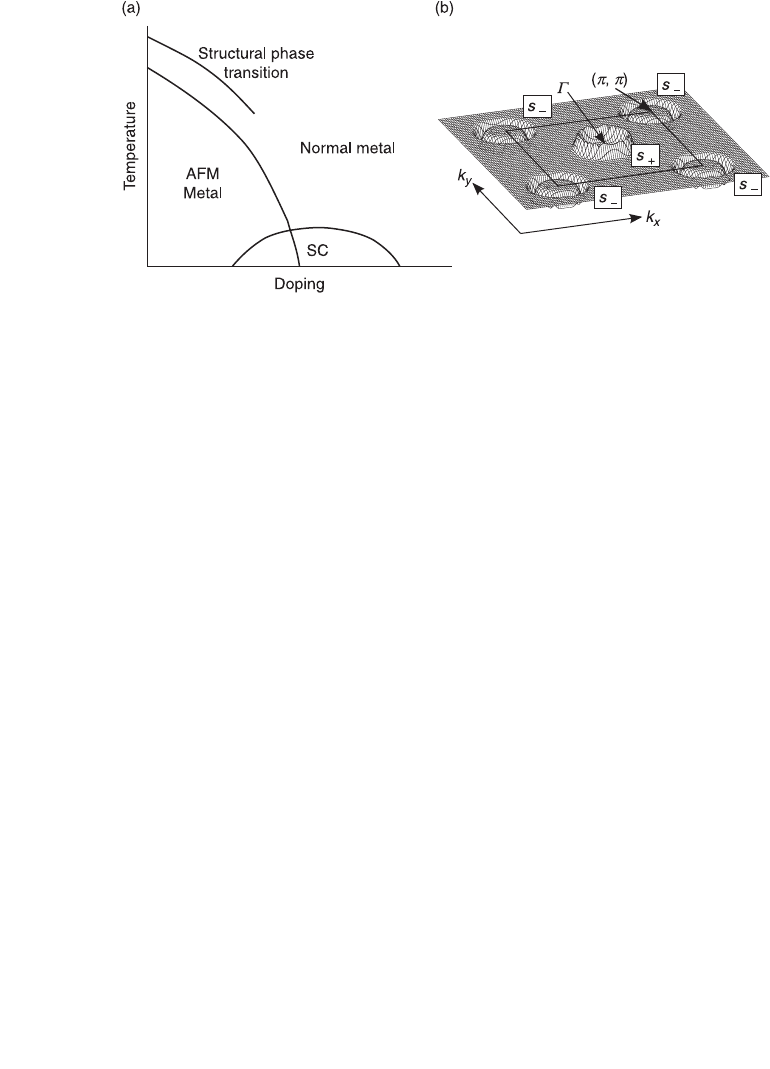
structure calculations (Mazin, 2010). A generic phase diagram of iron pnictides is

shown in Fig. 3.18 (a).



136 High-temperature superconductors

43X

At ﬁrst sight it is similar to the cuprates phase diagram (Fig. 3.1), but do not be

fooled! The only real similarity is the existence of a superconducting dome when

one varies the doping. Otherwise, the parent compound of pnictide superconductors

is an antiferromagnetic metal (as opposed to a Mott insulator). In the normal state

we ﬁnd a structural tetragonal–orthorhombic phase transition happening at

temperatures very close to the magnetic transition. The metallic state shows

several bands crossing the Fermi level. This multi-band metal, already seen in

MgB

, leads to an interesting property in the superconducting state. The right-

hand panel of Fig. 3.18 depicts schematically the location of the main bands in the

Brillouin zone and the expected s

gap symmetry – the band at the Γ point has an

s gap with a sign opposite to the s gap of the bands at (

). This gap phase change

has not been proved, yet. However, many experimental hints, some from the

optical conductivity as I will discuss below, indicate that this is the case.

These materials also show signs of electron–electron correlations as well as

phase competition and coexistence. When a new superconductor is discovered, the

ﬁrst question to pop in the optical conductivity community is ‘Can we see the gap?’

The iron pnictides were not an exception. One of the obvious possibilities is to

expect that, in a multi-band system, one can observe several gaps. The measured

optical conductivity, however, do not show any clear multigap signature.

Nevertheless, a single gap theoretical description does not ﬁt the data either. There

are propositions to describe the data using one (Li et al., 2008), two (van Heuman

et al., 2009), or three gaps (Kim et al., 2010) having values compatible with other

3.18 (a) Generic phase diagram for iron pnictide superconductors.

One should not be fooled by its similar appearance to the cuprates

phase diagram (Fig. 3.1). Here the parent compound is not a Mott

insulator but rather an antiferromagnetic (AFM) metal. Just above,

but close, to the AFM transition there is a structural phase transition

from a high-temperature tetragonal symmetry to a low-temperature

orthorhombic state. Also notice the overlap between the AFM state and

the superconducting dome. Panel (b) depicts the main band positions

in the Brillouin zone with the probable s

gap symmetry.



Optical conductivity of high-temperature superconductors 137

43X

techniques. Although no clear-cut picture emerges from this multigap ﬁtting

analysis, the s

symmetry allows for an interesting property. Chubukov et al.

(2009) showed that (non-magnetic) impurity scattering can create nodes in the gap.

The idea is that if carriers are scattered from a band having an s

gap to another

having an s

–

gap, they will annihilate each other. Impurity scattering can be pair-

breaking. Panel (a) of Fig. 3.19 reproduces data from Gorshunov et al. (2010). The

3.19 The left-hand panels show the far-infrared optical conductivity

in the normal and superconducting states for Ba(Fe,Co)

. In panel

(a) [adapted from Gorshunov et al. (2010)], the open squares are the

normal state and the solid circles the superconducting response. The

lines are ﬁts to Drude and Mattis-Bardeen behaviors. Gorshunov et al.

showed that a Mattis-Bardeen ﬁt fails at low frequencies, the measured

optical conductivity being larger. In panel (b) [from Lobo et al. (2010)],

the low frequency discrepancy is resolved by adding an extra Drude

peak in the superconducting state. This Drude response is the signature

of pair-breaking due to impurity scattering. Panel (c) [adapted from

Hu et al. (2008)], shows the density-wave-like gap corresponding to

the magnetic transition in BaFe

. Panel (d) [from Chen et al. (2009)],

shows that the description of the normal state optical conductivity of

BaNi

needs at least two Drude components. This seems to be a

generic property of pnictides.



138 High-temperature superconductors

43X

open squares represent the normal state optical conductivity and the solid circles

the superconducting response. The lines are ﬁts to Drude (normal state) and Mattis-

Bardeen (superconducting) behaviors. Gorshunov and co-workers point out that

although a gap seems to be present in the optical conductivity, the Mattis-Bardeen

response fails at low frequencies. The data shows a residual conductivity that is

much higher than that produced by thermally broken pairs. A possible solution for

this problem is to admit that impurity scattering breaks pairs giving origin to an

extra Drude peak in the superconducting state. The data by Lobo et al. (2010)

shows that this approach describes the data perfectly. The presence of this Drude

peak is only possible because of the s

gap symmetry.

The magnetic transition observed in the pnictides also has a marked optical

conductivity signature as shown in panel (c) of Fig. 3.19. It behaves like a density-

wave gap in the sense that there is a transfer of spectral from energies below the

gap to energies above the gap (Hu et al., 2008). This response is very similar to

the one observed in electron-doped cuprates (panel (b) of Fig. 3.12) and is

indicative of a Fermi surface reconstruction due to magnetic interactions.

Panel (d) of Fig. 3.19 illustrates the multi-band character of pnictides in a large

energy scale. Chen et al. (2009) showed that the optical conductivity of BaNi

can be described by a few Lorentz oscillators and two different Drude peaks,

allegedly due to carriers from different bands. As shown by Wu et al. (2010), the

presence of two Drude peaks is a generic feature observed in several families of

pnictides.

Although magnetism plays an important role in pnictides, phonons cannot be

ignored as they also have an unconventional behavior. Akrap et al. (2009) showed

that upon crossing the structural phase transition a sharp drop in the 260 cm

–1

(32 meV) phonon frequency is accompanied by an increase in its intensity. Akrap

and co-workers argue that this behavior is a signature of Fe-As orbital ordering.

Another intriguing phonon behavior was observed by Dong et al. (2010) who

show that substituting La by heavier atoms Nd and Sm in LaFeAsO leads to

phonon hardening instead of a conventional softening. This is indicative of a lattice

renormalization. Dong and co-workers argue that as T

is higher for the materials

with the heavier atoms, electron–phonon coupling cannot be dismissed out of hand.

A ﬁnal remark on the pnictides concerns the degree of correlation. These

systems are expected to be in a moderate correlation region as Coulomb repulsion

is neither negligible nor strong enough to localize carriers. In a single band

superconductor, Millis et al. (2005) proposed a way to measure correlations

comparing the spectral weight of the real part of the optical conductivity to values

expected from band structure calculations. Although, strictly speaking, this

method is only valid for a single band system, it is worth looking into the multiband

pnictides. Qazilbash et al. (2009) compared and estimated the degree of electron–

electron correlations in LaFePO and found a moderate, yet signiﬁcant, level of

correlations. After this promising result, it remains to be seen how this method to

measure correlations can be adapted to multi-band superconductors.



Optical conductivity of high-temperature superconductors 139

43X

3.6.3 Concluding remarks

During the past 20 years a full set of measurements on virtually any known

superconducting material has been carried out. Yet the comprehension we have of

superconductivity in cuprates is far from complete. In the past few years we have

seen an increasing interest in probing the properties spanning the phase diagram

working on the very same sample. This is a very difﬁcult and time consuming task

but it will surely enlighten our understanding on the physics of cuprates. The

availability of very low-energy-probing techniques such as THz and direct

measurement of far-infrared complex optical functions by ellipsometry are very

promising. We are facing a time where cross-spectroscopy approaches involving

optical conductivity, Raman, neutrons, STM and ARPES are unveiling the

microscopic excitations pertinent to high-temperature superconductivity. The

recent appearance of the new non-copper-based high-temperature pnictide

superconductors is a very welcome boost to the ﬁeld. Understanding this wealth

of information from optical conductivity will represent a lot of our future work.

3.7 References

Akrap A, Tu J J, Li L J, Cao G H, Xu Z A, and Homes C C (2009). ‘Infrared phonon

anomaly in BaFe

.’ Phys Rev B, 80, 180502. doi:10.1103/PhysRevB.80.180502

Allen P B (1971). ‘Electron–phonon effects in the infrared properties of metals.’ Phys Rev

B, 3, 305–320. doi:10.1103/PhysRevB.3.305

Alloul H, Ohno T, and Mendels P (1989). ‘Y

NMR evidence for a Fermi-liquid behavior

in YBa

6 + x

.’ Phys Rev Lett, 63, 1700–1703. doi:10.1103/PhysRevLett.63.1700

Anderson P W (1987). ‘The resonating valence bond state in La

CuO

and

superconductivity.’ Science, 235, 1196–1198. doi:10.1126/science.235.4793.1196

Armitage N P, Lu D H, Kim C, Damascelli A, Shen K M, et al. (2001). ‘Anomalous

electronic structure and pseudogap effects in Nd

1:85

0:15

CuO

.’ Phys Rev Lett, 87,

147003. doi:10.1103/PhysRevLett.87.147003

Ashcroft N W and Mermin N D (1976). Solid State Physics. Thomson Learning (College

Edition), p. 252. ISBN 0–03–083993–3.

Basov D N, Homes C C, Singley E J, Strongin M, Timusk T, et al. (2001). ‘Unconventional

energetics of the pseudogap state and superconducting state in high-T

cuprates.’ Phys

Rev B, 63, 134514. doi:10.1103/PhysRevB.63.134514

Basov D N, Liang R, Dabrowski B, Bonn D A, Hardy W N, and Timusk T (1996).

‘Pseudogap and charge dynamics in CuO

planes in YBCO.’ Phys Rev Lett, 77, 4090–

4093. doi:10.1103/PhysRevLett.77.4090

Basov D N, Singley E J, and Dordevic S V (2002). ‘Sum rules and electrodynamics of

high-T

cuprates in the pseudogap state.’ Phys Rev B, 65, 054516. doi:10.1103/

PhysRevB.65.054516

Basov D N and Timusk T (2005). ‘Electrodynamics of high-T

superconductors.’ Rev Mod

Phys, 77, 721–59. doi:10.1103/RevModPhys.77.721

Basov D N, Woods S I, Katz A S, Singley E J, Dynes R C, et al. (1999). ‘Sum rules and

interlayer conductivity of high-T

cuprates.’ Science, 283, 49–52. doi:10.1126/

science.283.5398.49

