Principles of Finance with Excel (Основы финансов c Excel)
Подождите немного. Документ загружается.

PFE Chapter 25, The Black-Scholes formula page 20
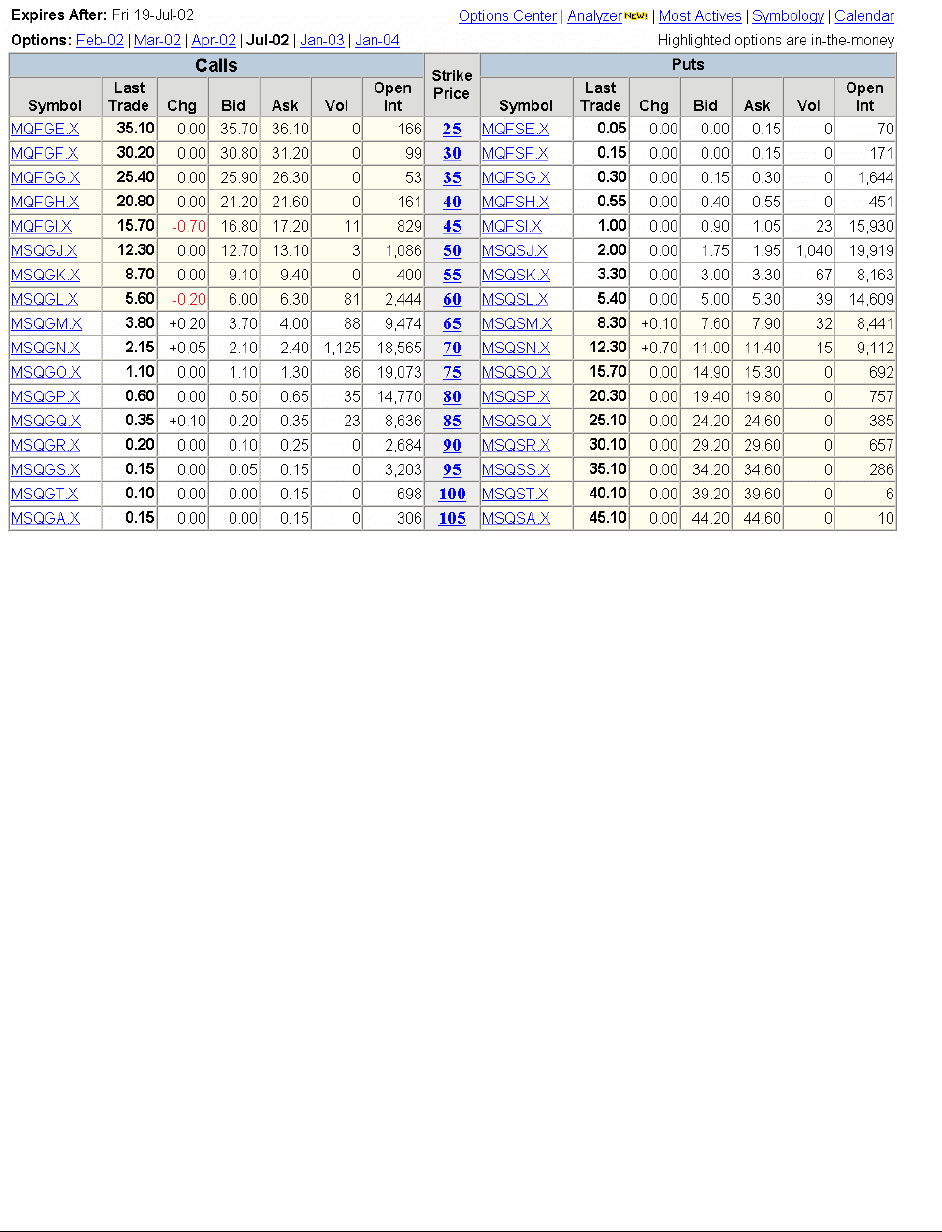
We now look at the closing prices of options on Microsoft stock which expire in July
2002. Clicking on
options in the above box leads us to the option prices:
Look carefully at the above box:
•
Not all the options were traded on 8 February. For example—there was no “volume”
(and hence no trading) of either calls or puts with exercise price (“strike price”) of 25.
•
Significant amounts of call options traded on 8 February were only for exercise prices
X=60, 65, 70, 75, 80, 85. Significant amounts of put options traded were only for
exercise prices X = 45, 50, 55, 60, 65, 70.
•
The price of the “last trade” is in bold face black. But where there is no volume for this
day, the price refers to a previous day’s price.
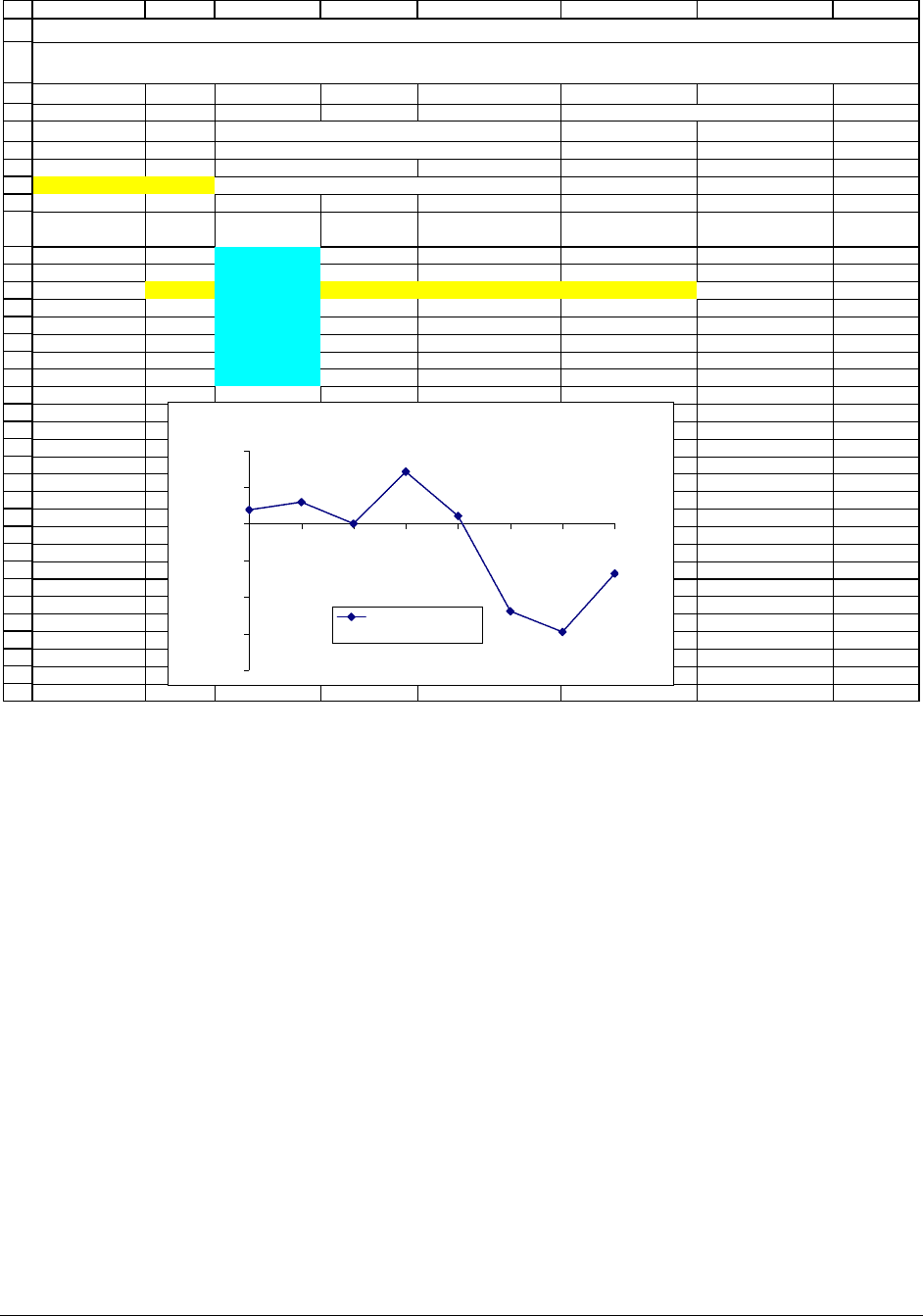
In the spreadsheet below we look at the Microsoft July call options which actually traded
on 8 February and compare the Black-Scholes price to the actual market price. We use the 6-
month Treasury bill rate of 1.7% as our risk-free rate.
PFE Chapter 25, The Black-Scholes formula page 21
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
ABC D E F G H
Computing the time to maturity T
S
0
60.65 Microsoft stock, closing price 8 Feb 02 Current date 8-Feb-02
T 0.44110 Time to maturity of option (in years) Expiration date 19-Jul-02
r 1.70% Risk-free rate of interest Time (days) 161 <-- =G6-G5
Sigma 31.66% <-- =CallVolatility(B5,60,B6,B7,D13) Time (% of year) 0.4411 <-- =G7/365
Exercise
price
BS call
price
Actual call
market price
Market minus BS
in dollars
Market minus BS
in percentage
50 12.07 12.30 0.23 1.89% <-- =(D11-C11)/D11
55 8.44 8.70 0.26 2.94% <-- =(D12-C12)/D12
60 5.60 5.60 0.00 0.00%
65 3.53 3.80 0.27 7.08%
70 2.13 2.15 0.02 1.05%
75 1.23 1.10 -0.13 -11.93%
80 0.69 0.60 -0.09 -14.74%
85 0.37 0.35 -0.02 -6.80%
This spreadsheet computes the Black-Scholes value of the Microsoft July 2002 options on 8 February 2002 and compares the prices to the
actual market prices. As you can see, the Black-Scholes formula works pretty well!
MICROSOFT CALL OPTIONS: Comparing BS to actual prices
BS Call Option Pricing
Microsoft July 2002 Call Options
-20%
-15%
-10%
-5%
0%
5%
10%
50 55 60 65 70 75 80 85
Market minus BS
in percentage
We first use the function
CallVolatility to compute the implied volatility of an at-the-
money call (cell B8). We then use this volatility to price all the Microsoft calls using the Black-
Scholes formula (cells C11:C18). Columns E and F compare the Black-Scholes prices to the
actual market prices in cells D11:D18. The Black-Scholes model does a very good job of pricing
the calls.
Below we repeat this exercise for Microsoft July puts.

PFE Chapter 25, The Black-Scholes formula page 22
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
ABC D E F G H
Computing the time to maturity T
S
0
60.65 Microsoft stock, closing price 8 Feb 02 Current date 8-Feb-02
T 0.44110 Time to maturity of option (in years) Expiration date 19-Jul-02
r 1.70% Risk-free rate of interest Time (days) 161 <-- =G6-G5
Sigma 37.35% <-- =putVolatility(B5,60,B6,B7,D14) Time (% of year) 0.4411 <-- =G7/365
Exercise
price
BS put
price
Actual put
market price
Market minus BS
in dollars
Market minus BS
in percentage
45 0.67 1.00 0.33 32.72% <-- =(D11-C11)/D11
50 1.60 2.00 0.40 19.79%
55 3.16 3.30 0.14 4.27%
60 5.40 5.40 0.00 0.00%
65 8.30 8.30 0.00 0.00%
70 11.77 12.30 0.53 4.31%
This spreadsheet computes the Black-Scholes value of the Microsoft July 2002 options on 8 February 2002 and compares the prices to the
actual market prices. As you can see, the Black-Scholes formula works pretty well!
MICROSOFT PUT OPTIONS: Comparing BS to actual prices
BS Put Option Pricing
Microsoft July 2002 Put Options
-5%
0%
5%
10%
15%
20%
25%
30%
35%
45 50 55 60 65 70
Market minus BS
in percentage
The Black-Scholes model works for both puts and calls. The one problematic feature of
the pricing is that the puts are priced at a higher implied volatility than the calls: The implied
volatility of the at-the-money calls is 31.66% versus an implied volatility for at-the-money puts
of 37.35%.
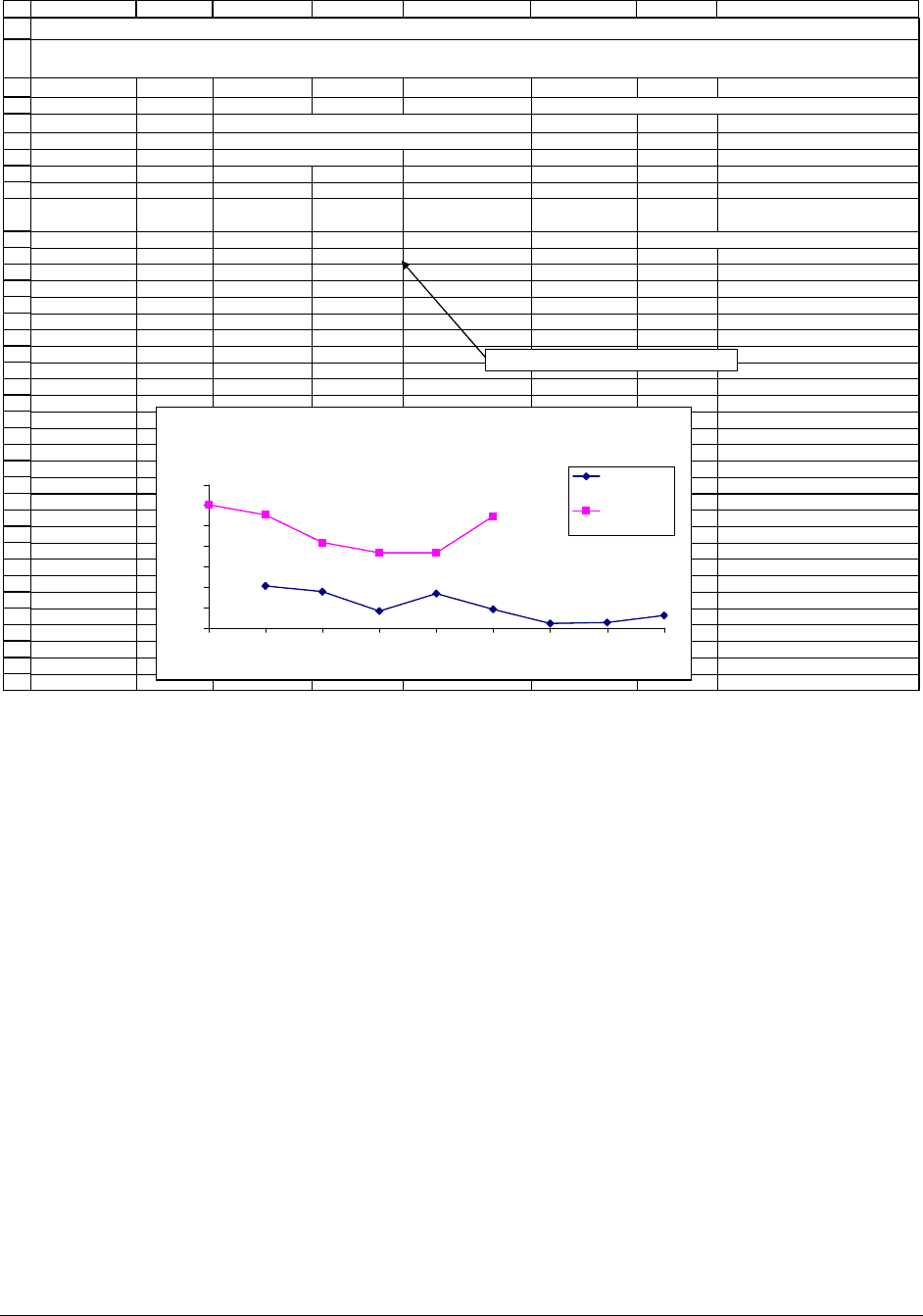
Does the Black-Scholes model work? Looking at implied volatilities
This is our second experiment. We take the Microsoft data above to calculate the implied
volatility for each option (using the functions
CallVolatility and PutVolatility discussed in
Section ????). Here’s our spreadsheet:
PFE Chapter 25, The Black-Scholes formula page 23
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
ABC D E F G H
Computing the time to maturity
S
0
60.65 Microsoft stock, closing price 8 Feb 02 Current date 8-Feb-02
T 0.44110 Time to maturity of option (in years) Expiration date 19-Jul-02
r 1.70% Risk-free rate of interest Time (days) 161 <-- =G6-G5
Time (% of year) 0.4411 <-- =G7/365
Exercise
price
Actual call
market price
Implied call
volatility
A
ctual put
market price
Implied put
volatility
45
1.00 42.05% <-- =putVolatility($B$5,B11,$B$6,$B$7,E11)
50 12.30 34.11% 2.00 41.05%
55 8.70 33.56% 3.30 38.37%
60 5.60 31.66% 5.40 37.35%
65 3.80 33.36% 8.30 37.36%
70 2.15 31.82% 12.30 40.92%
75 1.10 30.44%
80 0.60 30.52%
85 0.35 31.22%
This spreadsheet computes the implied volatility of the Microsoft July 2002 options on 8 February 2002. The average implied volatility of the calls is
lower than the average implied volatility of the puts.
MICROSOFT OPTIONS: Computing the implied volatilities
Comparing the Implied Volatility of MSFT July
2002 Calls and Puts
30%
32%
34%
36%
38%
40%
42%
44%
45 50 55 60 65 70 75 80 85
Exercise price, X
Implied call
volatility
Implied put
volatility
=CallVolatility($B$5,B12,$B$6,$B$7,C12)
The results are both encouraging and discouraging:
•
The implied volatilities for the calls are pretty close together, as are the implied
volatilities for the puts. This is good news.
•
On the other hand the implied volatilities for the puts are uniformly larger than the
implied volatilities for the calls. This is strange, since in the Black-Scholes formulation,
the implied volatility refers to the volatility of the stock’s return and hence has nothing to
do with whether we’re discussing a put or a call option.

PFE Chapter 25, The Black-Scholes formula page 24
• On the third hand,
5
the actual difference between the implied volatilities for the calls and
the puts is not that great (only about 6%).
This is not the place to summarize the vast finance literature on implied volatilities. For
our purposes, the Black-Scholes model works pretty well. That’s enough!
25.8. Real options (advanced topic)
Thus far in this chapter we have discussed the use of the Black-Scholes model to price
call or put options on shares. Such options are sometimes termed financial options because the
option is written on a stock, which is a financial asset. A growing field in finance discusses real
options. A real option is an option which becomes available as the result of an investment
opportunity. Here are some examples of real options:
•
Caulk Shipping is considering the purchase of a license to operate a ferry service from
Philadelphia to Camden. The license requires the company to operate one boat on the
ferry line, but allows Caulk Shipping the possibility of operating as many as ten ferry
boats on the line. This possibility—the option to expand the ferry service—should be
taken into account when Caulk Shipping evaluates the economics of buying the license.
•
Jones Oil is considering the purchase a plot which is known to contain a large quantity of
oil. Tom Shale, the company’s financial analyst has computed the NPV of the lease—he
5
Harry Truman is reported to have gotten so sick of hearing economists say “On the hand, ... . But on the other
hand, ... ” that he asked his chief of staff to get him a “one-handed economist.” History does not record if he
succeeded. The economist in this section’s bullets has at least 3 hands. Harry Truman would not have liked him.

PFE Chapter 25, The Black-Scholes formula page 25
assumes that once the oil drilling equipment is in place, the company will pump the oil
out of the ground at the maximum feasible rate. However, Tom also realizes that the
financial analysis of the plot purchase should include an important real option: If the
future oil price is low, Jones Oil can stop pumping the oil and wait until the price gets
higher. This option to delay has obvious value.
•
Merrill Widgets is considering the purchase of six new widget machines to replace
machines which are currently in place. The new machines employ an innovative
production technology and are much more sophisticated than the old machines. Simona
Mba, the company’s financial analyst, has determined that the NPV of replacing a single
machine is negative, and thus recommends against the replacement. Roberta Merrill, the
company’s owner, has a slightly different logic: She wants to purchase one widget
machine in order to learn about the machine’s possibilities; after a year she will then
decide whether to buy the remaining five widget machines. The purchase of a single new
widget machine gives Merrill Widgets the option to learn. The company’s financial
analysis should value this option. Below we return to this case and show how to value
the option to learn.
A simple example of the option to learn
In the rest of this section we will show how the Black-Scholes model can be used to
value Merrill Widget’s option to learn. Recall that the company is considering replacing each of
its existing six widget machines with a new machines. The new machines cost $1,000 each and
have a five-year life. Simona Mba, the company’s financial analyst, has estimated the expected
per-machine cash flows; these flows are defined as the incremental cash flow of replacing a
PFE Chapter 25, The Black-Scholes formula page 26
single old machine by a new machine and include the after-tax savings from introducing new
machines, the tax shield on incremental depreciation from replacing an old by a new machine,
and the sale of the old machine. It is important to emphasize that management does not know
the exact realization of these annual cash flows, but knows only their expected values. The
expected cash flows for the new machine are given below.
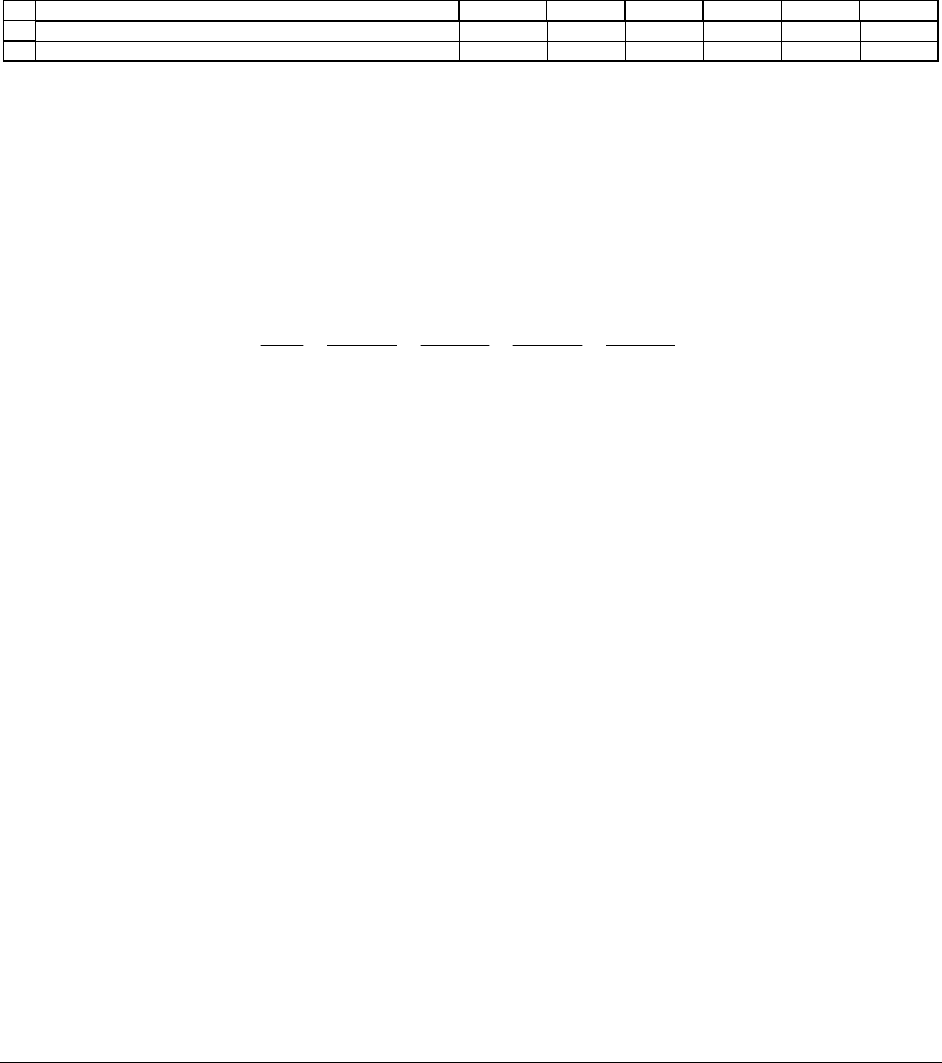
3
4
ABCDEFG
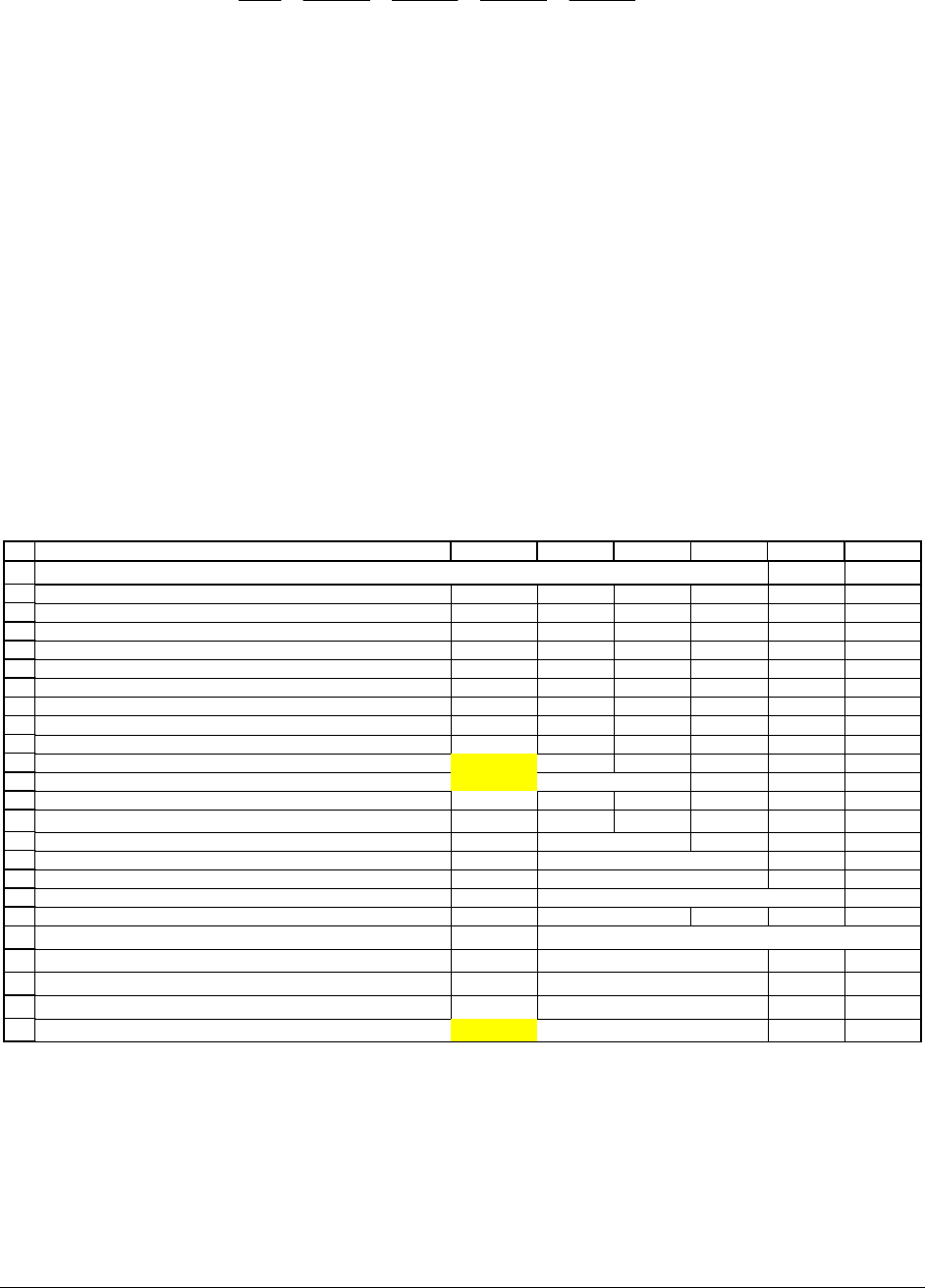
Year 012345
CF of sin
g
le machine -1000 220 300 400 200 150
Simona estimates the risk-adjusted cost of capital for the project as 12%. Using the expected
cash flows and a cost of capital of 12% for the project; Simona has concluded that the
replacement of a single old machine by a new machine is unprofitable, since the NPV is
negative:
()()()()
48.67
12.1
150
12.1
200
12.1
400
12.1
300
12.1
220
1000
5432
−=+++++−
Now comes the (real options) twist. Roberta Merrill, the company’s owner, says: “I
want to try one of the new machines for a year and learn the true realization of its cash flows. At
the end of the year, if the experiment is successful, I want to replace five other similar machines
on the line with the new machines. If I do not try one of the new machines, I will never know
their true cash flows.”
Does this change our previously negative conclusion about replacing a single machine?
The answer is “yes.” To see this, we now realize that what we have is a package:
•
Replacing a single machine today. This has a NPV of –67.48.
•
The option of replacing 5 more machines in one year. We can view each such option
as a call option on an asset which has current value of:
PFE Chapter 25, The Black-Scholes formula page 27
()()()()
52.932
12.1
150
12.1
200
12.1
400
12.1
300
12.1
220
5432
=++++=S
and an exercise price X = 1,000. Of course these call options can be exercised only if
we purchase the first machine now; in effect the real options model will be pricing the
learning costs.
Let’s suppose that the Black-Scholes option pricing model can price this call option. We
further suppose that the risk-free rate is 6% and the standard deviation of the cash flows is
σ
=
40%. The next figure shows that the value of the each of the options to acquire one machine in
one year is $143.98. It now follows that the value of the whole project is $652.39 (cell B12):
67.48 5*143.98 652.39
Project value = NPV of first machine+ 5 options to acquire
=− + =
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
ABCDEFG
Year 0 12345
CF of single machine -1000 220 300 400 200 150
Discount rate for machine cash flows 12%
Riskless discount rate 6%
NPV of single machine -67.48
Number of machines bought next year 5
Option value of single machine purchased in one more year 143.98 <-- =B24
NPV of total project 652.39 <-- =B8+B10*B11
Black-Scholes Option Pricing Formula
S 932.52 PV of machine CFs
X 1000.00 Exercise price = Machine cost
r 6.00% Risk-free rate of interest
T 1 Time to maturity of option (in years)
Sigma 40% <-- Volatility
d
1
0.1753 <-- (LN(S/X)+(r+0.5*sigma^2)*T)/(sigma*SQRT(T))
d
2
-0.2247
<-- d
1
- sigma*SQRT(T)
N(d
1
)
0.5696
<--- Uses formula NormSDist(d
1
)
N(d
2
)
0.4111
<--- Uses formula NormSDist(d
2
)
Option value = BS call price 143.98
<-- S*N(d
1
)-X*exp(-r*T)*N(d
2
)
THE OPTION TO EXPAND
Thus, buying one machine today, and in the process acquiring the option to purchase five more
machines in one year is a worthwhile project.

PFE Chapter 25, The Black-Scholes formula page 28
One critical element here is the volatility. The lower the volatility (i.e., the lower the
uncertainty), the less worthwhile this project is. By building a data table we can examine the
relation between the standard deviation
σ
and the project value:
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
BCDEFGHIJ
σ
652.39 <-- Table header: =B12
1% -63.48
10% 97.16
20% 283.09
30% 468.40
40% 652.39
50% 834.59
60% 1014.54
70% 1191.81
Data Table
Project Value as Function of Sigma
-200
0
200
400
600
800
1000
1200
1400
0% 10% 20% 30% 40% 50% 60% 70% 80%
Sigma
The value of the project as a whole comes from our uncertainty about the actual cash
flows one year from now. The less is this uncertainty (measured by
σ
), the less valuable the
project. In this particular example a very low uncertainty (
σ
> 4.75%) with respect the machine
cash flow returns is sufficient to justify its purchase.
6
6
Estimating the
σ
for real option cash flows is problematic, since there is little market data (as there is for stocks) to
guide us. Many authors use estimates in the range of 30% - 50% for the standard deviation of real option returns;
this is somewhat higher than the average standard deviation of U.S. market returns for equity, which are in the range
of 15% - 30%. To explore this issue, consult one of the three leading books in the area: Lenos Trigeorgis, Real
Options: Managerial Flexibility and Strategy in Resource Allocation, MIT Press, 1996; Martha Amram and Nalin
Kulik, Real Options, Harvard Business School, 1998; Tom Copeland and Vladimir Antikarov, Real Options: A
Practitioner’s Guide, Texere, 2001.

PFE Chapter 25, The Black-Scholes formula page 29
Real options: where do we go from here?
Real options are increasingly used in finance to value corporate investments. The
example of Merrill Widgets given above is only a small example of the use of the real options
technique. For deeper discussions, we suggest you consult one of the books mentioned in
footnote 000.
Summary
This chapter has given you a quick and hopefully practical insight into how to use the
Black-Scholes model. The Black-Scholes model is remarkably good at pricing options and is
widely used. It is also easy to use, provided you don’t get too hung up on the details of where
the formula comes from (in this chapter we’ve left these hang-ups behind us, and concentrated
exclusively on implementational details).
