Nakahara M. Geometry, Topology and Physics

Подождите немного. Документ загружается.

Figure 10.6. The ﬁbre of a quantum mechanical system which depends on adiabatic

parameters R.

Fixing the phase of |R at each point R ∈ M amounts to choosing a section.

Let σ(R) =|R be a local section over a chart U of M. The canonical local

trivialization is given by

−1

(|R) = (R, e). (10.138)

The ‘right’ action yields

−1

(|R·g) = ( R, e)g = (R, g). (10.139)

Now that the bundle structure is deﬁned, we provide it with a connection.

Let us deﬁne Berry’s connection by

≡R|(d |R) =−(dR|)|R (10.140)

where d = (∂/∂ R

)dR

is the exterior derivative in R-space. Note that is

anti-Hermitian since

0 = d(R|R) = (dR|)|R+R|d|R=R|d|R

∗

+R|d|R.

To see (10.140) is indeed a local form of a connection, we have to check the

compatibility condition. Let U

and U

be overlapping charts of M and let

(R) =|R

and σ

(R) =|R

be the respective local sections. They are

related by the transition function as |R

=|R

(R). We then ﬁnd that

(R) =

R|d|R

= t

(R)

−1

R|[d|R

(R) +|Rdt

(R)]

(R) +t

(R)

−1

(R). (10.141)

The set of one-forms {

} satisfying (10.141) deﬁnes an Ehresmann connection

on P(M, U(1)).

The ﬁeld strength

of is called Berry’s curvature and is given by

= d = (dR|) ∧ (d|R) =



∂R|

∂ R



∂|R

∂ R



∧ dR

. (10.142)

After an example from atomic physics, we shall clarify how this geometrical

structure is reﬂected in Berry’s phase.

Example 10.7. Let us consider a quantum mechanical system which contains

‘fast’ degrees of freedom r and ‘slow’ degrees of freedom R. For example, we

may imagine an electron moving under the potential of slowly vibrating ions.

Suppose the Hamiltonian is given by

H =

+ V (r; R) (10.143)

where p( P) is the momentum canonical conjugate to r( R).Asaﬁrst

approximation, we may consider the slow degrees of freedom are ‘frozen’ at some

value R and consider an instantaneous sub-Hamiltonian

h(R) =

+ V (r; R) (10.144)

and the eigenvalue problem

h(R)|R=

(R)|R (10.145)

where |R stands for the nth eigenvector |n; R of the ‘fast’ degrees of freedom.

We assume that the eigenvalue is isolated and non-degenerate. Berry’s connection

(R) =R|d|R, while the curvature is = (dR|) ∧ (d|R).

It is interesting to see how the fast degrees of freedom affect the slow degrees

of freedom. We assume the total wavefunction is written in the form

(r; R) = (R)|R (10.146)

and ﬁnd the ‘effective’ Schr¨odinger equation which (R), the wavefunction

of the ‘slow’ degrees of freedom, satisﬁes. The eigenvalue problem of the

Hamiltonian (10.143) is

H (r; R) =−

[∇

(R)|R+2∇

(R) ·∇

|R+(R)∇

|R]

− (R)

∇

|R+(R)V (r; R)|R

= E

(R)(R)|R.

If we multiply R| on the left and use the Schr¨odinger equation (10.145), this

equation becomes

−

[∇

(R) + 2∇

(R) ·R|∇

|R+(R)(R|∇

|R)

]

+ 

(R)(R) = E

(R)( R) (10.147)

where we have employed the Born–Oppenheimer approximation, in which all the

matrix elements except the diagonal ones are neglected,

n; R|∇



; R=0 n



= n. (10.148)

Now the effective Hamiltonian for |(R) is given by

eff

(n) ≡−



∂

∂ R

(R)



+ ε

(R) (10.149)

where

is a component of Berry’s connection,

(R) =R|

∂

∂ R

|R. (10.150)

It is remarkable that the fast degrees of freedom have induced a vector potential

coupled to the slow degrees of freedom. Note also that the eigenvalue ε

(R)

behaves as a potential energy in H

eff

. This ‘spontaneous creation’ of the gauge

symmetry reﬂects the phase degree of freedom of the wavefunction |R.

The Schr¨odinger equation describing the adiabatic change is

H (R(t))|R(t), t=i

|R(t), t (10.151a)

wherewenotethat|R (t), t has an explicit t-dependence as well as an implicit

one through R(t). Berry assumes that

|R(t), t=exp



− i



(t) dt



iη(t)

|R(t) (10.152a)

where |R is an instantaneous normalized eigenstate of H (R),

(R)|R=E

(R)|RR|R=1. (10.153)

The ﬁrst exponential is the ordinary dynamical phase while the second one is

Berry’s phase. It is convenient for our purpose to deﬁne an operator

(R) ≡ H (R) − E

(R) (10.154)

to dispose of the dynamical phase. The state |R is the zero-energy eigenstate of

(R): ( R)|R=0. The solution of the modiﬁed Schr¨odinger equation,

(R)|R(t), t=i

|R(t), t (10.151b)

is then given by

|R(t), t=e

iη(t)

|R(t).(10.152b)

We found in (10.136) that η is given by

η(t) = i



R(s)|

∂

∂ R

|R(s)=i



R(t)

R(0)

R|d|R. (10.155)

We show that Berry’s phase is a holonomy associated with the connection

(10.140) on P(M, U(1)). Take a section σ(R) =|R over a chart U of M.Let

R :[0, 1]→M be a loop in U.

We write a horizontal lift of R(t) with respect

to the connection (10.140) as

R(t) = σ(R(t))g( R(t)) (10.156)

where g(R(0)) is taken to be the unit element of U(1). The group element g(t)

satisﬁes (10.13b),

dg(t)

g(t)

−1

=−





=−R(t)|

|R(t) (10.157)

where g(t) stands for g(R(t)).Fromg(t) = exp(iη(t)), we obtain

dη(t)

=−R(t)|

|R(t)

which is easily integrated to yield

η(1) = i



R(s)|

|R(s)ds = i

R|d|R. (10.158)

Let us note that R(0) = R(1), hence |R(0)=|R(1). Then exp[iη(1)] is

regarded as a holonomy (ﬁgure 10.7)

R(1) = exp



−

R|d|R



·|R(0). (10.159a)

Exercise 10.14. Let S beasurfaceinM, which is bounded by the loop R(t).

Show that

R(1) = exp



−



·|R(0) (10.159b)

where

is given by (10.142).

Example 10.8. Let us consider a spin-

particle in a magnetic ﬁeld with the

Hamiltonian

H (R) = R · σ =



− iR

+ iR

−R



. (10.160)

We shall be a little sloppy in our notation.

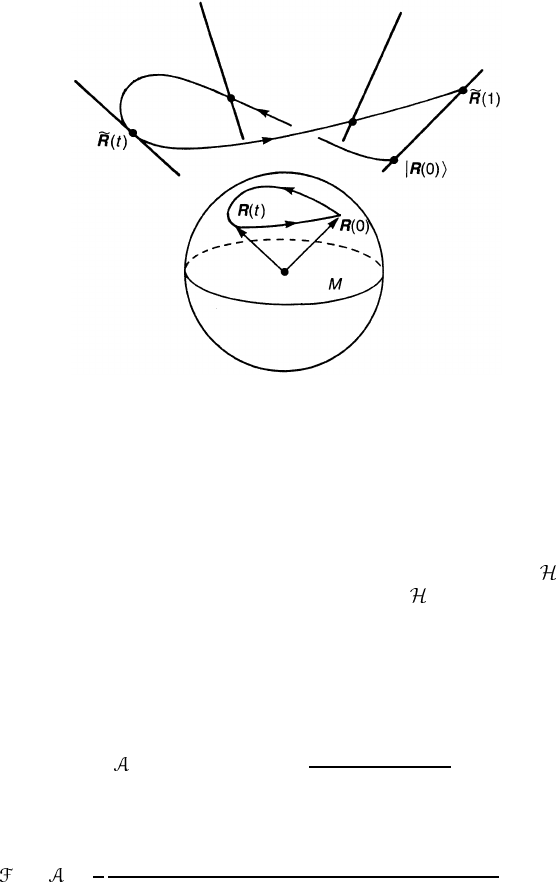
Figure 10.7. If the parameter changes adiabatically along a loop R(t), the state with initial

condition |R(0) becomes |

R(1) which is different from |R(0) in general. The difference

is the holonomy and is identiﬁed with Berry’s phase.

The parameter R corresponds to the applied magnetic ﬁeld. This is a two-level

system taking eigenvalues ±|R|. Let us consider the eigenvalue R =+|R|.

According to the prescription just described, we introduce a Hamiltonian

(R) ≡

H (R) −|R| and consider the zero-energy eigenstate of

(R) given by

|R

=[2R(R + R

)]

−1/2



R + R

+ iR



. (10.161)

The gauge potential is obtained after a straightforward but tedious calculation as

R|d|R

=−i

− R

2R(R + R

)

. (10.162)

Theﬁeldstrengthis

= d =

∧ dR

+ R

∧ dR

+ R

∧ dR

. (10.163)

So far we have assumed that the state |R is isolated. However, this

assumption breaks down if R = 0, in which case two eigenstates are degenerate.

Surprisingly, this singularity behaves like a magnetic monopole in R-space. To

see this, we introduce polar coordinates θ and φ in R-space,

= R sin θ cos φ R

= R sin θ sin φ R

= R cos θ.

The state (10.161) is expressed as

|R



cos(θ/2)

iφ

sin(θ/2)



. (10.164)

This state is singular at θ = π , reﬂecting that |R

is not deﬁned for R

=−R.

Consider another eigenvector

|R

≡ e

−iφ

|R



−iφ

cos(θ/2)

sin(θ/2)



=[2R(R − R

)]

−1/2



− iR

R − R



(10.165)

with the same eigenvalue. This eigenvector is singular at θ = 0, that is at

= R. Corresponding to these vectors, we have Berry’s gauge potentials in

polar coordinates,

i(1 − cos θ)dφθ= π (10.166a)

=−

i(1 + cos θ)dφθ= 0. (10.166b)

They are related by the gauge transformation,

− idφ =

+ e

iφ

−iφ

(10.167)

where g(π/2,φ) = exp(−iφ) is identiﬁed with the transition function t

Equation (10.166) is simply the vector potential of the Wu–Yang monopole of

strength −

, see sections 1.9 and 10.5. The total ﬂux of the monopole is

 = 4π(−

) =−2π.

The analogy between the present problem and the magnetic monopole is

evident by now. If we ﬁx the amplitude R of the magnetic ﬁeld, the restricted

parameter space is S

. At each point R of S

, the state has a phase degree

of freedom. Thus, we are dealing with a U(1) bundle P(S

, U(1)), which also

describes a magnetic monopole. For each choice of the parameters R,wehave

a ﬁbre corresponding to the nth eigenstate |n; R.TheﬁbreatR consists of the

equivalence class [|R] deﬁned by (10.137). The projection π maps a state to

the parameter on which it is deﬁned: π : e

iα

|R→R ∈ S

. As we have seen,

this bundle is non-trivial since it cannot be described by a single connection. The

non-triviality of the bundle implies the existence of a monopole at the origin. Note

that R = 0 (that is, B = 0) is a singular point at which all the eigenvalues are

degenerate.

Next we turn to the problem of holonomy. Take a standard point R(0)

on S

and choose a vector |R(0). We choose a loop R(t) on S

and execute

a parallel transportation of |R(0) along R(t), after which it comes back as

a vector exp[iη(1)]|R(0). The additional phase η represents the holonomy

−1

(R) → π

−1

(R) and corresponds to Berry’s phase. From (10.158), η(1)

is given by

η(1) = i

= i



(10.168)

where

= d is the ﬁeld strength and S is the surface bounded by the loop R(t).

It follows from (10.168) that Berry’s phase η(1) represents the ‘magnetic ﬂux’

through the area S.

Exercise 10.15. Use (10.165) to show that

− R

R(R − R

)

. (10.169)

Show also that

dφ =−

− R

(R + R

)(R − R

)

. (10.170)

Observe that dφ is singular at R

=±R.

Problems

10.1 Consider a two-dimensional plane M with coordinate R and a wavefunction

ψ which depends on R adiabatically as ψ = ψ(r, R ).LetR :[0, 1]→M

be a loop in M and suppose ψ(r, R(1)) =−ψ(r, R(0)), that is the phase of ψ

changes by π after an adiabatic change along the loop. Show that there is a point

within the loop at which the adiabatic assumption breaks down. See Longuet-

Higgins (1975).

CHARACTERISTIC CLASSES

Given a ﬁbre F, a structure group G and a base space M, we may construct

many ﬁbre bundles over M, depending on the choice of the transition functions.

Natural questions we may ask ourselves are how many bundles there are over M

with given F and G, and how much they differ from a trivial bundle M × F.For

example, we observed in section 10.5 that an SU(2) bundle over S

is classiﬁed

by the homotopy group π

(SU(2))

∼

. The number n ∈ tells us how the

transition functions twist the local pieces of the bundle when glued together.

We have also observed that this homotopy group is evaluated by integrating

∈ H

) over S

, see theorem 10.7.

Characteristic classes are subsets of the cohomology classes of the base

space and measure the non-triviality or twisting of a bundle. In this sense, they

are obstructions which prevent a bundle from being a trivial bundle. Most of the

characteristic classes are given by the de Rham cohomology classes. Besides their

importance in classiﬁcations of ﬁbre bundles, characteristic classes play central

roles in index theorems.

Here we follow Alvalez-Gaum´e and Ginsparg (1984), Eguchi et al (1980),

Gilkey (1995) and Wells (1980). See Bott and Tu (1982), Milnor and Stasheff

(1974) for more mathematical expositions.

11.1 Invariant polynomials and the Chern–Weil homomorphism

We give here a brief summary of the de Rham cohomology group (see chapter 6

for details). Let M be an m-dimensional manifold. An r-form ω ∈ 

(M) is

closed if dω = 0andexact if ω = dη for some η ∈ 

r−1

(M). The set of closed r-

forms is denoted by Z

(M) and the set of exact r -forms by B

(M).Sinced

= 0,

it follows that Z

(M) ⊃ B

(M).Wedeﬁnetherth de Rham cohomology group

(M) by

(M) ≡ Z

(M)/B

(M).

In H

(M), two closed r -forms ω

and ω

are identiﬁed if ω

−ω

= dη for some

η ∈ 

r−1

(M).LetM be an m-dimensional manifold. The formal sum

∗

(M) ≡ H

(M) ⊕ H

(M) ⊕···⊕H

(M)

is the cohomology ring with the product ∧:H

∗

(M) × H

∗

(M) → H

∗

(M)

induced by ∧:H

(M) × H

(M) → H

p+q

(M).Letf : M → N be a

smooth map. The pullback f

∗

: 

(N) → 

(M) naturally induces a linear

map f

∗

: H

(N) → H

(M) since f

∗

commutes with the exterior derivative:

∗

dω = d f

∗

ω. The pullback f

∗

preserves the algebraic structure of the

cohomology ring since f

∗

(ω ∧ η) = f

∗

ω ∧ f

∗

η.

11.1.1 Invariant polynomials

Let M(k,

) be the set of complex k × k matrices. Let S

(M(k, )) denote

the vector space of symmetric r-linear

-valued functions on M(k, ).Inother

words, a map

P :

⊗ M(k, ) →

is an element of S

(M(k, )) if it satisﬁes, in addition to linearity in each entry,

the symmetry

P(a

,...,a

)

P(a

,...,a

) 1 ≤ i, j ≤ r (11.1)

where a

∈ GL(k, ).Let

∗

(M(k, )) ≡

∞

⊕

r=0

(M(k, ))

denote the formal sum of symmetric multilinear

-valued functions. We deﬁne a

product of

P ∈ S

(M(k, )) and

Q ∈ S

(M(k, )) by

Q(X

,...,X

p+q

)

( p +q)!



P(X

P(1)

,...,X

P(p)

)

Q(X

P(p+1)

,...,X

P(p+q)

) (11.2)

where P is the permutation of (1,...,p + q). S

∗

(M(k, )) is an algebra with

this multiplication.

Let G be a matrix group and

its Lie algebra. In practice, we take

G = GL(k,

), U(k) or SU(k).TheLiealgebra is a subspace of M(k, )

and we may consider the restrictions S

( ) and S

∗

( ) ≡

r≥0

( ).

P ∈ S

( )

is said to be invariant if, for any g ∈ G and A

∈ ,

P satisﬁes

P(Ad

,...,Ad

) =

P(A

,...,A

) (11.3)

where Ad

= g

−1

g. For example,

P(A

, A

,...,A

) = str(A

, A

,...,A

)

≡



tr(A

P(1)

, A

P(2)

,...,A

P(r)

) (11.4)

is symmetric, r-linear and invariant, where ‘str’ stands for the symmetrized trace

and is deﬁned by the last equality. The set of G-invariant members of S

( ) is

denoted by I

(G). Note that

does not necessarily imply I

) =

). The product deﬁned by (11.2) naturally induces a multiplication

(G) ⊗ I

(G) → I

p+q

(G). (11.5)

The sum I

∗

(G) ≡

r≥0

(G) is an algebra with this product.

Take

P ∈ I

(G). The shorthand notation for the diagonal combination is

P( A) ≡

P(A, A,...,A

-. /

) A ∈ . (11.6)

Clearly, P is a polynomial of degree r and called an invariant polynomial. P is

also Ad G-invariant,

P(Ad

A) = P(g

−1

Ag) = P(A) A ∈ , g ∈ G. (11.7)

For example, tr( A

) is an invariant polynomial obtained from (11.4). In general,

an invariant polynomial may be written in terms of a sum of products of P

≡

tr(A

Conversely, any invariant polynomial P deﬁnes an invariant and symmetric

r-linear form

P by expanding P(t

+···+t

) as a polynomial in t

.Then

1/r ! times the coefﬁcient of t

···t

is invariant and symmetric by construction

and is called the polarization of P.TakeP(A) ≡ tr(A

), for example. Following

the previous prescription, we expand tr(t

+ t

)

in powers of t

, t

and t

. The coefﬁcient of t

tr(A

+ A

)

= 3tr(A

+ A

)

where the cyclicity of the trace has been used. The polarization is

P( A

, A

) =

tr(A

+ A

) = str(A

, A

In the previous chapter, we introduced the local gauge potential

and the ﬁeld strength =

µν

∧ dx

on a principal bundle. We have

shown that these geometrical objects describe the associated vector bundles as

well. Since the set of connections {

} describes the twisting of a ﬁbre bundle,

the non-triviality of a principal bundle is equally shared by its associated bundle.

In fact, if (10.57) is employed as a deﬁnition of the local connection in a vector

bundle, it can be deﬁned even without reference to the principal bundle with which

it is originally associated. Later, we encounter situations in which use of vector

bundles is essential (the Whitney sum bundle, the splitting principle and so on).