Mei C., Zhou J., Peng X. Simulation and Optimization of Furnaces and Kilns for Nonferrous Metallurgical Engineering

Подождите немного. Документ загружается.

2 Modeling of the Thermophysical Processes in FKNME 

constant current, arithmetical progressive changing current and linear changing

current.

In cases when there are more than one metallurgical furnaces in the workshop,

it is also necessary to take the effects of adjacent furnaces into account.

2.4.2



Mathematical model of current field

The purpose to study the current field is to determine the current distribution

in the melt of the furnace, so as to study the interactions between the current

and magnetic fields. On the basis of previous experience and some proper

hypothesis, the physical model is to be simplified into a two-dimensional

section model.

The boundary element method is used to simulate the current flow field. Basic

equations for problem are:

Su u

Sq u nq

⎫

⎪

⎬

⎪

⎭

Ω∇ =

=∂ ∂ =

(2.189)

where

u is the potential function; Ω is the solution domain; S

is the first

boundary condition; S

is the second boundary condition, S = S

+ S

is the

boundary of

Ω, and n is the exterior normal direction of S. According to Green

theorem or weighted residual method, the boundary integral equation can be

deduced by rendering the basic solution as a weight function:

Cu u u S

∂∂

⎛⎞

=−

⎜⎟

∂∂

⎝⎠

∫

(2.190)

iC =

⎧

⎪

⎨

⎪

⎩

1/2

∈Ω

⎫

⎪

∈

⎬

⎪

∉Ω

⎭

(2.191)

where u* is the basic solution.

For three-dimensional isotropic dielectric:

where r is the distance from the field point to the source point.

In a two-dimensional field:

2π

u = ln

  Ping Zhou, Feng Mei and Hui Cai

2.4.3



Mathematical models of magnetic field in conductive elements

Knowing current distribution in the electrodes (anode, bus bar), the electric

heating element and the melt, the total magnetic field is computed using the

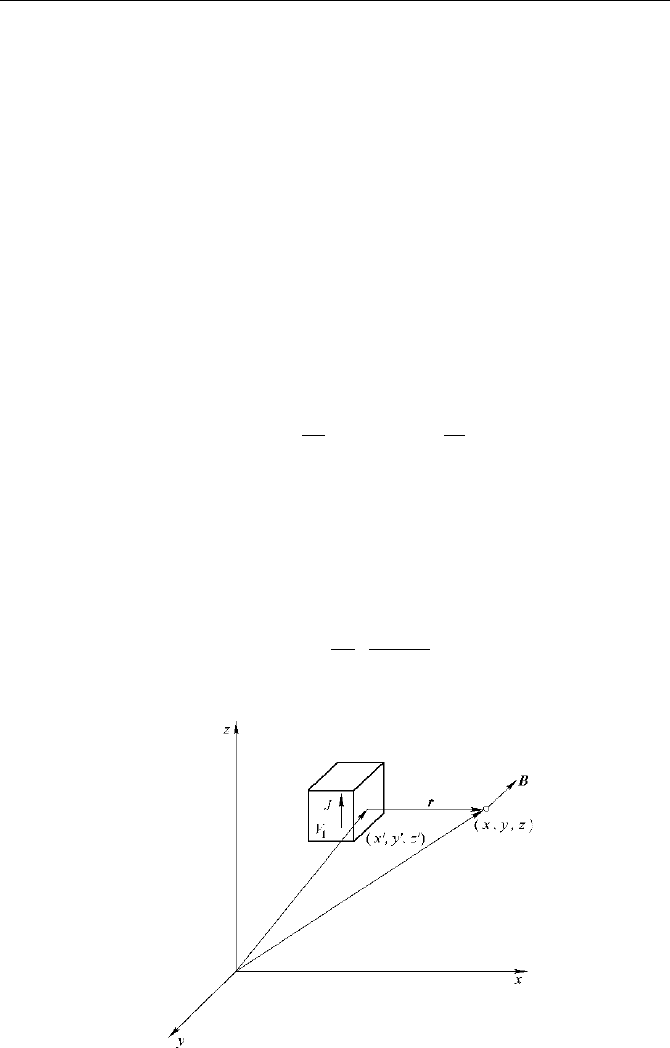
Biot-Savart law and its mathematical model is as follows (Yang, 1998).

2.4.3.1



Mathematical model of equivalent axial current

The equivalent axial current model assumes the current through the conductors all

concentrate along the axis so that can be represented by a linear current (Cai,

1992).

Given a constant magnetic field, the magnetic induction intensity generated by

the current flow can be computed with the Biot-Savart law.

(, ,) ( , , ) d

′′′

=×

∫

(2.192)

where

is the vacuum permeability,

= 4π×10

-7

H/m; dV is the

elementary volume around the source point;

(, ,)xyz

′′′

J is the current density;

the integral domain V

is the whole current carrying conductor domain (Fig. 2.8).

For linear currents, ( , , )xyz

′′′

J dV =J • ds • dl=I • dl. So, Eq. 2.192 can be

rewritten into:

()

x, y,z

∫

(2.193)

Fig. 2.8 Magnetic field of current

2 Modeling of the Thermophysical Processes in FKNME 

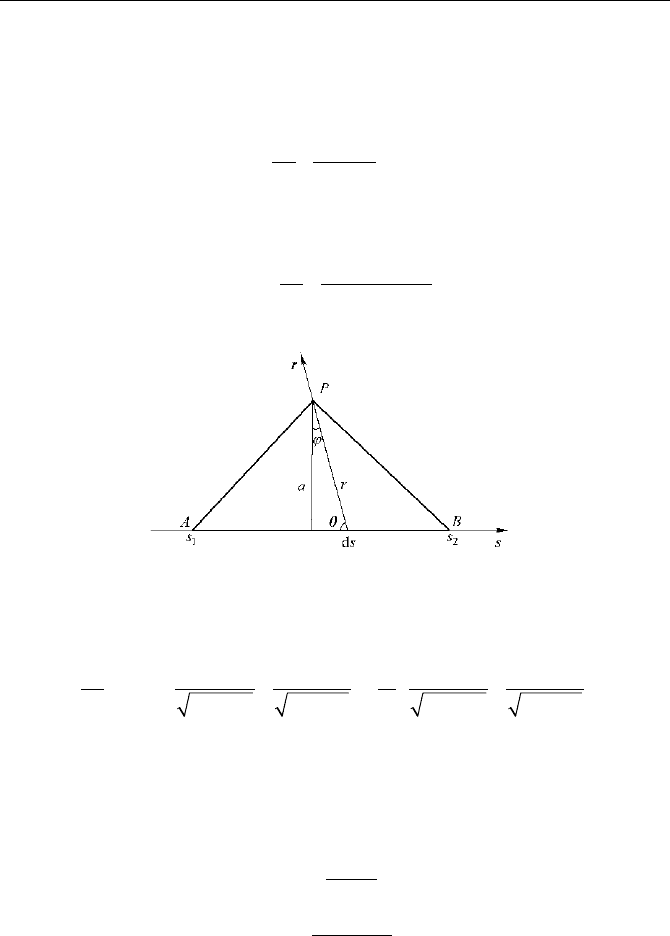
If there is linear varying current running along axis s, of which the current

equation is I=

αs + β , where AǃB are the two ends of the linear current, and the

corresponding coordinate is s

ǃs

), P is field point (Fig. 2.9), according to

Eq. 2.193, the magnetic induction intensity of point P is:

∫

(2.194)

of which, its value can be obtained as:

(+)sin

∫

B (2.195)

Fig. 2.9



Magnetic field of linear varying current

Because / cosra φ= ,/tansa φ= , the modulus of magnetic induction intensity

vector at point P is:

021

22 22 22 22

21 21

sa sa sa sa

⎡⎤

⎛⎞⎛⎞

=− − + −

⎢⎥

⎜⎟⎜⎟

++ ++⎢⎥

⎝⎠⎝⎠

⎣⎦

•

(2.196)

where

and

are unknown constants, and their values can be determined by the

current at point A and B. Supposing the current of points A and B are I

and I

respectively, then:

−

12 21

Is Is

−

Replacing above

and

into Eq. 2.196, the magnetic induction intensity

at point P (B), can be calculated with values of s

ǃs

ǃI

. The components B

ǃB

can then be determined by cosine of

d ×sr.

  Ping Zhou, Feng Mei and Hui Cai

When the value of current I is a constant, there is:

02 1

22 22

4π

Is s

sa sa

⎛⎞

=−

⎜⎟

⎝⎠

(2.197)

2.4.3.2



Mathematical model of equivalent column current

Mathematical model of equivalent column current flow is to replace the

conductive element of a cross section in any shape with a conductive column. The

equivalence follows three principles:

a) the cross-sectional area stays the same, so the current density remains

unchanged;

b) the axis of the conductive column coincides with that of the real conductive

element;

c) the equivalent column conductor and the real one share the same ends, thus

have the same length.

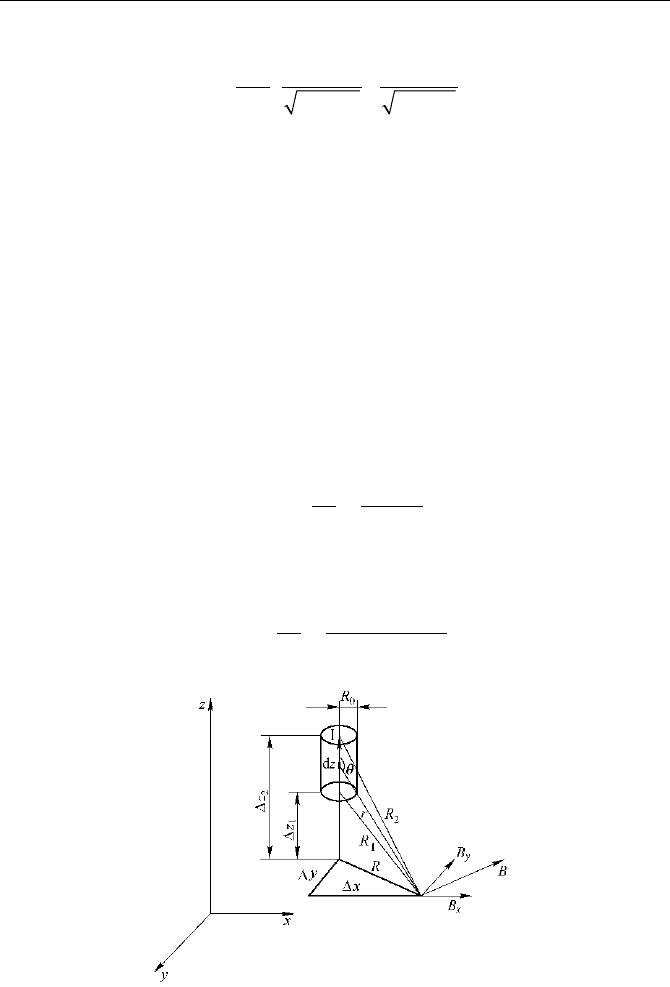
For a column conductor with radius R

and finite length (as shown in Fig. 2.10),

the magnetic induction intensity at point P in the space around it is (Feng, 1985):

∫

In Fig.2.10,

=+,

dsindz

×=zr ˈso, the modulus of the magnetic

induction intensity at point P is:

(s+)sind

∫

(2.198)

Fig. 2.10 Magnetic field of column current

Eq. 2.198 is only applicable to calculate the magnetic field outside the column

conductor (R

ıR

). Its form is the same as Eq. 2.195. When the interior magnetic

2 Modeling of the Thermophysical Processes in FKNME 

field of the column conductor is calculated, the magnetic induction intensity is:

= (0İRİR

)

where B

is the magnetic induction intensity when R=R

The above two mathematical models are equivalent. When the computed field

point is far away from the conductive element, the two models give the same

results with enough precision. When the conductive element is a column and the

point calculated is within the element, the results by the equivalent axis current

model will predict larger error, and the equivalent column current model should be

a better choice. When conductive element is rectangle (such as bus bar of

aluminum cell ) and the point calculated is within the element or near it, neither of

the models gives satisfactory result. In this case, a mathematical model for the

magnetic field in rectangular bus bar should be used.

2.4.3.3



Mathematical model for calculation of magnetic field in rectangular

bus bar

(for aluminum cells)

In order to improve the precision of calculation of the magnetic field in aluminum

cells, the problems of equivalent mathematical models mentioned above must be

overcome, that is, rectangular cross section of the bus bar in aluminum cells must

be taken into account. Hence, it would be better to compute the magnetic field in

aluminum cells, with a model in which the bus bar is a rectangular, with finite

length, and the current flowing though it is uniformly distributed. The model is

called the rectangular bus bar model of the magnetic field in aluminum cells, and

it is also applicable for magnetic fields of other rectangular conductive elements

(Liang,1990).

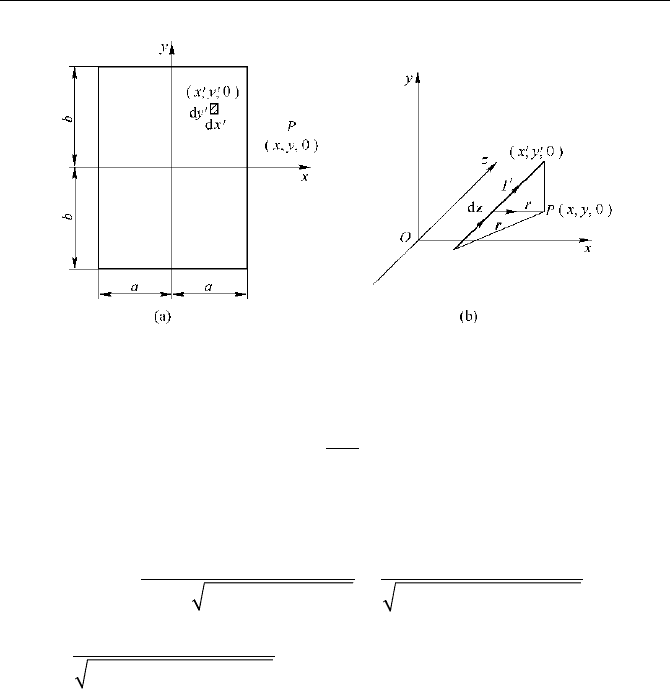
As shown in Fig. 2.11 (a), the bus bar is parallel to axis z, and the lengths of the

sides of the cross-section of the bus bar are respectively 2a and 2b. Meanwhile,

the length of the bus bar is L=z

z

, the current is I, and flows along axis z. Taking

a filament, of which the cross-sectional area is d 'd 'xy, the length is L, the current

is '

I and parallel to axis z (as illustrated in Fig. 2.11(b)). Then by Eq. 2.197, the

magnetic induction intensity caused by current '

I at the point P (x, y, 0) is:

π (' ) (' )

xx yy

=×

−+−

222

(' ) (' )

⎛

⎜

−

⎜

−+−+

⎝

222

(' ) (' )

⎞

⎟

−+−+

⎠

(2.199)

  Ping Zhou, Feng Mei and Hui Cai

Fig. 2.11 Magnetic field of rectangular bus bar

If the current in the rectangle is considered as uniformly distributed, then:

′′′

Replacing above equation into Eq.2.199 and integrating it, the magnetic

induction intensity at point

P is:

22 222

222

16π (' ) (' ) (' ) (' )

d'd'

(' ) (' )

ab x x

xx yy z

−−

⎛

⎜

=×−

⎜

−+− −+−+

⎝

⎞

⎟

−+−+

⎠

∫∫

With cosine of

d ×

r , the components B

, B

can then be obtained with the

result of Eq.2.200. When the magnetic induction intensity of any point P(x, y, z) is

to be computed in the cell, the coordinate should be changed first, that is, to move

the origin of the coordinate upwards (or down words) along axis z to point z, so

that the new coordinate of point P can be changed into P(x, y, 0), before the

magnetic field of the current carrying bus bar can be computed with the above

method.

2.4.4



Magnetic field models of ferromagnetic elements

Because of the existence of ferromagnetic materials, the influences of

ferromagnetic shield must be considered as well so that the magnetic field in the

furnaces can be simulated correctly. Two research techniques can be used to study

the influence of ferromagnetic elements on the magnetic field: the analogy method

and the numerical simulation method. The latter can be further classified as

(2.200)

2 Modeling of the Thermophysical Processes in FKNME 

differential equation method, integral equation method and hybrid method. There

is another method called the magnetic shielding factor method, which is

considered as an approach between the analogy method and the numerical

simulation method.

2.4.4.1



Analogy method

Analogy model is based on the analogy principle. It is a model that transforms

industrial data into laboratory model, with which the influence coefficients of

ferromagnetic material on the magnetic field can be studied, thus the value of

magnetic induction intensity of corresponding points in the cells can be computed.

The analogy model is particular useful when new furance is to be developed but

no reliable data is available to serve as development guidelines. This method

requires, however, particular analogy model be built up for each new furnace

development work and the model be physically identical to the prototype. These

requirement makes the analogy method very expensive and time-consuming.

2.4.4.2



Magnetic shielding factor method

If there are magnetic materials between the conductive element and the point to be

computed, the magnetic field will be weaker than that when there is no magnetic

material. By introducing parameter MAF, the magnetic field B with magnetic

materials can be computed from the magnetic field B

that is without magnetic

material:

B=MAF • B

where MAF is the magnetic attenuation factor, and usually MAF

İ1, but for

nonmagnetic material, MAF=1. Generally, MAF is relating to the shape, size and

relative permeability of the magnetic materials.

MAF can be determined by image method principle or model experiment.

2.4.4.3



Numerical method

Differential equation method

The intensity of the magnetic field at one point in the space (H) comes from H

and

, of which H

is the field intensity produced by the current in the space, and

is the field intensity caused by the ferromagnetic material.

H= H

+ H

If to describe the field by simplified scalar potential (

Φ is scalar potential), then

there is:

=−∇Φ

If the field is described by Poisson equation, then there is:

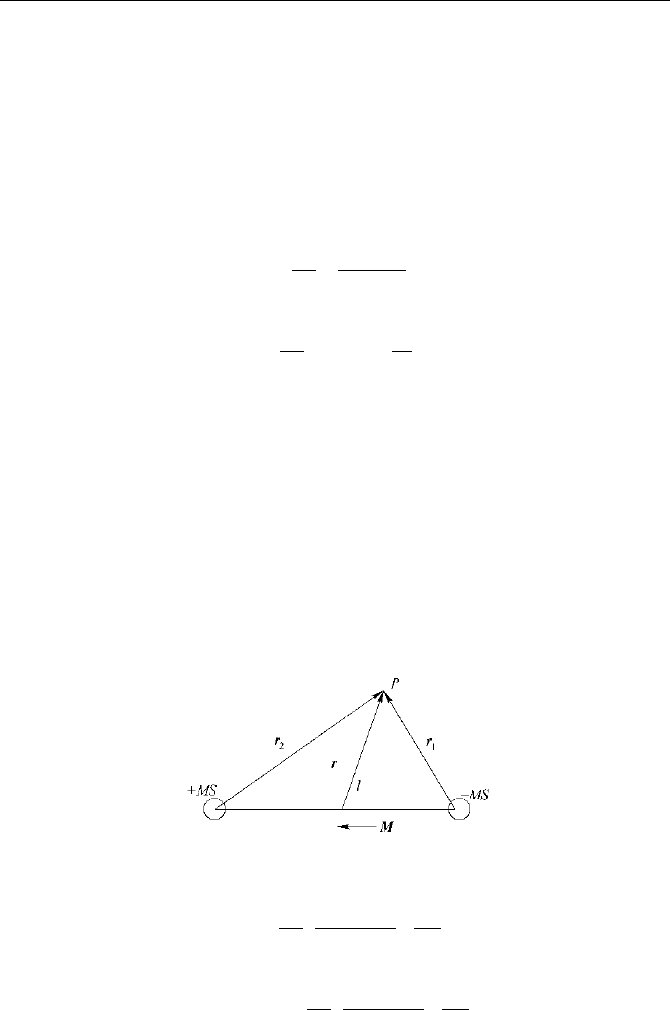
  Ping Zhou, Feng Mei and Hui Cai

∇∇•

Φ =

−∇ • H (2.201)

With proper boundary conditions, Eq. 2.201 can be solved with finite-element

method or finite difference method. This is the differential equation method.

Integral equation method

The field can be described with integral as (Fan and Yan, 1988):

1()

′

∫

(2.202)

∇

⎛⎞

=−

⎜⎟

⎝⎠

∫

••

(2.203)

where M is the magnetic polarization, m

//()qV sl=∇=Ml p , and p

is the

magnetic moment.

As in Eq.2.203,

M =B /

− H, M is a function of H, and the equation can be

solved by discretization within the whole field.

When the ferromagnetic material is simplified as a pair of magnetic dipole, it

can only be polarized along the axis, and this method is called the equivalent

magnetic dipole method.

As shown in Fig.2.12, the magnetic density and magnetic induction intensity

produced by the magnetic dipole at the point P is:

Fig. 2.12 Drawing for magnetic dipole

3( )

⎡⎤

=−

⎢⎥

⎣⎦

•prrp

3( )

⎡⎤

== −

⎢⎥

⎣⎦

•prrp

where

=SMlˈ in which S is sectional area of magnetic dipole, and M is

polarization of that.

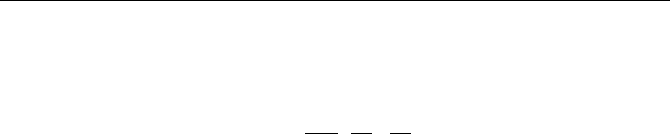
2 Modeling of the Thermophysical Processes in FKNME 

The above equation must satisfy the condition that r (the distance from point

P to the center of the magnetic dipole) is much longer than l. When the condition

is not satisfied, there is:

4π

⎛⎞

=−

⎜⎟

⎝⎠

Combination method

The hybrid method includes integral-differential equation method and

differential-integral equation method. Both methods need to eliminate unknown

variables by the boundary conditions at the interfaces. The major difficulty,

however, is how to determine such boundary conditions.

2.4.5



Three-dimensional mathematical model of magnetic field

In simulation of the magnetic field of aluminum reduction cells, there is a more

reliable method, that is the three-dimensional model which solve the magnetic

fields in both the bus bar and the cell with the Maxwell equation as:

∇× =

0∇=• B

The above equation can be solved with double scalar quantity method. The

three-dimensional model can be used to compute the distribution of the magnetic

field of ferromagnetic materials including steel cell shell.

2.5



Simulation on Melt Flow and Velocity Distribution in

Smelting Furnaces

The extracted metals or ores often appear as molten state in the process of

extracting and refining metal, such as the liquid aluminum in reduction cells,

copper (or nickel) matte in electric smelting furnace and liquid steel in tundish.

The smelting processes of these metals comprise heat transfer, momentum transfer

and mass transfer as well as chemical reaction in smelting equipment. And there

are inter-coupling actions among these transport phenomena. When reaction

system is determined, it is necessary to study how to determine flow, heat transfer

and mass transfer conditions of the fluid in order to improve metallurgical

reactions or machining processes. Among others, the flow process of high

temperature melts in the smelting furnace takes an important role in the efficiency,

rates of metallurgical reaction and the life of equipments.

It is not until recently that more and more attention has been paid to the

  Ping Zhou, Feng Mei and Hui Cai

concept of fluid flow on the research of metal smelting process. While the

traditional research methods used in fluid dynamics are still widely applied in

high temperature melts numerical simulation is becoming more and more

popular in studying complicated fluid flow in the metallurgy applications all

over the world.

2.5.1



Mathematical model for the melt flow in smelting furnace

The melt flow can be described by means of Navier-Stokes equations and the

tensor form in Cartesian coordinate system can be written in following.

Continuity equation:

()0

∂∂

(2.204)

The melt in smelting furnace can be seen as incompressible fluid, then fluid

divergence is equal to zero. Based on the generalized Newton’s viscosity law, the

momentum equation of the melt can be got:

eff si

() ( )

iji

jijji

uuu F

tx xxxx

ρρ μ

⎡⎤

⎛⎞

∂

∂∂ ∂∂

+=−+ ++

⎢⎥

⎜⎟

∂∂ ∂∂∂∂

⎢⎥

⎝⎠

⎣⎦

(2.205)

The sensible enthalpy equation (refer to Section 2.3.1) can be given by:

() ( )

jjHj

HuH Q

tx txcx

ρρ

⎡⎤

⎛⎞

∂∂ ∂∂ ∂

+=−+++

⎢⎥

⎜⎟

∂∂ ∂∂ ∂

⎢⎥

⎝⎠

⎣⎦

(2.206)

where

is melt density; t is time; p is pressure; u

˄j=1,2,3˅ is melt velocity

components in three coordinate directions;

eff

is efficient viscosity˄the sum of

the molecule viscosity and turbulent viscosity, or

˅; F

is the body force

components acting on the melt˄including gravity, buoyancy and electro-magnetic

force˅; H is sensible enthalpy;

is melt thermal conductivity; c

is melt specific

heat;

is Prandtl numbers of enthalpy; Q

is chemical reaction heat.

Above equations (including continuity, momentum and enthalpy equation)

comprise the basic equations used in studying turbulence of high temperature melt.

The fundamental methods to solve these equations are direct numerical simulation

(DNS), large eddy simulation (LES) and the Reynolds-averaged simulation (RAS).

But the former two need to take quite many computer capacity and CPU time, and

there are definite gap from solving the practical problems. At present, RAS is the

most economic and efficient method used in solving practical engineering

problem.

The basic thought of RAS is to use lower order relevant variables and feature of

time-averaged flow to model the unknown higher order relevant variables, which

make the time-average equations or correlation equations closed. In the engineering