Mei C., Zhou J., Peng X. Simulation and Optimization of Furnaces and Kilns for Nonferrous Metallurgical Engineering
Подождите немного. Документ загружается.


Modeling of
the Thermophysical
Processes in FKNME
Ping Zhou, Feng Mei and Hui Cai
The fluid (including molten mass) flow, heat transfer and combustion processes in
the FKNME are collectively called as the FKNME thermal processes. The
modeling of these processes is the foundation to simulate the FKNME as well as
the key element. In this chapter we discuss the theories and applications of the
modeling of the flow filed, temperature field, species concentration field and
electro-magnetic field that are usually involved in the FKNME.
2.1
Modeling of the Fluid Flow in the FKNME
As one aspect of the thermal processes, fluid flow in the FKNME is usually
accompanied with combustion and heat transfer, thus it is quite complicated and
difficult for modeling. As a result, different models have then been developed and
proposed to describe and investigate the complex process in the FKNME.
2.1.1
Introduction
In the FKNME applications large variety of fluids (gases, molten mass, gas-particle mix
etc.) and flow patterns have been involved. For the convenience of investigation, we
generally classified them per their fluid dynamics characteristics as shown in Table 2.1.
Table 2.1 Patterns of fluid flow usually applicable to FKNME
Examples
Patterns
Single phase
Two-phase
(gas-particles)
Characteristics of
the flow field
Pipe flow
Gas transportation pipes
Molten mass transportation
pipes
Pneumatic
transportation
pipes
1D turbulent flow
1D laminar flow
or turbulent flow

Ping Zhou, Feng Mei and Hui Cai
Continues Table 2.1
Examples
Patterns
Single phase
Two-phase
(gas-particles)
Characteristics of
the flow field
Simple jet
Straight flame
burners
Pulverized coal
burners
2D or 3D turbulent
flow
Jet flow
Swirling jet Flat flames burners
Swirling
pulverized coal
burners
3D turbulent flow
Recirculation flow
Recirculation zones
in the flame furnaces
Stirring of the melting
pool
Electro-magnetic
flow in the Al redu-
ction cell
Upper part of the
flash smelting
furnaces
Tangentially
fired burners
3D turbulent flow
Gas-particle two-phase
flow
Dense phase flu-
idized bed
Circulating
fluidized bed
One of 3D or 1D
turbulent flows
Table 2.1 illustrates that the fluid flows in the FKNME are dominated by
complex turbulent flows. In this section we chiefly discuss the incompressible
steady-state turbulent flows with Mach number far less than 1. The recirculation
flows of the molten mass in the smelters are to be covered in Section 2.5 and the
general fluidized beds are to be discussed in Chapter 8.
Different from the laminar flows that are usually investigated at the molecular
motion level, turbulent flows are mainly investigated at the molecular
micro-conglomerations (called eddies) level with main interests on the generation,
transportation, breaking up and interactions of these conglomerations. Up to date
we have still not fully understood the turbulence phenomena. We still have
difficulty to describe them in a perfect mathematic way. From a physical point of
view, however, turbulence can be generally viewed as a set of eddies in a large
range of sizes. The large eddies are usually situated in the middle of the flows to
carry out the major part of the energy. The small ones are usually close to the
confined boundaries where energy is dissipated. Larger eddies are strongly
anisotropic whereas the smaller ones are more homogeneous. Smaller eddies are
usually generated through the break down of larger eddies, in the meantime energy
is transferred from larger eddies to the smaller ones. The energy containing in an
eddy falls dramatically when the size of the eddy is below certain criterion level,
which is measured by the Kolmogorov scale. Our major interest is the turbulence
phenomena above the Kolmogorov scale level.
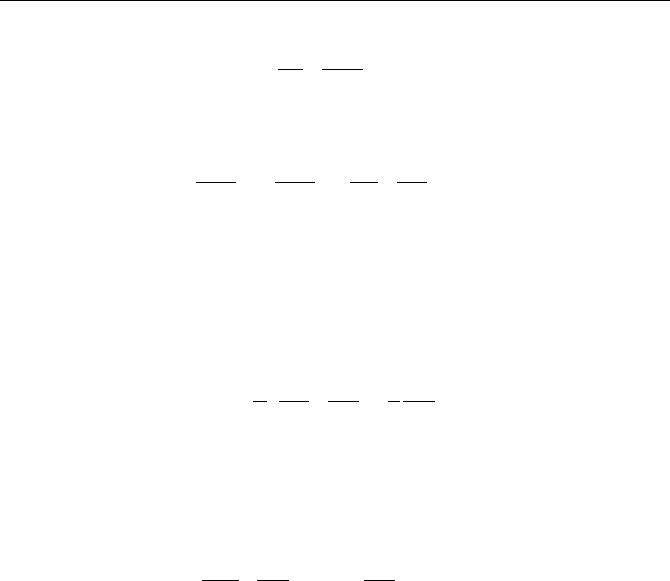
The governing equations set of the viscous flows consists of the conservations of
mass, momentum and other related scalars, as shown in Eq.2.1~Eq.2.6.
2 Modeling of the Thermophysical Processes in FKNME
The mass conservation (or called continuity equation):
0=
∂
∂
+
∂
∂
i
i
x
u
t
ρ
ρ
(2.1)
where
i
u is the instantaneous velocity on the i direction.
The momentum conservation equation:
i
j
ij
ij
i
j
i
g
x
t
x
p
x
u
u
t
u
ρ
ρρ
+
∂
∂
+
∂
∂
−=
∂
∂
+
∂
∂
(2.2)
where
p is the instantaneous static pressure;
ρ
g
i
is the gravity on i direction (this
term is usually ignored when forced flow predominates);
ij
t is the viscous stresses
tensor, which is defined as:
ijij
st
0
2
μ
= (2.3)
where
0
μ
is the molecular viscosity,
ij
s is the strain rate tensor, which is defined as:
ij
k
k
i
j
j
i
ij
x
u
x
u
x
u
s
δ
∂
∂
−
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
+
∂
∂
=
3
1
2
1
(2.4)
where
ij
δ
is the so-called Kronecker number:
⎩
⎨
⎧
=
≠
=
ji
ji
ij
,1
,0
δ
(2.5)
The scalar conservation equations are generally written as:
() ()
ϕϕ
ϕρ
ϕ
SJ
x
u
xt
p
j
j
j
+
∂
∂
=
∂
∂
+
∂
∂
(2.6)
where
ϕ
is the concerned scalar of any kind; J
φ
is the diffusion flux of
ϕ
on the
j direction; S
φ
the source term of the scalar
ϕ
. The form of Eq.2.6 is principally
applicable to all conservative scalars. This makes it possible to develop a general
problem-solving scheme that first establish conservative equations for a selected
number of scalars in the form of Eq.2.6 and then solve the equations set using the
same numerical procedure. The difficulty of this scheme is, however, that the
source terms of these equations are often difficult to be treated.
The computational capacity of modern computers still does not allow us to
solve the equations set with instantaneous variables. Even though large eddy
simulation (LES) has been increasingly used in the recent years, the modeling
approach stays to be the mainstream in most engineering applications. With the
modeling approach, the instantaneous form of the equations set has to be
transformed into a time-averaged form before it is numerically solved.
2.1.2
The Reynolds-averaging and the Favre-averaging methods
The Eq.2.1, Eq.2.2 and Eq.2.6 are called the general Navier-Stokes equations set.
This equation set is usually solved by the Reynolds averaging method is normally
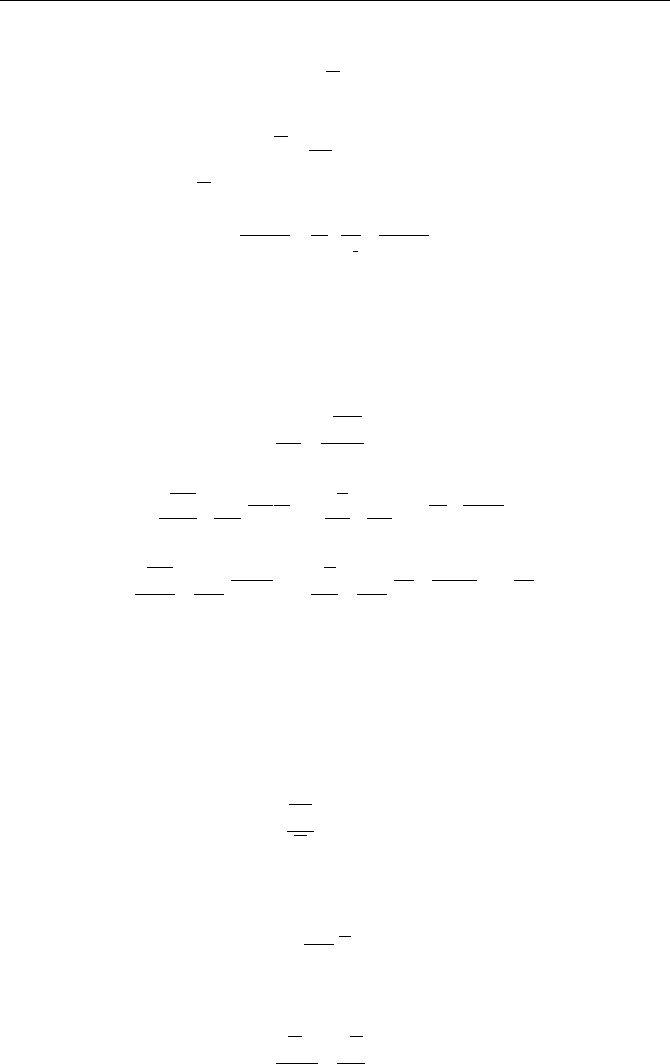
Ping Zhou, Feng Mei and Hui Cai
applied. The Reynolds averaging method, which defines an instantaneous variable
as a sum of a time-averaged part and a fluctuating part:
ΦΦΦ
′
+= (2.7)
where
Φ is the time-averaged
ϕ
:
∫
Δ
Δ
=
t
t
t
Φ
0
d
1
ϕ
(2.8)
Being aware that
ϕ
′
=0, we can simplify the production of the two
instantaneous quantity into
12 1 12
2
ϕϕ ϕϕ ϕϕ
•••
′′
=+ (2.9)
Substituting Eq.2.7 and Eq.2.9 into the equation set Eq.2.1~Eq.2.6 we come
down with the Reynolds averaging Navier-Stokes equations set as shown in
Eq.2.10~Eq.2.12 by assuming that the higher order fluctuation terms are ignorable
and the fluctuation of density is not significantly correlated with the other
variables.
0=
∂
∂
+
∂
∂
i
i
x
u
t
ρ
ρ
(2.10)
()
)2(
0 jiij
ii
ji
j
i
uuS
xx
p
uu
xt
u
′′
−
∂
∂
+
∂
∂
−=
∂
∂
+
∂
∂
ρμρ
ρ
(2.11)
() ()
ϕ
ρρρϕ
ρϕ
SuuJ
xx
p
u
xt
jik
ji
j
j
+
′′
−
∂
∂
+
∂
∂
−=
∂
∂
+
∂
∂
(2.12)
Removing the density-correlated terms should not bring in substantial errors so
long as the density does not vary remarkably. This is apparently not the case if
combustions and (or) chemical reactions involve. Theoretically speaking the Favre
averaging (or called weighted averaging), is considered more appropriate.
Different from the Reynolds averaging, the Favre averaging includes the density
as a weighing factor into the definition of the averaged part of the variables:
ρ
ρ
ϕ
u
=
~
, φφ=φ
~
′′
(2.13)
The Favre averaging enables the elimination of the fluctuation term in the
continuity equation without simplification. The convective term turns to be:
u
x
j
~
ρ
∂
∂
The Favre averaging method leads to the following mass, momentum and scalar
conservation equations set:
0=
∂
∂
+
∂
∂
tx
u
i
i
ρ
ρ
(2.14)

2 Modeling of the Thermophysical Processes in FKNME
)2()
~~
()
~
(
0 jiij
ji
ji
j
i
uuS
xx
p
uu
x
u
t
′′′′
−
∂
∂
+
∂
∂
−=
∂
∂
+
∂
∂
ρμρρ
(2.15)
where Reynolds averaging S
ij
term:
ij
k
k
i
j
j
i
ij
x
u
x
u
x
u
S
δ
∂
∂
−
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
+
∂
∂
=
3
1
2
1
(2.15a)
ϕϕ
ρϕρϕρϕ
ρ
SuJ
x
u
xt
j
j
j
j
+
⎟
⎠
⎞
⎜
⎝
⎛
′′′′
−
∂
∂
=
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
+
∂
∂
~~~
(2.16)
the transport flux of scalar
ϕ
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
=
j
x
J
ϕ
δ
μ
ϕ
ϕ
0
(2.16a)
Density-related correlation terms are eliminated by the Favre averaging but the
Reynolds averaging viscous stresses terms in Eq.2.15 and the viscous scalar flux
terms in Eq.2.16 stay. These terms are usually treated by one of the following two
means in most engineering practices. The first means is to ignore the viscous
stresses effects under the high Reynolds number assumption therefore Eq.2.15a
and Eq.2.16a are entirely eliminated. The other means is to assume, in case that
viscous stresses are not ignorable, that the results of Favre averaging is identical to
that of Reynolds averaging, namely
0
~
uu = .
There is no clear-cut rules that can decide whether the Reynolds averaging
method or the Favre averaging method should be used in a specific circumstance.
Generally speaking, Favre averaging method is more computationally
“economical”, which is an important advantage when substantial density variation
must be considered. The velocities measured by pitot tubes or hot wires are closer
to the Favre averaging, meanwhile Laser Doppler Velocimeters technique is more
likely to measure Reynolds averaging quantities. The temperature measured by
thermal couples should be closer to the Favre averaging. This is because the
measurements determined by contacting-natured measuring methods are closely
associated to the kinetic energy of the fluid flow and therefore are more likely to
be reflected by Favre averaging method.
For practical reasons the discussion here-after will only use the Reynolds
averaging form and the averaging bar will be also left out.
2.1.3
Turbulence models
Applying the averaging process considerably simplifies the computation but also
bring up new unknown quantities, which are:
ji
uu
′′′′
ρ
or
j
u
′′
ϕρ
These two terms are called the turbulent stresses and the turbulent scalar flux.

Ping Zhou, Feng Mei and Hui Cai
The objective of modeling turbulence is to correlate these terms with any known
variables so that the equations set can be closed. Starting from Boussinesq who
proposed the first turbulence model, the investigation of turbulence modeling
has gone through a history over 100 years. There have been a large number of
turbulence models and turbulence theories. Mathematically there are algebraic
models and differential models. Physically there are turbulent viscosity models
and Reynolds stresses models. Influenced by the availability of computation
capacity and inclinations towards certain theories, each age has its “favorable”
models.
The revolutionary development of computer technology has dramatically
reshaped the landscape of CFD (computational fluid dynamics) from the 1990s.
Many models that were developed mainly to simplify computation have gradually
faded out. The winners are those either robust enough for wide range of
applications or accurate in predicting some specific problems. The interest of this
book focuses on a few
k-
ε
models that have been widely used in engineering
practice, followed by a brief introduction of the Reynolds Stresses models. The
readers are referred to other textbooks and monographs for other classic turbulent
models such as the Prandtl Mixing Length model from the Algebraic Turbulent
Stresses model group, the single equation turbulence model from the Differential
Turbulent Stresses model group or the algebraic stresses model from the Reynolds
Stresses model group.
Boussinesq assumed that the turbulent stresses be proportional to the mean
velocity gradient. He defined the turbulent stresses in analogy to molecular
viscous stresses:
ijij
k
n
i
i
i
i
Tjiij
k
x
u
x
u
x
u
uu
δρδμρτ
3
2
3
2
−
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
−
∂
∂
+
∂
∂
=
′′
−=
(2.17)
where
T
μ is a hypothetic “turbulent viscosity”, which is not a physical property of
the fluid but a local variable depending on the turbulent flow. Introducing this
turbulent viscosity re-directs the investigation interest from how to determine
turbulent stresses to how to determine turbulent viscosity, from which various
k-
ε
models have been raised.
A very nature idea when we are studying turbulence is to measure the
turbulence by its kinetic energy. The definition of turbulent kinetic energy is:
k =
jj
uu
′′′′
2
1
Or written in the Cartesian coordinate system as
⎟
⎠
⎞
⎜
⎝
⎛
′
+
′
+
′
=
222
2
1
zyx
uuuk (2.18)
Turbulent kinetic energy measures the intensity of turbulence in the three
directions. From the viewpoint of turbulence microstructure, the turbulent energy
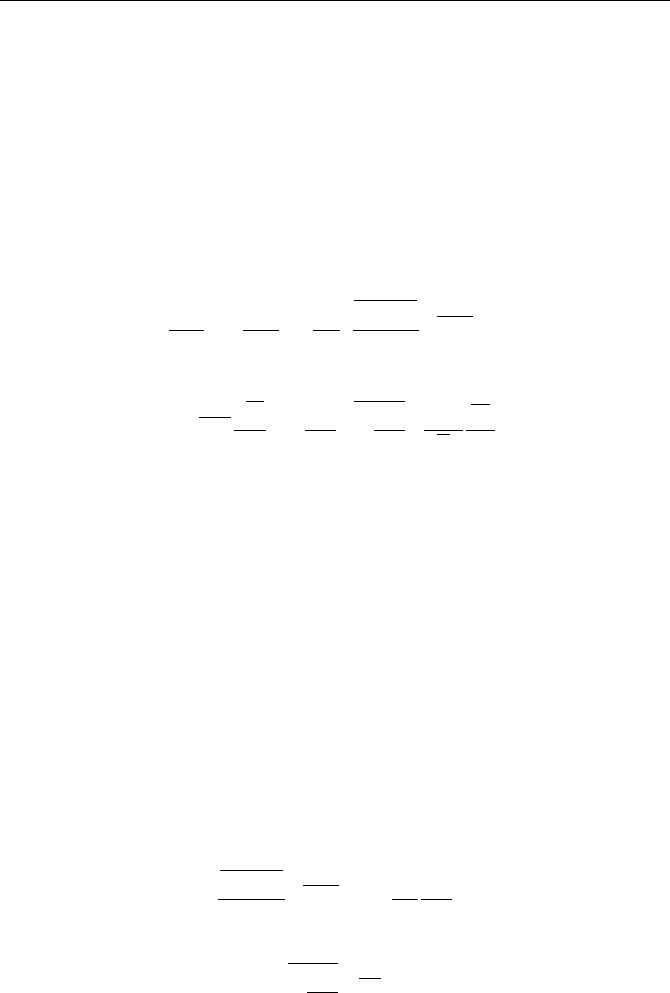
2 Modeling of the Thermophysical Processes in FKNME
is mainly stored in large scale eddies. Therefore k
1/2
is also an indication of the
large eddies. Through dimension analysis the Kolmogorov-Prantl expression is
obtained:
lkC
T
2/1
μ
ρμ
=
(2.19)
where
l is the characteristic length of the turbulence,
μ
C is a constant,
ρ
is the
density.
Eq.2.19 indicates that
T
μ
is obtainable so long as k and l can be determined.
Kolmogorov and Prantl derived the accurate transport equation for
k out of the
Navier-Stokes equation. Under high Reynolds number condition, the mean
turbulent kinetic energy equation becomes:
2
( ) (II) (III) (IV)
ijj
jjij
ji
uuu
kk
upu
txx
ρ
ρρ
δ
⎛⎞
′′ ′
∂∂ ∂
′′
⎜⎟+=− +
⎜⎟
∂∂∂
⎝⎠
,
(V) (VI) (VII) (VIII)
jjj
k
ij ij
iki j
uuu
u
p
uu p
xxx x
ρ
ρτ
ρ
′′′
∂∂
′
∂
∂
′′ ′
−+−+
∂∂∂ ∂
(2.20)
The physical meaning of each term as follows:
(
, ) The transitional effect
(
II ) The mean velocity-based convective term
(
III ) The diffusion transport of the fluctuation of turbulent kinetic energy
(
IV ) The diffusion transport of the fluctuation of pressure
(
V ) The contribution of shear stresses to the generation of k, which is the
interaction of the local fluctuation and mean flow
( VI ) A source of turbulence noise, which is usually ignored under low Mach
number
( VII ) The part of viscous dissipation that is transformed into internal energy
( VIII ) The generation of turbulent energy due to the density fluctuation caused
by pressure gradient
The above terms are modeled as follows:
( III + IV ) the diffusion term:
kk
T
ijj
jj
i
x
k
up
uuu
∂
∂
−=
′′
+
′′′
σ
μ
δ
ρ
2
(2.21)
( VII ) the dissipation term:
ρετ
=
∂
′
∂
i
j
ij
x
u
(2.22)
( V + VIII ) the generation term:

Ping Zhou, Feng Mei and Hui Cai
ji
T
i
j
ij
k
k
T
i
i
i
i
T
xx
p
x
u
k
x
u
x
u
x
u
G
∂
∂
∂
∂
−
∂
∂
⎥
⎥
⎦
⎤
⎢
⎢
⎣
⎡
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
+
∂
∂
−
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
+
∂
∂
=
ρ
ρ
μ
δρμμ
2
3
2
(2.23)
Under minor density variation, Eq.2.23 is further simplified into:
i
j
ij
i
i
i
i
T
x
u
k
x
u
x
u
G
∂
∂
⎥
⎥
⎦
⎤
⎢
⎢
⎣
⎡
−
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
+
∂
∂
=
δρμ
3
2
(2.24)
Substituting above correlations into Eq.2.20 gives the final
k transport equation
ρε
σ
μ
ρ
ρ
−+
∂
∂
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
=
∂
∂
+
∂
∂
G
x
k
xx
ku
t
k
jk
T
jj
j
(2.25)
The next task is to determine the local characteristic length of turbulent energy.
Many models have been proposed but the most successful one so far came from P.
Y. Zhou (Zhou,1945), Davidor et al. with their correlation between turbulence
dissipation
¦
to l and k:
2/3
~ kl (2.26)
2
T
Ck
μ
μρ
ε
= (2.27)
The interest is now redirected to determining
ε
. The derivation of the transport
equation for
ε
is similar to that for k, namely we first derive the accurate
equation followed by a number of modeling treatments to close the equaiton. What
is different is that the accurate equation for
ε
is much more complicated than
that for
k. Under high Reynolds number and local balance assumptions, the
transport equation for
ε
is written as:
)()(
21
ρε
εε
σ
μ
ερ
ρε
εε
ε
CGC
kxx
u
xt
j
T
j
j
j
−+
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
∂
∂
=
∂
∂
+
∂
∂
(2.28)
The equations set Eq.2.25~Eq.2.28 is the most frequently used
k-
ε
two-equation
model or called the standard
k-
ε
model. The constants employed in the model
are listed in Table 2.2.
Table 2.2 Constants for k-
ε
equations
Constant
μ
C
1
ε
C
2
ε
C
k
σ
ε
σ
Value 0.09 1.44 1.92 1.0 1.3
The equations for the turbulent transport of scalars can be closed in analogy to
the way closing the turbulent stresses. The turbulent transport of a scalar is
assumed proportional to the local gradient of the scalar, namely:
=
′′
−
j
u
ϕρ
φ
j
x∂
∂
ϕ
(2.29)
where
φ
is the turbulent diffusion coefficient, which is assumed proportional to
the turbulent viscosity:

2 Modeling of the Thermophysical Processes in FKNME
φ
=
ϕ
σ
μ
T
(2.30)
where
σ
φ
is the turbulent Prandtl number of the concerned scalar. Same to the
turbulent viscosity, the turbulent diffusion coefficient is not a physical property but
a variable depending on the process. It is worthwhile to mention that the present
analogy approach is not merely out of the need of simplification but is also backed
by some experimental observations. As it is widely known, the temperature field
and the flow field are indeed found similar under certain conditions.
The transport equations for vectors and scalars can be uniformly written as
j
j
j
x
u
xt ∂
∂
=
∂
∂
+
∂
∂
)(
ϕρ
ρϕ
ϕ
ϕ
S
x
Γ
j
φ
+
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
(2.31)
where
φ denotes any variables including velocities, k,
ε
, energy, components etc.
S
φ
denotes the source term and
φ
the diffusion coefficient. Eq. 2.31 is called the
generalized turbulent Navier-Stokes equations set. Table 2.3 and Table 2.4 list the
detailed settings of Eq. 2.31 in two-dimensional Cartesian coordinates and
Cylindrical coordinates.
Under the cylindrical coordinates, the generalized NS equations set is written as:
φφφrz
S
r
Γr
zz
Γr
zr
vr
r
vr
zrt
+
⎥
⎦
⎤
⎢
⎣
⎡
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
+
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
=
⎥
⎦
⎤
⎢
⎣
⎡
∂
∂
+
∂
∂
+
∂
∂
ϕϕ
ϕρϕρ
ρϕ
1
)()(
1)(
(2.31a)
The standard
k-
ε
model has been proven robust and widely applicable through
long time and extensive tests by practical use, which makes it the so far the most
widely recognized turbulence model. However, this model may result in
unsatisfactory predictions if one or more following conditions is (are) applicable:
a) Strong swirling flow.
b) Buoyancy flow.
c) Gravity separation flow.
d) Curving wall boundary.
e) Low Reynolds number flow.
f) Non-homogenous turbulent flow.
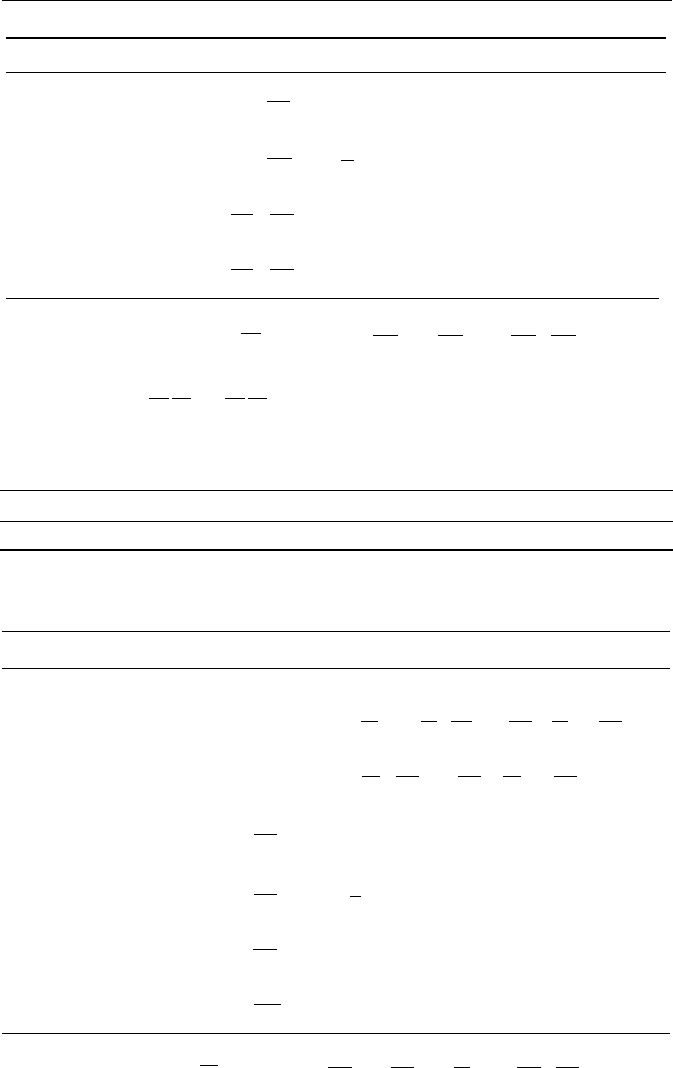
Table 2.3 The expressions of Eq.2.31 in two dimensional Cartesian coordinates
ķ
Equations
ϕ
φ
S
φ
Continuty 1 0 0
x-momentum
y-momentum
x
u
y
u
()
T
μ
μ
μ
+=
0eff
()
T
μ
μ
μ
+=
0eff
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
∂
∂
+
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
+
∂
∂
−
x
u
yx
u
xx
p
y
x
effeff
μμ
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
∂
∂
+
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
∂
∂
∂
∂
+
∂
∂
−
y
u
yy
u
xy
p
y
x
effeff
μμ
Ping Zhou, Feng Mei and Hui Cai
Continues Table 2.3
Equations
ϕ
φ
S
φ
Turbulent
kinetic
energy
k
k
T
σ
μ
μ
+
0
ρε
−+
bk
GG
Turbulence
dissipation
¦
ε
σ
μ
μ
T
+
0
()
ρε
ε
εε
21
− CGC
k
k
Energy h
0
T
h
Pr
μ
μ
σ
+
q
r
(heat effect of radiation or chemical
reaction)
Species
γ
0
T
r
Pr
μ
μ
σ
+
¹
s
(generation of chemical reaction or
combustion)
ķ
eff
2
0
,
TT
k
C
μ
μμμμ ρ
ε
=+ =
;
22
2
2
yy
xx
kT
uu
uu
G
xy yx
μ
∂∂
∂∂
=+++
∂∂ ∂∂
⎧⎫
⎡⎤
⎛⎞⎛ ⎞
⎪⎪
⎛⎞
⎢⎥
⎨⎬
⎜⎟⎜ ⎟
⎜⎟
⎝⎠
⎢⎥
⎝⎠⎝ ⎠
⎪⎪
⎣⎦
⎩⎭
;
TT
bx y
TT
TT
Gg g
x
y
μμ
βρ
σσ
∂∂
=− +
∂∂
⎛⎞
⎜⎟
⎝⎠
;
where g is the gravity; £ is the gas volumetric expansion coefficient.
The values of the constants are given as follows:
μ
C
1
ε
σ
2
ε
C
k
σ
ε
σ
h
σ
y
σ
T
σ
0.09 1.44 1.92 1.0 1.3 0.9 0.9 0.9
Note in some literatures, the value of
ε
σ
is set as 1.11, and that of
h
σ
is 0.7.
Table 2.4 The expressions of Eg.2.31 in two dimensional cylindrical coordinates
Equations
ϕ
φ
ϕ
S
Continuity 1 0 0
r-momentum
r
u
eff
μ
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
+
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
+−
∂
∂
−
r
u
zr
u
r
rrr
u
r
p
zrr
effeff
2
eff
2
μμμ
z-momentum
z
u
eff
μ
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
+
⎟
⎠
⎞
⎜
⎝
⎛
∂
∂
∂
∂
+
∂
∂
−
z
u
yz
u
r
rrz
p
zr
effeff
μμ
Turbulent
kinetic
energy
k
k
T
σ
μ
μ
+
0
ρε
−+
bk
GG
Turbulence
dissipation
ε
ε
σ
μ
μ
T
+
0
()
ρε
ε
εε
21
− CGC
k
k
ķ
Energy
h
T
T
σ
μ
μ
+
0
r
q−
Species
Y
Y
T
'
0
σ
μ
μ
+
s
ω
−
ķ
eff 0
2
;
TT
k
C
μ
μμμμ ρ
ε
=+ =
;
222 2
2
zrr zr
kT
uuu uu
G
zrr rz
μ
∂∂ ∂∂
=++++
∂∂ ∂∂
⎧⎫
⎡⎤
⎪⎪
⎛⎞⎛⎞⎛⎞⎛ ⎞
⎢⎥
⎨⎬
⎜⎟⎜⎟⎜⎟⎜ ⎟
⎝⎠⎝⎠⎝⎠⎝ ⎠
⎢⎥
⎪⎪
⎣⎦
⎩⎭
.
