Mei C., Zhou J., Peng X. Simulation and Optimization of Furnaces and Kilns for Nonferrous Metallurgical Engineering

Подождите немного. Документ загружается.

5 Hologram Simulation of Aluminum Reduction Cells 

eff eff eff

221/2

eff

( )

vuvvv p v v u

txy yx xy yx y

uv v F

yyyH

ννν

⎛⎞⎛⎞

∂∂∂ ∂∂∂∂∂∂∂

⎛⎞

++=− + + + +

⎜⎟⎜⎟

⎜⎟

∂∂ ∂ ∂∂ ∂ ∂ ∂ ∂ ∂

⎝⎠

⎝⎠⎝⎠

⎛⎞

∂∂ ∂

−− + +

⎜⎟

∂∂∂

⎝⎠

(5.40)

where

F and

F are the electromagnetic forces of the unit volume;

C is

friction resistance coefficiency between the melt and the wall;

H is the depth of

the melt layer. To describe the turbulent flow, k-

equations are used and averaged

along the depth of the melt layer.

eff eff

() ()

νν

σσ

⎛⎞⎛⎞

∂∂ ∂ ∂ ∂ ∂ ∂

++= + ++−

⎜⎟⎜⎟

∂∂ ∂ ∂ ∂ ∂ ∂

⎝⎠⎝⎠

kkv

kkk

uk vk P P

tx y x x y y

(5.41)

eff eff

() ()

εε

νν

εεεεε

εε

σσ

⎛⎞⎛⎞

∂∂ ∂ ∂ ∂ ∂ ∂

++= + ++−

⎜⎟⎜⎟

∂∂ ∂ ∂ ∂ ∂ ∂

⎝⎠⎝⎠

uv CPPC

(5.42)

eff

νννν

=+ =+

(5.43)

where

C =1.44,

C =1.92,

=1.0,

=1.3,

C =0.09. The initial conditions

are considered as static, and the boundary conditions are solid, non-slip walls.

Solutions of above equations should be jointed with wall functions on the side

walls.

With the Navier-Stocks equations, the height of two molten liquid layers (as

shown in Fig. 5.19) can be obtained by averaging the momentum equation of

direction:

me m m e e

mm ee

( ) [( ) ( ) ( )]

()()

FHh

gF gF

ρρ

−− − + − −

′

−− −

••

(5.44)

where

and

P are the static

pressure respectively at the bottom

surface of the molten aluminum and the

top of the electrolyte;

and

are

the densities of the molten aluminum and

electrolyte;

and

are vertical

electromagnetic force in the molten

aluminum and electrolyte (the vectors

point to z direction, for solution of the

vectors, please refer to Section 5.2); and

is the gravity.

The above equations can be discretized with finite difference method. Using

SIMPLE algorithm and staggered-grid, the equations can be solved by assigning

velocity field and pressure field at different nodes. In references (Sun and Mei,

1989; Huang et al., 1994; Liang et al., 1998; Morris and Davidson, 2003; Gerbeau

Fig. 5.19 Interface of melt

  Naijun Zhou

et al., 2003; Sun et al., 2004), the velocity field of various of prebaked anode cells

were computed and analyzed. Computational results of a 280kA cell in China

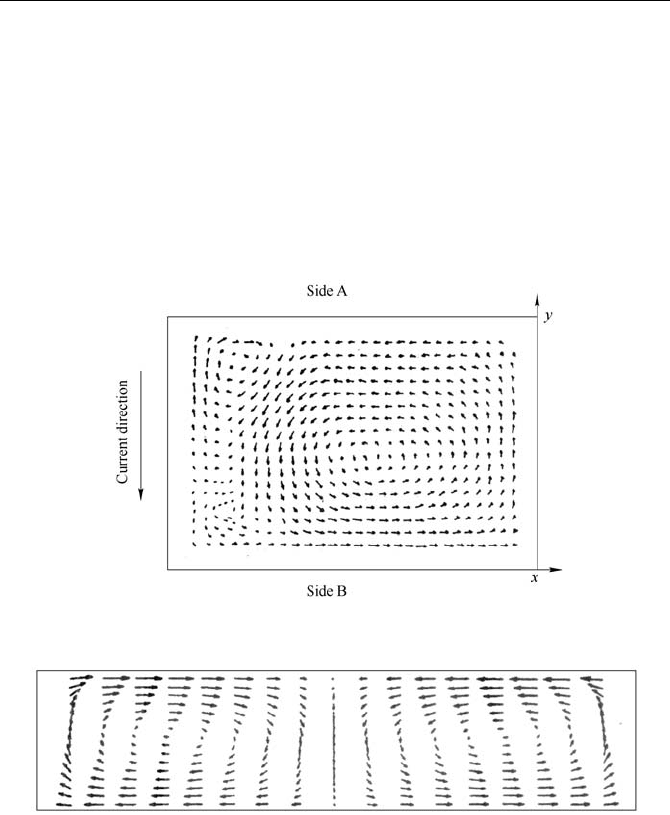
cited from reference (Huang et al., 1994) are shown in Fig. 5.20 and Fig 5.21. Cai

Qifeng et al. (Cai et al., 1993) had ever measured the velocity of the molten

aluminum in the industrial aluminum reduction cells using iron bar dissolution

method for many times.

It should be noted that the computation of the velocity field is so hard to be

highly accurate, because of the inaccurate analysis of the electromagnetic force

field computation as well as the complicated movement of fluid in the cells.

Fig. 5.20



Horizontal velocity distribution in melted aluminum for a 160kA cell (a half cell)

(Sun and Mei, 1989)

Fig. 5.21



Horizontal velocity distribution in electrolyte for a 280kA cell (Huang et al., 1994)

5.4



Analysis of Thermal Field in Aluminum Reduction Cells

The aim of analysis of the static thermal field is to solve the voltage distribution

and the temperature distribution inside the cell as well as the heat balance of the

cell under certain structure and working current conditions, thus to optimize the

design and structure of reduction cells. For such kind of analysis, steady-state

conduction and thermal conductivity models are used, with given melt

5 Hologram Simulation of Aluminum Reduction Cells 

temperature, melt position and shape of the ledge. Hence it is a “static”

problem.

The original analysis of thermal field was made separately for three parts, i.e.,

the anode, the cathode and the bottom of slot, which may be limited by computer

capacity and speed at that time. Nowadays, there is no need for such a

separation.

Because of the strong coupling between the electric field and the temperature

field in reduction cells, the temperature field cannot be analyzed independently.

Instead, it has to be solved simultaneously with the electric field, as introduced in

Section 5.2. In fact, in Section 5.2.2, details of analysis of the thermal field of the

anode have been described, and here will not be repeated any more. In this section,

it is to treat the aluminum electrolysis cell as a whole to discuss models and

solutions of the analysis of thermal field.

5.4.1



Control equations and boundary conditions

Under the assumption of steady state, equations of electrical and thermal

conduction in anode and cathode are the same as Eq. 5.3 and Eq. 5.4. What is

different is that, at non-conductive part (i.e. the cell body outside ledge and below

the cathode), the equation of thermal conduction can be transformed into the

Laplace equation without solving the electrical conduction equation.

∇∇=• (5.45)

Since the anode is symmetrical, it generally takes only half of the anode for a

prebaked cell(or even a quarter) as the computational geometry; but for a

self-baking cell, it is an area including two halves of conducting bar. It is assumed

that no transverse current and heat flow through the cross section. Obviously,

computation of thermal field for the anode must be treated as a three-dimensional

problem. Those of the cathode and other parts of the cell is actually a mix of two

and three dimensions, of which the cathode bar is to be treated as three-dimension,

while others can be computed with two-dimensional models. Temperature field of

the electrolyte and the molten aluminum are both homogeneous and their

temperature are specified. It must be noted that the computational slices should be

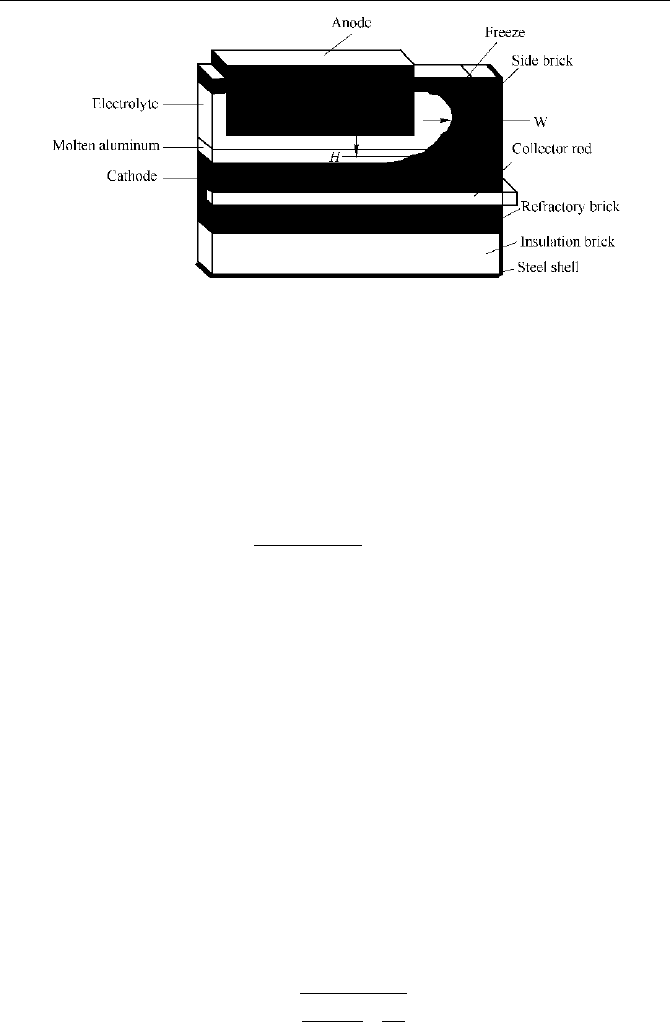
taken from the big and end faces of the cell. A schematic of the computational

slice is given in Fig. 5.22.

The boundary conditions for the electrical conduction equation are:

a) Regarding the cathode block surface as basic potential surface, and the

potentials in the molten aluminum are considered as equal.

b) The ledge is not conductive and all currents pass through the cathode block.

c) The currents passing through each anode and cathode rod are known and the

value is an average.

  Naijun Zhou

Fig. 5.22



Schematic of the computational slice of an aluminum reduction cell

The boundary conditions of the thermal conduction equation are:

a) The electrolyte and molten aluminum layers are an isothermal region, their

temperatures are to be decided with experience and by request.

b) The top of the cell is assumed to be adiabatic.

c) The environmental temperature around the cell is certain.

d) The coefficient of heat transfer between the external surface and the environment

is:

0.25 4 4 2

wa wa

5.6 10

( ) ( ) (W/(m K))CT T T T

−

=− + −

−

•

(5.46)

where C is an experimental coefficient, which is 2.6 for the side walls, 2.0 for the

bottom, and 3.3 for the top plate;

is the radiance of the external wall, of

which the value is usually taken as 0.82.

e) Factors that influence the heat transfer between the melt and the internal

faces are quite complicated, and no consist and reliable results can be referred (see

Table 5.2). Generally, the coefficient can be calculated with following empirical

formula (Mei and Tang, 1986; Mei et al., 1992; Zhou and Mei, 1992; Mei et al.,

1996).

Heat transfer from the melt to the ledge:

0.8 0.33

0.0365Nu Re Pr= • (5.47)

Heat transfer from the molten aluminum to the cathode block:

0.8

50.025( )Nu Re Pr=+ • (5.48)

Big error may be found in Eq. 5.48. So in practice, it is usually corrected with

additional thin film thermal-resistance method:

λλ

′

•

(5.49)

where

L is the inter width of the cell, m; Nu is the Nusselt number to be

determined with Eq. 5.47 and Eq. 5.48;

and

are respectively the

5 Hologram Simulation of Aluminum Reduction Cells 

thermal conductivity of the melt and the boundary thinfilm, W/(m·K); and

is the thickness of the boundary thinfilm, m. The melt velocity used above

equations are calculated with Eq. 5.36 and Eq. 5.37. Besides, the thermal

properties of main materials used in the computation are given in Table 5.3, or

refer to reference (Qiu, 1982).

Table 5.2 Coefficient of heat transfer between melts and ledge (W/(m

gK))

Author Electrolyte-ledge Aluminum-ledge Remark

[Japan]Haruhiko

Ikenouchi et al.

200

20~150

400

Effective heat transfer

coefficient during side

feeding (Ikenouchi et al.,



1978);

measured

value(Ikenouchi et al.,



1978)

[USA]W.E.Haupin 370

1500

1100

Calculated with criterion

equation (Haupin,1971);

measured value

(Haupin, 1971)

[Japan]Arai et al. 232

793 (

=0.3mm)

334 (

=1.0mm)

183 (

=2.0mm)

Arai and Ramazaki, 1975

[USA]J.G.Peacey 175~210 300~384 Peacey and Medlin, 1979)

[Norway]Perutne 200 Perutne, 1982

[USSR]

U.D.Dekopov et al.

14000

W/m

10500

W/m

Dekopov, 1978

[USSR]

B.P.Romanov et al.

141~184 150~268 Romanov, 1980

[Norway]A.Solhiem

For prebaked cells:

250~500

For self-baking cells:

300~650

Solhiem et al., 1983

  Naijun Zhou

Table 5.3 Thermal properties of materials

Material

Conductance

g(mgK)

−1

Density

/kggm

−3

Specific heat

capacity

gkg

−1

Semi-graphited cathode

carbon block (Aparotsev,

1998)

8.81

−0.072T

−3

1573/(1+7.9

h10

−6

307/(1+4.3

−4

Cathode iron

rod (Aparotsev, 1998)

58.85

−0.0414 T

−3

7830/(1+11.1

h10

−6

465/(1+12.1

−4

Cast iron (filled in iron rod

and carbon block)

(Aparotsev, 1998)

51.25

−0.064 T

−3

7270/(1+12.1

h10

−6

419/(1+5.7

−4

Side ledge

(CSIOFMAM, 1977)

2.16

−0.365h10

−2

+0.0558

h10

−4

Bottom ledge

(CSIOFMAM, 1977)

4.97

0.869h10

−2

+0.098

h10

−4

Insulation impervious

material

0.319+1.37

h10

−4

5.4.2



Calculation methods

In computation of the thermal field, finite difference method and Gaussian

elimination method (or other methods) are usually used to solve the linear

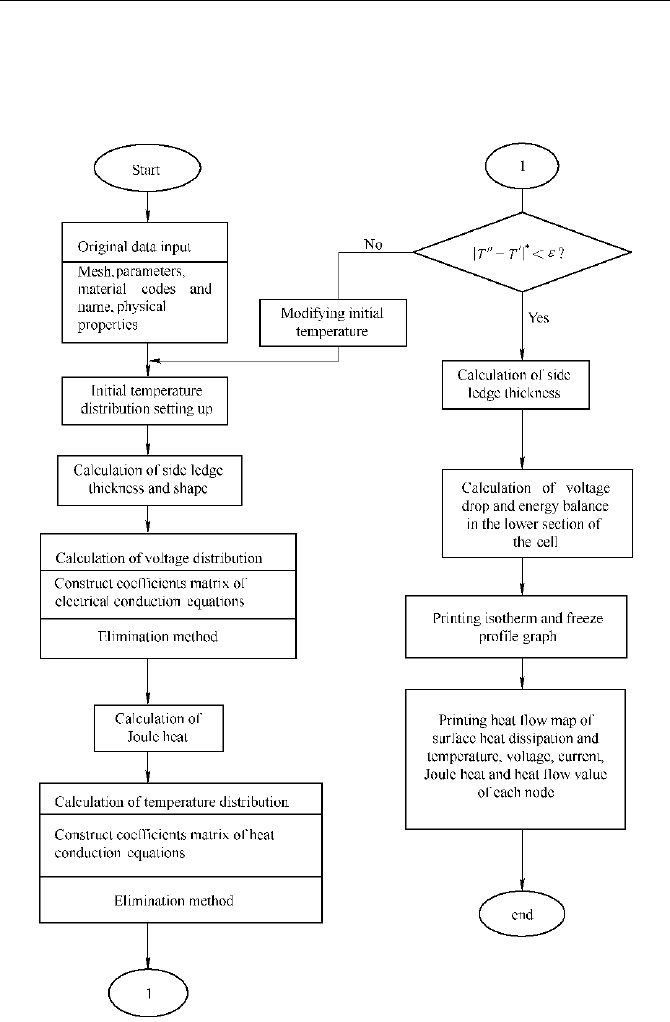
equations. The program chart of the computation is shown in Fig. 5.23. This

algorithm was used in references (Mei and Tang,1986;Mei et al., 1992; Zhou and

Mei, 1992; Mei et al., 1996; Mei et al., 1997; Mei et al; 1998; Zuo, 1996; You,

1997; You et al., 1998; Zhou et al., 1998) to analyze the thermal fields of various

types of reduction cells, which provided examples of systematic research on the

design of thermal fields and optimization of cell structures. A typical analytical

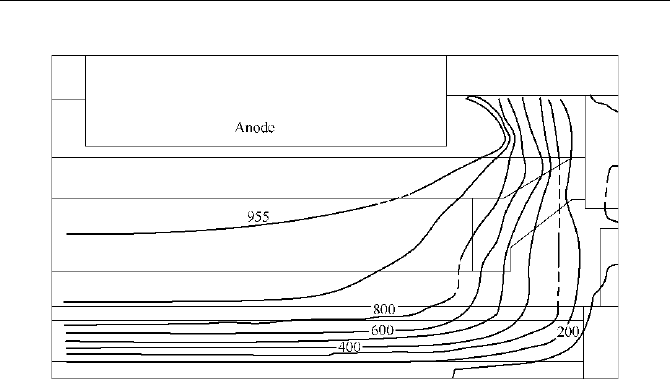
result is shown in Fig. 5.24.

5 Hologram Simulation of Aluminum Reduction Cells 

Fig. 5.23



Program chart of static analysis of the thermal field (Mei and Tang., 1986)

  Naijun Zhou

Fig. 5.24



Analytical result of the temperature field in cathode (Mei et al., 1996)

5.5



Dynamic Simulation for Aluminum Reduction Cells

An aluminum electrolysis cell is a complex high temperature electrochemical

reactor, whose operation conditions are effected by many factors. Any changes of

the factors will cause unstable state of the cells. A worse operation condition will

even result in an increasing of power consumption, decreasing of production, and

shorter cell life. Obviously, it’s difficult to accurately predict the effects of various

technical parameters on the operation conditions with the static models discussed

in previous sections. Hence, the dynamic simulation is needed and will be

introduced in this section.

The aim of the dynamic simulation is to simulate the process in the cell with

realtime information obtained by computer and output (forecast) the results, in

order for online monitoring the operation conditions and to instruct the operations.

Equipped with automatic control system, it can then realize functions of online

optimization and monitoring.

Practices indicate that there are three critical factors for operations of

aluminum reduction cells, that is, the electrolyte temperature, the cell freeze

profile and the current efficiency. Since effective online measurements of these

factors haven’t been found so far, it is hence of particular practical significance

to simulate the parameters. At present, the primary task of the dynamic

simulation of aluminum reduction cells is the dynamic forecasting of the

electrolyte temperature and the current efficiency, as well as the online

simulation of the cell freeze profile. The Central South University and some

5 Hologram Simulation of Aluminum Reduction Cells 

other institutes had carried out research in this fields for many years, and made

great progresses (Mei et al., 1997; Mei et al., 1998; Zuo, 1996; You, 1997; You

et al., 1998; Zhou et al., 1998). In this section, it is to be discussed the methods

for online simulation of the electrolyte temperature and the dynamic forecasting

of the cell freeze profile.

5.5.1



Factors influencing operation conditions and principle of

the dynamic simulation

You Wang (You, 1997; You et al., 1998)

had studied factors influencing the

operation conditions of the aluminum reduction cells. In his opinion, the factors

can be put into two categories. One is the static factors, including bus bar

configuration, cell structure, electro-thermal properties of materials, melt

properties, and heat transfer conditions of the cell body etc. The other is the

dynamic (transient) factors, including processing parameters such as series current,

cell voltage, anode-cathode distance (ACD), molecular ratio, temperature of the

electrolyte and molten aluminum, height of the as well as the electrolyte and

molten aluminum, anode effect coefficient etc., as well as the routine operations

such as alumina feeding, aluminum discharging, ACD adjusting, anode changing,

edge treating, effect treating and so on. The static factors only influence medium

and long term behavior of the cell, thus they can be used to establish the basic

energy balance of the cell. The dynamic factors influence dynamic behaviors and

they are the basis of dynamic simulation. All these factors must be quantitatively

transformed into energy budget of the cell or corresponding disturbance

parameters.

The principle of the dynamic simulation is based on following facts. When a

disturbance factor causes the change of energy inputting into the cell, it will firstly

cause changes of the melt temperatures (especially, the change of the electrolyte

temperature). As the liquidus temperature T

is fixed corresponding to certain

composition of the electrolyte, the change of the melt temperatures will result in

melting or freezing of the electrolyte crust, or in other word, changing in the

thickness of the side ledge. As a result, heat transfer through the ledge will also

change until a new balance of energy is reached. Therefore, based on the

contribution of every factor to the energy budget of the system, the energy balance

of a cell can be continuously obtained to calculate the bath temperature with

measured intensity and duration of the disturbance, thus to simulate the freeze

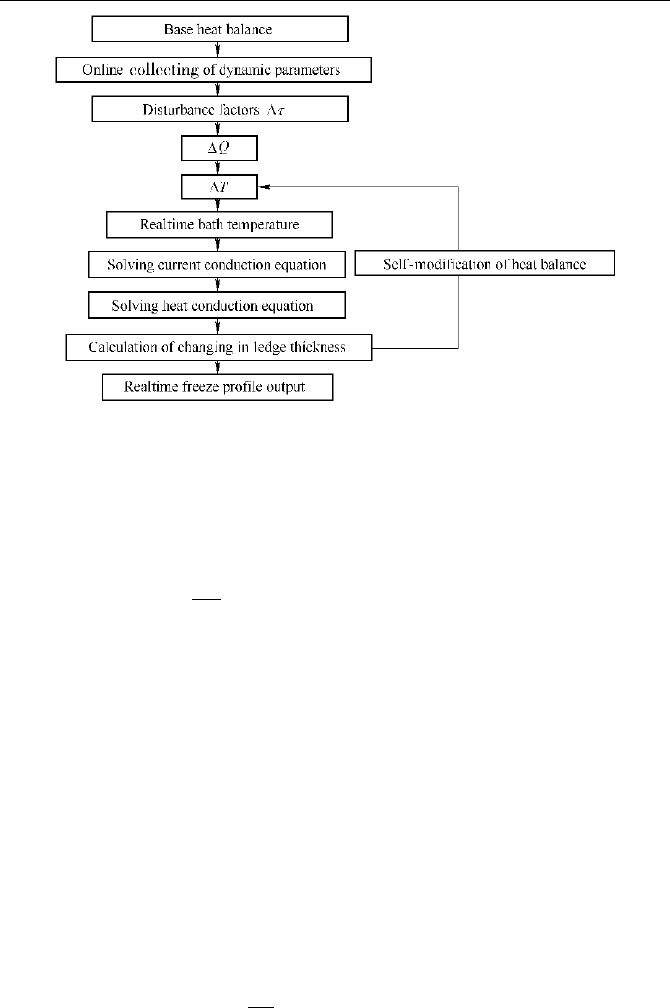
profile by solving the unsteady heat conduction equation. The program chart of

the process is given in Fig. 5.25.

  Naijun Zhou

Fig. 5.25



Program chart of the dynamic simulation for aluminum reduction cells

5.5.2



Models and algorithm

When viscous dissipation of the melt flow is neglected, energy equation of the

system is:

vol

div( ) div( grad )

HkTq

ρρ

∂

+= +

∂

•v (5.50)

The relation between the enthalpy and temperature is:

For solid phase

TT < :

HcT= •

For liquid phase

TT > :

HcT

=+•

At the interface

TT = :

HcTn

=+••

where

is the melting point of the electrolyte;

c is the specific heat capacity;

is the latent heat of melting, n is the molten fraction (0İnİ1).

As the melt motion in reduction cells is complicated. Eq. 5.50 is actually hard to

be solved. A simple method is: to replace the thermal conductivity

k with the

effective thermal conductivity

eff

k , so that the convection term can be eliminated:

teff

kkk += (5.51)

where k is the molecular thermal conductivity;

k is the turbulent thermal

conductivity.

Furthermore, assuming c

is a constant, Eq. 5.50 can then be simplified as:

eff vol

div( grad )

ckTq

∂

•• (5.52)

This is an unsteady conduction equation with internal heat sources, in which the

source term

vol

is the heat produced when current passing through the melt, that