Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook

Подождите немного. Документ загружается.

Caution: “Dry rock” is not the same as gas-saturated rock

The dry-frame modulus refers to the incremental bulk deformation resulting

from an increment of applied confining pressure with pore pressure held constant.

This corresponds to a “drained” experiment in which pore fluids can flow freely in

or out of the sample to ensure constant pore pressure. Alternatively, the dry-frame

modulus can correspond to an undrained experiment in which the pore fluid has

zero bulk modulus, and in which pore compressions therefore do not induce

changes in pore pressure. This is approximately the case for an air-filled sample

at standard temperature and pressure. However, at reservoir conditions, the gas

takes on a non-negligible bulk modulus and should be treated as a saturating fluid.

An equivalent expression for the dry rock compressibility or bulk modulus is

dry

¼ K

ð1  b Þ

where b is sometimes called the Biot coefficient, which describes the ratio of pore-

volume change Du

to total bulk-volume change DV under dry or drained conditions:

b ¼



V



dry

K

dry



Stress-induced pore pressure: Skempton’s coefficient

If this arbitrary pore space is filled with a pore fluid with bulk modulus, K

, the

saturated solid is stiffer under compression than the dry solid, because an increment

of pore-fluid pressure is induced that resists the volumetric strain. The ratio of the

induced pore pressure, dP, to the applied compressive stress, ds, is sometimes called

Skempton’s coefficient and can be written as

B 

d

1 þ K



1=K

 1=K

ðÞ

1 þ  1=K

 1=K

ðÞ1=K

dry

 1=K



1

where K

is the dry pore-space stiffness defined earlier in this section. For this

definition to be true, the pore pressure must be uniform throughout the pore space,

as will be the case if:

(1) there is only one pore,

(2) all pores are well connected and the frequency and viscosity are low enough for

any pressure differences to equilibrate, or

(3) all pores have the same dry pore stiffness.

57 2.9 Deformation of inclusions and cavities

Given these conditions, there is no additional limitation on pore geometry or

concentration. All of the necessary information concerning pore stiffness and geom-

etry is contained in the parameter K

Saturated stress-induced pore-volume change

The corresponding change in fluid-saturated pore volume, u

, caused by the remote

stress is



d

d



sat

d

1=K

1 þ K



1=K

 1=K

ðÞ

Low-frequency saturated compressibility

The low-frequency saturated bulk modulus, K

sat

, can be derived from Gassmann’s

equation (see Section 6.3 on Gassmann). One equivalent form is

sat



K





þ K

where, again, all of the necessary information concerning pore stiffness and geometry

is contained in the dry pore stiffness K

, and we must ensure that the stress-induced

pore pressure is uniform throughout the pore space.

Three-dimensional ellipsoidal cavities

Many effective media models are based on ellipsoidal inclusions or cavities. These

are mathematically convenient shapes and allow quantitative estimates of, for

example, K

, which was defined earlier in this section. Eshelby (1957) discovered

that the strain, e

, inside an ellipsoidal inclusion is homogeneous when a homoge-

neous strain, e

, (or stress) is applied at infinity. Because the inclusion strain is

homogeneous, operations such as determining the inclusion stress or integrating to

obtain the displacement field are straightforward.

It is very important to remember that the following results assume a single isolated

cavity in an infinite medium. Therefore, substituting them directly into the preceding

formulas for dry and saturated moduli gives estimates that are strictly valid only for

low concentrations of pores (see also Section 4.8 on self-consistent theories).

Spherical cavity

For a single spherical cavity with volume 

and a hydrostatic stress, ds,

applied at infinity, the radial strain of the cavity is

58 Elasticity and Hooke’s law

ð1  vÞ

2ð1  2vÞ

d

where v and K

are the Poisson ratio and bulk modulus of the solid material,

respectively. The change of pore volume is

d

3ð1  vÞ

2ð1  2vÞ



d

Then, the volumetric strain of the sphere is

d



3ð1  vÞ

2ð1  2vÞ

d

and the single-pore stiffness is





d

d

3ð1  vÞ

2ð1  2v Þ

Remember that this estimate of K

assumes a single isolated spherical cavity in an

infinite medium.

Under a remotely applied homogeneous shear stress, t

, corresponding to remote

shear strain e

¼ t

/2m

, the effective shear strain in the spherical cavity is

e ¼

15ð1 vÞ

ð7  5vÞ

where m

is the shear modulus of the solid. Note that this results in approximately

twice the strain that would occur without the cavity.

Penny-shaped crack: oblate spheroid

Consider a dry penny-shaped ellipsoidal cavity with semiaxes a  b ¼ c. When a

remote uniform tensional stress, ds, is applied normal to the plane of the crack, each

crack face undergoes an outward displacement, U, normal to the plane of the crack,

given by the radially symmetric distribution

UðrÞ¼

4ð1  v

Þc

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1  r=cðÞ

3pK

ð1  2vÞ

d

where r is the radial distance from the axis of the crack. For any arbitrary homoge-

neous remote stress, ds is the component of stress normal to the plane of the crack.

Thus, ds can also be thought of as a remote hydrostatic stress field.

This displacement function is also an ellipsoid with semiminor axis, U(r ¼ 0),

and semimajor axis, c. Therefore the volume change, du, which is simply the

59 2.9 Deformation of inclusions and cavities

integral of U(r) over the faces of the crack, is just the volume of the displacement

ellipsoid:

d

pUð0Þc

16c

ð1  v

ð1  2vÞ

d

Then the volumetric strain of the cavity is

d



4ðc=aÞ

3pK

ð1  v

ð1  2vÞ

d

and the pore stiffness is





d

d

4ðc=aÞ

3pK

ð1  v

ð1  2vÞ

An interesting case is an applied compressive stress causing a displacement, U(0),

equal to the original half-width of the crack, thus closing the crack. Setting U(0) ¼ a

allows one to compute the closing stress:



3pð1  2vÞ

4ð1  v

2ð1  vÞ

where a ¼ (a/c) is the aspect ratio.

Now, under a remotely applied homogeneous shear stress, t

, corresponding to

remote shear strain e

¼ t

/2m

, the effective shear strain in the cavity is

e ¼ t

2ðc=aÞ

ð1  vÞ

ð2  vÞ

Needle-shaped pore: prolate spheroid

Consider a dry needle-shaped ellipsoidal cavity with semiaxes a  b ¼ c and with

pore volume  ¼

pac

. When a remote hydrostatic stress, ds, is applied, the pore

volume change is

d

ð5  4vÞ

ð1  2vÞ



d

The volumetric strain of the cavity is then

d



ð5  4vÞ

ð1  2vÞ

d

and the pore stiffness is





d

d

ð5  4vÞ

ð1  2vÞ

60 Elasticity and Hooke’s law

Note that in the limit of very large a/c, these results are exactly the same as for a

two-dimensional circular cylinder.

Estimate the increment of pore pressure induced in a water-saturated rock when a

1 bar increment of hydrostatic confining pressure is applied. Assume that the

rock consists of stiff, spherical pores in a quartz matrix. Compare this with a rock

with thin, penny-shaped cracks (aspect ratio a ¼ 0.001) in a quartz matrix. The

moduli of the individual constituents are K

quartz

¼ 36 GPa, K

water

¼ 2.2 GPa, and

quartz

¼ 0.07.

The pore-space stiffnesses are given by

-sphere

¼ K

quartz

2ð1  2v

quartz

3ð1  v

quartz

¼ 22:2 GPa

-crack

3paK

quartz

ð1  2v

quartz

ð1  v

quartz

¼ 0:0733 GPa

The pore-pressure increment is computed from Skempton’s coefficient:

P

pore

P

confining

¼ B ¼

1 þ K



ðK

1

water

 K

1

quartz

sphere

1 þ 22:2½ð1=2:2Þð1=36Þ

¼ 0:095

crack

1 þ 0:0733½ð 1=2:2Þð1=36Þ

¼ 0:970

Therefore, the pore pressure induced in the spherical pores is 0.095 bar and the

pore pressure induced in the cracks is 0.98 bar.

Two-dimensional tubes

A special two-dimensional case of long, tubular pores was treated by Mavko (1980)

to describe melt or fluids arranged along the edges of grains. The cross-sectional

shape is described by the equations

x ¼ R cos y þ

2 þ g

cos 2y



y ¼ R sin y þ

2 þ g

sin 2y



where g is a parameter describing the roundness (Figure 2.9.1).

61 2.9 Deformation of inclusions and cavities

Consider, in particular, the case on the left, g ¼ 0. The pore volume is

paR

where a  R is the length of the tube. When a remote hydrostatic stress, ds,is

applied, the pore volume change is

d

ð13  4v  8v

ð1  2vÞ



d

The volumetric strain of the cavity is then

d



ð13  4v  8v

ð1  2vÞ

d

and the pore stiffness is





d

d

ð13  4v  8v

ð1  2vÞ

In the extreme, g !1, the shape becomes a circular cylinder, and the expression for

pore stiffness, K

, is exactly the same as that derived for the needle-shaped pores

above. Note that the triangular cavity (g ¼ 0) has about half the pore stiffness of the

circular one; that is, the triangular tube can give approximately the same effective

modulus as the circular tube with about half the porosity.

Caution

These expressions for K

, du

, and e

include an estimate of tube shortening as

well as a reduction in pore cross-sectional area under hydrostatic stress. Hence, the

deformation is neither plane stress nor plane strain.

Plane strain

The plane-strain compressibility in terms of the reduction in cross-sectional area A is

given by



d

6ð1vÞ

; g ! 0

2ð1vÞ

; g !1

(

g =0 g =1 g = ∞

Figure 2.9.1 The cross-sectional shapes of various two-dimensional tubes.

62 Elasticity and Hooke’s law

The latter case (g !1) corresponds to a tube with a circular cross-section and agrees

(as it should) with the expression given below for the limiting case of a tube with an

elliptical cross-section with aspect ratio unity.

A general method of determining K



for nearly arbitrarily shaped two-dimensional

cavities under plane-strain deformation was developed by Zimmerman (1986, 1991a)

and involves conformal mapping of the tube shape into circular pores. For example,

pores with cross-sectional shapes that are n-sided hypotrochoids given by

x ¼ cosðyÞþ

ðn  1Þ

cosðn  1Þy

y ¼sinðyÞþ

ðn  1Þ

sinðn  1Þy

(see the examples labeled (1) in Table 2.9.1) have plane-strain compressibilities



d

0cir



1 þðn  1Þ

1

1 ðn  1Þ

1

where 1=K

0cir



is the plane-strain compressibility of a circular tube given by

0cir



2ð1  nÞ

Table 2.9.1 summarizes a few plane-strain pore compressibilities.

Two-dimensional thin cracks

A convenient description of very thin two-dimensional cracks is in terms of elastic

line dislocations. Consider a crack lying along –c < x < c in the y ¼ 0 plane and very

long in the z direction. The total relative displacement of the crack faces u(x),

defined as the displacement of the negative face (y ¼ 0

–

) relative to the positive face

(y ¼ 0

), is related to the dislocation density function by

BðxÞ¼

where B(x) dx represents the total length of the Burger vectors of the dislocations

lying between x and x þ dx. The stress change in the plane of the crack that

results from introduction of a dislocation line with unit Burger vector at the origin is

 ¼

2pDx

where D ¼ 1 for screw dislocations and D ¼ (1 – v) for edge dislocations (v is

Poisson’s ratio and m

is the shear modulus). Edge dislocations can be used to describe

mode I and mode II cracks; screw dislocations can be used to describe mode III cracks.

63 2.9 Deformation of inclusions and cavities

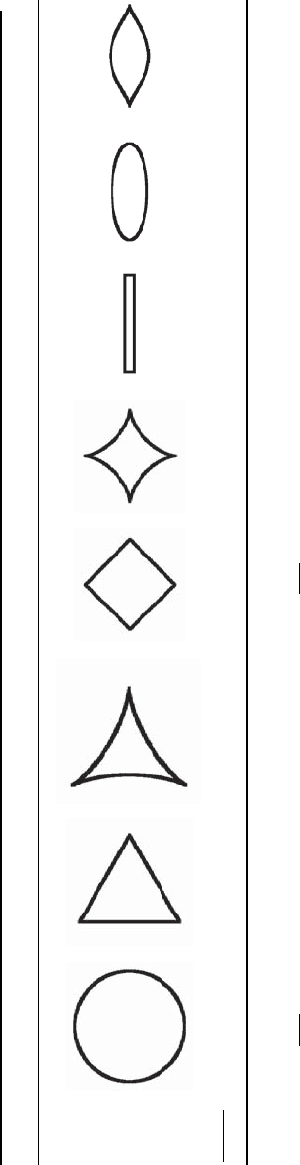
Table 2.9.1 Plane-strain compressibility normalized by the compressibility of a circular tube.

(1) (1) (1) (2) (3)

2ð1nÞK



1 1.581 3 1.188 2 p/8a 1/2a 2/3a

Notes:

(1)

x ¼ cosðyÞþ

ðn1Þ

cosðn  1Þy, where n ¼ number of sides; y ¼sinðyÞþ

ðn1Þ

sinðn  1Þ y.

(2)

y ¼ 2b

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 ðx=cÞ

ðellipseÞ.

(3)

y ¼ 2b 1 ðx=cÞ

3=2

(nonelliptical, “tapered” crack).

The stress here is the component of traction in the crack plane parallel to the

displacement: normal stress for mode I deformation, in-plane shear for mode II

deformation, and out-of-plane shear for mode III deformation.

Then the stress resulting from the distribution B(x) is given by the convolution

ðxÞ¼

2pD

c

Bðx

Þdx

x  x

The special case of interest for nonfrictional cavities is the deformation for stress-

free crack faces under a remote uniform tensional stress, ds, acting normal to the

plane of the crack. The outward displacement distribution of each crack face is given

by (using edge dislocations)

UðxÞ¼

cð1  vÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 ðx=cÞ

2cð1  v

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 ðx=cÞ

ð1  2vÞ

The volume change is then given by

d

d

¼ pUð0Þca ¼

2pc

ð1  v

ð1  2vÞ

It is important to note that these results for displacement and volume change apply

to any two-dimensional crack of arbitrary cross-section as long as it is very thin and

approximately planar. They are not limited to cracks of elliptical cross-section.

For the special case in which the very thin crack is elliptical in cross-section with

half-width b in the thin direction, the volume is u ¼ pabc, and the pore stiffness under

plane-strain deformation is given by



d

ðc=bÞð1  vÞ

2ðc=bÞ

ð1  v

ð1  2vÞ

Another special case is a crack of nonelliptical form (Mavko and Nur, 1978) with

initial shape given by

ðxÞ¼2b 1 



3=2

where c

is the crack half-length and 2b is the maximum crack width. This crack is

plotted in Figure 2.9.2. Note that unlike elliptical cracks that have rounded or blunted

ends, this crack has tapered tips where faces make a smooth, tangent contact. If we

apply a pressure, P, the crack shortens as well as thins, and the pressure-dependent

length is given by

c ¼ c

1 

2ð1  vÞ

ðb=c



1=2

65 2.9 Deformation of inclusions and cavities

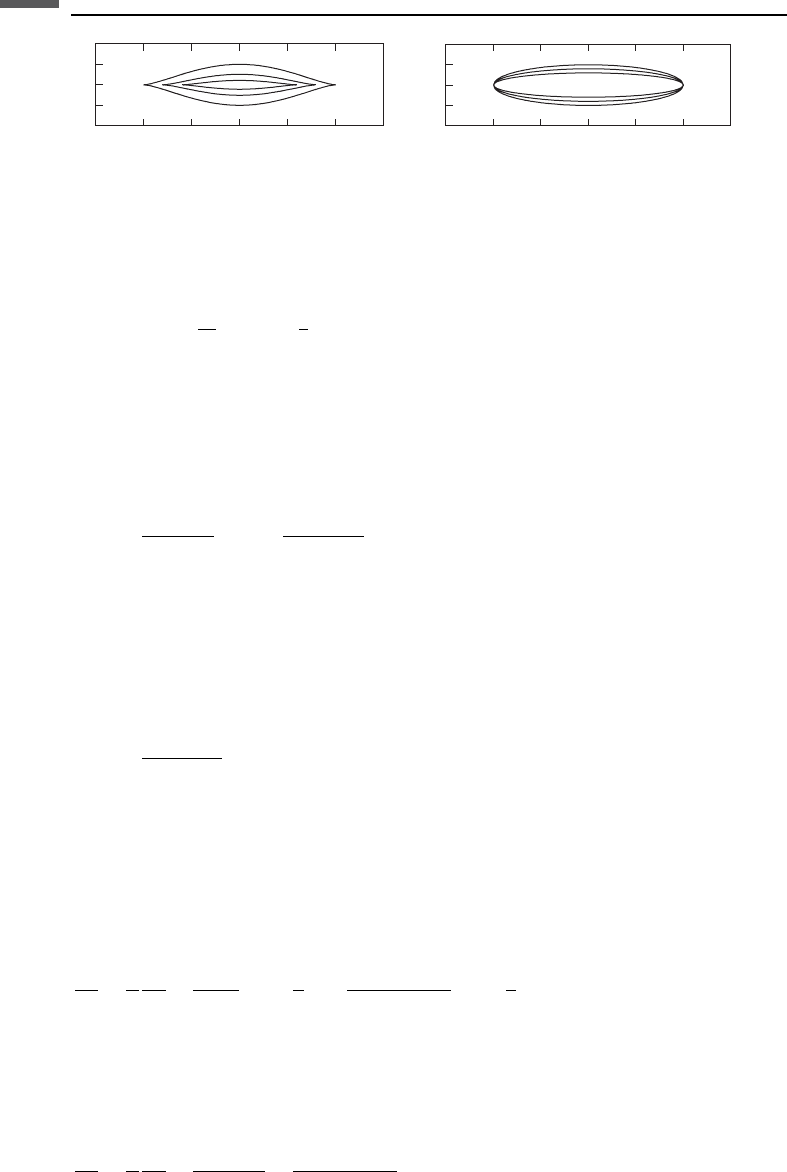
Then the deformed shape is

Uðx; PÞ¼2b



1 





3=2

; jxjc

An important consequence of the smoothly tapered crack tips and the gradual crack

shortening is that there is no stress singularity at the crack tips. In this case, crack

closure occurs (i.e., U ! 0) as the crack length goes to zero (c ! 0). The closing

stress is



2ð1  vÞ

4ð1  v

where a

¼ b/c

is the original crack aspect ratio, and m

and E

are the shear and

Young’s moduli of the solid material, respectively. This expression is consistent with

the usual rule of thumb that the crack-closing stress is numerically ~a

. The exact

factor depends on the details of the original crack shape. In comparison, the pressure

required to close a two-dimensional elliptical crack of aspect ratio a



2ð1  v

Ellipsoidal cracks of finite thickness

The pore compressibility under plane-strain deformation of a two-dimensional ellip-

tical cavity of arbitrary aspect ratio a is given by (Zimmerman, 1991a)



d

1  v

a þ



2ð1  v

ð1  2vÞ

a þ



where m

, K

, and v are the shear modulus, bulk modulus, and Poisson’s ratio of the

mineral material, respectively. Circular pores (tubes) correspond to aspect ratio a ¼ 1

and the pore compressibility is given as



d

2ð1  vÞ

4ð1  v

ð1  2vÞ

−b

−c

−b

−c

Figure 2.9.2 A nonelliptical crack shortens as well as narrows under compression.

An elliptical crack only narrows.

66 Elasticity and Hooke’s law