Marathe K. Topics in Physical Mathematics

Подождите немного. Документ загружается.

328 10 3-Manifold Invariants

γ :



i=1

× D

→ Y

such that the restrictions



i=1

×{x}→Y and γ

: S

× D

→ Y

are smooth embeddings for each x ∈ D

and for each i, 1 ≤ i ≤ m.Letˆγ

denote the family of holonomy maps

ˆγ

: A

→ SU(2) ×···×SU(2)



 

m times

,x∈ D

The holonomy is conjugated under the action of the group of gauge transfor-

mations and we continue to denote by ˆγ

the induced map on the quotient

= A

/G.LetF

denote the set of smooth functions

h : SU(2) ×···×SU(2)



 

m times

→ R

which are invariant under the adjoint action of SU(2). Floer’s set of pertur-

bations Π is deﬁned as

Π :=



m∈N

×F

Floer proves that for each (γ,h) ∈ Π the function

: B

→ R deﬁned by h

(α)=



h(ˆγ

(α))

is a smooth function and that for a dense subset P⊂RM(Y )×Π the critical

points of the perturbed function

(γ,h)

:= f

+ h

are non-degenerate. The corresponding moduli space decomposes into smooth

oriented manifolds of regular trajectories of the gradient ﬂow of the function

(γ,h)

with respect to a generic metric σ ∈RM(Y ). Furthermore, the ho-

mology groups of the perturbed chain complex are independent of the choice

of perturbation in P. We shall assume that a suitable perturbation has been

chosen. Let α, β be two critical points of the function f

. Considering the

spectral ﬂow (denoted by sf)fromα to β we obtain the moduli space M(α, β)

as the moduli space of self-dual connections on Y × R which are asymptotic

to α and β (as t →±∞). Let M

(α, β) denote the component of dimension j

in M(α, β). There is a natural action of R on M(α, β). Let

(α, β)denote

the component of dimension j −1inM(α, β)/R.Let#

(α, β)denotethe

10.5 Floer Homology 329

signed sum of the number of points in

(α, β). Floer deﬁnes the Morse

index of α by considering the spectral ﬂow from α to the trivial connection θ.

It can be shown that the spectral ﬂow and hence the Morse index are deﬁned

modulo 8.

Now deﬁne the chain groups by

(Y )=Z{α ∈R

∗

(Y ) | sf(α)=n},n∈ Z

and deﬁne the boundary operator ∂

∂ : R

(Y ) →R

n−1

(Y )

∂α =



β∈R

n−1

(Y )

(α, β)β. (10.35)

It can be shown that ∂

= 0 and hence (R(Y ),∂) is a complex. This complex

can be thought of as an inﬁnite-dimensional generalization [131] of Witten’s

instanton tunneling and we will call it the Floer–Witten Complex of the

pair (Y,SU(2)). Since the spectral ﬂow and hence the dimensions of the

components of M(α, β) are congruent modulo 8, this complex deﬁnes the

Floer homology groups FH

(Y ),j∈ Z

,wherej is the spectral ﬂow of α to

θ modulo 8. If r

denotes the rank of the Floer homology group FH

(Y ),j∈

, then we can deﬁne the corresponding Euler characteristic χ

(Y )by

(Y ):=



j∈Z

(−1)

Combining this with Taubes’ interpretation of the Casson invariant c(Y )we

get

c(Y )=χ

(Y )=



j∈Z

(−1)

. (10.36)

An important feature of Floer’s instanton homology is that it can be re-

garded as a functor from the category of homology 3-spheres to the category

of graded Abelian groups, with morphisms given by oriented cobordism. Let

M be a smooth oriented cobordism from Y

to Y

so that ∂M = Y

−Y

.By

a careful analysis of instantons on M, Floer showed [130]thatM induces a

graded homomorphism

: FH

) → FH

j+b(M)

),j∈ Z

, (10.37)

where

b(M)=3(b

(M) − b

(M)). (10.38)

Then the homomorphisms induced by cobordism have the following functorial

properties:

330 10 3-Manifold Invariants

(Y × R)

= id, (10.39)

(MN)

= M

j+b(N)

. (10.40)

An algorithm for computing the Floer homology groups for Seifert-ﬁbered

homology 3-spheres with three exceptional ﬁbers (or orbits) is discussed in

[127]. In the following example we present some of the results given in that

article.

Example 10.1 Let Y denote the Seifert-ﬁbered integral homology 3-sphere

with exceptional orbits of order a

.ThenY = Σ(a

) can be

represented as the Brieskorn homology 3-sphere

Σ(a

):={(z

) | z

+ z

=0}∩S

Floer homology is computed by using the moduli space of SO(3)-connections

over Y . In this case the representation space R(Y ) contains only regular

representations and hence is a ﬁnite set. The Chern–Simons function

: R(Y ) → R/4Z

is deﬁned by

α → (2e

)(mod4), (10.41)

where e

∈ Z is the Euler class of the representation α. The algorithm to

determine this number is one of the highlights of [127]. Moreover, it can be

shown that α is a critical point of index n where

n ≡



i=1

−1



k=1

cot



πak



cot



πk



sin



πe



(mod 8).

(10.42)

It can be shown that the index n is odd for all critical points and hence

the boundary operator ∂ deﬁned in (10.35) is zero. It follows that the Floer

homology groups FH

(Σ(a

)) are zero for odd i and are free for even

i.Ifwedenotebyr

(Y ) therankoftheith Floer homology group of the

manifold Y then the set of ranks {r

(Y ),r

(Y )} determines the

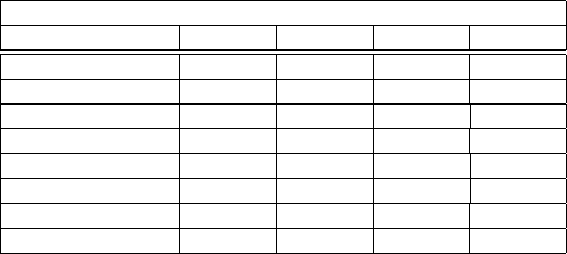
Floer homology of Y in this case. In Table 10.1 we give some computations

of these ranks (see [127] for further details).

An examination of Table 10.1 shows that Floer homology of Brieskorn

spheres is periodic of period 4, i.e.,

(Σ(a

)) = FH

i+4

(Σ(a

)), ∀i ∈ Z

It has been conjectured that this property of periodicity holds for Floer homol-

ogy groups of any homology 3-sphere.

The result discussed in the above example can be extended to compute

the Floer homology groups of any Seifert-ﬁbered integral homology 3-sphere.

10.5 Floer Homology 331

Table 10.1 Floer homology of Brieskorn spheres

Y = Σ(a

)

), (−1)

(Y ) r

(Y )

(2, 3, 6k ± 1), −1 (k ∓ 1)/2 (k ± 1)/2 (k ∓ 1)/2 (k ± 1)/2

(2, 3, 6k ± 1), 1 k/2 k/2 k/2 k/2

(2, 5, 10k ± 1), −1 (3k ∓ 1)/2 (3k ± 1)/2 (3k ∓ 1)/2 (3k ± 1)/2

(2, 5, 10k ± 1), 1 3k/2 3k/2 3k/2 3k/2

(2, 5, 10k ± 3), −1 (3k ± 1)/2 (3k ± 1)/2 (3k ± 1)/2 (3k ± 1)/2

(2, 5, 10k ± 3), 1 (3k ± 2)/2 3k/2 (3k ± 2)/2 3k/2

(2, 7, 14k ± 1), −1 3k ∓ 1 3k ± 1 3k ∓ 1 3k ± 1

(2, 7, 14k ± 1), 1 3k 3k 3k 3k

Further examples of the computation of Floer homology groups can be found

in [335].

Instanton Homology for Oriented 3-Manifolds

We now discuss brieﬂy some of the results of Fukaya (see [147]) on the instan-

ton homology for oriented 3-manifolds. Let Y be a closed connected oriented

3-manifold. We restrict ourselves to the special case of H

(Y ; Z) torsion-free.

Let γ

,...,γ

be a basis for H

(Y,Z), where k = b

(Y ) is the ﬁrst Betti

number of Y . Fukaya constructs a function using this basis to modify the

Chern–Simons function of the Floer theory. To deﬁne this function we begin

by observing that there is a natural gauge-invariant function associated to

alooponY which generalizes the Wilson loop functional well known in the

physics literature. We recall a deﬁnition of this functional from Chapter 7.

It is given in a more general form than we need. It is of independent interest

in the TQFT interpretation of knot and link invariants, as we will show in

Chapter 11.

We begin with a general deﬁnition of the Wilson loop functional that

was introduced as an example of a quantum observable in Chapter 7. This

functional is also used in the TQFT interpretation of the Jones polynomial

in Chapter 11.

Deﬁnition 10.1 (Wilson loop functional) Let ρ denote a representation of

G on V .Letα ∈ Ω(M, x

) denote a loop at x

∈ M. Let π : P (M,G) → M

be the canonical projection and let p ∈ π

−1

). If ω is a connection on P ,

then the parallel translation along α maps the ﬁber π

−1

) into itself. Let

ˆα

: π

−1

) → π

−1

) denote this map. Since G acts transitively on the

ﬁbers, ∃g

∈ G such that ˆα

(p)=pg

. Now deﬁne

332 10 3-Manifold Invariants

ρ,α

(ω):=Tr[ρ(g

)], ∀ω ∈A. (10.43)

We note that g

and hence ρ(g

) change by conjugation if, instead of p,we

choose another point in the ﬁber π

−1

), but the trace remains unchanged.

Alternatively, we can consider the vector bundle P ×

V associated to the

principal bundle P and parallel displacement of its ﬁbers induced by α.Letπ :

P ×

V → M be the canonical projection. We note that in this case π

−1

)

∼

V . Now the map ˆα

: π

−1

) → π

−1

) is a linear transformation and we

can deﬁne

ρ,α

(ω):=Tr[ˆα

], ∀ω ∈A. (10.44)

We call these W

ρ,α

the Wilson loop functionals associated to the represen-

tation ρ and the loop α. In the particular case when ρ =Ad, the adjoint

representation of G on g, our constructions reduce to those considered in

physical applications.

A gauge transformation f ∈Gacts on ω ∈Aby a vertical automorphism

of P and therefore changes the holonomy by conjugation by an element of the

gauge group G. This leaves the trace invariant and hence we have

ρ,f ·α

(ω)=W

ρ,α

(ω), ∀ω ∈Aand f ∈G. (10.45)

Equation (10.45) implies that the Wilson loop functional is gauge-invariant

and hence descends to the moduli space B = A/G of gauge potentials. We

shall continue to denote it by W.

In the application that we want to consider, we are interested in the special

case of gauge group G = SU(2) and ρ the deﬁning representation of SU(2).

It can be shown that if α, β are loops representing the same homology class

then

ρ,α

= W

ρ,β

. (10.46)

Thus, W can be regarded as deﬁned on the ﬁrst homology H

(Y,Z). Let α

be a loop representing the basis element γ

of the homology H

(Y,Z). The

loop α

: S

→ Y can be extended to an embedding α

: S

× D

→ Y .

Deﬁne the loop

i,x

: S

→ Y

i,x

(θ)=α

(θ, x),θ∈ S

Choose a non-negative function u : D

→ R with compact support such that



u(x)dx =1.

We deﬁne W

: D

×B

→ R by

(x, ω):=W

ρ,α

i,x

(ω), 1 ≤ i ≤ k. (10.47)

10.6 In t eger-Graded Instanton Homology 333

Now ﬁx an >0 and deﬁne f



: B

→ R by



(ω):=



i=1



(x, ω)u(x)dx. (10.48)

It can be shown that for suﬃciently small  the function f

−f



on B

has

ﬁnitely many non-degenerate critical points and that the Morse index of each

critical point is well-deﬁned modulo 8. We now proceed as in Floer theory by

considering the gradient ﬂow of the function f

− f



on B

deﬁned by

∂c(t)

∂t

= ∗

c(t)

− grad

c(t)



. (10.49)

The corresponding chain complex is denoted by (C

,∂) and the Fukaya ho-

mology groups are denoted by I

,n∈ Z

.WhenY is a homology 3-sphere

the Fukaya theory goes over into the Floer theory and hence we refer to these

collectively as the Fukaya–Floer homology groups. By tensoring the chains

of the complex with symmetric powers of H

(Y,Z) Fukaya deﬁnes new chain

complexes (C

,∂

) indexed by non-negative integer s<3. The main result

of [147] is the following theorem.

Theorem 10.1 Let I

, n ∈ Z

, denote the homology groups of the complex

,∂

) indexed by non-negative integer s<3. Then the groups I

do not

depend on the choice of metrics, the bases γ

for the homology H

(Y,Z),and

the other choices made in perturbing the function f

− f



on B

,andthey

are topological invariants of the manifold Y .

The signiﬁcance of these new invariants and their relation to other known

invariants is not yet clear.

10.6 Integer-Graded Instanton Homology

In Section 10.4 we discussed the Z

-graded homology theory due to Floer and

its extension to arbitrary closed oriented 3-manifolds by Fukaya. Fintushel

and Stern [128] have extended Floer homology theory in another direction

by deﬁning a Z-graded homology theory for homology 3-spheres. We now

discuss this extension.

To extend FH to a Z-graded homology we use the universal cover (inﬁnite

cyclic)

of B

deﬁned by

= A/G

. (10.50)

The Chern–Simons action functional and the spectral ﬂow (denoted by sf)

on A descend to

→ R.Let

∗

(Y )bethecoverofR

∗

(Y )andlet

334 10 3-Manifold Invariants

= R \ c(

∗

(Y )) (10.51)

be the set of regular values of the Chern–Simons functional. We note that the

set c(

∗

(Y )) is ﬁnite modulo Z.Fixμ ∈ R

and let α

(μ)

∈

R(Y ) ⊂

the unique lift of α ∈R(Y ) (see section 10.4) such that c(α

(μ)

) ∈ (μ, μ +1).

Deﬁne

(μ)

(Y ):=Z{α ∈R

∗

(Y ) | sf (α

(μ)

)=n} (10.52)

and deﬁne the boundary operator ∂

(μ)

∂

(μ)

: R

(μ)

(Y ) →R

(μ)

n−1

(Y )

∂

(μ)

α =



β∈R

(μ)

n−1

(Y )

(α, β))β, (10.53)

where

(α, β) is deﬁned by a construction similar to that in the Floer

theory. It can be shown that ∂

(μ)

∂

(μ)

= 0 and hence (R

(μ)

(Y ),∂

(μ)

)isa

complex. The resulting homology groups are denoted by I

(μ)

,n∈ Z,andare

called the integer-graded instanton homology groups. In general, not

all the representations in R(Y ) are regular. However, as in the Floer theory,

one can deﬁne the integer-graded instanton homology groups by perturbing

the Chern–Simons function. The resulting homology groups are independent

of the perturbations and satisfy the following properties:

1. For μ ∈ [μ

,μ

] ⊂ R

(μ

)

= I

(μ)

= I

(μ

)

, ∀n ∈ Z,

2. For μ ∈ R

(μ)

= I

(μ+1)

, ∀n ∈ Z.

The homology groups I

(μ)

, n ∈ Z, determine the Floer homology groups by

ﬁltering the Floer chain complex. If Φ

(μ)

s,n

(Y ) denotes the ﬁltration induced

on the Floer homology then we have the following theorem.

Theorem 10.2 Let n ∈ Z

, s ∈ Z,ands ≡ n (mod 8). Then there exists

aspectralsequence(E

s,n

(Y ),d

) such that

s,n

(Y )

∼

(μ)

, (10.54)

∞

s,n

(Y )

∼

(μ)

s,n

(Y )/Φ

(μ)

s+8,n

(Y ). (10.55)

The groups E

s,n

(Y ) of the spectral sequence are topological invariants of Y .

The groups E

s,n

(Y ) as well as the instanton homology groups I

(μ)

, n ∈

Z, can be regarded as functors from the category of homology 3-spheres to

the category of graded Abelian groups with morphisms given by oriented

cobordism.

10.6 In t eger-Graded Instanton Homology 335

Theorem 10.3 If M is an oriented cobordism from Y

to Y

so that ∂M =

− Y

then for μ ∈ R

∩ R

, M induces graded homomorphisms

(μ)

: E

s,n

) → E

s+b(M),n+b(M)

where b(M )=3(b

(M) −b

(M)). Moreover, we have the following relations:

(Y × R)

= id, (10.56)

(MN)

= M

s+b(N)

. (10.57)

Proofs of the above two theorems are given in [128].

In Example 10.1, we presented some results on the Floer homology groups

(Σ(a

)), i ∈ Z

, where Σ(a

) is the Seifert-ﬁbered homol-

ogy 3-sphere with exceptional orbits of order a

. An algorithm for com-

puting these Floer homology groups discussed in [127]isextendedin[128]to

compute the integer-graded instanton homology groups I

(μ)

(Σ(a

)),

i ∈ Z. In the following example we present some of the results given in that

article.

Example 10.2 As in Example 10.1, we let Y denote the Seifert-ﬁbered inte-

gral homology 3-sphere with exceptional orbits of order a

. Integer-

graded instanton homology is computed via the moduli space of SO(3)-

connections over Y . The representation space R(Y ) contains only regular

representations. Let e

denote the Euler class of the representation α.Let

(μ)

∈ (μ, 4+μ) be deﬁned by the congruence

(μ)

≡ (2e

(mod 4).

Then α is a critical point of the lift of the Chern–Simons functional

(μ)

: R

(μ)

(Y ) → R/4Z deﬁned by α → e

(μ)

. (10.58)

The index l

(μ)

(α) of α is given by

(μ)

(α)=

2(e

(μ)

)



i=1

−1



k=1

cot



πak



cot



πk



sin



πe

(μ)



It can be shown that the index l

(μ)

(α) is always an odd integer. It follows

that the boundary operator ∂

(μ)

is identically zero in this case and hence the

integer-graded instanton homology is given by

(μ)

(Σ(a

))

∼



(μ)

(Σ(a

)), for odd n

0, for even n.

It can be shown that I

(μ)

(Σ(a

)) is free of ﬁnite rank for all n for which

it is non-zero. For example, we can obtain the ranks of the homology groups

336 10 3-Manifold Invariants

(0)

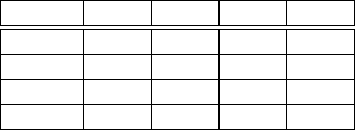
(Σ(2, 3, 6k ±1)) after some explicit computations. Using this information

we can write down the Poincar´e–Laurent polynomials p(Σ(2, 3, 6k ±1))(t) of

Σ(2, 3, 6k ± 1). A list of these polynomials for the values of k from 1 to 9 is

given in Table 10.2. We have corrected some typographical errors in the list

given in [128].

Table 10.2 Poincar´e–Laurent polynomials of Σ(2, 3, 6k ± 1)

k p(Σ(2, 3, 6k − 1))(t) p(Σ(2, 3, 6k + 1))(t)

1 t + t

−1

+ t

2 t + t

+ t

−1

+ t + t

+ t

3 t + t

+2t

+ t

−1

+ t +2t

+ t

4 t +2t

+2t

+ t

−1

+2t +2t

+2t

5 t + t

+3t

+2t

+ t

−1

+2t +3t

+2t

+ t

6 t +2t

+3t

+2t

+ t

−1

+3t +3t

+3t

+2t

7 t + t

+4t

+3t

+2t

−1

+3t +4t

+3t

+2t

8 t +2t

+3t

+4t

+3t

+2t

+ t

−1

+4t +4t

+4t

+3t

9 t + t

+4t

+3t

+ t

−1

+3t +5t

+4t

+3t

+ t

At the end of Example 10.1 we saw that the conjecture that the Floer

homology is periodic with period 4 is veriﬁed for the Brieskorn spheres. An

examination of Table 10.2 shows that the integer-graded instanton homology

groups of the Brieskorn spheres do not have this periodicity property. The

integer-graded instanton homology groups can be thought of as a reﬁnement

of the Z

-graded Floer homology groups just as the Floer homology groups

can be thought of as a reﬁnement of the Casson invariant of an integral ho-

mology 3-sphere. An extension of the Casson invariant to a rational homology

3-sphere is deﬁned in Walker [394](see[64] for another approach).

A method for calculating the spectral ﬂow in the Fukaya–Floer theory for

a split manifold is given in [416]. Recall that a connected closed oriented

3-manifold is said to be split if

M = M





= ∂M

= Σ

,g>1,

where M

, M

are 0-codimension submanifolds of M and Σ

is a connected,

closed Riemann surface of genus g oriented as the boundary of M

.Thenwe

have the following theorem

Theorem 10.4 (Yoshida) Let M be split manifold. Let A

, i =1, 2,be

smooth irreducible ﬂat connections on the trivial bundle M × SU(2) with

Ker(d

)=0. Assume that the connections A

restrict to smooth irreducible

We would like to thank Ron Stern for conﬁrming these corrections.

10.6 In t eger-Graded Instanton Homology 337

ﬂat connections B

on the trivial bundle Σ

× SU(2). Then there exists a

metric on M and a smooth generic path of connections A

, 0 ≤ t ≤ 1,on

M × SU(2) such that A

restricts to a path B

× 1 on a tubular neighbor-

hood of Σ

and deﬁnes a path

in the space of all Lagrangian pairs in

the 6(g −1)-dimensional symplectic vector space of the equivalence classes of

representations of π

(Σ

) into SU(2).Letγ(

) be the corresponding Maslov

index. Then

sf(A

)=γ(

) (10.59)

where sf denotes the spectral ﬂow.

This theorem can be viewed as a link between the Fukaya–Floer homology

and the symplectic homology. In fact, similar ideas have been used by Taubes

in his interpretation of the Casson invariant. An application of the above

theorem leads to a calculation of the Floer homology groups of a class of

manifolds as indicated in the following theorem (see [416] for a proof).

Theorem 10.5 Let N

, 0 >k∈ Z, denote the integral homology 3-

sphere obtained by the (1/k)-Dehn surgery along the ﬁgure eight knot in

. Then it can be shown that the Floer homology groups FH

) are

zero for odd i and are free for even i.Ifwedenotebyr

) the rank

of the ith Floer homology group of the manifold N

then the set of ranks

),r

)} determines the Floer homology of N

.Com-

putations of these ranks for all k ∈ Z, k<0, is given in the Table 10.3.

Table 10.3 Floer homology of homology 3-spheres N

, k<0

k r

) r

)

−4m 2m 2m 2m 2m

−4m +12m −1 2m 2m − 1 2m

−4m +22m −1 2m −1 2m −1 2m − 1

−4m +32m −2 2m −1 2m −2 2m − 1

This table can also be used to determine the Floer homology groups of the

homology 3-spheres N

−k

, 0 >k∈ Z, by noting that N

−k

is orientation-

preserving diﬀeomorphic to −N

and that

(−N

)=FH

3−i

), ∀i ∈ Z

An examination of Table 10.3 shows that Floer homology of the homology

3-spheres N

is periodic of period 4, i.e.,

)=FH

i+4

), ∀i ∈ Z