Kothari D.P., Nagrath I.J. Modern Power Systems Analysis
Подождите немного. Документ загружается.

lM
I
ftrodern
Power
System
Analysis
(i)
Et
is
V,= 7
d
by 20Vo
at same
real load.
Now
As
per
Eq.
(a.28)
l
E
,llv,l
P=
' '
sind
x,
0.9
=
(2'388xr
)
,i' d
\ 1.344
)
sin d=
0.5065
6
=
30.4"
=
0.89
-
j0.79
=
1.183
l-
4L2"
(a)
pf
=
cos
4I.2"
=
0.75
lagging
(b)
Reactive
power
drawn
bY
load
Q
=
lV,lllol
sin
/
=1x1.183x0.659
=
0.78
pu
or
502.8
MVAR
(ii)
E,
decreased
by
20o/o
or
Ef=
1.99
x
0.8
=
1.59
Substituting
in Eq.
(i)
oe=
(L2\L),in
6
\ 1.344
)
which
gives
6
-
49.5"
In=
t.59149,5"-rlo"
4'r
Figure
P-4'l
shows
the
schematic
diagram
of
a
radial
transmission
system'
The
ratings
and
reactances
of
the
various
components
are
shown
rherein.
A load
of
60
MW
at
0.9- power
factor
ragging
is
tapped
from
the
66
kv
substation
which
is
to
be
mainraineo
alt
oo
kv.
calcurate
rhe
terminal
voltage
of
the
synchronous
machine.
Represent
the
transmission
line
and
the
transforrners
by
series
reactances
ontu.
.
(b)
or
Q
=
0.024
x
645
=
15.2
MVAR
PROB
IEIvI
S
11t220
kv
V1
100
MVA
X
=
10o/o
Fig.
p_4.1
4.2
Draw
the pu
inrpedance
diagram
fbr
the
power
system
shown
in
nig.
e-
4.2.
Neglect
resistance,
and
use
a
base
of
ioo
vrre
,
220
kv
in
50
()
rine.
The
ratings
of
the
generator,
motor
and
transformers
are
Generator
40
MVA,
25
kV,
Xu
=
20Vo
Motor
50
MVA,
I I
kV,
X,t
=
30Vo
Y-Iltransformer,
40
MVA,
33
y_220
y
kV,
X
=
I5Vo
Y-l
transformer,
30
MVA,
ll
L_220
y
kV,
X
=
l5*o
(i)
or
or
2.388130.4"-110"
jr.344
220t66
kV
dh
160
kv
_-__-EF_-----l___>
60
MW
5 F
|
0.9
pf
tagging
100
MVA
V2
X
=
Bo/o
-1f
1M)
Y
\')
/
I
U-vGi-r+1,-__iF--r-F
,50o
-.*fF-.,
l_
.l
l--YY-
I
[y^
2
Fig.
p-4.2
A
synchronous
generator
is
rated
60
MVA,
1r
kv.
It
has
a
resistance
Ro
=
0-l pu
and
xo
7
r.65
pu.
It
is
feeding
into
an
infinite
bus
bar
ar
11
kV
delivering
a
cirrrent
3.15
kA
at
0.g
ff
hgging.
(a)
Determine
E,
and
angte
d
(b)
Draw
a
phasor
diagram
for
this
operation.
(c)
Bus
bar
voltage
fails
to
10
kv
while
the
mechanical
power
input
to
generator
and
its
excitation
remains
unchanged.
wtrat
is
the
value
and pf
of
the
current
delivered
to
the
bus.
In
this
case
assume
the
.i1.344
=0.9-j0.024
=
0.9
l-1.5"
=
1;
unity
pf
tation
of
Power
x
0.9
x
sin
1.5
=
0.024
(a)
pf
=
cos
1.5"
4.3

I
126
|
Modern
Power
System Analysis
T
generator
resistance
to be
negligible.
4.4
A 250
MVA, 16
kV rated
generator
is feeding
into an infinite
bus bar
at
15
kV. The
generator
has
a synchronous
reactance
of 1.62pu.lt
is found
that
the machine
excitation
and
mechanical
power
input are adiusted
to
give
E,
=
24
kY and
power
angle
6
=
30o.
(a)
Determine
the line current
and
active and
reactive
powers
fed
to the
bus
bars.
(b)
The
mechanical power
input
to the
generator
is
increased by
20Vo
from
that
in
part
(a)
but its
excitation
is
not changed. Find
the
new
line
current
and
power
factor.
(c)
With
reference
to
part
(a)
current
is to
be reduced by
20Vo at
the
same
power
factor
by adjusting
mechanical
power
input
to
the
generator
and its excitation.
Determine
Ey, 6 and mechanical
power
rnput.
(d)
With the
reduced
current as
in
part
(c),
the
power
is to be
delivered
to bus
bars
at unity
pf,
what
are ttre
corresponding values
of El
and
d and also
the rnechanical power
input
to the
generator.
4.5 The
generator
of Problern
4.4 is
feeding
150 MVA at
0.85
pf
lagging
to
infinite bus
bar at 15 kV.
(a)
Determine
Ey
and d for the
above operation.
What are
P and
Q
fed
to the
bus
bars?
(b)
Now E,
is reduced
by l0o/o
keeping
mechanical
input to
generator
same, find
new dand
Q
delivered.
(c)
Et is now
maintained
as in
part
(a)
but
mechanical
power
input to
generator
is
adjusted till
Q
=
0. Find new
d and P.
(d)
For the value
of Eyin
part
(a)
what is
the maximum
Qthat
can
be
delivered
to bus bar. What
is the
corresponding
6and
{,?
Sketch
the
phasor
diagram
for each
part.
Answers
4.1
12 kV
4.3
(a)
26.8 kV
(line),
42.3" leading
(c)
i.i3
l-28.8" kA;
0.876 lag
4.4
(a)
0.5ll"l- 25.6"
kA; 108
MW,
51.15 MVAR
(b)
6.14 kA,
0.908
lagging
(c)
1.578,
13.5o,
53.3 MW
(d)
18.37 kv,'35.5",
96 MW
4.5
(a)
25.28
kV, 20.2',127.5
MW,
79.05 MVAR
(b)
33.9", 54 14 MVAR
(c)
41.1",
150.4 MW
(d)
184.45,MVAR,
53.6",
-
7
0.787
pu
-
Representation
oflgygr_gyg!"_!L_qg[p!g$q
REFERE
I.ICES
Books
l' Nagrath,
I'J.
and
D.P-
Kothari,
Electric
Machines,
2nd
edn
Tata
McGraw-Hill,
New
Delhi,
7997.
2' van
E'
Mablekos,
Electric
Machine
Theory
for
Power
Engineers,Harperlno
Raw,
New
York,
1980.
3.
Delroro,
v.,
Electric
Machines
and
power
systems,
prentice_Hall,
Inc.,
New
Jersey,1985.
4'
Kothari,
D.P.
and
I.J.
Nagrath,
Theory
and.
Problems
of
Electric
Machines,
2nd
Edn,
Tata
McGraw-Hill,
New
De\hi,
2002.
5.
Kothari,
D.p.
and
I.J.
Nagrath,
Basic
Electicar
Engineering,
2nd
Edn.,
Tata
McGraw-Hill,
New
Delhi.
2002.
Paper
6'
IEEE
Cornittee
Report, "The
Effect
of
Frequency
and
Voltage
on
power
System
Load",
Presented
at
IEEE
winter
po,ver
Meeting,
New
york,
1966.

5.1
INTRODUCTION
This
chapter
deals primarily
with
the
characteristics
and performance
of
transmission
lines.
A
problem
of
major
importance
in
power
systems
is the
flow
of
load
over
transmission
lines
such
that
the
voltage
at
various
nodes
is
maintained
within
specified
limits.
While
this
general
interconnected
system
problem
will
be dealt
with
in
Chapter
6,
attention
is presently
focussed
on
performance
of a
single
transmission
line
so
as
to
give
the
reader
a clear
understanding
of the
principle
involved.
Transmission
lines
are
normally
operated
with
a balanced
three-phase
load;
the
analysis
can
therefore
proceed
on a
per
phase
basis.
A
transmission
line
on
a
per
phase
basis
can
be
regarded
as
a two-port
network,
wherein
the
sending-
end voltage
Vr
and
current
15 are
related
to the
receiving-end
voltage
Vo and
current
1o through
ABCD
constants"
as
ehara-cteristics
and
Perforr"nance
of
povrer
Transmission
Lines
The
following
nomenclature
has
been
adopted
in this
chapter:
z
=
series
impedance/unit
length/phase
y
=
shunt
admittance/unit
length/phase
to neutral
;=;l_*#:,T.":Jff::
C
=
cepacitance/unit
length/phase
to neutral
/
=
transmission
line
length
Z
=
zl
=
total
series
impedance/phase
Y
=
ll
=
total
shunt
admittance/phase
to
neutral
Note:
Subscript
,S stands
for
a
sending-end
quantity
and
subscript
R
stands
for
a receiving-end
quantity
5.2
SHORT
TRANSMISSION
LINE
For
short
lines
of length
100
km
or less,
the
total
50
Hz
shunt
admittance*
QwCl)
is small
enough
to
be
negligible
resulting
in
the
simple
equivalent
circuit
of Fig.
5.1.
Fig.
5.1
Equivalent
circuit
of a
short
line
This
being
a
simple
series
circuit,
the
relationship
between
sending-end
receiving-end
voltages
and
currents
can
be
immediately
written
as:
lyrl Il
zflvol
Lr,J
=
Lo
rJLr-.J
(5'3)
The
phasor
diagram
for
the
short
line
is
shown
in
Fig.
5.2
for
the
lagging
current
case.
From
this
figure
we
can
write
lV5l
=
l(ly^l
cos
/o
+
lllR)z
+
(lV^l
sin
dn
+
lllxyzlr/2
lv5
l=
[tvRP+
llt2
(Rz
+
f)
*ztvR|il (Rcos
Q^+
X
sin
fu)rl2
(5.4)
(s
r)
/< t\
These
constants
can
be
determined
easily
for
short
and
medium-length
lines
by
suitable
approximations
lumping
the
line
impedance
and
shunt
admittance.
For
long
lines
exact
analysis
has
to
be
carried
out
by
considering
the
distribution
of
resistance,
inductance
and
capacitance
parameters
and
the
ABCD
constants
of the line
are
determined
therefrom.
Equations
for
power
flow
on
a
line
and receiving-
and
sending-end
circle
diagrams
will
also
be
developed
in
this
chapter
so
that various
types
of
end
conditions
can
be handled.
*R"f'..
to
Appenclix
B.
lyrl lA
BlIy*l
L1,J
Lc
plLroJ
Also the
following
identity
holds
for
ABCD
constants:
AD-BC=l
It2
tvRP
-;-
-
For
overhead
transmission
lines,
shunt
admittance
is
mainly
capacitive
susceptance
(iwcl)
as the
line
conductance (also
called
leakance)
is
always
negligible.
=
tvnt
f
r
*
?JlJr
cos
/o
+
4#rin
Qp
t
"L
lVRl
/A
,I/i-"
-{
w
. rl/
rl
J
1il2
1n2
+
x2
The
last
term is usually
of
negligible
order.
Characteristics and
Performance of
Power
Transmission
Lines
J"#,
--1
l/l
R
cos
/^+l1l
X sin
d^
x
100
I
yRl
In the above
derivation,
Q*has
been considered
positive
for
a
lagging
load.
(s.7)
(s.8)
(5.e)
Expanding binomially and retaining first order
terms, we
get
(s.s)
The
above
equation is
quite
accurate for the normal
load range.
Fig. 5.2
Phasor diagram of
a short
line for lagging
current
Voltage Regulation
Voltage regulation
of a transmission
line is defined
as the rise in
voltage at the
receiving-end,
expressed as
percentage
of full
load voltage,
when full load at
a
specified
power
factor
is thrown off,
i.e.
Per cent
regutation
=
''^?)-:'Yu'x
100
(5.6)
lvRLl
where lVool
=
magnitude
of no load
receiving-end
voltage
lVprl
=
magnitude
of full load
receiving-end
voltage
I
vs l=l v^ rfr
.
ff
cos
/^
+
ff
sin
f^)'''
lV5 l= lV^l+lX(Rcos
/o+Xsin
/o)
(for
leading load)
Voltage regulation
becomes
negative
(i.e.
load voltage is more than
no load
voltage),
when in Eq.
(5.8)
X sin
Qo>
R cos
/p,
or tan </o
(leading)
>+
X
It also
follows
from Eq.
(5.8)
that for zero
voltage
regulation
per
cenr regularion
-
|
!!4 9"-t
!t:l
n x tin
4-r
x
100
lvRl
tan
/n=
X
=cot
d
i.e.,
/^(teading=
[-
e
where d
is the angle of the
transmission
line impedance. This is, however,
an
approximate
condition.
The exact
condition for zero regulation is determined
as
follows:
Fig. 5.3
Phasor diagram
under zero
regulation condition
Figure 5.3
shows
the
phasor
diagram
under conditions of zero
voltage
regulation,
i.e.
lV5
I
=
lVpl
or
OC= OA
sn
/AOD
_
AD
-
AClz
-_
llllzl
oA
lyR |
zlvRl
(at
a
specified
power
factor)
For short
line, lV^61
=
lVsl,
lVsl
=
lVpl
Per cent
regutation
=
'u1|,'%'
lvRl
',
or
IAOD=
sin-r
U!4
zlvRl
lt
follows
from
the
geometry
of
angles
at
A,
that
for
zero
voltaee
regulation,
/p
(leading)
=
Characteristics
and
Performance
of
Power
Transmission
Lines
133
or
new
value
of
lVr
|
=
11.09
kV
Figure
5.4
shows
the
equivalent
circuit
of
the
line
with
a capacitive
reactance
placed
in
parallel
with
the
load.
R+jx
ll!
l-
-,L-
- -l
Fig. 5.4
Assuming
cos
y''^
now
to
be the
power factor
of
load
and capacitive
reactance
taken
together,
we
can
write
(11.09
-
10)
x
103
=
l1n
|
(R
cos
d^+
X sin
dn)
Since
the
capacitance
does
not
draw
any real
power,
we have
5000
l/ol=
10
x
cos
/^
Solving
Eqs.
(i)
and
(ii),
we
get
cos
dn=
0'911
lagging
and
llal=
549
A
Now
Ic=
In-
I
=
549(0.911
-
j0'412)
-707(0.107
-
j0.70'7)
=
0.29
+
j273'7
Note
that
the
real
part
of 0.29
appears
due the
approximation
in
(i)
Ignoring
it,
we
have
I,
=
j273.7
A
'
Y
:ltl
-loxlooo
'^L
3wxc
ll. I
273'7
or
C-81
P'F
(c)
Efficiency
of
transmission;
(5.10)
From
the
above
discussion
it
is
seen
that
the
voltage
regulation
6f
a
line
is
heavily
dependent
upon
load
power
factor.
voltage
regulation
improves
(decreases)
as
the power
factor
of
a lagging
load
is
increased
and
it
becomes
zero
at
a
leading
power
factor
given
by
Eq.
(5.10).
A
single-phase
50
Hz generator
supplies
an
inductive
load
of
5,000
kw
at
a
power
factor
of
0'707
lagging
by
means
of
an
overhead
transmission
line
20
km
long.
The
line
resistance
and
inductance
are
0.0195
ohm
and
0.63
mH
per
km'
The
voltage
at
the
receiving-end
is
required
to
be
kept
constant
at
10
kv.
Find
(a)
the
sending-end
voltage
and
voltage
regulation
of
the
line;
(b)
the
value
of
the
capacitors
to
be
placed
in parailet
viittr
the
load
such
that
the
regulation
is
reduced
to
50vo
of
that
obtained
in part
(a);
and
(c)
compare
the
transmission
efficiency
in parrs
(a)
and
(b).
Solution
The
line
constants
are
R
=
0.0195
x
20
=
0.39
f)
X
=
3I4
x
0.63
x
10-3
x
Z0
=
3.96
e
(a)
This
is
the
case
of
a
short
line
with
I
=
Ia=
1,
given
by
l1l
=
--5000
=707
A
10x0.70i
From
Eq.
(5.5),
lV5
l=
lVol+
l1l
(R
cos
Q*+
Xsin
/^)
=
10,000
+
707(0.39
x
0.701
+
3.96 x
0.707,\
y
=
72.175
kV
Voltage
regulation
=
pfTL--
l9-
x
roo
:Zt.j7vo
10
(b)
Voltage
regularion
desired
=
?+t
=
l0.9Vo
lys
t-
10
(i)
(ii)
t0
=
0.109
Case
(a)

Case
(b)
characteristics
and Pedormance
of Power
Transmission
Lines
LI{S
f*
Per unit
transformer
impedance,
5000
r/=
-
g7.7%o
'
5000
+
(549)2
x
0.39
x
10-3
-
'
/u
Lrr6f.L
uJ
prdvrtg
,
uapacltor
ln
parallel
wlth
the
load,
the
receiving-end
power
factor
improves
(from
0.707
iug
to
0.911
lag),
the
line
current
reduces
(from
707
A
to
549
A),
the
line
voitage
regulation
decreases
(one
half
the
previous
value)
and
the
transmission
"ffi"i"nJy
i-proves
(from
96'2
to
97 '7vo)'
Adding
capacitors
in
parallel
with
load
is
a
powerful
method
of
improving
the performance
of
a transmission
system
and
will
be
discussed
further
towards
the
end
of
this
chapter.
=
f
(O.OU
+
7O.36)
=
(0.02
+
/0.12)
O/phase
Zr=
(0.02+
j0.12)x5
_
(0.5+13.75)x5
6.q2
Q'2
A
substation
as shown
in
Fig.
5.5
receives
5
MVA
at
6
kv,
0.g5
lagging
power
factor
on
the
low
voltage
side
of
a
transforner
from
a
power
station
through
a
cable
having
per
phase
resistance
and
reactance
of
8 and
2.5
ohms,
respectively.
Identical
6.6/33
kV
transfoffners
are
installed
at
each
end
of
the
line.
The
6'6
kV
side
of
the
transfonners
is
delta
connected
while
the
33
kV
side
is
star
connected.
The
resistance
and
reactance
of
the
star
connected
windings
are
0.5
and
3'75
ohms,
respectively
and
for
the
delta
connected
windings
arJ0.06
and
0.36
ohms.
what
is
the
voltage
at
the
bus
at
the power
station
end?
6.6/33
kV
33/6.6
kV
Fig.
5.5
Solution
lt
is
convenient
here
to
employ
the per
unit
method.
Let
us
choose,
Base
MVA
=
5
Base
kV
=
6.6
on
low
voltage
side
=
33
on
high
voltage
side
Cabie
impeciance
=
(8
+
jZ.S)
e/phase
_
(8+r2.s)xs
(33)'
:
=
(0'037
+
io'0r15)
Pu
Equivalent
star
impedance
of
6.6
kv
winding
of
the
transformer
=
(0.0046
+
j0.030)
pu
Total
series impedance
=
(0.037
+
j0.0115)
+
2(0.0046
+
j0.030)
=
(0.046
+
j0.072)
pu
Given: Load
MVA
=
1
pu
Loadvoltage
=
+
=
0.91
pu
6.6
Load current
=
-.1-
=
1.1
pu
0.91
Using Eq.
(5.5),
we
get
lVs
|
=
0.91 + 1.1(0.046 x
0.85 +
0.072
x
0.527)
=
0.995
pu
=
0.995
x
6.6
-
6.57 kV
(line-to-line)
Input to a single-phase
short line
shown
in Fig.
5.6 is
2,000
kw
at 0.8
lagging
power
factor. The
line has
a series
impedance
of
(0.4
+
j0.a)
ohms.
If
the
load
voltage is
3 kV, find
the load and
receiving-end
power
f'actor.
Also
tind
the
supply
voltage.
2,000 kw
I
at 0.8
pf
*Vs
lassins
L
Fig.
5.6
Solution It is a
problem
with
mixed-end
conditions-load
voltage
and
input
power
are
specified.
The exact
solution is
outlined
below:
Sending-end active/reactive power
=
receiving-end
active/reactive
power
+
activ ekeactive
line
losses
For active
power
lys I l1l
cos
ds=
lVRl lll cos
/a
+
l1l2p
(i)
For reactive
power
lys
I
l/l
sin
g55=
lVpl l1l
sin
Qo+
ltl2X
(ii)
I
I
3kv
I
__1
'F6,
I
Modern
Power
System
Anatysis
Squaring (i)
and
(ii),
adding
and
simplifying,
we
get
lvrl2
lll2
=
lVnlz
lll2 +
zlvRl
lll2
(l1lR
cos
/o
+
tItX
sh
/n)
+
tlta
@2
+
f)
(iii)
the
numerical
values
given
lZl2=
(R2+
f)=0.32
lysl
l1l
-
2,oo-ox1o3
-
2,500
x
103
0.8
lVs
I l1l
cos
/,
-
2,000
x
103
lysllll
sin
/5=
2,500
x
103 x
0.6
=
1,500 x
103
From
Eqs.
(i)
and
(ii),
we get
,n
2000x103
-0.4ltP
l1l
cosPo=F
l1l
sin
/o
1500x103
-
0.4tIf
3000
Substituting
all the
known
values
in
Eq.
(iii),
we
have
(2,500
x
103;2
=
(3,000)'
til2
+ 2 x
3,000
t|210.4*
29W
"19!
p'{lt
L
3000
+0.4x
1s00xlq1r0.4112
l+
o.zz
tt
ta
3000
J
Simplifying,
we
get
0.32
Vf
-
11.8
x
106
lll2+
6.25
x
1012
=
0
which
upon
solution yields
t|_725
A
Substituting
for
l1l in
Eq.
(iv),
we
ger
cos
Qp
=
0.82
Load
Pn
=
lVRl
lll cos
/a
=
3,000
x
725
x
0.82
=
1,790
kW
Now
lV5
|
=
l1l cos
ds
=
2,000
2000
Characteristics
and
Performance
of
Power
Transmission
Lines
l.
l3?.,-{
I-
5.3
MEDIUM
TRANSMISSION
I,INE
For lines
more
than
100
km long,
charging
currents
due to shunt
admittance
100 km
to 250
km leneth. it
is
sufficiently
accurate
to lump
all
the
line admittance
at the
receiving-end
resulting
in
the
equivalent
diagram
shown
in Fig. 5.7.
Starting
frorn
fundamental
circuit
equations,
it
is fairly straightforward
to
write the transmission
line
equations
in
the ABCD
constant
form
given below:
[:]
=l'*;'llVl
Fig. 5.7
Medium
line,
localized
load-end
capacitance
Nominal-f
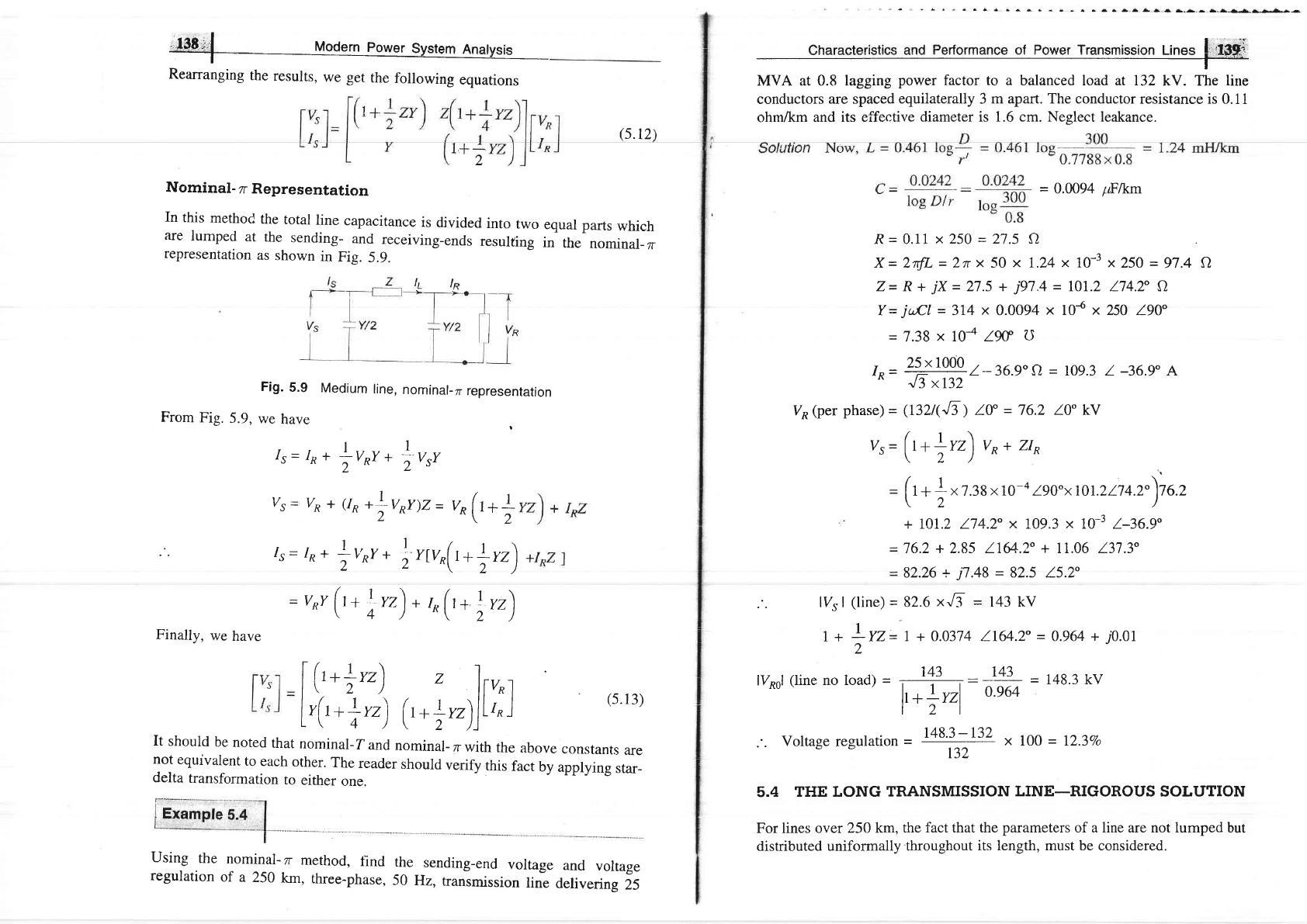
Representation
If
all
the shunt
capacitance
is
lumped
at
the middle
of
the line, it
leads
to the
nominal-Z
circuit
shown
in
Fig. 5.8.
Fig.
5.8
Medium
line, nominal-T
representation
For
the
nominal-Z
circuit,
the following
circuit
equations
can be
written:
Vc=
Vn+
Io(Zl2)
Is
=
In
+ VrY
=
In
-r
Wo+
IR(Z|L)Y
Vs
=
Vc
+
it
(ZiZ)
Substituting
for
Vg
and
1,
in the
last equation,
we
get
(s.1
1)
(iv)
(v)
vs
=
vn
+ I^
(zt2)
+
(zD)
[r^(t
.
+)+
YvR)
=
vn(,.
t{)+
r
nz(t.
tf)
lV5
l=
725x0.8
:3.44
kY
Rearranging
the
results,
we get
the
following
equations
(s.12
+
2
Nominal-
zr
Representation
In
this
methoc
the
total
line
capacitance
is
divided
into
two
are
lumped
at
the
sending-
and
receiving-ends
resulting
representation
as
shown
in
Fie.
5.9.
equal
parts
which
in
the
nominal-
zr
l.
-r
Characteristics
and
Performance of Power Tralq4lqslon
Lines
[l$k
t
MVA at 0.8 lagging
power
factor to a
balanced load at 132
kV. The
line
conductors
are spaced
equilaterally
3
m apart. The conductor
resistance is 0.11
ohmlkm
and its effective
diameter is 1.6 cm. Neglect leakance.
=
0.0094
pFlkrrr
Fig.
S.9
Medium
line,
nominal_7r
representation
From
Fig.
5.9,
we
have
_tl
Is=
In
+
-VoY
+
2Vsy
R
=
0.11
x
250
=
27.5 C)
X
-
ZrfL
=
2rx 50
x
I.24
x
10-3
x
250
=
97.4
Q
Z= R +
jX
=
27.5 +
j97^4
=
I0I.2 174.T
Q
Y
=
jutl
=
314
x
0.0094
x
10{
x
250
lg0"
=
7.38
x
104 lW
U
r^
=
#9
l--i6.g"o
=
109.3 I
-369"
A
"13
xl32
vo
(per
phase)
=
(I32/d,
)
10"
=
76.2 l0 kv
/1\
vs=
[
t++YZl
vR+ zI*
\ 2 )"
r1
I
i
+:
x
7.38
x
io-a 190"x10r.2134.2"\to.z
\2)
+ 101.2 174.2"
x
109.3
x
10-3 l-36.9"
76.2 + 2.85 l1&.2'
+
11.06 137.3"
82.26
+
j7.48
-
825 15.2"
82.6
xJl
-
143 kv
1 + 0.0374 ll&.2"
=
0.964
+
70.01
rv^or
(rine
no load)
=
ffid:;#
=
148.3 kv
tzl
Voltage regulation
=
W#2
x 100
-
l2.3vo
5.4
THE LONG
TRANSMISSION
LINE-RIGOROUS
SOLUTION
For lines over
250 km, the
fact that the
parameters
of a line are not
lumped
but
distributed uniformally
throughout
its length, must be
considered.
Vs=
1s=
vo
+
eo
*,)vov>z
=
vn(r.*
r**
tvoy
+
t;trr-(t+|vz)
vov
(t
+
t^vz).
+
(,
+
)vz)
f / 1 \
I
('*
i4
z
l,u.r
|
'r
-'t
',
/ |
.,
ll -" |
(5.13)
LrU+;rt)
[t*r")]L1nr
nominal-zand
nominal-rrwith
the
above
constants
are
ther.
The
reader
should
verify
this
fact
by
applying
star_
either
one.
at
to
to
lV5 |
(line)
=
I +
LYZ=
2
d
tha
each
ion
t
l noted
3nt
to
e
ormati<
Jbe
vale
nsfc
uld
t
lurva
trans
hou
equ
:a tr
It
sl
not
delt
Finally,
we
have
Using
the
regulation
nominai-
z-
method,
find
of
a
250
km,
three-phase,
the
sending-end
voltage
50
Hz,
transmission
line
and
voltage
delivering
25
-149
1
mooetn
Po*",
Sv
sis
I
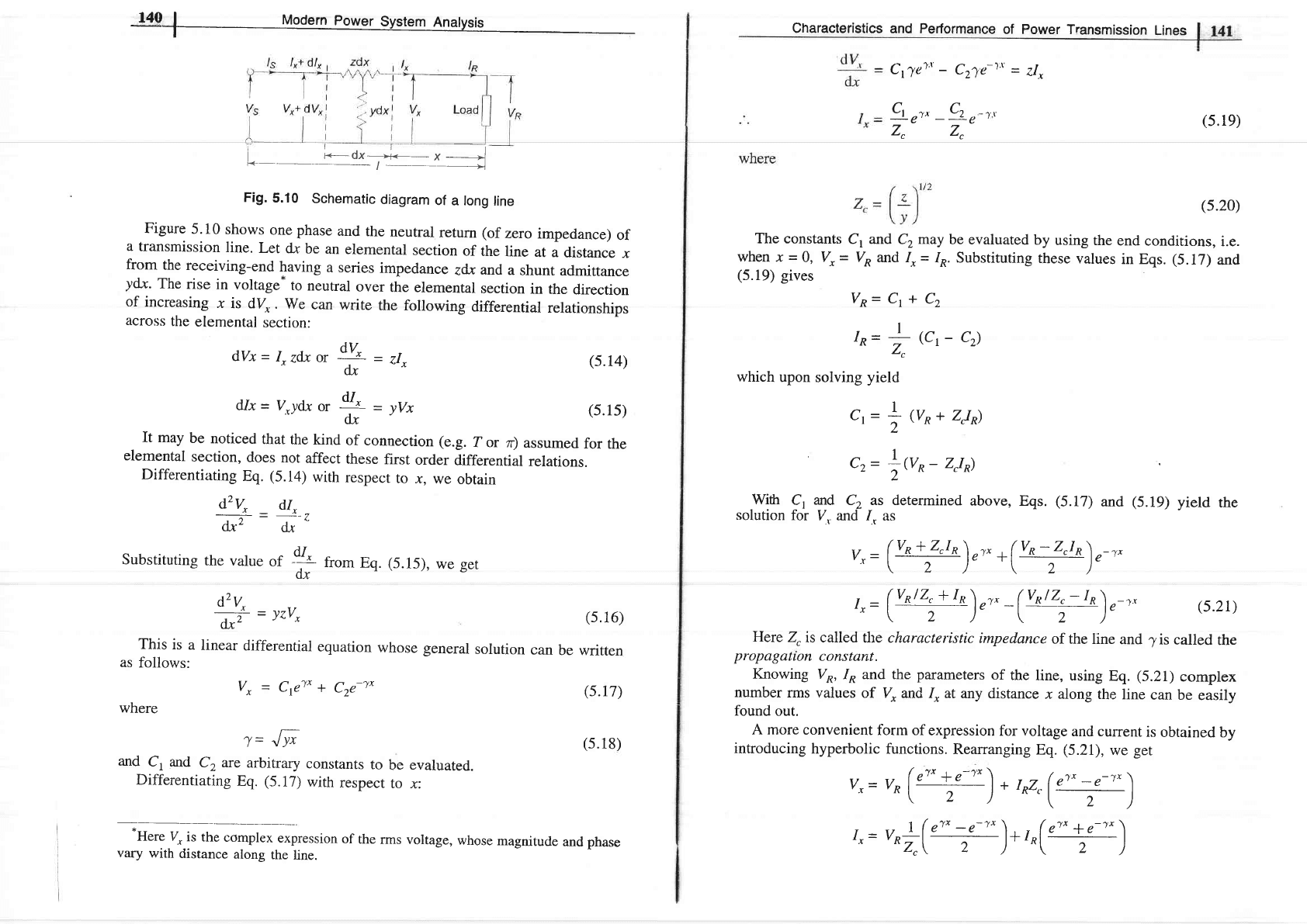
Fig.
5.10
Schematic
diagram
of
a long
line
Figure
5.10
shows
one phase
and
the
neutral
return
(of
zero
impedance)
of
a
transmission
line.
Let
dx
be
an
elemental
section
of
the
line
at u
dirtunr.
"
from
the
receiving-end
having
a series
impedance
zdx
and
a
shunt
admittance
yd-r.
The
rise
in
voltage*
to
neutral
over
the
elemental
section
in the
direction
of
increasing
"r
is dV".
We
can
write
the following
differential
relationships
across
the
elemental
section:
dVx
=
Irzdx
o,
Y-
=
ZI,
d,lx
=
v*ldx
o,
!1'
=
yvx
It may
be
noticed
that
the
kind
of
connection (e.g.
T or
r) assumed
for
the
elemental
section,
does
not
affect
these
first
order
differential
relations.
Differentiating
Eq.
(5.14)
with
respecr
ro
-tr,
we
obtain
drv,
dI.
-d-T
=
i''
Substituting
the
value
of
+
from
Eq.
(5.15),
we
ger
dx
d2v
Ea
=
rZv'
This
is
a
linear
differentiai
equation
whose
general
solution
can
be written
as
follows:
where
V*=CpI**Cre-1x
7=
,lW
and
C, and
C, are
arbitrary
constants
to
be evaluated.
Differentiating
Eq.
(5.17)
with
respect
to
x:
*-_-
Here V'
is
the
complex
expression
of
the
rms
voltage,
whose
magnitude
and phase
vary
with
distance
along
the
line.
characteristics
and Performance
of Power
Transmission
Lines
C17e)r'-Czle-)'-21.,
9t
,r,
-C,
,-',',
Z,
Z,
(5.20)
The constants
C, and
C2 may be
evaluated
by
using
the
end
conditions,
i.e.
when .r
=
0, Vr= Vn
and 1r=
In.
Substituting
these
values
in Eqs.
(5.17)
and
(5.19)
gives
Vn=
Cr
+
Cz
r^=
!
(ct-
cz)
Lc
which
upon
solving
yield
(5.1e)
(s.2r)
(s.14)
(s.1s)
(5.16)
(s.r7)
(s.18)
,r=
*
(vn+
zJn)
1
Cr=
2Un-
ZJ*)
with
9r
ant
c, as determined
above,
Eqs.
(5.r7)
and
(5.19)
yield
the
solution
for
V.- and 1.
as
,,=
(Yn+/o),,.
*(h.?
),-,.
,.
-_
(bITk),,.
_
(w*),-,.
Here
Z,
is called
the charqcteristic
impedance
of the
line
and
7is
called
the
propagatton
constant.
Knowing
vp,
In and the
parameters
of
the
line, using
Eq.
(5.21)
complex
number
rms values
of I/, and I, at
any distance
x along
the
line
can
be
easily
found
out.
A more convenient
form of
expression
fbr voltage
and current
is
obtained
by
introducing
hyperbolic
functions.
Rearranging
Eq.
(5.21),
we
get
(
o7* + o-7'\ / +
v*=vnt+l+
r^2,(e:J::)
\
2
/
"'(
2
)
I.
=
VoL(
"*
-.t-"
)*
,"
(
e1'
+
e-t'
\
" "2"\
2
)
"\
2
)

These
can
be rewritten
after
intloducing
hyperbolic
functions,
as
Vr=
Vn cosh
1r
+
I^2,
sinh
1r
(5,22)
I,=
Io
cosh
rr
+
V-
-l-.i
(s.23)
Characteristics
and Performance
of
Power
Transmission
Lines
ffi
-
sinh
7/
-
|.+*4*
..=Jyz(H+)
(s.28a)
3!
)!
\^'
6.)
\
This
series
converges
rapidly
for
values
of
7t
usually
encountered
for
power
lines
and can he convenie-nflw
qrrrrrnwi-o.l.r
^l-^i^-.^
-r,L-
--
when
x
=
l,
Vr=
V,
Ir=
Is
Hl
=l;::,,
':::i;:l;;t
Here
A=D
-cosh
7/
B
=
Z,
sinh
7/
c
=
J-sinh
:r/
Z,
A=D=l*
YZ
2
B x,
z
(t.+)
c
x
Y
(r*V\
\
6)
The
above
approximation
is
computationally
convenient
and quite
accurate
for
lines
up
to
400/500
km.
Method
3
cosh
(o/
+
i7t)
-
tat"iot
+-'-?t'-iot
1
Z
_=
;(e"
tpt
+
expressions
for
ABCD
constanis
art
sinh
(o/
+
jpl) -
,at"i0t
_e:de-ipt
*In
case
[vs
1s
]
is known
,
fvn
Inl
can
be
easily
found
by inverting
Eq.
(5.23).
Hl
=l-i,
-:l
[:]
(s.2s)
Evaluation
of ABCD
Constants
The
ABCD
constants
of
a long
line
can
be
evaluated
fiorn
the
results
given
in
F4.
6.24).
It
must
be
noted
that
7
-Jw
is
in
general
a
complex
number
and
can
be
expressed
as
^
7-
a+
jp
(s.26)
The
hyperbolic
function
of.complex
numbers
involved
in
evaluating
ABCD
constants
can be
computed
by
any
one
of the
three
methods
given
uJtow.
Method
I
cosh
(cr./
+
j1l)
=
cosh
ul cos
gl+
j
sinh
a/
sn
pt
(5.27)
sinh
(a/
+
jQl) =
sinh
al
cos
gl
+
j
cosh
a/
sn
pt
Note
that
sinh,
cosh,
sin
and
cos
of
real
numbers
as in
Eq.
(5.27)
can
be
looked
tp
in
standard
tables.
Method
2
(s.24)
:
){r",
tpt
-
(s.28b)
e-"1
l-Bt1
(s.2e)
t-d
l-Bt1
(5.30)
5.5
INTERPRETATION
OF
THE
LONG
LINE
EOUATIONS
As
already
said
in
Eq.
(5.26),
7is
a
comprex
number
which
can
be
expressed
as
7=
a+
jp
The
real part
a
is
called
the
attenuation
constant
and
the
imaginary
part
Bis
called
the
phase
constant.
Now
v, of
Eq.
(5.2r)
can
be
writtin
as
^
V,
=
IVR+
Z,lRlr.,,ritgx+n
*lVn-Z,lnl o--c,x,-tt/,x-e2)
^
|
2
|
| z I'
where
Qr=
I
(Izn
+ I&,)
dz=
I
(Vn-
InZ,)
The
instantaneous
voltage
v*(t)
can
be
written
from
Eq.
(5.30)
as
v*
(t)
=
xd
Ol!-
+
3/tl
,o'
,i@t+r,,.+o,)
Lt2l
cosh
7/
=
r +
+*
#*.
.=(t
.+)
