Kothari D.P., Nagrath I.J. Modern Power Systems Analysis
Подождите немного. Документ загружается.


ffiffi
N4odern
power
System Alqtysis
,
20. Kothari, D.P.
and D.K.
Sharma
(Eds),
Energy Engineering.
Theory
and
practice,
S.
Chand, 2000.
21. Kothari, D,P.
and
I.J. Nagrath,
Basic Electrical
Engineering,
2nd edn,
Tata
McGraw-Hill,
New Delhi,
2002.
(Ch.
l5).
22. Wehenkel,
L.A.
Automatic
Learning
Techniques
in Power
Systems, Norwell
MA:
Kiuwer,
i997.
23.
Philipson, L
and H. Lee
Willis,
(Jnderstanding
Electric
Utilities and
Deregulation,
Marcel Dekker
Inc,
NY. 1999.
Papers
24.
Kusko, A.,
'A
Prediction
of
Power
System Development,
1968-2030',
IEEE
Spectrum, Apl.
1968, 75.
25. Fink, L.
and K.
Carlsen,
'Operating
under
S/ress and
Strain',IEEE
Spectrum,Mar.
r978.
26. Talukdar,
s.N., et. al.,
'Methods
for
Assessing energy
Management
options', IEEE
Trans., Jan.
1981, PAS-100,
no.
I, 273.
27. Morgen,
M.G.
and S.N.
Talukdar,
'Electric
Power Load
Management:
some
Technological,
Economic,
Regularity
and
Social
Issues', Proc.
IEEE, Feb.
L979,
vol.
67, no.2,241.
28. Sachdev,
M.S.,'Load
Forecasting-Bibliography,
IEEE Trans.,
PAS-96,
1977,697.
29.
Spom, P.,
'Our
Environment-Options
on the
Way into
the Future', ibid
May
1977,49.
30. Kothari
D.P., Energy
Problems
Facing the
Third
World,
Seminar to the Bio-
Physics Workshop,
8 Oct., 1986
Trieste
Italy
31.
Kothari,
D.P,'Energy
system
Planning
and Energy
conservation',
presented
at
YYIV Nntinnnl fnnvontinn n ^F llltr NI^r', n-lhi E-k lOQO
s
vJ ttrL, r \w w
yvttll,
I wu, I
7QL,
32. Kothari, D.P.
et. a1.,
'Minimization
of Air Pollution
due to
Thermal
Plants'.
,I1E
(India),
Feb.
1977,
57, 65.
33. Kothari, D.P, and
J. Nanda,
'Power
Supply
Scenario
in India'
'Retrospects
and
Prospects',
Proc. NPC
Cong.,
on Captive
Power
Generation,
New Delhi,
Mar.
1986.
34. National Solar Energy
Convention,
Organised
by
SESI, 1-3 Dec.
1988, Hyderabad.
35.
Kothari, D.P.,
"Mini
and
Micro Flydropower
Systems in India",
invited
chapter in
the book, Energy
Resources
and Technology,
Scientific Publishers,
1992,
pp
147-
158.
36. Power Line, vol.5.
no.
9, June 2001.
37. United Nations.' Electricity
Costs atxd Tariffs:
A
General
Study; 1972.
38. Shikha,
T.S.
Bhatti and
D.P. Kothari,
"Wind
as an Eco-friendly
Energy
Source to
meet the
Electricity Needs
of
sAARC Region",
Proc. Int.
conf.
(icME
2001),
BUET, Dhaka,
Bangladesh. Dec.
2001, pp
11-16.
39. Bansal, R.
C., D.P. Kothari
& T.
S. Bhatti,
"On
Some
of the Design
Aspects of
Wind Energy
Conversion
Systems",
Int.
J. of Energy
Conversion and
Managment,
Vol. 43,
16, Nov. 2002, pp.2175-2187.
40. l). P. Kothari
and Amit Arora,
"Fuel
Cells in Transporation-Beyond
Batteries",
Proc. Nut.
Conf. on Transportation
Systems, IIT Delhi,
April
2002,
pp.
173-176.
41.
Saxena, Anshu,
D. P. Kothari et
al,
"Analysis
of Multimedia
and Hypermediafor
Computer Simulation and
Growtft",
EJEISA,
UK,
Vol
3, 1 Sep 2001,
14-28.
j
^a
2.I
INTRODUCTION
The
four
parameters
which
affect
the
performance
of
a
transmission
line
as
an
elementofapowerSystemareinductance,capacitance,resistanceand
, .rL..++
^^nrrrrnrAnce
which
is
normally
due
to
leakale
over
line
illffilf?;
L*",,;i;;.";;r""i"J
ii
overhead
transmission
lines'
rhis
r -r- --'i+r- +ha caricq line narameters,
1'e'
lnductance
and
resistance'
ffiffir"##r'"*
#;;t;
oi.itiuu,.d
atong
the
line
and
thev
together
i";
th"
,"ri.,
imPedance
of
the
line'
Inductar,c.
i,
;;f;
the
most
dominant
line
parameter
from
a
power
system
engineer,sviewpoint.Asweshallseeinlaterchapters,itistheinductive
reactance
which
limits
the
transmission
capacity
of
a
line'
2,2
DEFINITION
OF
INDUCTANCE
Voltage
induced
in
a
circuit
is
given
by
,
=VY
",
,ti"
flux
linkages
of
the
circuit
in
weber-turns
(Wb-T)'
This
can
be
written
in
the
form
drb
di
,
di.,
e=
,-:L-:v
dt
dr
dr
ance
of
the
circ'lit
in
henrys'
which
in
near
magnetic
circuit,
i'e''
a
circuit
Ies
vary
linearly
with
current
such
that
(2.r)
(2.2)
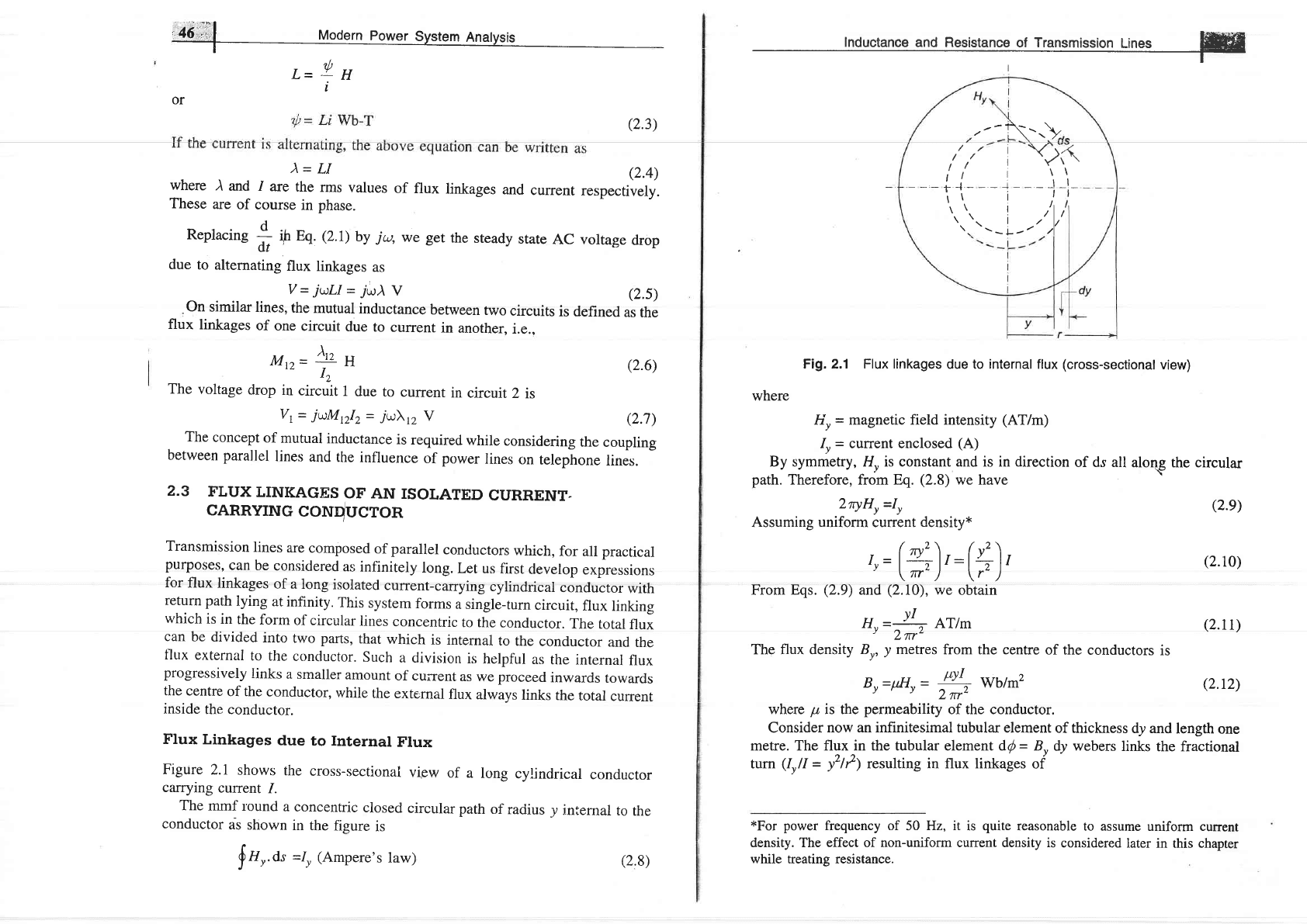
+f
,,il
Modern
po**,
Syrtm
An"lyri,
L=!H
I
or
lnductance
and Resistance
of Transmission
Lines
ffffi
-
Fig. 2.1 Flux linkages
due to internal flux
(cross-sectional
view)
where
H,
=
magnetic field intensity
(AT/m)
/y
=
current enclosed
(A)
By symmetry, H, is.constant and is in direction
of
ds all
alongi the circular
path.
Therefore, from Eq.
(2.8)
we have
(2.e)
(2.10)
(2.rr)
(2.r2)
Mrn=
)t,
,
rL
Iz
The
voltage
drop
in
circuit
1
due
to
current
in
circuit
2
is
V,
=
jwMnlz
=
7t
l\12
V
A
=
LI
e.4)
where
)
and I arc
the
rms
values
of
flux
linkages
and
current
respectively.
These
are
of
course
in
phase.
Replacing
i
+
Eq.
(2.1)
by
ir,
we get
the
steady
state
AC volrage
drop
due
to
alternating
flux
linkages
as
Y=
jwLI =
jt^r)
V
e.5)
-On
similar
lines,
the
mutual
inductance
between
two
circuits
is defined
as
the
flux
linkages
of
one
circuit
due
to current
in
another,
i.e.,
(2.6)
(2.7)
The
concept
of
mutual
inductance
is
required
while
considering
the
coupling
between parallel
lines
and
the
influence
of
power
lines
on telephone
lines.
2.3
FLUX
LINKAGES
OF
AN
ISOTATED
CURRENT.
CARRYTNG
coNqucroR
Transmission
lines
are
composed
of parallel
contluctors
which,
for
all
practical
purposes,
can
be
considered
as
infinitely
long.
Let
us
first
develop
expressions
for
flux
linkages
of a
long
isolated
current-canying
cylindricat
conductor
with
return path
lying
at
infinity.
This
system
forms
a single-turn
circuit,
flux
linking
which
is in
the
form
of circular
lines
concentric
to the
conductor.
The total
flux
can
be
divided
into
two
parts,
that
which
is
internal
to
the
conductor
and
the
flux
external
to
the
conductor.
Such
a division
is
helpful
as
the
internal
flux
progressively
links
a
smaller
amount
of
current
as
we
proceed
inwards
towards
the
centre
of the
conductor,
while
the
external
flux
always
links
the
total
current
inside
the conductor.
Flux
Linkages
due
to Internal
Flux
Figure
2.1
shows
the
cross-sectional
vi,ew
of
a long
cylindrical
conductor
carrying
current
1.
The
mmf
round
a
concentric
closed
circular
path
of
radius y
internal
to
the
conductor
as
shown
in the
figure
is
2rryH,
=1,
Assrrmino rrniform crrrrenf dcnsifv*
,"=(-)t:[4)
,
'
\rrr') \r")
From Eqs.
(2.9)
and
(2.10),
we
obtain
H,.
=)!-
AT/m
t
2Tr"
The flux density
By,
y
metres
from the centre
of the
conductors
is
r ntI
Bu=pHu=
:+
Wb/m2
z ztr-
where
p
is the
permeability
of the conductor.
Consider
now an infinitesimal tubular
element
of thickness
dy and
length
one
metre. The flux in the tubular element dd
=
Bu
dy webers
links
the fractional
trrrn
(Iril
-
yzly'l
resulting in flux linkages
of
(2.3)
*For
power
frequency of
50
Hz, it
is
quite
reasonable
to assume
uniform current
density. The
effect
of
non-uniform
current density is
considered
later in
this
chapter
while
treating resistance.
,---f\-]y
,'.rt
t,
-?5
tll
ir'
i
\\
i+----r
-l-l-
1t
i
!
\
\.
I
t'l
t.rtt-
-ir-." r1/
{nr.ds
=Iy
(Ampere's
law)
(2,8)
Integrating,
we
get
the
total
internal
flux
lin
^^,=I#fdy:ffwat^
(2.14)
For
a relative permeability
lf,,
=
|
(non-magnetic
conductor),
1t
=
4n x
l0-'[Vm.
therefore
f-
and
^rn
=T*10-/
wb-T/m
Lint=
]xto-7
rVm
z
Flux
Linkage
due
to Flux
Between
Two
Points External
to
Conductor
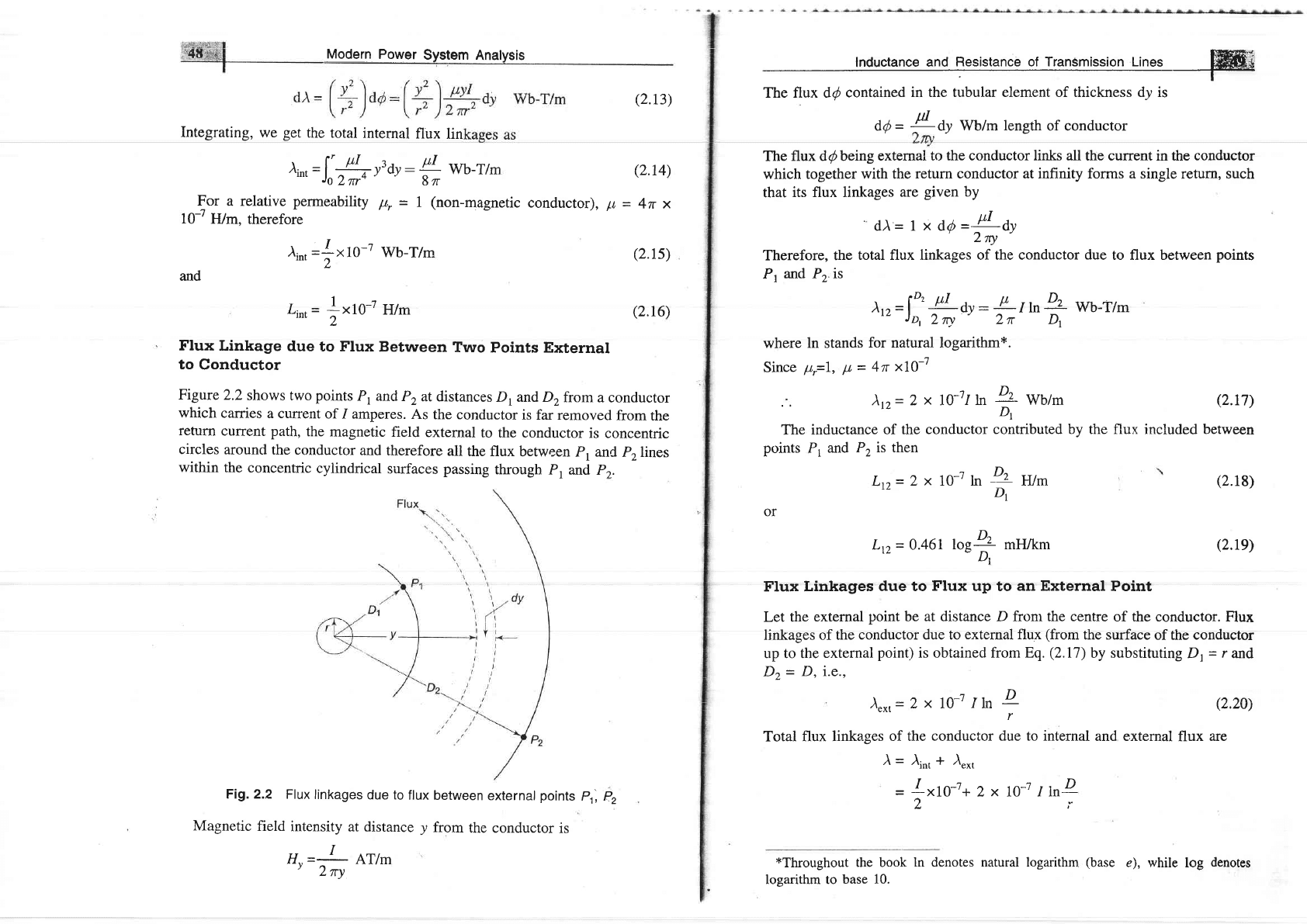
Figure
2.2
shows
two
points
P,
and Prat
distances
D, and Drftoma
conductor
which
carries
a cunent
of 1 amperes.
As
the conductor
is far removed
from
the
return
current path,
the
magnetic
field
external
to
the conductor
is concentric
circles
around the
conductor
and
therefore
all
the flux
between
P, and
Pr lines
within
the
concentric
cylindrical
surfaces
passing
through
P, and
P2.
Fig.2.2
Flux
linkages
due
to flux
between
external
points
Magnetic
field
intensity
at distance y
from
the conductor
is
lnductance
and
Resistance of rransmission Lines
ffi.$
t-
The flux dd
contained
in the tubular element of thickness dy is
dd
=
+dy
Wb/m length
of conductor
The
flux dQbeing
external to
the conductor links all
the current in the
conductor
which together
with the
return conductor at infinity forms a
single return,
such
that
its flux linkages
are
given
by
d)=1
xd6=FI
d,
'
2ny
Therefore,
the
total flux linkages of the conductor
due
to flux between
points
P,
and Pr is
pD",,f
n
\,"
=
|
'
tn'
dy
-
-t"-
I ln
"2
wb-T/m
,1Dt
2 n.v 2r Dr
where
ln
stands
for natural logarithm*.
Since
Fr=I, F
=
4t
x10-7
Modern
Pqwer
System
Analysis
(2.r3)
(2.1s)
(2.16)
The
points
or
-n
)rz= 2
x
l}-tl
ln
=L
wb/m
Dr
inductance
of the conductor
contributed bv
P, and
Pr is then
Lrz
=
2
x
I0-1 fn
-?
fV*
Dl
L,n
=
0.461
los.D' mH,/km
LL
"Dl
(2.r7)
the flux included between
(2.18)
(2.1e)
(2.20)
external
flux
are
H.,=
I
AT/m
'
Zrry
Flux Linkages
due to
Flux
up to
an External
Point
Let the
external
point
be at distance D from the centre
of the conductor. Flux
linkages
of the
conductor due to
external flux
(from
the surface
of the conductor
up to the
external
point)
is obtained from Eq.
(2.17)
by substitutin
E
D
t
=
r
and
Dz
=
D, i'e',
).*,= 2x70-1
lln
D
r
Total
flux linkages
of the
conductor due to internal
and
)= )in,*
)"*,
=Ix1o-7+Zxro-:.
IIn2
2r
*Throughout
the book
ln denotes natural logarithm
logarithm to
base 10.
PI, P2
(base
e),
while log
denotes
W
Modern Power
systgm
Analysis
-.^-
t+rn2)
=2x
10-,/[-4
r )
x
ro_71 t"
*J_r,
I-et
,t
-
,r-r/4
=
0.7788r
\
=
2 x
ro4r h
+
wb-T/m
rl
Inductance
of
the conductor
due to flux
up to an
external
(2.Zra)
point
is
therefore
L=
2x
1o-7 n
I
w^
(z.zrb)
r
Here
r' can be regarded
as the radius
of a fictitious
conductor
with
no
internal
inductance
but
the same total
inductance
as the actual
conductor.
2.4
INDUCTANCE
OF A SINGLE.PHASE
TWO.WIRE
LINE
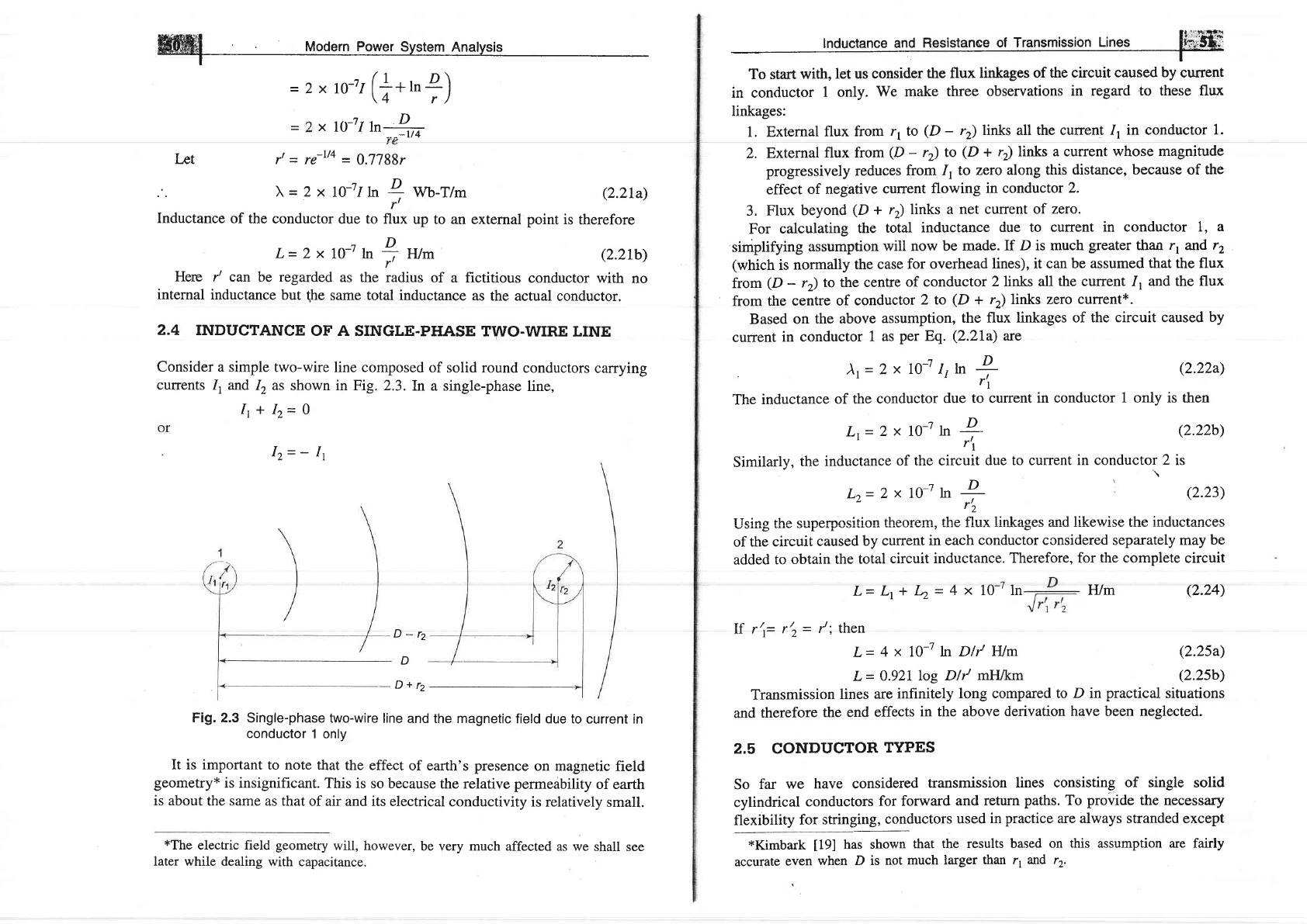
Consider a
simple two-wire
line composed
of solid
round conductors
carrying
currents 1, and
1, as
shown in Fig. 2.3.|n
a single-phase
line,
11+ Ir=
Q
Iz=
-
It
D-rz
D
D+rz
Fig. 2.3
Single-phase
two-wire line
and the magnetic
field due to
current in
conductor
1 only
It is important
to note that
the effect
of earth's presence
on magnetic
field
geometry*
is insignificant.
This
is so because
the relative permeability
of earth
is about
the same
as that of air
and its electrical
conductivitv
is relativelv
small.
*The
electric field
geometry
will, however,
be very much
affected as we
shall see
later
while dealing
with capacitance.
- -
lnductance
and
Flesistanae
of Transmission
Lines
li$Bfr
f--
To start
with,
let us consider
the flux
linkages of
the circuit caused
by
current
in conductor
1 only.
We make
three
observations in
regard to these
flux
linkages:
1. External
flux from
11 to
(D
-
,) links all the current
It in conductor
1.
2.
External
flux
from
(D
-
r) to
(D
+
rr)
links
a current whose
magnitude
progressively reduces
from Irto
zero
along this
distance, because of
the
effect
of negative
current
flowing
in conductor
2.
3.
Flux
beyond
(D
+ 12)
links a net
cunent of
zero.
For
calculating
the
total
inductance
due to
current in conductor 1,
a
simplifying
assumption
will now be
made.
If D is much
greater
than
rt
and
12
(which
is
normally
the
case for
overhead
lines), it can
be assumed that the
flux
from
(D
-
r)
to the centre
of
conductor
2 links all
the current ^Ir and the
flux
from the
centre
of conductor
2 to
(D
+
rr) links zero
current*.
Based
on
the above
assumption,
the flux linkages
of the
circuit caused
by
current
in conductor
1 as
per
Eq.
(2.2Ia)
are
(2.22a)
The
inductance
of the
conductor
due to current
in conductor
1 only is
then
)r=2x10-7
1,
ln
-L
r\
Lt=
2
x
10-7
l"
+
f'1
(2.22b)
Similarly,
the
inductance
of the circuit
due to current
in conductor
2
is
Lz=2x10-7h
D
.
'
r,2
(2'23)
rloinc rha crrncrrrnsifinn fhenrern fhe flrrx linkaoes and likewise fhe indttcfances
vurrrE
ruv
usrvrr
!arvv^v^^^t
of the
circuit
caused
by
current
in each
conductor ccnsidered separately may
be
added
to
obtain the
total
circuit
inductance.
Therefore, for
the complete
circuit
L=
Lt+
4=
4
x
10-'ln
If r/r=
r'z=
/; then
L=
4
x
10-'ln
D// Wm
L
-
0.92t
log Dlr'
mHlkm
FVm
(2.24)
Transmission
lines
are
infinitely
long compared
to D in
practical
situations
and
therefore
the end
effects
in the above
derivation
have
been
neglected.
2.5
CONDUCTOR
TYPES
So
far
we
have considered
transmission
lines consisting of
single solid
cylindrical
conductors
for forward
and
return
paths. To
provide
the necessary
flexibility
for
stringing,
conductors
used
in
practice
are always stranded
except
*Kimbark
[l9]
has shown
that the
results based
on this assumption are fairly
accurate
even
when D is
not much
larger
than 11
and 12.
(2.25a)
(2.zsb)
52
|
Vodern Power
System Analysis
I
fo,
.r.ry small cross-sectional
areas. Stranded
conductors are composed of
strands of
wire,
electrically in
parallel,
with alternate layers spiralled in
opposite direction to
prevent
unwinding. The total number
of strands
(M)
in
concentrically
stranded
cables
with total annular space
filled with strands of
uniform diameter
(rD
is
given
by
N=3x'-3x+l
(2.26a)
where
x
is the number of layers wherein
the single central strand is counted as
the first layer. The overall diameter
(D)
of a stranded
conductor is
P-(2x-r)d
(2.26b)
Aluminium is now the most commonly
employed
conductor
material.
It
has
the advdntages of being cheaper and lighter than copper though with
less
conductivity and tensile strength. Low density
and
low
conductivity result in
larger overall
conductor diameter,
which offers another incidental advantage in
high voltage lines.
Increased diameter results in reduced electrical stress at
conductor surface for a
given
voltage
so that the line is
corona
free.
The low
tensile strength of aluminium conductors is made up
by
providing
central
strands
of high tensile strength
steel. Such
a conductor is
known as alurninium
conductor steel
reinforced
(ACSR)
and is most commonly used in overhead
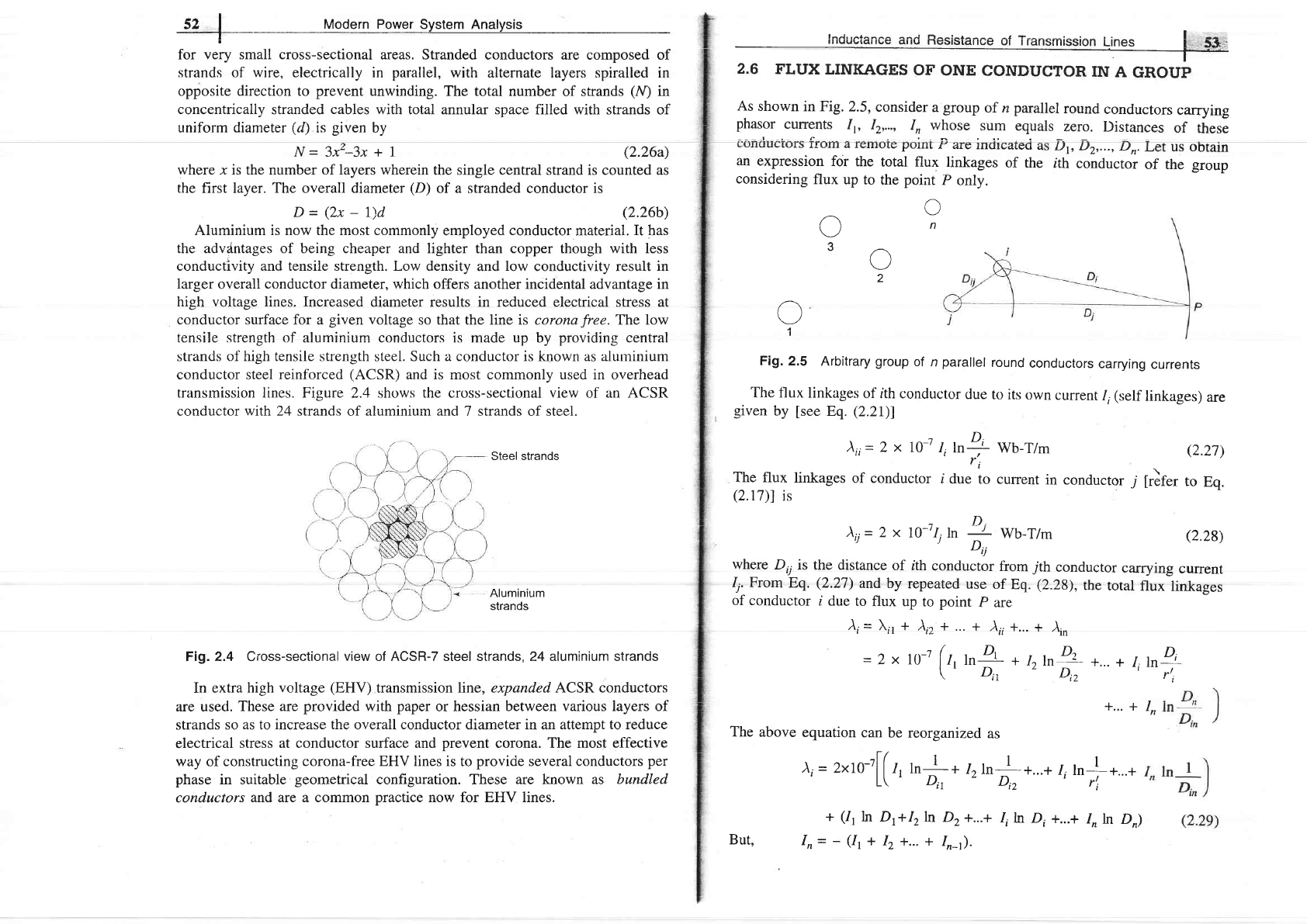
transmission lines. Figure 2.4 shows the cross-sectional view of an ACSR
conductor
wrth 24
strands of aluminium
and
7 strands of steel.
Steel strands
Aluminium
strands
Fig.2.4
Cross-sectional
view
of ACSR-7 steel strands, 24 aluminium strands
In extra
high
voltage
(EHV)
transmission
line, expanded ACSR conductors
are used. These
are
provided
with
paper
or hessian
between various layers of
strands
so as to increase
the
overall
conductor
diameter in an
attempt to reduce
electrical
stress at conductor surface and
prevent
corona. The most effective
way of constructing
corona-free EHV lines is
to
provide
several
conductors
per
phase
in suitable
geometrical
configuration. These are known as
bundled
conductors
and are a common
practice
now for EHV lines.
lnelr rn+ana^ ^^-f at^^l^.^--- ^t r-^- --^:- -! - , r
.
| -^
rrrLrL.vtclttutt
ct'ttu
nt'stl'latlug
ut I
lallsrnlsslon
Unes
!
5.*
"
l*
2.6
FIUX
LINI{AGES
OF
ONE
CONDUCTOR
IN
A
GROUP
As
shown
in Fig.
2.5,
consider
a
group
of n pnallel
round
conductors
carrying
phasor
currents
Ip
12,-,
I, vvhose
sum
equals
zero.
I)istances
of
these
an
expression
for
the
total
flux linkages
of the
lt
ult,..t
un.
us oDtam
ith
conductor
of
the
group
considering
flux
up to
the
point
P
only.
Fig.
2.5
Arbitrary
group
of
n
parallel
round
conductors
carrying
currents
The flux
linkages
of ith conductor
due
to its
own
current
1,
(self
linkages)
are
given
by
[see
F,q.
(2.21)]
o
n
(,
3
2
-
4
I
(2.27)
The
flux linkages
of
conductor
i
due
to current
in
conductor
7
1rlf"r
to
Eq.
(2.17)l
is
)ii=
2
x
10-7
t,
h!
Wb-T/m
ri
5,,= 2
x
l;-il,fn
a
Wb-T/m
Dij
)i
=
Xir +
)iz +
... +
)ii *...
*
)in
=
2
x
rca
(r,
t'*
+
4u
!-
+...+
1,
\^
Dt
'
D,z
(2.28)
where
Du
is the distance
of ith
conductor
from
7th
conductor
carrying
current
1r.
From
F,q.
(2.27)
and
by
repeated
use
of
Eq.
(2.28),
rhe
rotal
flux
linkages
of conductor
i due
to
flux up
to
point
P are
,Di
ln
rl.
+...
+
In
The
above
equation
can
be reorganized
as
)i
=
2xro'[[r,
h+
+
hn[*.+
I,m]+..+
+
(/,
ln
But,
I,
-
-
(1,
+
Dr+Irh
Dz+...+
Iik
D, +..+
In^
O,)
Iz
+... +
In-).
Substiiuti'g
for
/n in
the
second
term
of Eq.
(2.29)and
simplifying,
we
have
f/
)i
=
2 *
to-tl
I
t,
ml
*
L,
h
L_.+...+1,
rn
a
'
L('
Dir'-""'Diz""''''^'rti
lnductanec anrl Flaaioranaa ^i T---^--!
.
f..
, rsrqrrug
ur
I rallsmlssfon
Llnes k,...i<Er
-
I
---
a /1 lA\t^cr
I
u,
Applying
Eq. (2-30)
ro
filamenr
i of
conductor
A,
weobtain
its
flux
tintages
zxfir
!
h+a6J-a...*rnl*...*rn
I
,(
-2xto-7
4lt"]-*
6-J-1...*ln
t
)
^,
\
Dir,
^^'
Diz,
|
"
'
'
'r
D,^,
)
=
2
x
lo-716
(P,r:D,r,...D,t
Wb_T/m
(D,rD,r.
.
.
D,,.
.
.
D,n)r'
"
The
inductance-of
filament
f
is
then
Li=]-
!
:
2nx70-t,n
(Dn'
"'
D,,'
"'
D,^')1/^'
* I,ln
=e+.
..*li
ln
at-
...
*
I,-r1n
Dr-t
1)
'4))
In
order
to
account
for
total
flux
linkages
of
conductor
i,
let
the point
p
now
recede
to
infinity.
The
terms
such
as
ln
D1/Dn,
etc.
approach
ln
t
=
o.
Also
for
the
sake
of
symmetry,
denoting
,.{^
D,,,-
wi
have
li=
z x
1or
I
trn**
rr','!-+1,
ln
-1
U
Dt
'
D,2
-"
---
Dii
+...+I^t"
+)
wb-r/m
(D,rD,r...D,,
.D
'*
FVm
(2.3r)
7/n
(2.30)
2.7
INDUCTANCE
OF
COMPOSITE
CONDUCTOR
LINES
We
are
now
ready
to
study
the
inductance
of transmission
lines
composed
of
composite
conductors.
Future
2.6
shows
such
a
single-phase
line
comprising
composite
conductors
A
and
B
with
A
having
n
paraliel
filaments
and
B
having
mt
parallel
filaments.
Though
the
inductance
of each
filament
will
be
somewhat
different
(their
resistances
will
be
equal
if
conductor
diameters
are
chosen
to
be
unttorm),
it is
sufficiently
accurate
fo
assum.e
that
the
current
is
equally
divided
among
the
filaments
of
each
composite
conductor.
Thus,
each
filament
of
A is
taken
to
carry
a
current
I/n,
while
each
filament
of
conductor
B carries
the
return
current
of
-
Ihnt.
o
Composite
conductor
A
Fig.
2.6
single-phase
line
consisting
of
two
composite
conductors
The
average
inductance
of
the
filaments
of
composite
conductor
A
is
Luu,=
Lt+L2+4+"'+Ln
Since
conductor
a
is
co#posed
of
n
filaments
electrically
in parallel,
its
inductance
is
, _Lu,,
_
\+
12+...+L,
_A
=-
*ru"rorr:rr"rh"
.*pr"f,rion
for
rir"#i"t
indu*ance
from
Eq. (2.31)i"
E;.
3'::r',
Le=2
x
10-7
ln
l(Dn,
...
Dr
j,
...Dr.,)...(D^,
...
prj,
...D,^,)...
(Dnr,
..
. Dnj,
...
Dr^,17r/ntn
[(Dn...
Du
...
Drn)...(Dit..n,t.
4.
Htm (2.33)
(Dnt...
Dni
...
D
rn)]r,n'
11? x-.::ir:l :f
,he,arsumenr
of
the
logarithm
in
Eq. (2.33)
is
rhe
m,nth
t:?,T,:!"^:
u.]-tl-*'of
conductor
Ati
m'
iri"L.il
"il"#il#:ili:ffi;
l:#1:# *:
jr:lr*
ry
n2.th
int
of
nz
p,;d;;;._,i;
ff
ffiilil:;
yflr,""1i)
T:l
set
of
n
product
term
pertains
to
"
fii";;;;rrj"",i,sts
of
I!:::::1li:*Ti:r{.
rnl
denominaror
is
defin"o
u,
;;;w;;:;;:;,1;::;
!:{Kr,"-li:t:t:y?
of
conduc
tor
A,
and
,s
auureviate;
;,
"J
"r';#;;;":::"*
o
o
2
o
2l
I
r)
m
GMD
is
also
called
geometric
mean
radius
(GMR).
lf
In
terms
of
the
above
symbols,
we
can
write
Eq (2.33)
as
56
,1
todern Fower
System Analysis
I
Lt=2x
10-7h
+-
Iilm
Dr.t
(2.34a)
'^
^Hn^
Note the
similarity of the
above relation with Eq.
(2.22b),
which
gives
the
inductance
of one conductor
of a single-phase
line
for the special
case of two
solid, round conductors. In
Eq.
(2.22b)
r\ is
the
self
GMD of a single
conductor and
D is the mutual
GMD of two single conductors.
The
inductance of the
composite conductor B
is
determined in
a similar
manner, and the
total
inductance
of the line
is
L= Le+
Ln
(2.3s)
A conductor is composed
of seven identical copper strands, each having
a
radius
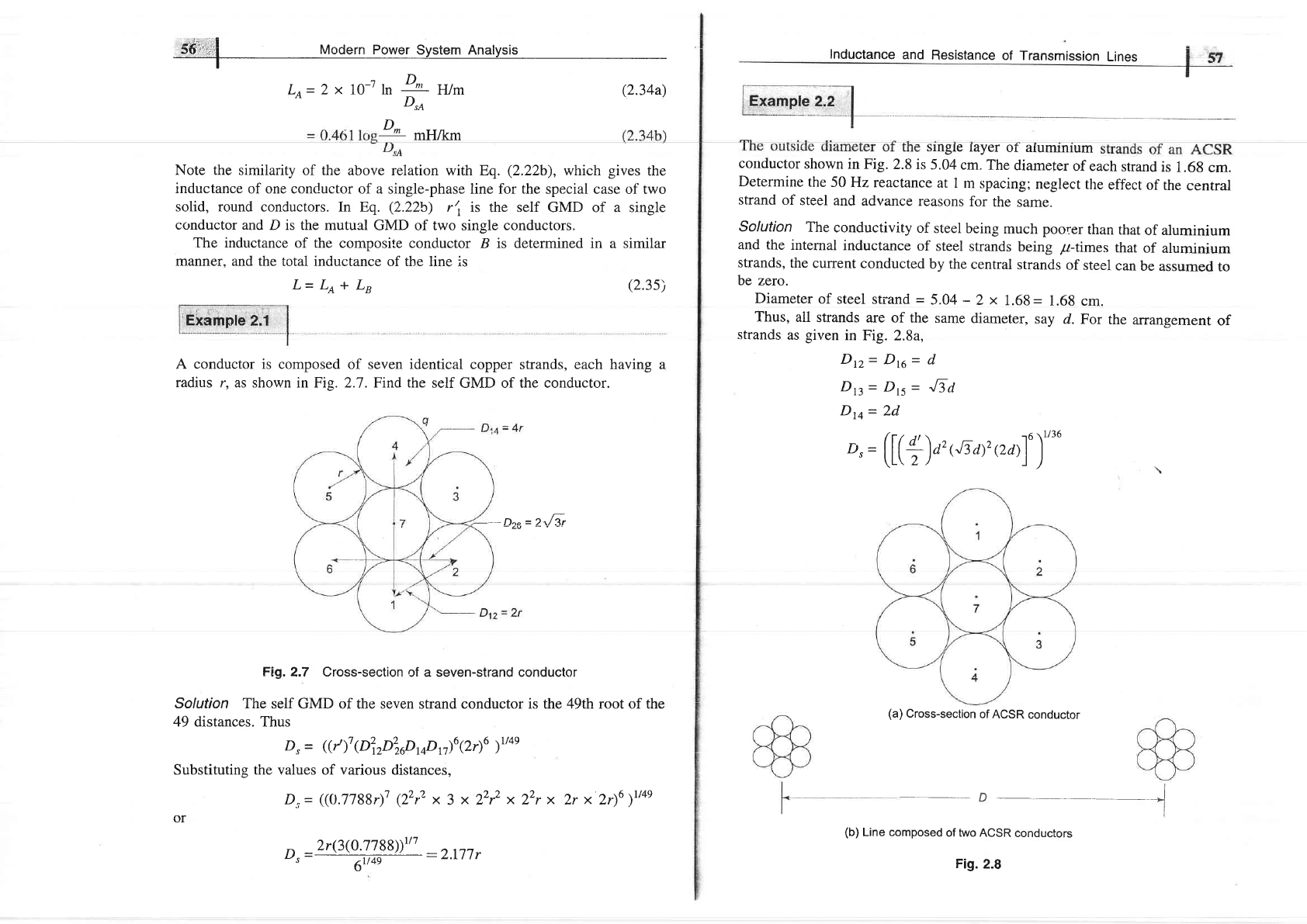
r, as shown in Fig. 2.7. Frnd the
self GMD of the conductor.
--
D^^
=
2..fir
Fig.2.7 Cross-section
of a seven-strand conductor
Solution The
self GMD of the seven
strand
conductor is the 49th root of the
49
distances. Thus
D,
=
(V)7 (D1zD'ruD
rp r)u
(zr)u
)t'on
Substituting
the
values of
various
distances,
p..-
((tJ.7788r)7
(2212
x
3
x
22f
x
22rx 2r
x
2r)u)t'o'
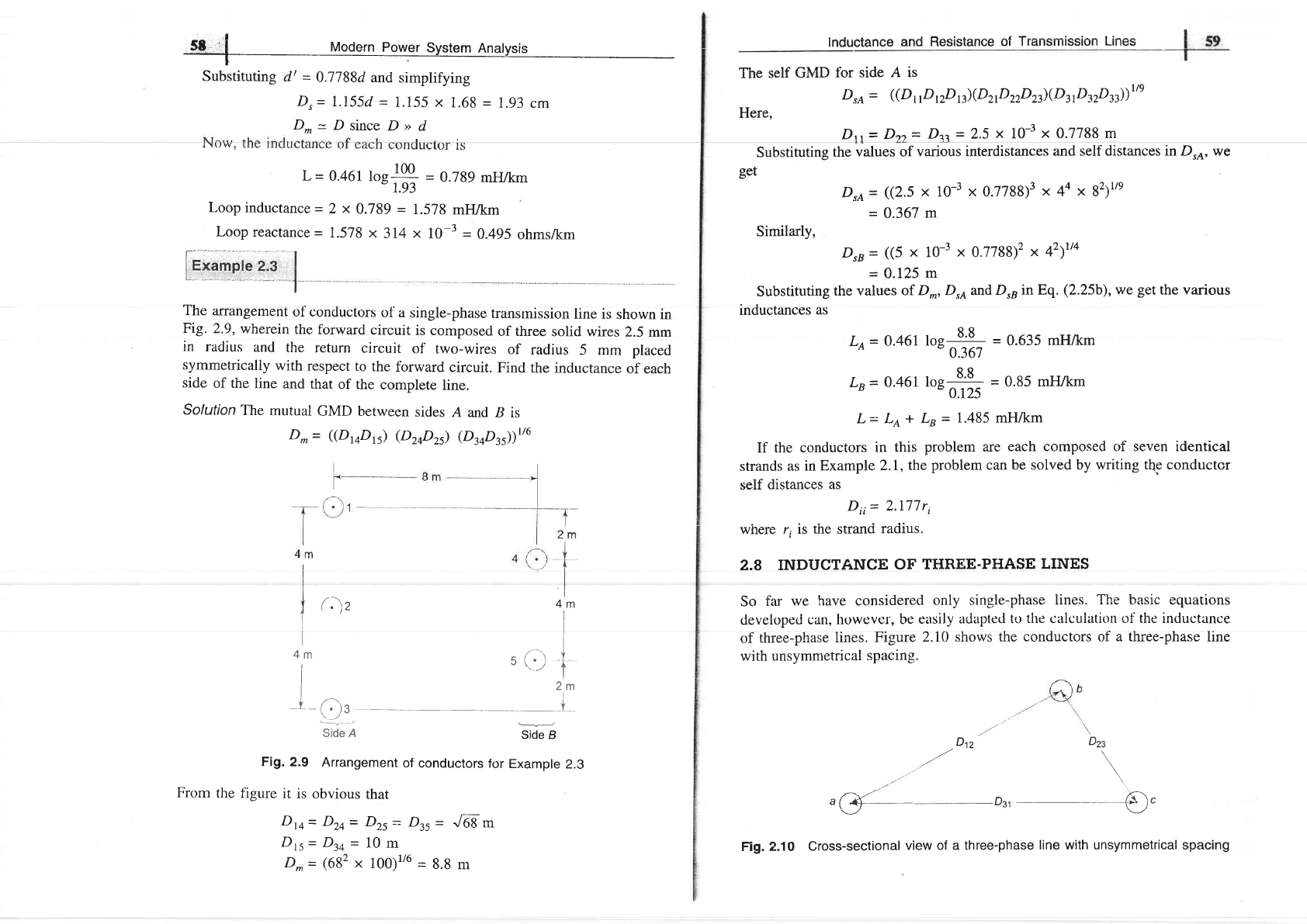
srngre
layer
oI alurrunlum
conductor
shown
in Fig.
2.8 is
5.04
cm. The
diameter
of each
strand
is
1.6g
cm.
Determine
the
50
Hz reactance
at I rn
spacing;
neglect
the
effect
of
the
central
strand
of
steel
and advance
reasons
for
the
same.
Solution
The
conductivity
of
steel
being
much poorer
than
that
of aluminium
and the
internal
inductance
of
steel
strands
being
p-times
that
of
aluminium
strands,
the
current
conducted
by the
central
strands
of
steel
can
be
assumed
to
be
zero.
Diameter
of
steel strand
=
5.04
-2
x
1.68=
1.68
cm.
Thus,
all
strands
are of
the same
diameter,
say
d.
For
the
arrangement
of
strands
as
given
in
Fig. 2.8a,
Dtz=
Drc=
d
Drt=
Dn= Jld
Du=
2d
Dr=
(l(+)-
dTil,eilf')
(b)
Line composed
of two ACSR
conductors
Inductance
and
Resistance
of Transmission
Lines
(a)
Cross-section
of
ACSR
conductor
,
_2r(3(0.7788))tt7
_
2.t77 rus
-
6U+e
Fig.
2.8
I
5t
I
Modern
Power
Svstem
Analvsis
Substituting
d'
=
0.7188d
and
simplifying
D,=
l.l55d
=
1.155 x
1.68
=
1.93 cm
D^=
Dsince
D>>
d
L=
0.46t
toe
1P
-
0.789
mH/km
"
1.93
Loop
inductance
-
2 x
0.789
=
1.578
mHlkm
Loop
reactance
=
1.578
x
314
x
10-3
-
0.495
ohms/lcm
;;;,;,;
I
lnductance
and Resistance
oi
Transmission
Lines
The self
GMD
fbr side
A is
D,A
=
((D
nD nD n)(DztDzzDn)(D3tDtrDtr))''e
Here.
D,,
=
Doc
=
Dat
=
2.5
x
10-3
x
0.7788
m
Substituting
the
values of
various interdistances
and self distances in D16, we
get
D,A=
(2.5
x
10-3
x
0.778U3
x
4a
x
8\tte
=
0.367
m
D,B=
((5
x
10-3
x
0.778q2
,4')t'o
=
0.125
m
Substituting
the
values of
D^, D6 and
Dr,
in
Eq.
(2.25b),
we
get
the various
inductances
as
L^
=
0.461
los
8'8
=
0.635
mHlkm
^
"
0.367
L.
=
0.461
toe
8'8
=
0.85
mH/<m
D
-
0J25
L
=
Lt
+ Ln
=
1.485 mH/km
If the
conductors
in
this
problem
are each
composed of seven identical
strands
as in Example
2.1, the
problem
can
be solved by
writing
t[e
conductcr
self
distances
as
Dii=
2'177rt
where
r, is the stranci
raciius.
2.8
INDUCTANCE
OF
THREE.PHASE
LINES
So
far
we
have considered
only single-phase
lines.
The
basic equations
developed
can, however,
be
easily
adapled to
the calculation of the inductance
of
three-phase
lines.
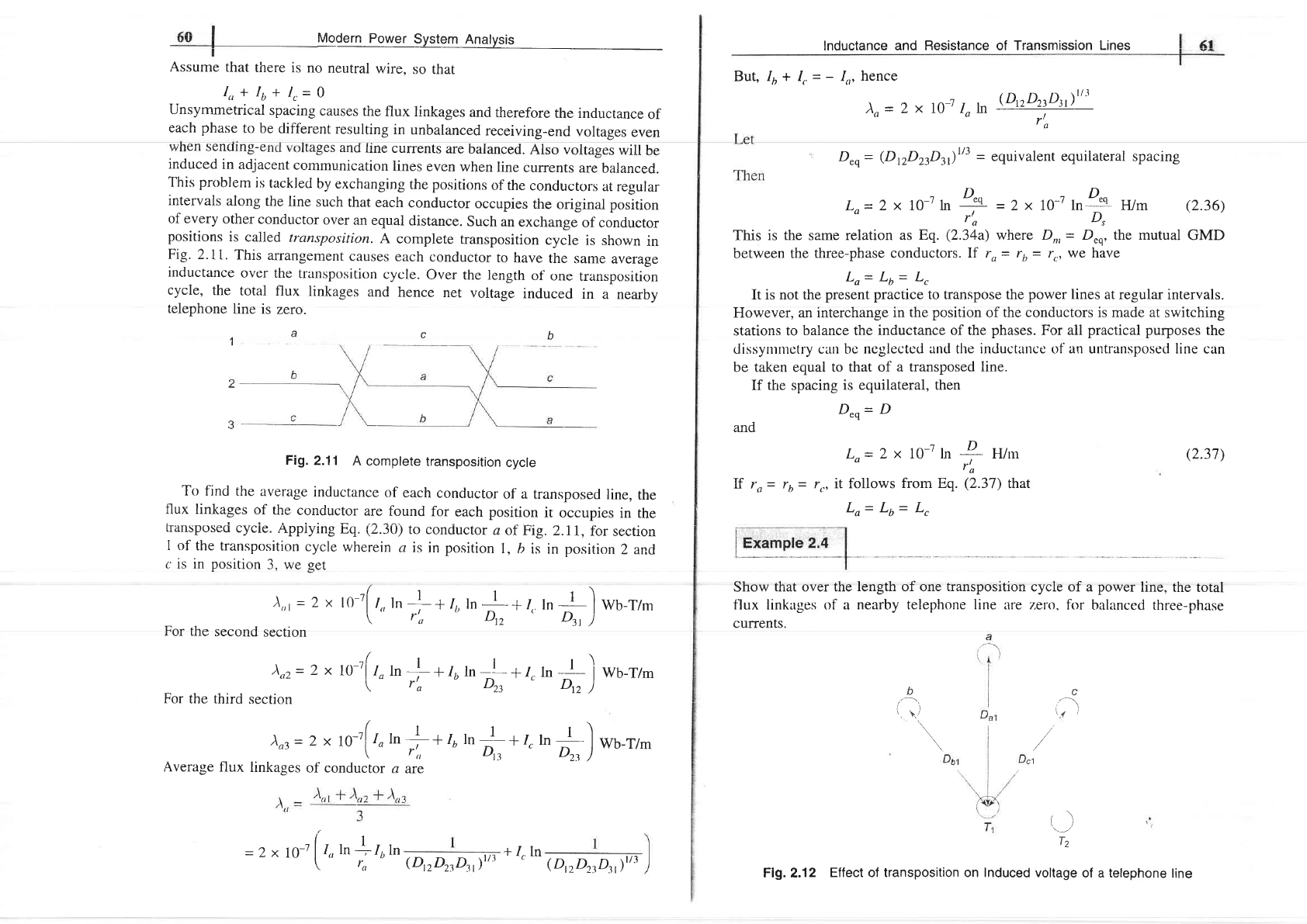
Figure
2.10
shows the
conductors of a three-phase
line
with
unsymmetrical
spacing.
Similarly,
The
arrangement
of conductors
of a
single-phase
transmission
line
is
shown in
Fig.2.9,
wherein
the
forward
circuit
is composed
of three
solid wires
2.5
mm
in
radius
and the
return
circuit
of
two-wires
of
radius
5 mm placed
symmetrically
with
respect
to
the
forward
circuit.
Find
the
inductance
of each
side
of the
line
and
that
of the
complete
line.
Solution
The
mutual
GMD between
sides
A and
B is
D.=
((DMD$)
(Dz+Dz)
1D3aD3))tt6
()z
4m
li
4m
s(tl I
I
"'"'t
Side
A
Side
B
Fig.
2.9
Arrangement
of conductors
for
Example
2.3
From
the figure
it is
obvious
that
Dtq=
Dzq=
Dzs-
D.u=
JOa
m
Drs=
Dy
=
10
m
D^=
(682
x
100)l/6
=
8.8
m
Dzs
Dn
Fig. 2.10
Cross-sectional
view of
a three-phase
line with unsymmetrical spacing
Ugdern
Power
System
A
Assume
that
there
is
no neutral
wire,
so that
Ir,+
Ir+
Ir-0
Unsymmetrical
spacing
causes
the
flux
linkages
and
therefore
the inductance
of
each
phase
to be
different
resulting
in
unbalanced
receiving-end
voltages
even
en
senolng-e
tages
and
llne currents
are
balanced.
AIso
voltages
will
be
induced
in
adjacent
communication
lines
even
when line
currents
are
balanced.
This
problem
is
tackled
by
exchanging
the positions
of the
conductors
at
regular
intervais
aiong
the
line
such
that
each
conductor
occupies
the
original position
of every
other
conductor
over
an
equal
distance.
Such an
exchange
of conductor
positions
is called
transposition.
A complete
transposition
cycle
is
shown
in
Fig.2.11.
This
alrangement
causes
each
conductor
to
have
the
same
average
inductance
over the
transposition
cycle.
Over
the length
of
one
transposition
cycle,
the
total
flux
linkages
and
hence
net
voltage
induced
in
a nearby
telephone
line
is zero.
b
1
lnductance
and Resistance of Transmission
Lines
But,
1, I
I,
=
-
/n,
hence
Ao=
2
x
10-7 Iok
(D"D'trDt')'''
r'a
D"o
(DtzDnDrr)t''
-
equivalent equilateral spacing
Lo=
2x t0-7 h
+
=
2
x
fO-? fn{:r FVm
f'oD,
This
is the
same
relation as
Eq.
(2.34a)
where Dn,
=
D"o, the mutual
GMD
between the
three-phase conductors.
lf ro
=
11,
=
r6t we have
Lo= LO= L,
It is not the
present
practice
to transpose the
power
lines
at regular intervals.
However, an interchange
in
the
position
of
the conductors is made at
switching
stations to
balance the inductance
of the
phases.
For all
practical purposes
the
dissynrnrctry can bc
neglectcd and the
inductancc
of an untransposecl line can
be taken equal
to that of a transposed
line.
If ttre spacing
is equilateral, then
D"o=
D
and
Lo=2x
l0-7m
I
fmn
ra
If ro
=
11,
=
r,-, it follows
from F,q.
(2.37)
that
Lo= Ltr= L,
Fig.
2.11
A
complete
transposition
cycle
To find
the
average
inductance
of each
conductor
of a
transposed
line,
the
flux linkages
of
the
conductor
are
found
for
each position
it
occupies
in
the
iransposeci
cycie.
appiying
Eq.
(2.s0)
to conciuctor
a
of Fig.
z.lI,
for
section
1 of
the
transposition
cycle
wherein
a is
in position
1,
b is
in
position
2 and
c is in
position
3, we get
)ur
=
2
x
to-rf
,,,r"
*
I
I,,m]-*l
In
-1
j*o-rr,r,
\"
''r,
"
Dr,
(
Dt,)
For the
second
section
-( | |
\
For
the tr,ira
,".* :=
'x
to-?[r"
k
+
*
16 tn
b:
.
'' t"
],;i
wu
v-
)o3
=
z x
ro-71
r"
h
!t
16 tn*+
r.,n
l-l
*o-rr-
\
f',,
"
D,
uzr
/
Average
flux
linkages
of conductor
a
are
(2.36)
(2.37)
Show
that over the
length
of
one transposition
cycle of a
power
line,
the total
flux linkages of a
nearby telephone line
are
z,ero,
ftrr
balanced three-phase
currents.
b
(l)
c
i'-)
\7
c'l
rctlI,,h!r,,tn
\4,
()
T2
-1u
(DnDrDlt)t/3
+1.ln
(Dt2D2.)3t)t/3
F19.2.12
Effect of transposition on Induced voltage
of a telephone
line
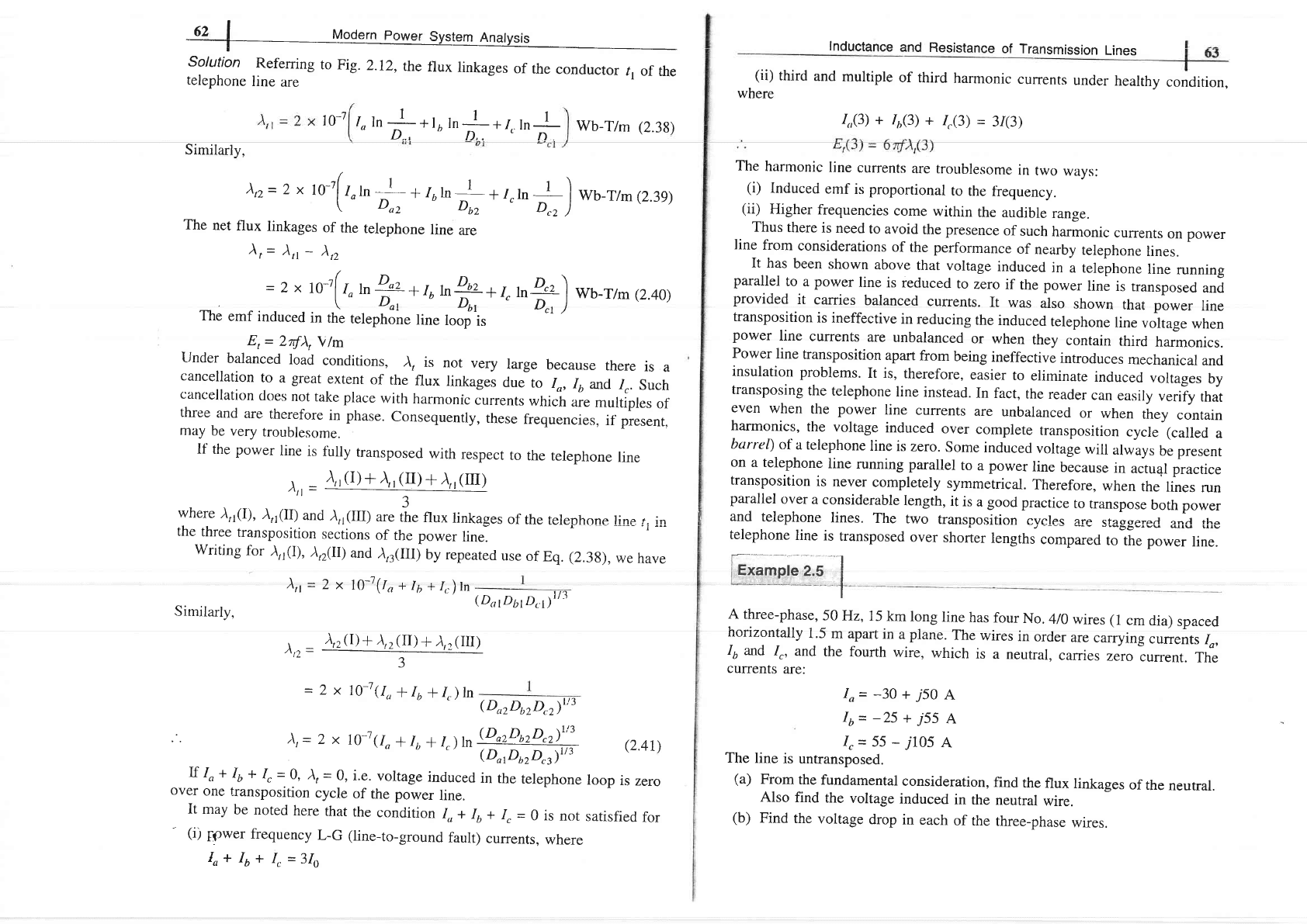
)t2=2xtotl
,"Ln
5
*r6tn+*1.1n+lwb_rim
(2.3s)
(
"
Doz
-o--
Du,
D,,
)
"v
''t
The
net
flux
linkages
of
the
telephone
line
are
),=
),t-
)tz
=
2 x
to-r(
t"
h
D:,
*
16
tn?
*r"
,"+)
wb-rim
(2.40)
rhe
emf
induced
,"
,n!
,.,"p'#ir"
t*
"3Jti,
"
-
D"
)
E,=
Zrf),\lm
fjnder
balanced
load
conditions,
),
is
not
very
large
because
there
is
a
cancellation
to
a great
extent
of
the
flux
linkages
due
to
Io,
16
and
1r.
Such
cancellation
does
not
take
place
with
harmonr.
"u.r.nts
which
are
multiples
of
three
and
are
therefore
in phase.
Consequently,
these
frequencies,
if present.
may
be
very
troublesome.
lf
the power
line
is
f'ully
transposed
with
respect
to
the
telephone
line
),,
=
)"
(I)
*
4,
(tr)
*
),,(III)
3
where
),r(I),
)/r(II)
ancl
),,(III)
are
the
flux
linkages
of
the
telephone
line
r,
in
the
three
transposition
sections
of
the
power
line.
Writing
for
),,(l),
,\/2(II)
and
),r([l)
by
repeared
use
of
Eq.
(2.3g),
we
have
A,t=
2 x
l0-/(tn+
16+
1,,)ln
(DntDutD,.itt3
Similarly,
=2x104(1,+.Ib+1,)ln
(Dn2Db2D,,r)t,,
)r=
2 x
10-711,
+
It,
+
I,)ln(D"2Db2D,)rt:
'
(DorDarD,r)r,,
If
Io
+
Iu
+
I,
=
0,
),
=
0, i.e.
voltage
induced
in
the
telephone
loop
is
zero
over
one
transposition
cycle
of
the
power
line.
It
may
be
noted
here
that
the
condition
Iu+
Iu+
I,
=
0 is
not
satisfied
for
-
(i)
rpwer
frequency
L-G
(line-to-ground
fault)
currents.
where
Io+
Iu*
Ir=J[o
62
|
Modern
Power
Svstem Anatrrqic.
solution
Referring
to
Fig.
2.r2,
the
flux
linkages
of
the
conductor
r,
of
the
telephone
line
are
,"^*+1,
ln
*.1
t"*
Wb-T/m
(2.38)
Similarly,
(2.4r)
lnductance
and
Resistance
of Transmission
Lines
(ii)
third
and
multiple
of
third
harmonic
currenrs
under
healthy
.ondirion,
where
1,,(3)+
IoQ)+
I,(3)=
31(3)
The
harmonic
line
currents
are troublesome
in
two
wavs:
(i)
Induced
ernf
is proportional
to
the
frequency.
(ii)
Higher
frequencies
come
within
the
audible
range.
Thus
there
is
need
to
avoid
the
presence
of
such
harmonic
currents
on
power
Iine
from
considerations
of the performance
of
nearby
telephone
lines.
It has
been
shown
above
that
voltage
induced
in
a telephone
line
running
parallel
to
a
power
line
is
reduced
to
zero
if
the power
line
is
transposed
and
provided
it
carries
balanced
currents.
It
was
also
shown
that
ptwer
line
transposition
is ineffective
in
reducing
the
induced
telephone
line
uoitug.
when
power
line
currents
are
unbalanced
or when
they
contain
third
harmonics.
Power
line
transposition
aparrfrom
being
ineffective
introduces
mechanical
and
insulation
problems.
It is,
therefore,
easier
to
eliminate
induced
voltages
by
transposing
the
telephone
line
instead.
In
fact,
the
reader
can
easily
verify
that
even
when
the power
line
currents
are
unbalanced
or when
t-h"y
cgntain
harmonics,
the
voltage
induced
over
complete
transposition
cycle (called
a
barrel)
of a telephone
line
is
zero.
Some
induced
voltage
will
always
be
present
on
a telephone
line
running
parallel
to
a
power
line
because
in
actu4l
practice
transposition
is
never
completely
symmetrical.
Therefore,
when
the
lines
run
parallel
over
a
considerable
length,
it
is
a
good
practice
to
transpose
both
power
and
telephone
lines.
The
two
transposition
cyeles ^re
staggered.
and
the
telephone
line
is
transposed
over
shorter
lengths
compared
to
the power
line.
rc-7
i,."rp,"
i
u
I
-f
A three-phase,
50 Hz,15
km
long
line
has
four
No.
4/0
wires
(1
cm
dia)
spaced
horizontally
1.5
m
apart
in
a
plane.
The
wires
in
order
are
carrying
currents
1o,
Iu
and
I,
and
the
fourth
wire,
which
is
a neutral,
carries
,".o
iu.."nt.
The
currents
are:
Io=
-30
+
750
A
Iu=
-25
+
j55
A
I"=
55
-
j105
A
The
line
is untransposed.
(a)
From
the
fundamental
consideration,
find
the
flux
linkages
of
the
neutral.
Also
find
the
voltage
induced
in
the
neutral
wire.
(b)
Find
the
voltage
drop
in
each
of
the
three-phase
wires.
