Kothari D.P., Nagrath I.J. Modern Power Systems Analysis

.2Oftril

Modern

Power

System

Analysis

I

eA

=

e+t

=

+

-

-1-

.o,

(6r

-

64)

=

0.04

pu

*+'

0.1

Reactive

power flows

on

other

lines

can

be

similarly

calculated.

Generations

and load

demands

at

all the

buses

and

all

the

line

flows

are

6.5

GAUSS.SEIDEL

METHOD

The

Gauss-Seidel

(GS)

method

is

an iterative

algorithm

for

solving

a set

of

non-linear

algebraic

equations.

To start

with,

a solution

vector

is

assumed,

based

on

guidance from

practical experience

in

a

physical situation.

One

of the

equations

is then

used

to obtain

the

revised

value of

a

particular

variable

by

substituting

in it

the

present

values

of the

remaining

variables.

The

solution

vector is

immecliately

updatecl

in respect

of

this

variable.

The

process

is then

repeated

for

all

the variables

thereby

completing

one

iteration.

The

iterative

process

is then

repeated

till the

solution

vector

converges

within

prescribed

accuracy.

The

convergence

is

quite sensitive

to

the starting

values

assumed'

Fortunately,

in a

load

flow

study

a

starting

vector

close

to the final

solution

can

be

easily

identified

with

previous experience.

To

explain

how

the

GS

method

is

applied

to

obtain

the

load

flow

solution,

let it be

assumed

that

all

buses

other

than

the slack

bus

are PB

buses.

We shall

see

later that

the

method

can be

easily

adopted

to

include

PV

buses

as well.

The

slack

bus

voltage being

specified,

there

are

(n

-

1) bus

voltages

starting

values

of

whose

magnitudes

and

angles

are

assumed.

These

values

are

then

updated

through

an iterative

process. During

the

course

of

any

iteration,

the

revised

voltage

at the

ith

bus

is obtained

as follows:

I en*.

carried

out at the

end of a complete iteration,

the

process

is

known as

the

Gauss

iterative

method.

It is much slower

to converge

and

may

sometimes

fail

to do

so.

Algorithm

for Load Flow

Solution

Presently

we

shall continue

to consider

the case where

all

buses

other

than

the

slack are

PQ buses. The

steps of a computational

algorithm'are

given

below:

1. With the

load

profile

known

at

each

bus

(i.e.

P^

and

0p;

known),

allocate*

Po, and.

Q5;

to all

generating

stations.

While

active and

reactive

generations

are allocated

to

the slack

bus, these

are

permitted

to

vary

during iterative

computation.

This

is necessary

as

voltage

magnitude and

angle are specified

at this bus

(only

two variables

can

be specified at any

bus).

With this step,

bus iniections

(P,

+

jQ)

are known

at

all buses

other

than

the slack

bus.

2. Assembly

of bus' admittance

matrix

rsus: with

the line

and

shunt

admittance data stored in

the computer,

Yru,

is

assembled

by

using

the

rule for self

and

mutual admittances

(Sec.

6.2).

Alternatively

yru,

is

assembled using Eq.

(6.23)

where

input

data are

in the

form

of

primitive

matrix Y

and singular

connection

matrix

A.

3.

Iterative

computation

of bus voltages

(V;;

L

=

2,

3,..., n):

To

start

the

iterations a

set of initial

voltage

values

is assumed.

Since, in

a

power

system

the

voltage

spread

is not

too wide.

it is

normal practice

ro

use a

flat

voltage

start,** i... initialiy

ali voltages

are

set equal

to

(r

+

70)

except

the

voltage

of the

slack bus which

is fixed.

It should

be

noted

that

(n

-

l) equations

(6.41)

in cornplex

numbers

are

to

be solved

iteratively

for findin1

@

-

1) complex voltages

V2,

V3, ...,

V,. If

complex

number

operations

zlre Dot available

in a

computer.

Eqs

(6.41)

can

be converted

rnto 2(n

-

1) equations in real

unknowns

(ei,

fr

or

lV,l,

5)

by

writing

Vr

=

€i +

ifi

=

lV,l ei6'

(6.42)

A significant

reduction in the

computer

time

can

be achieved

by

performing

in advance

all the arithmetic

operations

that

do

not

change

with

the iterations.

Define

i

=

2,3,

...,

ft (6.43)

'Active

and reactive

generation

allocations

are made on

econorfc

considerations

discussed in Chapter 7.

.*A

flat

voltage

start means that to start the

iteration set the

voltage

magnitudes

and

angles

at all buses other than the PV

buses equal to

(i

+

l0).

The slack

bus

angle is

conveniently

taken as zero. The

voltage

magnitudes

at

the PV buses

and

slack

bus

are

set equal to the specified values.

J,

=

(P,

-

jQ)lvi

[from

Eq.

(6.25a)]

From

Eq.

(6.5)

,[

I

v,=*lL,_

fv,ovol

,,'

L

T:i

I

Substituting

fbr

J, from

Eq.

(6.39)

into

(6.40)

t^

.

,

-l

v,

=

*l

'':jq

-

t

Yit' vr'

l

"

=

2' 3""'

n

Y"

I

vr*

ilt

I

k*t

I

(6.3e)

(6.40)

(6.4r)

The

voltages substituted

in the

right

hand

side

of

Eq.

(6.41)

are the

most

recently

calcuiated

(updated)

values

for

the

corresponding

buses.

During

each

iteration

voltages

at buses

i

=

2,3,

..., n

are sequentially

updated

through

use

of

Eq.

(6.41).

Vr,

the slack

bus

voltage

being

fixed

is not

required

to be

updated.

Iterations

are repeated

till

no

bus

voltage

magnitude

changes

by

more

than

a

prescribed

value

during

an

iteration.

The

computation

process

is then

said

to converge

to

a solution.

206

|

Modern

power

Svstem

Anatvsis

(6.44)

I*rl.d

flows

on

the

lines

terminating

at

the

slack

bus.

Acceleration

of

convergence

acceleration

factor.

For

the

tth

(r

+

l)th

iteration

is given

by

up

by

the

use

of

the

bus,

the

accelerated

value

of

voltage

at

the

y(r+r)

(accelerated)

=

V,Q'*

a(v.G+r)

-

V,Ql,

where

a is

a real

number

called

the

acceleration

factor.

A suitable

value

of

a

for

any

system

can

be

obtained

by

trial

load

flow

sfudies.

A generally

recommended

value

is

a

=

1.6.

A

wrong

choice

of

o.

may

indeed

slow

down

convergence

or

even

cause

the

method

to

diverge.

This

concludes

the

load

flow

analysis

for

thJ

case

of

pe

buses

onry.

Algorithm

Modification

when

pv

Buses

are

arso

present

At

the

PVbuses,

p

andrv]r

are

specified

and

e

ancr

dare

the

unknowns

to

be

detcnnincd.'l'hererirre,

the values

of

e

and

d

are

to

be

updated

in

every

GS

iteration

through

appropriate

bus

equations.

This

is

accomplished

in

the

following

steps

for

the

ith

pV

bus.

l.

From

Eq.

(6.26b)

f")

ei

=

-

Im

j

yr*

D,

y,ovof

L

ft:l

)

The

revised

value

of

ei

is

obtained

from

the

above

equation

by

substituting

most

updated

values

of

voltages

on

rhe

right

hand

side.

In

fact,

for

the

(.r

+

1)th

iteration

one

can

write

from

the

above

equation

I

g.(r+t)

=

-Ln

],r,',)

-

i

y,rv,,(,*t)

a

1y.r,1-

D

y,kvk,,,I

,u.ro,

I

Lr,

f!,

"

)

2'

The

revised

value

of

{.is

obtained

from

Eq. (6.45)

immediately

following

step

1.

Thus

6Q+r)

_

ay!+r)

=

Ansle

"t

fei]l-

-

i

Bovo(,+r)

-

D

B,ovo,,,l

(6.s1)

L

(t1''')*

,r:

r

k:,+r

J

where

.

^(r+t)-

P-i?t'rtt

r1.;

.=__

,u

_

6.52)

The

algorithm

for

pe

buses

remains

unchanged.

17(r+l) -

Ai

'S

- rt(,

n

vi'

-tB,ovo,,*t,-

f

r,ovl,,

i=2,3,...,n

(Vl")

ilt

k=irl

(6.4s)

The iterative

process

is continued

till the

change

in

magnitude

of

bus

voltage,

lav.('*r)|,

between

two

consecutive

iterations

is less

than

a

certain tolerance

for

all

bus

voltages,

i.e.

IAV.G*r)l

_

1y.t+r)

_

V,e)l <

6,

;

i

=

2,

3, ...,

n

4.

5.

computation

of slack

bus power:

substitution

of

all

bus

voltages

computed

in

step 3

along

with

V, in Eq.

(6.25b)

yields

Sf

=

pr-

jey

computtttion

ofline

.flows:

This

is thc

last

step in

thc

loacl

flow analysis

wherein

the

power

flows

on

the various

lines

of the

network

are

computed.

Consider

the

line

connecting

buses

i and k.

The line

and

transformers

at

each

end can

be represented

by a circuit

with

series

admittance y*

and

two

shunt

admittances

1l;ro

and

)no

as

shown

in

Fig.

6.8.

Bus i

Bus

k

(6.46)

(6.47)

(6.48)

(6.4e)

l*io

sm

Fig.

6.8 7i'-representation

of

a

line

and transformers

connected

between

two

buses

The current

fed

by

bus i

into

the line

can

be

expressed

as

Iit

=

Iitt +

Iirc

=

(Vi

-

V)

!it,+

V,y,oo

The

power

fed

into

the line

from

bus

i is.

S*

=

Pir*

jQiF

Vi

lfr=

Vi(Vf

-

Vr\

yft+

V!,*y,f,

Similarly,

the

power

fed into

the

line

from

bus

k is

Sri

=

Vk

(V*k-

Vf) yfo+

VoVfyi,l,

The

power

loss

in

the

(t

-

t)th

line

is the

sum

of

the

power

flows

determined

frorn

Eqs.

(6.48)

and

(6.49).

Total

transmission

loss

can

be

computed

by

sununing

all

the line

llows

(i.e.

5';a +

Sri fbr

all i,

/<).

7

Voltage

magnitude

limits

I

Vr

I

min

and

I

Vi

I

max'lor

'-(J

o

b

n"u"iiu"

pow"t

limits Qi

min

and Q;

max

for

PV buses

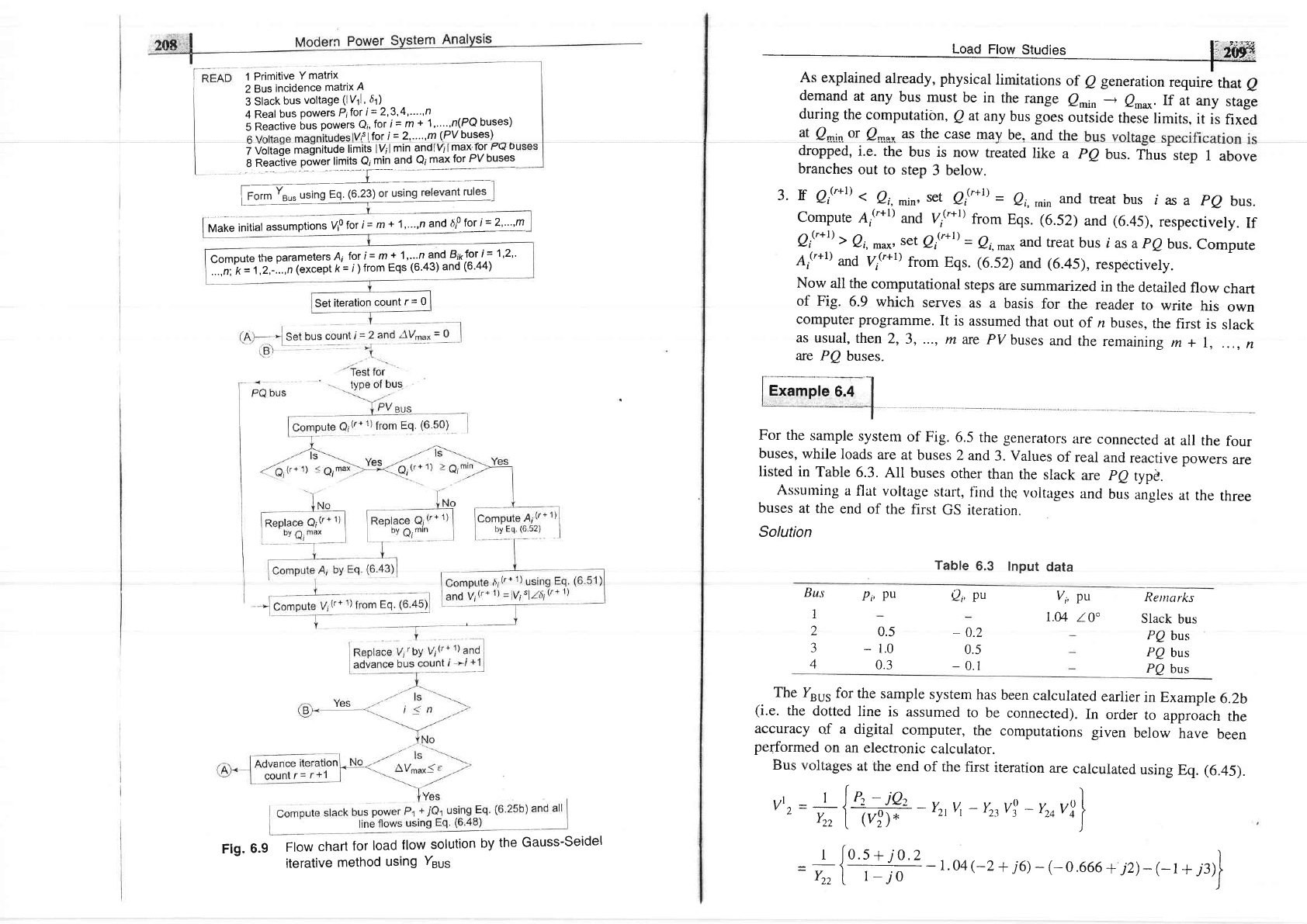
Fig.6.9F|owchartforloadflowso|utionbytheGauss.Seide|

iterative

method

using

YBUS

l'fiffi

demand

at

any

bus

must

be

in

the

range

e^rn

-

e^u*.If

ai

any

stage

during

the

computatibn,

Q

at

any

bus goes

outside

ttreJe

timits,

it

is

fixed

At

Q^in

or

Q^o,

as

the case

may

be.

and

the

dropped,

i.e.

the

bus is

now

treated

like

a

pe

bus.

Thus

step

1

above

branches

out

to step

3 below.

3. ff

9.('+r)

a

0;,6;n,

set

e,Q*r)

-

er,r^n

and

treat

bus

f as

a

pe

bus.

compute

4-(r+1)

and

y(r+t)

from

Eqs.

(6.52)

and

(6.45),

respectively.

If

Otu..:,')

Qt,

^

,

set

O-(r+l)

=

ei,^*and

treat

bus

i as

a

pebus.

Compure

4.Q+r)

-6

y(r+l)

from

Eqs.

(6.52)

and

(6.45),

respecrively.

Now

all the

computational

steps

are

summarized

in the

detailed

flow

chart

of Fig.

6.9

which

serves

as

a

basis

for

the

reader

to

write

his

own

computer programme.

It

is

assumed

that

out

of

n buses,

the

first is

slack

as

usual,

then2,3,

...,

m are

PVbuses

and

the

remaining

m +

l,

...,

ft

are

PQ

buses.

For the

sample

system

of

Fig.

6.5

the generators

are

connected

at

all the

four

buses,

while

loads

are

at

buses

2

and

3.

Values

of

real

and

reactive

powers

are

listed

in Table

6.3.

All

buses

other

than

the

slack

are

pe

typd.

Assuming

a

flat voltage

start,

f)nd

the voltages

and

bus

angles

at

the

three

buses

at the

end

of the

first

GS iteration.

Solution

Table

6.3

lnput

data

Bus

1 Primitive

Y

matrix

2

Bus

incidence

matrix

A

3

Slack

bus

voltage

(lY1l, 61)

4 Real

bus

powers

Pifor

i=

2,3'4,""'n

5

Reactive

bus

powers

Qi,

for

I

=

m

+

1"

-{fO

buses)

Vf I

for

i

=

2,....,m

(PV

buses)

I

e

6.4

Exampl

Retnarks

Slack

bus

PQ

bus

Pp

bus

Pp

bus

The l"ur

for

the

sample

system

has

been

calculated

earlier

in

Example

6.2b

(i.e.

the

dotted

line

is

assumed

to

be

connected).

In

order

to

approach

the

accuracy

of

a digital

computer,

the

computations

given

below

have

been

performed

on an

electronic

calculator.

T)--- ---r-- - , ,i

-Dus

voltages

at

rne

eno

or tne llrst

rteratron

are

calculated

using

Eq.

(6.a5).

I

2

J

4

Pp

PU

0.5

-

1.0

0.3

0u

pu

-

0.2

0.5

-

0.1

Vt'

Pu

r.u

10"

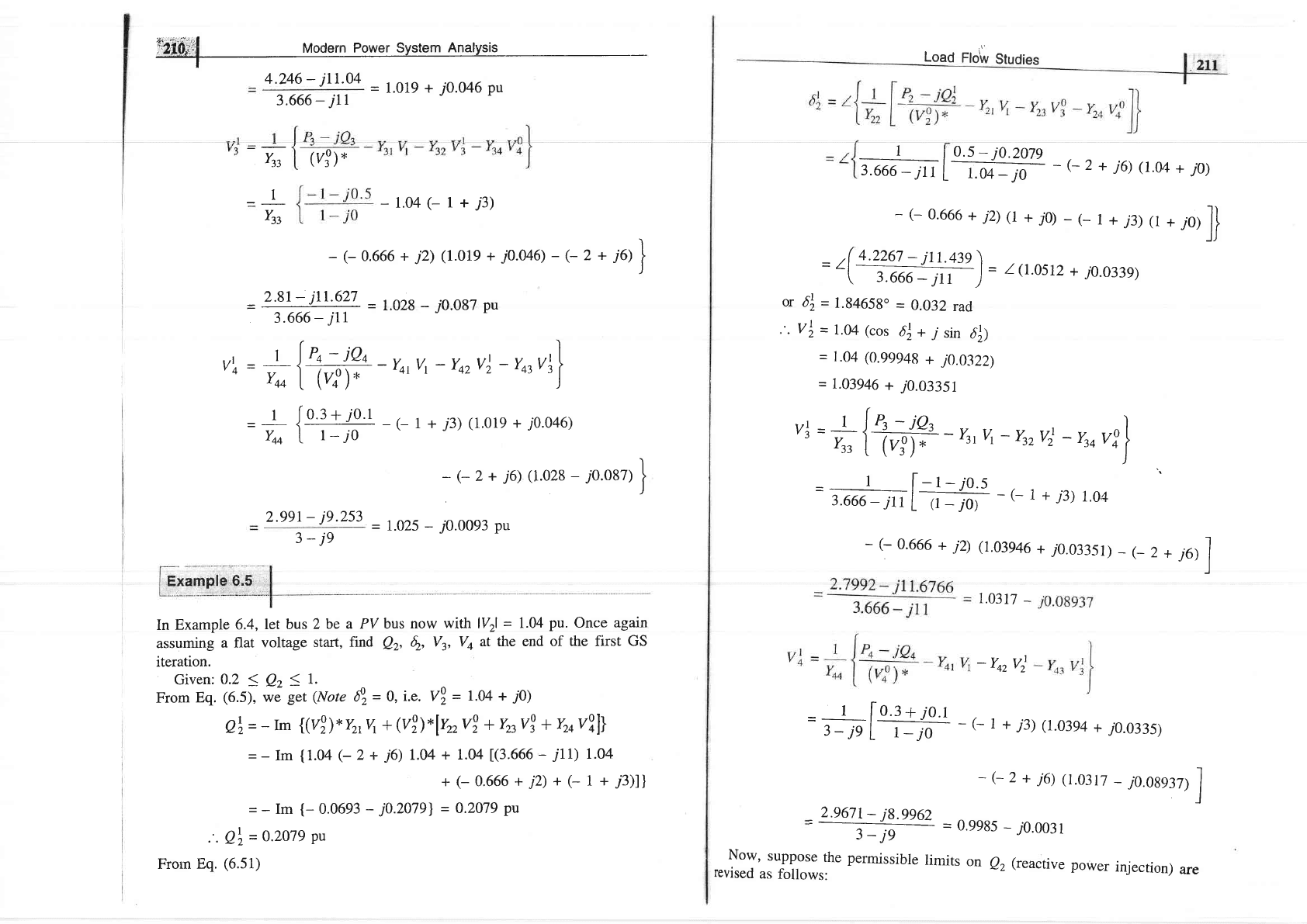
vtz=+{W-Yztvt-Y"v!

-

'r^'iI

tg.

Yr,.

r.i"g

Eq.

(6.2

Make

initial

assumptions

Vio

for

I

=

m

+

1,"',n

and

O;0

for

i

=

2!:'m

Compute

the

parameters

A;

for

i

=

^

t

1,"n

.and

B;p'for

i

=

1'2"

-.;;

i:

1

,2,-'...,n

(except

k

=

i

)

from

Eqs

(6'43) and

(6'44)

Set

iteration

count

r

=

0

l

10.5+i0.2

')

-

----

1-'-

r

-'-

-

1.04

(-z+

j6)-

(-0.666

+

j2)_(_t

+

fll

-

Yzz

I

t

-iO

-'-'\

-

'

r-/

\

v'vvv

'

r!

|

fei.fii,',1 Modern

Power System

Analysis

-

I

4.246

-

jrr.04

=

1.019

+

70.046

pu

3.666

-

jLr

(vl)

*

T#-frL+re

-

e

2

+

i6)

(to4

+

io)

-

(-

0.666

+

j2)

(t

+

70)

_

(_

1+

r)

j3)

(t.

io)

jl

=

t(

+'z^?e;t

-

itt'lzs

J

=

,

(r.rstz+

70.033e)

(

3.666_jtt

)-

or

612

=

1.84658o =

0.032

rad

.'.

v)

=

1.04

(cos

6)*

j

sin

dj)

=

1.04 (0.99948

+

j0.0322)

=

L03946

+

70.03351

,

(o

vl=-l-

j

'1-=iQt

-y.v.

-y_vt

_v ,,0I

'

t

=

1

114tr

-

Y"v'

-

Yszv;

-

Yrovl

,l

[-r_70.s-

3.666-in

L

,f;;

-

(-

t

+

i3)

1'04

-

(-

0.666

+

j2)

(1.03s46

+

70.03351)

_

(_

z

+

io)f

I

[0.3+ io.r

=

il

tf:;

-

(-

I

+

i3)

(1.03e4

+

70.0335)

-

(-

2

+

j6)

(r.03t7

_

yo.08e3z)

I

J

_

2.967r

_

j8.9962

3-ig

=0'9985-7O'0031

",,il1;,;llii,""rrirhe

permissibte

limits

on

ez

(reactive

power

injection)

are

1--

"

Ytt

_1

Yzt

-

r.04

(-

1

+

13)

Load

Flow

Studies

-

Yo,

vJ

I

-

(-

0.666

+

j2)

(1.019

+

;0.046)

-

(-

2

*

i6)

|

=

''!'-,-1"'.9?'

=

1.028

-

70.087

pu

3.666

-

jrr

I

-

(-

2

+

j6)

(1.028

-

jo.o87)

|

)

-

1.025

-

70.0093

pu

-

r^rr\l

19

+

70.046)

2.99r

-

j9.2s3

3-je

In

Example

6.4,let

bus

2 be

a PV bus

now

with

lV2l

=

1.04

pu.

Once

again

assuming

a flat

voltage

start,

find

Qz,

6.,

V3, V4

at the end

of the first GS

iteration.

Given:

0.2

<

Q,

<

l.

From

Eq.

(6.5),

ii

e",

(Note

fz=

0,

i.e. Vl

-

I.04 +

i0)

n,

=_

:

[J

.,:,,,:.

A,,i".

^r!:, J,

^.,,?,:

:

:;:

!::

^

+

(-

0.666

+

j2)

+

(-

I

+

i3)l

)

=

-

Im

{-

0.0693

-

j0.20791

-

0.2079

pu

'

O"

=

0'2079

Pu

From

Eq.

(6.51)

r.0317

-

Modern

Power

System

Analysis

0.25

<

Qz

<

i.0

pu

It is

clear,

that

other

data

remaining

the

same,

the

calcul

ated

e2

(=

0.2079)

is

now less

than

the

Qz,

^in.Hence

e,

it

set equal

to

ez,

_in,

i.e.

Qz

=

0'25

Pu

a

-

p

---

uvrvrvrv,

tf

2t

vqLt llv

longer

remain

fiied

at

I.04 pu.

The

value

of

V,

ar the

end

of

the

first iteration

is calculated

as

follows.

(Note

VL

=

t

+

70

bf virrue

of

a flat

start.)

I aar{ Ela.., Or.,-r:^^

Lwqv

I tvyy

\)t,U(JlUli

6.6

NEWTON-RAPHSON

(NR)

METHOD

The

Newton-Raphson

method

is

a powerful

method

of

solving

non-linear

algebraic

equations.

It

works

faster

and

is

sure

to

converge

in

most

cases

as

vL

=

l-(

'r,

^!?,

-

yztvt

-

yztv?

- yrovl)

'z

Yr,

[

(Y3)-

-zt

|

-zr

J

-'"

")

_[o.s-jo.zs

3.666

-

jrl

L

t-r,

(*

2 +

i6)1.04

-

(-

0.666

+

.i2)

-

(-

-

4'246-

jlr-,?

=

t.05.59

+

io.o341

3.666

-

jtl

(

vj

=

: l':-:*

-.

Y,,v,

-

Y,,vJ

"

r,,

[

(Y,')'

[_t;u.t

_

(_

r +

j3)

toa

3.666-jrrL

r-;o

*

Consider

a

set of

n

non-linear

algebraic

equations

fi(\,

x2, ...,

xn)

=

0; i

=

I,2,

-..,

n

r+

i3)]

Assur.c

initial

vulucs

of u'know's

as

*l:

*'), ...,

r"r.

Let

J.r(1,

Jg,

...,

J_rl

be

the

corrections,

which

on

being

added

to

the

initial

guess,

givelhe

actual

solution.

Therefore

Jt@\+

futl,

*ur+

Axl,

..,,

x0,+

Axf;1

=

g;

i

=

1,2,

...,

n

(6.54)

Expanding

these

equations

in

Taylor

series

around

the

initial

guess,

we

have

f;(x01,

xor,

(6.ss)

Neglecting

higher

order

terins

we

can

write

Eq.

(6.55)

in

matrix

fbrm

(6.s3)

-

(-

0.666

+

j2X1.0s5e

+

70.0

341)

-

(-

2 +

j6)

]

,,r).[[*)' a*0,

.(#)'

Axt

+

/ ^- \0 I

*[

-l!t]

o-i

l*

n,rn-,

order

re,,ns

-

o

\ux,

)

l

whcrefg)',(

!f, )'. .f9

)'

\

d"r

/

(

Dr,

)

'

[

,",

,/

are

the

derivatives

of

It

with

respect

to

x1,

x2, ...,

x,

evaluated

at

(x!,

*1,

...,

*0,).

vL

=

;;W,#

-

Yo,v,

-

Yo,u|

-

v*v))

=

+,t"=#

-

(-

I +,r3)

(r'0s0e

+

i0'0341)

_

2.8t

t2

-

jn.709

3.666

-

jrr

/,.0630

-

j9

.42M

_

3-

je

1.0347

-

70.0893

pu

-1

-

(-

2 +

j6)

(r.034i

-

j0.08e3)

I

1.0775

+

j0.0923

pu

f''' l

I'rl

a'l'

Llxi

Ax:

or

in vector

matrix

form

(6.56a)

2ll I Modern Power Svstem Analvsis

-

l(,

+

.f,

A*,

*

0

(6.56b)

,f

is k,o*n

as the Jacobian

matrix

(obtained

by

differentiating

the

function

vector

f

with respect to x and

evaluating

it at r01.

Equation

(6.56b)

can be

written

as

:l_J

Approximate

values

of corrections

/-r0

can be obtained

from

Eq

(6.57).

These

being

a set

of linear

algebraic equations

can be solved

efficiently by

triangularization

and back substitution

(see

Appendix

C).

Updated

values of x are

then

tl

="0

+ AxI

or,

in

general, for the

(r

+ 1)th iteration

"(r+l)_r(r)+AxQ)

(6.s8)

Iterations

are continued

till Eq.

(6.53)

is

satisfied

to any desired

accuracy,

i.e.

(6.63a)

at

Alvml

=

0.

We

(6.62c)

mth

bus

First,

assume

that all buses

are

PQ buses.

At any

PQbus

the load

flow solution

rnust

satisfy

the following

non-linear

algebraic equations

lJiG")

)l

<

e

(a

specified

value); i

=

1,2,

..., n

NR Algorithm

for

Load

flow Solution

fip=

Pi

(specified)

-

Pi

(calculated)

=

APi

.fie=

Qi(specified)

-

Qi

@alculated)

-

AQi

(6.5e)

(6.60a)

(6.60b)

(6.61a)

(6.61b)

J'ip

(lV,

6)

=

I'i

(sPccificcl)

-

Pi

=

0

fiq

(1v1,

6)

=

Qi

(specified)

-

Qi

=

o

where

expressions

for

P, and

Q,

are

given

in Eqs.

(6.21)

and

(6.28).

For

a trial

set

of

variables

lV;1, 6;,

the

vector

of

residuals

/0

of Eq.

(6.57)

comesponds

to

while

the

vector

of

corrections

y'xo

corresponds

to

alvil,

a,i

Equation

(6.51)

for

obtaining

the approximate

corrections

vector can be

written

for

the

load

flow case

as

If

the mth

br-rs is also

a

can now

wnte

I

fth busl

,lPi

aQi

ithbusl

np,

l=l T:tT'

I

L-

---J t--

----

----rH''l

- l

46^

AlV^

I

(6.62a)

jmtn

ous

m

It is to

be immediately

observed

that

the Jacobian

elements

corresponding

to

the

ith

bus residuals

and

mth bus

corrections

are a 2

x

2

matrix

enclosed

in the

box

in Eq.

(6.62a)

where i

and m

Ne

both PQ

buses.

Since at the slackbus

(bus

number

l),

Prand

Qr

are

unspecified

and

lV,l,

Q

are fixed,

there

are no equations

corresponding

to Eq.

(6.60)

at this

bus.

Hence

the

slack bus does

not

enter

the

Jacobian

in Eq.

(6.62a).

consider now

the

presence

of PV

buses.

If

the ith

bus

is

a

pv

bus,

e,

is

unspecified

so

that there is

no

equation

corresponding

to Eq.

(6.60b)

for

this

bus.

Therclbrc,

the

Jacobian

eleurents

of the lth

bus become

a

sinele

row

pertaining

to AP,,

i.e.

rthbusF

l=

(6.62b)

PV

bus,

lVrl becomes

fixed

so th

mth

bus

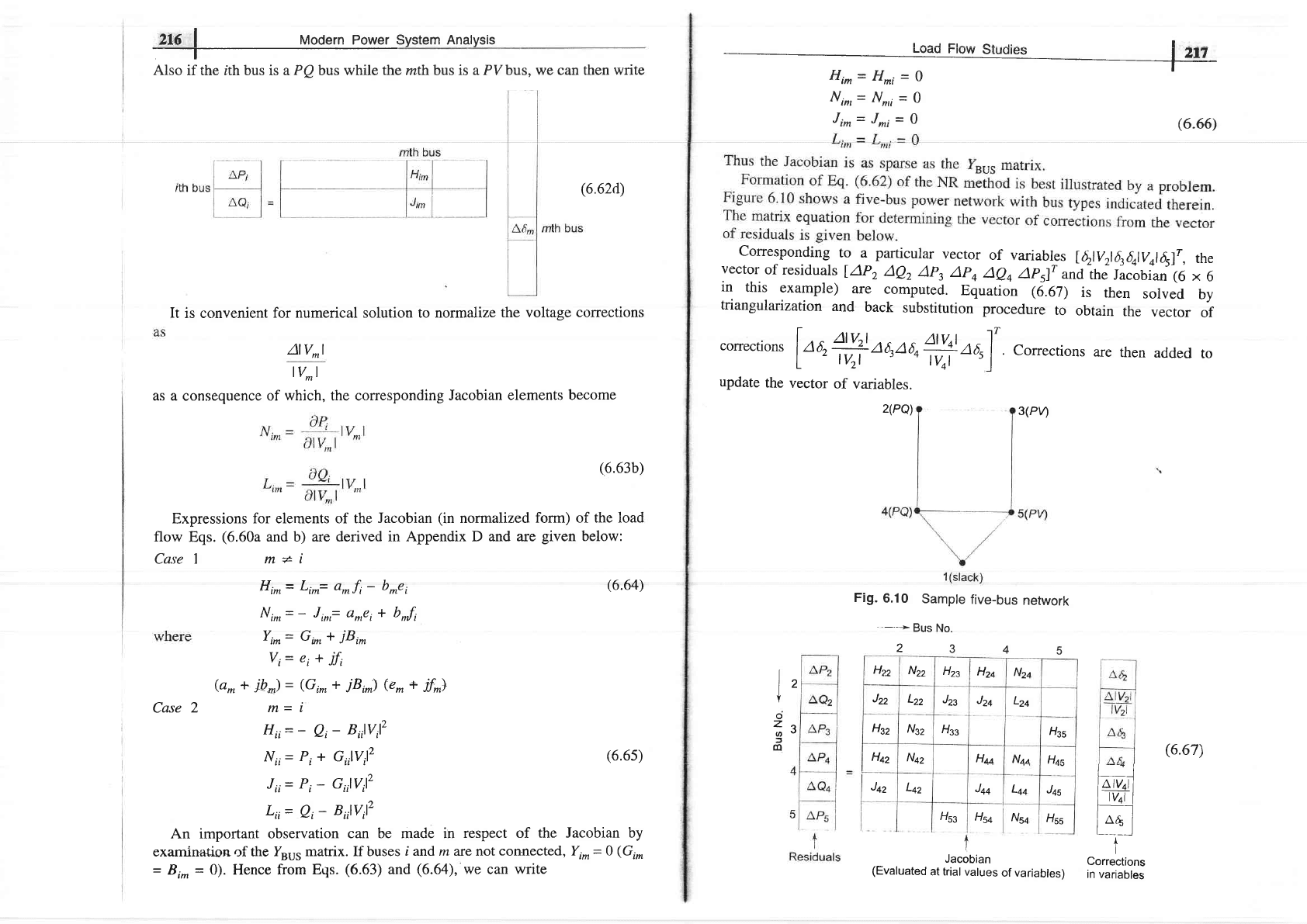
2L6

|

rtrodern Power System Analysis

Also

if the ith bus is a PQ bus while

the mth

bus

is a PV bus, we can then write

(6.62d)

It

is convenient

for numerical solution to normalize

the voltage

corrections

alv^l

lv^l

as a

consequence

of

which,

the corresponding

Jacobian

elements become

l

ztz

t

Ninr=

N^i

=

0

l,^=J^;=O

(6.66)

corresponding

to

a parricular

vecror

of

variables

tqlv2lq64lval6lr,

the

vector

of

residuars

[aP2

aez

ah

ap4

ae4

Apr],

and

the

Jacobian (6

x

6

in

this

example)

are

computed.

Equation

rc.an

is

then

solved

by

triangularization

and

back

substitution

procedure

to

obtain

the

vector

of

f nvt Atrl| 1r

corrections

I

oo,

#

oo1464

+

/,6r

l

.

corrections

are

then

added

to

L

lv2l

J

a

lVor

I

update

the

vector

of

variables.

2(Pa)

3(PVl

(6.63b)

Expressions

for elements of the

Jacobian

(in

normalized

form) of the load

flow

Eqs.

(6.60a

and b) are derived

in Appendix D and are

given

below:

Case I

mtl

H,^= Li^= a^fi

-

b*et

6.64)

Nr*=-

Jirr=

a.er+ bJt

Yi^=

G*

+

jB,*

Vi=

e, +

jf,

(a*

i

ib)

=

(Gi-

+

jBi*)

@*

+

jk\

case

2

I,,==t-

n,

-

Biirvirz

Mii=

Pi +

G,,lV,lz

IA A<\

\\J

' \'J,'

J

ti

=

Pi

-

Giilvil'

Li;=

Qi-

Biilvilz

An

important

observation

can be made in

respect of

the Jacobian by

examination of the

Y"u5 matrix. If buses

i and m are not

connected, Yi^= 0

(Gi^

=

Bi^

-

0). Hence from

Eqs.

(6.63)

and

(6.64),

we can write

I

l2

I

Y

o

f,z

J

c0

Fig.

6.10

Sample

five-bus

network

-'-*

Bus

No.

2345

Jacobian

(Evaluated

at

trial values

of variables)

[:_]

lalv4ll

l]ql-l

i_it_l

t

Corrections

in variables

Hzz

Nzz

Hzs

Hzn

Nza

Jzz

Lzz

Jzs

Jz+

Lz+

Hsz

Nsz

Hss

Hqz

Nn

Haa

Naz.

H+s

Jqz

Lqz

Jcc

Lu

J+s

Hss

H5a

Nsq

Hss

t

(6.67)

,re I rrrrodcrn Power Svstem

Analvsis

LLV

I

-T--

Iterative

Algorithm

Omitting

programming

details,

the

iterative

algorithm

fbr

the

solution

of

the

load

flow

problem

by

the

NR

method

is

as

follows:

L

W'ith

voittge

ancl

angle

(usually

f'=

€I) at

s

absence

of

anY

tlther

at atl

PQ

buses

and

d

at

all

PV

buses'

In

the

information

flat

voltage

start

is

recommended'

2.

Compute

AP,(for

PV

and

PB

buses)

and

AQ,(for

aII

PQ

buses)

from

Eqs.

(6.60a

and

b).

If

all

the

values

are

less

than

the

prescribed

toletance,

stop

the

iterations,

calculate

P,

and

Q,

and

print the

entire

solution

including

line

flows.

3.

If

the

convergence

criterion

is not

satisfied,

evaluate

elements

of

the

Jacobian

using

Eqs.

(6.64)

and

(6.65).

4. Solve

Eq.

(6.67)

for

corrections

of

voltage

angles

and

magnitudes'

5.

Update

voltage

angles

and

magnitudes

by

adding

the

corresponding

changes

to

the

and

return

to

step

2'

Note:

1.

In step

2,

if

there

are

limits

on

the

controllable

B

sources

at PV

buses,

Q

is

computed

each

time

and

if

it violates

the

limits,

it

is made

equal

to

the

limiting

value

and

the

corresponding

PV

bus

is

made

a

PQ

bus

in that

iteration.

If

in

the

subsequent

computation,

Q

does

come

within

the

prescribed

limits,

the

bus

is

switched

back

to

a PV

bus.

2.

If

there

are

limits

on

the

voltage

of

a PQ

bus

and

if

any

of

these

limits

is

violated,

the

corresponding

Pp

bus

is

made

a PV

bus

in

that

iteration

with

voltage

fixed

at

the

limiting

value.

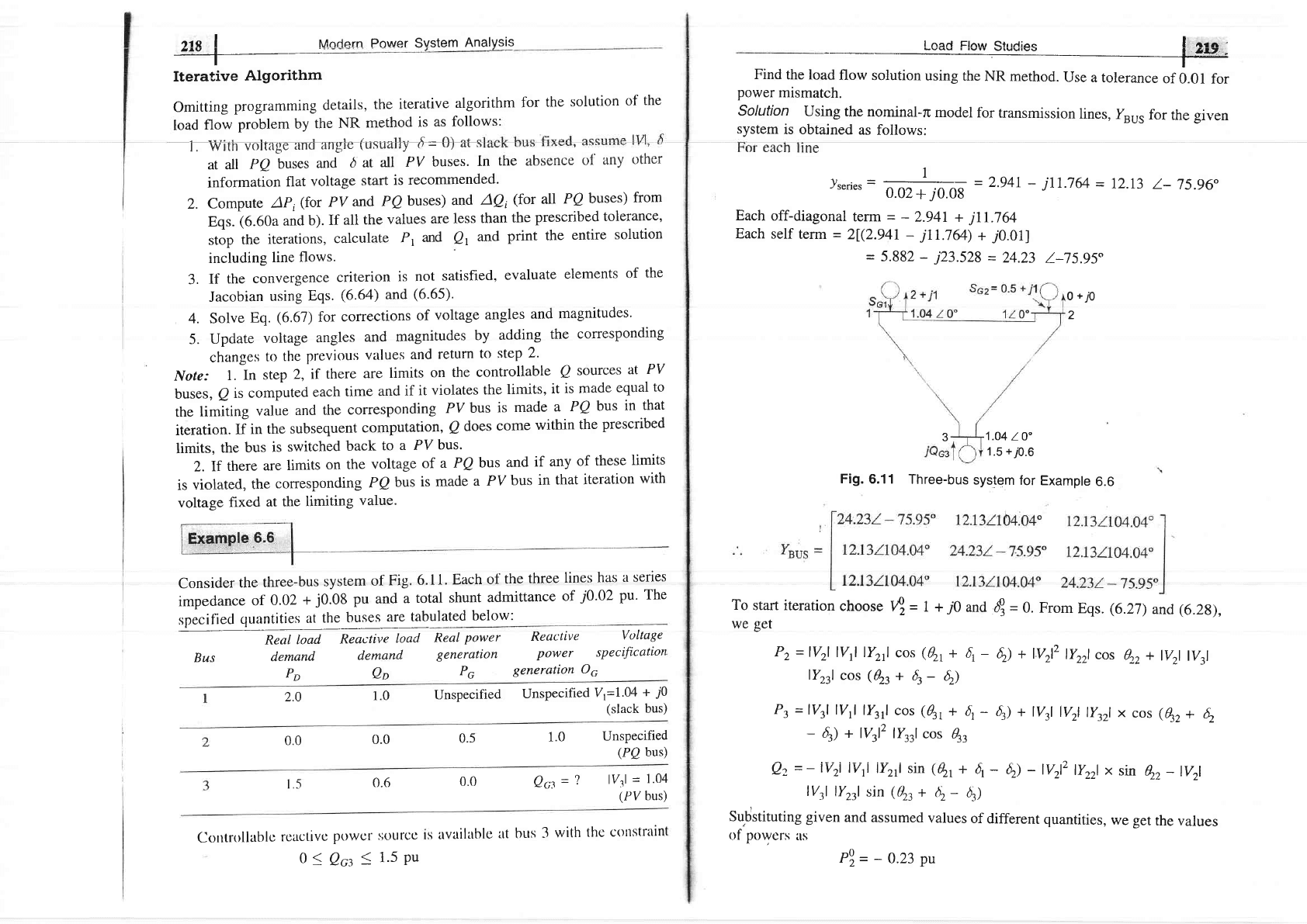
Consider

the

three-bus

system

of

Fig.

6.11.

Each

of

the

three

lines

has

a

series

impedance

of

0.02

+

1O.OS

pu

and

a

total

shunt

admittance

of

70'02

pu' The

specifiecl

quantities

at

the

_b"!91j9!qulated

below:

RealloadReactiveloadRealpowerReactiveVoltage

Lcad Ftor;

Studies

I i:ig,:

-

Find

the load

flow

solution

using

the

NR method.

Use

a tolerance

of

0.01 for

power

mismatch.

Solution

Using

the nominal-rc

model

for

transmission

lines,

I"u,

for

the given

system

is obtained

as follows:

eacn

ltne

1,sen."=

I

^

-z.g4r

-

j11.7&

-

r2.r3

l-75.96"

nes

o.oz+jo.og

Each

off-diagonal

term

=

-

2.94I

+

jll.764

Each

self

term

=

2l(2.941

-

j11.764)

+

j0.011

=

5.882

-

j23.528

=

24.23

l-75.95"

0

+,O

2

Fig.

6.11

Three-bus

system

for

Example

6.6

Pz

=

lVzl

lvl lY2l

cos

(0r1

+

6r

-

$)

+

lVrlz

lyrrl cos

0zz +

lvzl

l\l

lYrrl

cos

(Qzt+

q-

6)

Pt

=

lVl lvl

l\rl

cos

(dr,

+

6r

-

6r)

+

lVrl

lv2l

l\zl x

cos

(ez

+

h

-

6r) +

lV,rlz

lyrrl cos

0r,

Qz

=-ivzi

ivl

tyzl sin

(gr+

dt-

h)

-

lvzlz

ly22l

x

sn

4z_lv2l

lv3l

lY.,.l

sin

(0r,

+

bz

-

6)

Substituting given

and assumed

values

of

different

quantities,

we get

the values

trf

Powers

rts

PB.

=

-0.23

pu

To

start iteration

choose

4=

t +70

and

,4

=

0. From

Eqs.

(6.27)

and

(6.2g),

we

get

Bus

2.0

1.0

Unspecified

V'=1.94

*

;g

(slack

bus)

0.0

Unspecified

(PO

hus)

0.6 Qct=?

lvll

=

1.04

(PV

bus)

Controllablc

rcactivo

powor

sourcc

ls

availablc

nt btts

3

with

the

ctlnstraint

0<Qct

S

1.5Pu

demand

PD

demand

generation

power

specification

Qo

PG

generation

O6

0.0

1.0

0.5

0.0

l5

3

1.04

lO"

1.5

+7O.6

O}nr'l ilada-n Darrrar Crralam Anahraia

ttv.l

rvruuErrr r VYYEr

\)yolgltr

nrrqryoro

t

P3

=

0.12

pu

Qor=

-

0'96

Pu

Power

residuals as

per Eq.

(6.61)

are

-

rt (calcu

(-

0.23)

=

0.73

Aror-

-1.5 -

(0.12)

=

-

!.62

tQT=

1

-

(-

o'e6)

-

t'e6

The changes

in variables at the end of the first iteration are obtained

as follows:

0P, 0P, 0P,

06, 061 alv2l

0P,

aP, 7Pu

06, 061,

0lv2l

aQ, }Qz aQ,

06, 06:'

'av|

Jacobian

elements can be evaluated

by differentiating the expressions

given

above

fr>r

Pr, Py

Qz

with respectto

6r,

d1

and

lVrl and substituting

the

given

and

assumed values at the start of

iteraticln. The chanses

in variables are

obtained

as

Load

Ftow

Studies

i zzr

ti^--i.

Sz=0.5+j1.00

|

-

Sr=-1.5-j0.15

Transmission

loss

=

0.031

Line

flows

The

the

real

part

of

line

flows

0.1913r2E00

0.839861E'00-l

0.0

0.6s4697

E00

|

-0.673847

E00

0.0

J

the

imaginary

part

of

line

flows

-0.5994&E00

_0.r9178zE00]

0.0

0.39604s800

I

-0.37sr6s800

0.0

I

Rectangular

Power-Mismatch

Version

This

version

uses

e,

ancl

.f

,the

rear

ancr

imaginary

parts

of

the

v.ltages

resllectivcly,

as

variables'

'fhe

number

of

equations

and

variables

is greater

than

thart

tirr

Eq.

(6.6r),

by

the

nurnber

<tt

pi

buses.

Since

at

pv

buses

e,and

.f;

can

vary

but

,,'

+.f,'

-

lviP,

a

voltage-magnitude

squarecl

misn-latch

equation

is

required

tbr

each

PV

bus.

with

sparsity

programming,

this

increase

in

order

is

of

hardly

any

significance.

Indeed

each

iteration

is

rnarginally

faster

than

for

Eq'

(6'67)

since

there

are

no

time-consunring

sine

ancl

cosine

terms.

It

nray,

however,

be

noted

that

even

the polar

version

avoids

these

as

far

as possible

9f

using

rectangular

arirhemetic

in

constructing

Eqs (6.64)

and (6.65).

However'

thc

rcct:tttgtrlltr

vcrsion

sccnls

to

bc

slighiiy

t.r,

rcliable

but

faster

in

convergence

than

the polar

version.

The

total

number

of

non-linear

power

flow

equations

considered

in

this

case

arc

fixed

iurd

cqual

2

(trl).

These

lbllow

fr.o'i

Eqs.

(6.26a)

and (6.26b)

and

are

following

matrix

shows

I

oo

I-0.r8422eE00

L-0.826213^800

following

matrix

shows

I

o.o

I

l|0.60s274800

L0.224742E00

Itdjll-24.4i

-t2.23

s.64-1-r[0.i3f

[-0.023-1

| ^r I | .^^^ ^^-l | -^l | ^^.-.1

I

Aai

l:l-

t/..25 /.4.e)

-J.u)l

|

-

t.ozl:l-u.uo)4

|

[nrv,r'-]

L-uu

3.0s

zz.s4)

L

r.qol

I

ooarl

la)l Iai-l l-^4 I t-0.1 [

002.3

I [

0023.1

I

a]

l:l

I

l*l

^4

l:lol*l-006s41:l-006s41

Itv,t'j L'y,roJ lnrv.,r'.1

L'i I

oosoJ

I

r.08eJ

We

can now calculate

fusing

Eq.

(6.28)]

Qtt

=

0.a671

Qo\= Q\

+

Qrt

=

0.4677

+

0.6

=

1.0677

which is

within limits.

If' the

sanre

problem

is solved using a digital

computer,

the

solution

converges

in

three iterations. The final results are

given

below:

Vz=

1.081

l-

0.024

rad

Vt

=

I.M l- 0.0655 rad

Qu

=

-

0.I5

+

0.6

=

0.45

(within

linrits)

Sr

=

1.031 *

j(-

0.791)

P,

(specifi"d)

-I{

e,(epG11,

-

f,B,t)

+

fihrG*

-t

epB,o}

:

0

k:l

i

=

l,

2,

...,

n

i

=

slack(s)

n

rt.

B;

(specifi"d)

-)

lf,koG,o

-

ftB,t1-

e;(.f*Gi*

*

eoB,oy]}

=

g

k=l

(6.68)

(lbr

each

PQ

bus)

(6.69)

222

|

Modern

Porgl

Jyllem Anatysis

I

l(l

(specified)2

-

@i2

+

f,')

=

0

(for

each

PV bus)

(6.70)

Using the NR

method,

the linearised

equations

in the rft

iteration

of iterative

process

can

be written

as

Jr

J2

Load

Flow

Studies

necessary

motivation

in

developing

the

decoupled

load

flow (DLF)

method,

in

which

P-6

and

Q-V

problems

are

solvecl

separately.

Decoupled

Newton

Methods

For

i*

j

Juj=

-

Jqij

=

Gij ei

fi

Jzij=

Jt,j=-

Bijei+

Gilfi

6.72)

Jsij= Jo,j

=

O

For i=,r

t;^"'::: .-::::!-

tlr,,,

-!'

u,u-'

l,) i

J:,

Jsii=

2"i

J6ii

=

2fi

a, and b, are

the components

of the

current

flouring

into node i, i.e.,

a,+

jb,=

frCo

*

jBi)@r+

jf*)

k:l

(6.74)

Steps in solution procedure

are similar

to

the

polar

coordinates

case,

except

that the

initial

estimates

of

real and imaginary

parts

of

the voltages

at the

PQ

buses are

made

and the corrections

required

are obtained

in

each iteration

using

The

corrections

are then

applied

to e andf

and the

calculations

are

repeated

till

convergence

is achieved.

A

detailed

flow

chart describing

the

procedure

for

load flow analysis

using

NR

method is given

in Fig.

6.12.

FE FFAA'?hIEh 'AA

O. I L'|aUL,UTL|1L'

L('AL'

.F LL'VV IVI.EI.tsI(-,L'Ii

An important

characteristic

of

any

practical

electric

power

transmission

system

operating in

steady

state is the

strong

interdependence

between

real

powers

and

bus

voltages

angles

and between

reactive powers

and voltage

magnitudes.

This

interesting

property

of

weak

coupling

between

P-6

and

Q-V

variable.s

gave

the

In

any

conventional

Newton

method,

half

of

the

elements

of

the

Jacobean

matrix

represent

the

weak

coupling

referred

to

above,

and

therefore

may

be

ignored'

Any

such

approximation

reduces

the

true

quadratic

convergence

to

geometric

one,

but-there

are

compensating

computational

benents.i

large

number

of

decoupled

algorithms

have

been

dlveloped

in

the

literature.

However,

only

the

most

popular

decoupled

Newton

veision

is presented

here

t1el.

In

Eq.

(6.67),

the

elements

to

be

neglected

are

submatrices

[1v]

and

[,/].

The

resulting

decoupled

linear

Newton

equations

become

tApl

=

lHl

lA6l

I^Qt

-

tLt l4!1

Ltvt

J

where

it

can

be

shown

that

gU

=

Lij

=

lvil

lvjl

(CU

sin

AQ

AIVP

J3

J4

Js

J6

(6.73)

(6.7s)

Hii=

-

8,,lViP

-

Lii=

-

8,,

lV,lz

+

6u

-

B,i

cos

{r)

i*j

(Eq.

(6.6s))

(Eq.

(6.6s))

Qi

(6.76)

(6.77)

(6.78)

(6.7e)

(6.80)

Equations (6.76)

and

(6.77)

can

be

constructed

and

solved

simultaneously

with

each

other

at

each

iteration,

updating

the

[H]

and

[r]

matrices

in

each

iteration

using

Eqs

(6.78)

to

(6.80).

A

better

opproo.h

is

to

conduct

each

iteration

by

frst

solving

Eq.

(6.76)

for

44

*o

use

the

updared

6

in

constructing

and

then

solving

Eq.

(6.77)

for

Alvl.

This

will

result

in

faster

convcl'geuce

than

in

the

sirnultaneous

mode.

The

main

advantage

of the

Decoupred

Load

Flow

(DLF)

as

compared

to

the

NR

method

is

its

reduced

memory

requirements

in

storing

the

Jacotean.

There

is

not

much

of

an

advantage

from

the point

of

view

of

speed

since

the

time

per

iteration

of

the DLF

is

almost

the

same

as

that

of

NR

method

and

it

always

takes

more

number

of

iterations

to

converge

because

of

the

approximation.

Fast

Decoupled

Load

Flow

(FDLF)

Further

physically justifiable

simplifications

may

be

carried

out

to

achieve

some

speed

advantage

without

much

loss

in

accuracy

of

solution

using

the

DLF

model

described

in

the

This

effort

culminated

in

the

developmenr

of

the

Fast

Decoupled

Load

Frow (FDLF)

merhod

by

B.

stott

in

1974l2ll.

The

assumptions

which

are

valid

in

normal

power

ryr,"n1

operation

are

made

as

follows:

Kothari D.P., Nagrath I.J. Modern Power Systems Analysis

Подождите немного. Документ загружается.