Keyfitz N., Caswell H. Applied Mathematical Demography

Подождите немного. Документ загружается.

1.9. Diﬀerential Fertility Due to the Demographic Transition 25

1.9 Diﬀerential Fertility Due to the Demographic

Transition

Books on population treat the demographic transition in one chapter and in

a quite diﬀerent chapter deal with the diﬀerentials of fertility between social

classes, educational groups, and religious denominations. The transition

is thought of as applying to whole countries, and diﬀerential fertility as

applying to groups within a country, in which, grosso modo, the better

oﬀ, better educated, and urban have the fewest children. There may well

be uncertainty as to how far these classical diﬀerentials are permanent,

applying to all societies at all times, and how far they occur in a particular

historical conjuncture, that in which all birth rates are falling but with

diﬀerent timing. Some observations show a positive relation—the richer the

group, the higher its birth rate—both before the transition and after it has

been passed and birth control techniques made eﬀectively available to all

strata. Much of the economic theory of fertility, summarized by Leibenstein

(1974), Becker (1960), T. W. Schultz (1974), and T. P. Schultz (1973), has

a bearing on this issue. The present section will examine a simple aspect of

the problem: to what degree the diﬀerent times of entry into the transition

can account for the diﬀerent levels of fertility among social classes at any

one moment.

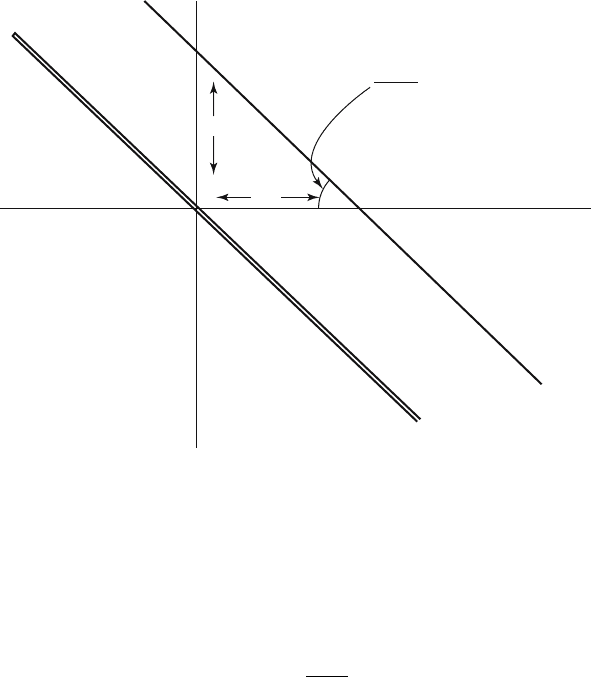
We will approximate the birth rate b(t) by a straight line going from

upper left to lower right in the range of interest; the slope or derivative

db (t)/dt is taken as negative. We suppose also that the slope is the same

for all social groups, and that these are distinguished from one another only

by their degree of horizontal displacement.

The sloping line on the right in Figure 1.2 is displaced from the popula-

tion mean by ∆t, say. It represents a social group whose fall in fertility takes

place later by ∆t than the average of all groups in the country in question.

Suppose that because of its lag this group has fertility ∆b higher than the

average at the time when the country as a whole is passing through the

midpoint of its drop from high to low rates. Then the derivative db(t)/dt

common to all the sloping lines serves to relate for the given subgroup of

the population the departure of the birth rate from the mean at a given

moment and the lag in time:

∆b =

db(t)

∆t. (1.9.1)

Now suppose many sloping lines, representing the several groups in the

country, and square and average over these lines on both sides of (1.9.1) to

ﬁnd



db(t)



, (1.9.2)

26 1. Introduction: Population Without Age

∆b

∆t

Time

Births

b(t)

–db(t)

Average of

all groups

Figure 1.2. Simpliﬁed explanation of diﬀerential fertility in terms of demographic

transition.

where σ

is the variance of birth rates at the time when the population

average goes through the halfway point of decline, and σ

is the variance

of the times at which the several groups go through this point. Take square

roots of (1.9.2) to ﬁnd

db(t)

. (1.9.3)

In this stylized version each statistically identiﬁable subpopulation has its

own demographic transition and falls according to its own straight line, but

with all the straight lines having the same slope. The result (1.9.3) relates

diﬀerential fertility σ

to variation in time of undergoing the transition σ

1.10 Matrices in Demography

1.10.1 A Two-Subgroup Model

Within a population in which there exist statistically recognizable

subpopulations—regions of a country, social classes, educational levels—

we consider now not changes within, but transfers among, such groupings.

Given the rate of growth in each subpopulation and the rates of transfer

among subpopulations, it is possible to describe a trajectory by a set of

1.10. Matrices in Demography 27

diﬀerential or diﬀerence equations. For a simple special case, suppose two

subpopulations of sizes n

(t)andn

(t)attimet.Ifgrowthofthesub-

populations is accompanied by migration in both directions, change in the

system can be described in terms of constants a

, i =1, 2,j =1, 2:

(t +1)=a

(t)+a

(t)

(t +1)=a

(t)+a

(t).

(1.10.1)

Equations 1.10.1 are identical to the matrix equation





(t +1)=







(t), (1.10.2)

which can be written compactly as

n(t +1)=An(t), (1.10.3)

where n(t) is the population vector at time t,andA is the matrix of growth

and transfer rates, referred to indiﬀerently as a projection matrix. If the

rates a

, a

,anda

are ﬁxed over time, (1.10.3) recurrently deter-

mines the population at any arbitrary time subsequent to t. The population

at time t + k equals that at time t successively operated on k times by A:

n(t + k)=A(...(A(An(t)) ...)=A

n(t). (1.10.4)

If a

= a

=0,anda

= a

, the argument of Section 1.5 applies; the

two subpopulations never come into a ﬁnite, nonzero ratio to each another,

and the subgroup with the higher rate keeps growing relative to the one

with the lower rate. But if a

and a

are positive, then, no matter how

diﬀerent a

and a

may be, the two subpopulations will ultimately tend

to increase at the same rate. This stability of the ratio of one population

to the other will of course occur more quickly if a

and a

are large in

relation to the diﬀerence between a

and a

The aspect of stability referred to above is that in which the ratio of

the sizes of the subpopulations ultimately ceases to depend on time. When

this occurs, it follows that each of the subgroups will increase at a rate

not depending on time, that is to say, in geometric progression. For when

population n

is c times as large as population n

, for all times, then, from

the ﬁrst member of (1.10.1)

(t +1)=a

(t)+a

(t)=(a

+ ca

) n

(t),

which proves that n

(t) is a constant multiple of n

(t). This applies to any

number of subgroups, and shows for the linear model of (1.10.1) that, if

the ratio of the sizes of the subgroups to one another is constant, each

is increasing geometrically. It can then be shown that all the groups are

increasing at the same rate. Conversely, if the subgroups are increasing

geometrically and at the same rate, they are in ﬁxed ratios to one another.

When the process of which (1.10.1) is an example attains stability, not

only are all its subpopulations increasing at the same rate and in ﬁxed

28 1. Introduction: Population Without Age

ratios to one another, but also those ratios are not in any way inﬂuenced

by the starting ratios. It could be that n

(0) is 1 million times as large

as n

(0), or that n

(0) is 1 million times as large as n

(0); the two cases

will have the same ultimate ratio of n

(t)ton

(t). This property of the

process, forgetting its past, is a third aspect of stability (see Section 7.2).

Chapter 7 will show how to determine which projection matrices will result

in stability, using life cycle graphs.

There is no moment when stability is suddenly attained. Stability is

a limiting property by which a time can be found when the several

subpopulations increase at rates that are arbitrarily close to one another.

Chapter 5 considers in much detail one case of stability—a population

developing under ﬁxed mortality and rate of increase. To prepare for it

we need to transform raw mortality data into a life table, and this is the

subject of Chapter 2.

The Life Table

The main part of this book starts where demography itself started, with

the life table. The life table is couched in terms of probabilities for indi-

viduals, but for populations it is a deterministic model of mortality and

survivorship. That it presents expected values and disregards random vari-

ation is contrary to the way nature works and, in particular, oversimpliﬁes

demographic mechanisms, yet a rich variety of useful results is based on it.

A method is valuable in direct proportion to the substantive conclusions

to which it leads, and in inverse proportion to its complexity.

The life table is mathematically simple and has a large substantive payoﬀ.

First, it answers questions concerning individuals: what is the probability

that a man aged 30 will survive until he retires at 65, or that he will outlive

his wife, aged 27? But it also answers questions concerning cohorts, groups

of individuals born at the same time: what fraction of the births of this year

will still be alive in the year 2000, or how many of them will live to the

age of retirement? Third, it answers population questions: if births were

constant from year to year in a closed population of constant mortality,

what fraction of the population would be 65 and over?

2.1 Deﬁnition of Life Table Functions

The probability of surviving from birth to age x is designated as l(x) for a

continuous function of x and as l

for discrete x. Life tables usually present

the probability multiplied by 100,000, which is to say, on a radix, l

,equal

30 2. The Life Table

to 100,000. If l

is a probability, then, strictly speaking, what life tables

show is 100,000l

, but it would be pedantic to repeat the 100,000 each time

one refers to the l

column. When l

is interpreted as surviving members

of the cohort, the radix is arbitrary; and setting l

= 100,000 enables one

more easily to think of the column as numbers of persons reaching the given

age.

The diﬀerence in number of survivors for successive ages, l

− l

x+1

,is

designated as d

; and, more generally, the diﬀerence for ages n years apart,

− l

x+n

,is

. This divided by l

is the probability of dying during the

next n years for a person who has reached age x:

− l

x+n

The total number of years lived during the next n years by those who

have attained age x is



x+n

l(a) da, (2.1.1)

which is also the number of persons aged exactly x to x+n (or x to x+n−1

at last birthday) in the stationary population. Setting n equal to inﬁnity

or (indiﬀerently) to ω −x,whereω is the highest age to which anyone lives,

gives

∞

= T



l(a) da.

The quantity T

is the total number of years remaining to the cohort, when

it reaches age x, until the last member dies at age ω. Dividing by l

gives

the average share in this total per person reaching age x:



l(a) da

In terms of probabilities

is the mean of the distribution of years to death

for persons of age x andiscalledtheexpectation of life.

The age-speciﬁc death rate in the life table population is

. This may be compared with the probability

,and

one can think of

as something less than n times

. It is distinct

from

, the observed death rate in a real population;

for

increasing populations at ages beyond about 10.

Theoretical statements on mortality are often expressed most simply in

terms of the age-speciﬁc death rate in a narrow age interval dx, designated

as µ(x):

µ(x) = limit

n→0

− l

x+n



x+n

l(a) da

−1

l(x)

dl(x)

2.2. Life Tables Based on Data 31

[Show that the solution of this gives (1.6.5).] The age-speciﬁc death rate

couldalsobewrittenas

µ(x) = limit

n→0

= limit

n→0

if the mixing of continuous and discrete notation can be excused.

Mortality the Same for All Ages. A mathematical form that has often been

used for short intervals of age, is one in which the force of mortality is

constant. If µ(x)=µ, solving the diﬀerential equation that deﬁnes µ,

µ =

−1

l(x)

dl(x)

gives

l(x)=e

−µx

The probability of living at least an additional n years after one has attained

age x is

x+n

−µ(x+n)

−µx

= e

−µn

The expectation of life is

l(x)



∞

l(x + t) dt

−µx



∞

−µ(x+t)

dt =

Both the probability of living an additional n years beyond age x and the

expectation of life at age x are constants independent of x. The prospect

ahead of any living person is the same, no matter how old he is, on this

peculiar table. All the columns of the life table are in general derivable

from any one column, and if any column is age-independent all the others

referring to a person’s prospects must be also.

Application of these deﬁnitions to other mathematically speciﬁed forms

of µ(x) requires more diﬃcult integration to ﬁnd l(x)and

. The function

µ(a)=µ

/(ω − a) is applied in Section 4.3. Here we proceed to methods

for constructing a life table from empirical data.

2.2 Life Tables Based on Data

We read that for the United States during the year 1967, 122,672 men aged

65 to 69 years of age at last birthday died, and that the number of men

of these ages alive on July 1, 1967, was 2,958,000. These may be called

observations. We can divide the ﬁrst ﬁgure by the second and ﬁnd that the

32 2. The Life Table

age-speciﬁc death rate for males 65 to 69, denoted as

. was 0.04147,

again straightforward enough to be called an observation. How do these

observations tell us the probability, 1 − (l

), that a man chosen at

random from those aged exactly 65 will die before reaching age 70? This

is the same as asking what fraction of a cohort of men aged 65 would be

expected to die before reaching age 70, and its complement, l

,the

fraction of those aged 65 who survive to age 70. From time period data we

want to know something about a cohort, men moving from age 65 to 70.

This cohort is necessarily hypothetical, for with only one period of obser-

vation the best we can manage is to suppose mortality to be unchanging

through time. No real cohort is likely to exhibit the regime that will be

inferred from 1967 data.

The observations of a given period, the calendar year 1967, are, moreover,

ambiguous because we know nothing about the distribution of exposure

within each 5-year age interval, aﬀected as it is by all the accidents of the

birth curve and of migration.

2.2.1 Assuming Constant Probability of Dying with the Age

Interval

If, in addition to supposing that the probability of dying is invariant with

respect to time, we suppose it to be invariant with respect to age within

the 5-year group, the probability l

can be inferred from the rate

in a

straightforward manner. We saw in Section 1.6 that a population initially

of size N

and increasing at constant rate r reaches a total N

after t

years. If it is decreasing at constant rate µ,aswillbethecaseifitisclosed

and subject to a constant death rate µ,anditnumbersl

at the start, then

at the end of 5 years it will be l

x+5

= l

−5µ

, as in the preceding section.

Identifying

with µ gives

x+5

= e

−5

(2.2.1)

as a ﬁrst approximation to the desired ratio l

x+5

. For our data on the

United States in 1967, (2.2.1) is

= e

−5

= e

−5(0.04147)

=0.81274.

This would be exact if either (a) the death rate were constant through the

5-year age interval, or (b) the population exposed to risk were constant

through the 5-year age interval. Neither of these, however, applies in prac-

tice; in general, beyond age 10 the death rate is increasing through the

interval and the population is diminishing.

2.2. Life Tables Based on Data 33

2.2.2 The Basic Equation and a Conventional Solution

Suppose that the age distribution of the exposed population is given by

p(a) within the age interval x to x + 5, and that at exact age a the age-

speciﬁc death rate is µ(a). Then the observed rate

can be identiﬁed

with a ratio of integrals:



x+5

p(a)µ(a) da



x+5

p(a) da

. (2.2.2)

Making a life table is a matter of inferring l

x+5

from (2.2.2). This is

equivalent to inferring the unweighted



x+5

µ(a) da from the weighted av-

erage of the µ(a) in (2.2.2), for from (1.6.5) we know that



x+5

µ(a) da =

−log(l

x+5

), so the unweighted average of the µ(a) gives the l(x) column

(Weck 1947).

Basic equation 2.2.2 reminds us that the interpretation of the observed

depends on the (unknown) distribution of population within the age

interval x to x + n. We must somehow extract



x+5

µ(a) da from (2.2.2), a

task that appears hopeless when nothing is known but

. Yet every life

table based on empirical data in 5-year groups implicitly infers



x+5

µ(a) da

from (2.2.2); this is achieved by making assumptions that somehow restrict

p(a).

One common solution of (2.2.2) is to assume p(a)=l(a), and also to

suppose that l(x) is a straight line. The integral under the straight line is



x+5

l(a) da =

+ l

x+5

). (2.2.3)

Since



x+5

l(a)µ(a) da = l

− l

x+5

, (2.2.2) may be written as

− l

x+5

+ l

x+5

)

, (2.2.4)

from which the value of l

x+5

may be obtained by dividing numerator

and denominator on the right by l

, and then solving a linear equation for

x+5

to ﬁnd

x+5

1 − 5

1+5

. (2.2.5)

For United States males aged 65 to 69 this is 0.81213, obtained from

0.04147. This is closer to the corrected value than the 0.81274 of (2.2.1).

From (2.2.5) the probability of dying is

=1−

x+5

1+5

. (2.2.6)

34 2. The Life Table

2.2.3 A Precise Life Table Without Iteration or Graduation

To improve on (2.2.5) or the exponential (2.2.1), we go a step further in

extracting from (2.2.2) the quantity



x+5

µ(a) da. We would expect the

answer to emerge as



x+5

µ(a) da =5(

+ C), where it will turn out

that C is a correction easily obtained on the assumption that both the

population p(a) and the death rate µ(a) change linearly within the interval.

We calculate the correction to

for a general interval of n years,

starting at age x. First we change variables by writing a = x +(n/2) + t;

(2.2.2) with n in place of 5 becomes



+n/2

−n/2

p[x +(n/2) + t]µ[x +(n/2) + t] dt



x+n

p(a) da

. (2.2.7)

Expanding each of the fractions in the numerator by Taylor’s series to the

term linear in t gives



+n/2

−n/2

[p(x + n/2) + tp



(x + n/2)][µ(x + n/2) + tµ



(x + n/2)] dt



x+n

p(a) da



tp(x + n/2)µ(x + n/2) +



(x + n/2)µ



(x + n/2)



+n/2

−n/2



x+n

p(a) da

np(x + n/2)µ(x + n/2) + (n

/12)p



(x + n/2)µ



(x + n/2)



x+n

p(a) da

(2.2.8)

But this can be translated into known quantities as follows:

1. If p(a) is a straight line in the interval between x and x + n,

then np(x + n/2) is the same as



x+n

p(a) da and cancels with the

denominator.

2. If µ(a) is a straight line between x and x + n, then the midvalue

µ(x + n/2) is 1/n of the integral we seek, i.e., is (1/n)



x+n

µ(a) da.

3. The integral



x+n

p(a) da is the observed population in the age

interval,