Keyfitz N., Caswell H. Applied Mathematical Demography

Подождите немного. Документ загружается.

4.1. The Multiplicity of Index Numbers 75

Table 4.2. Ratio of male to female mortality, United States, 1967, and ﬁve other

countries

United West

States, Austria, France, Germany, Greece, Mexico,

Index 1967 1966–68 1967 1967 1968 1966

1.871 2.035 1.918 1.879 1.578 1.211

1.874 1.997 1.912 1.855 1.573 1.218

1.732 1.706 1.863 1.649 1.363 1.176

1.614 1.560 1.669 1.546 1.297 1.144

1.774 1.890 1.847 1.780 1.530 1.186

1.779 1.866 1.840 1.781 1.526 1.192

1.654 1.620 1.775 1.580 1.317 1.142

1.530 1.487 1.589 1.485 1.260 1.105

1.821 1.961 1.884 1.839 1.555 1.198

1.825 1.930 1.878 1.818 1.550 1.205

1.694 1.663 1.820 1.614 1.339 1.160

1.572 1.522 1.628 1.514 1.278 1.125

1.630 1.697 1.703 1.687 1.371 1.374

1.629 1.675 1.699 1.673 1.371 1.375

1.407 1.444 1.631 1.445 1.185 1.960

1.286 1.334 1.470 1.370 1.158 1.359

1.108 1.102 1.109 1.091 1.052 1.056

For the male-population-weighted case for the United States of 1967 (Table

4.2)

=1.871,H

=1.774,

of which means the geometric mean is 1.822, the same as G

except for

rounding.

The index numbers for the countries of low mortality in Table 4.2 typi-

cally show males to have 50 percent higher mortality than females. On the

other hand, the expectation of life for females is nowhere much more than

10 percent higher than that for males. The ratio of the female to male

that is,

, may be thought of as the ratio of male to female death

rates in the respective stationary populations, and why it is so much lower

than the other indices is investigated in Section 4.3.

4.1.2 Aggregative Indices Versus Averages of Relatives

Suppose one set up the criterion that an index ought to be aﬀected in about

the same amount by a change of one death at one age as at another. At

least, it can be argued, the eﬀect of an added death at one age ought not to

76 4. Mortality Comparisons; The Male-Female Ratio

be 100 times as great as the eﬀect at another age. The addition of a single

death to males at age x raises the death rate by 1/p

and thus raises the

numerator of the aggregative index I

of (4.1.4) by p

. Insofar as the

ratio of males to females does not vary greatly in absolute numbers among

age groups, the weight given to an extra male death in I

is approximately

invariant with age.

The same point can be made more generally by considering the eﬀect

of a small change ∆µ

in a male death rate on I

; taking a small ﬁnite

diﬀerence, we obtain

∆I

∆µ



The denominator of this expression is the same regardless of the age x at

which the change occurs; the numerator p

∆µ

is of the order of magnitude

of the additional absolute number of deaths.

Quite diﬀerent is the eﬀect on the weighted relatives I

of (4.1.1), where

the eﬀect of an increment ∆µ

in the male death rate is

∆I

(∆µ

/µ

)



which depends on the reciprocal of the female death rate at the age in

question. Now the numerator contains p

(∆µ

), which is the number of

additional male deaths, but it also contains the reciprocal of µ

,whichcan

be anywhere from 1/0.001 to 1/0.1 or even fall outside this range. That a

small change in male deaths can have an eﬀect on the comparison 10 times

as great at age 20 as at age 70 argues against the index I

4.2 Should We Index Death Rates

or Survivorships?

Superimposed on the great variation in the above indices is an even greater

variation arising from the choice between death rates and survival rates

for the comparison. Consider the United States versus England and Wales,

each about the time of the last census, and take males aged 70 to 75 as an

example. The probabilities of dying are as follows:

U.S.:

=0.2526 versus England and Wales:

=0.2915,

so that England and Wales, 1960–62, would have 0.2915/0.2526 = 1.154

times as high a probability of dying as the United States in 1959–61. The

corresponding probabilities of surviving are

=1−

U.S.:

=0.7474 versus England and Wales:

=0.7085,

4.2. Should We Index Death Rates or Survivorships? 77

so that the United States has 1.055 times the survival probability of Eng-

land and Wales. We do not know from this whether to say that the United

States is healthier (for the age group in question) than England and Wales

by 5.5 percent, or that England and Wales are more hazardous than the

United States by 15.4 percent.

Without further sharpening of the model, as Sheps (1959) insists, we

must abandon the statement of a percentage excess in either direction and

be satisﬁed with the diﬀerence. The most that can be said is that the

diﬀerence in the probability of dying is 0.2915−0.2526 = 0.0389, this being

identical with the diﬀerence 0.7474 −0.7085 in the probability of surviving.

To say more invites the inconsistencies of the preceding paragraph.

But suppose it were the case (presented here only for illustration) that

Englishmen of this age die of all the causes Americans die of plus some

causes special to them. American mortality, represented by the probability

of dying within a year, is q, say, and English mortality for those who do

not die of the American causes is δ. Then the English probability of dying

is q +(1− q)δ, and the ratio of this to q is 1 + [(1 − q)/q]δ, an expression

that involves q and δ intertwined in complex fashion. But the American

chance of survival is 1 − q, and the English is (1 − q)(1 − δ), so the ratio

of probabilities of survival is (1 − q)(1 − δ)/(1 − q)=1− δ. This involves

δ and omits q altogether. Its complement is simply δ, the pure additional

English mortality, obtainable easily from the ratio of survivorships but not

in any direct way from the probabilities of dying.

Insofar as we adhere to this model, we ought to make an index consisting

of the weighted complements of the age-speciﬁc death rates, and then take

the complement of the resultant index. But the argument depends on the

Englishman’s being subject to a source of extra mortality that enters only

if he escapes the American sources. Sheps (1959) raises the point in regard

to smokers and nonsmokers; the former do have a clear added hazard if

they escape all the causes of death to which the latter are liable. It is not

obvious that such a model applies to the whole of intercountry or intersex

comparisons, but it could apply in part. There could be a climatic or other

special hazard in England; there could be some added hazard through heart

disease (or, at younger ages, accident) for males in addition to the dangers

to which women are subject.

Let us take the Sheps model one stage further and suppose some limited

overlap, that is to say, common causes of mortality as well as causes special

to each group. Let both men and women be subject to q;andmenin

addition to δ

and women in addition to δ

, the additional causes being

restricted to individuals who do not die of the common causes. These are the

parts of the model assumed to underlie the observed survival probabilities

for men (P

) and for women (P

). Then we have P

=(1− q)(1 − δ

)

78 4. Mortality Comparisons; The Male-Female Ratio

and P

=(1− q)(1 − δ

); the ratio of survivorships is

(1 − q)(1 − δ

)

(1 − q)(1 − δ

)

1 − δ

This is not bad: the ratio of survivorships taken from the raw data gives

exactly the ratio of the chances of escaping the special female and male

hazards, respectively.

Compare this with the ratio of the probabilities of dying, the comple-

ments of the P ’s, now taken as male to female:

1 − P

1 − (1 − q)(1 − δ

)

1 − (1 − q)(1 − δ

)

+ q(1 − δ

)

+ q(1 − δ

)

The interpretation of this is straightforward only if q = 0, that is, if there

are no common causes. If, however, there are common causes, the ratio

of observed death rates does not provide the ratio δ

/δ

of the causes

special to the sexes, but a biased estimate of this ratio. Where q may be

appreciable, the terms in q in numerator and denominator obscure the

/δ

that it is natural to seek.

Uncertainty as to the overlap of causes, and hence uncertainty as to

whether one ought to be comparing the chances of survival or of death,

constitute a major obstacle to precise comparison of overall rates. In non-

experimental comparisons, this consideration serves as a caution against

excessive reﬁnement in index numbers of the type presented in (4.1.1) to

(4.1.4).

4.3 Eﬀect on

of Change in µ(x)

The index number problem applies as much to changes through time as to

comparisons across space. Given a general initial age schedule of mortality,

suppose a certain kind of change in that schedule and see what the eﬀect

is on the expectation of life. We do this ﬁrst with a constant increase δ in

µ(x) at all ages, so that µ(x) becomes µ(x)+δ.

When the ﬁxed quantity δ is added to mortality at every age, the prob-

ability of surviving to age x becomes exp[−



[µ(a)+δ] da]=e

−δx

l(x),

that is, is altered in the ratio e

−δx

; if the odd probability was l(x), the new

one is e

−δx

l(x). Also, the new expectation of life (distinguished by

∗

)isthe

integral of this through the whole of life:

∗



−δx

l(x) dx. (4.3.1)

4.3. Eﬀect on

of Change in µ(x)79

To ﬁnd the eﬀect on the expectation of life of the addition δ to the

age-speciﬁc death rates we seek the derivative d

∗

/dδ:

∗

dδ

= −



−δx

l(x) dx = −¯x

evaluated at δ =0,if¯x is the mean age of the stationary population. In

ﬁnite terms for δ small,

∆

≈−¯x

δ or

∆

≈−¯xδ. (4.3.2)

The relative change in the expectation of life equals minus the change in

the death rate times the mean age in the life table population. Thus, if the

expectation of life is 70 years, 0.001 is subtracted from mortality µ(x)at

every age, and the mean age in the life table population is 35 years, the

fraction added to the expectation is approximately (35)(0.001) = 0.035 or,

in absolute amount, (0.035)(70) = 2.45 years.

4.3.1 A Proportional Diﬀerence Uniform at All Ages

However, although the eﬀect of a ﬁxed diﬀerence in death rates is express-

ible in simple form, we are more likely to be interested in the eﬀect of

a given proportional diﬀerence. Ratios of age-speciﬁc mortality rates are

hardly constant between any two groups, but for developed countries the

ratio of male to female mortality ranges from about 1.10 to about 2.80

(Table 4.1); certainly the ratios are closer to constancy than the diﬀerences

(not shown), which vary from about 0.002 to 0.0400. The largest ratio is 2

to 3 times the smallest; the largest diﬀerence is 200 times the smallest.

Suppose now that the death rate µ(x) is multiplied by 1 + δ,sothat

∗

(x) = (1+δ)µ(x). Then the new probability of surviving to age x becomes

∗

=exp



−



∗

(a) da



=exp



−



(1 + δ)µ(a) da



= l

1+δ

and the new expectation of life is

∗



l(a)

1+δ

da. (4.3.3)

The application of this to the special function µ(x)=µ

/(ω − x)is

satisfactorily simple. Integrating and taking the exponential gives l(x)=

[1 − (x/ω)]

. Integrating this in turn gives

=(ω − x)/(µ

+ 1). Then

we have

∗



[l(x)]

1+δ



l(x) dx

(1 + δ)+1

. (4.3.4)

80 4. Mortality Comparisons; The Male-Female Ratio

If mortality at all ages rises by δ,then

∗

declines but by a lesser amount,

as (4.3.4) shows. For males compared with females δ might be 0.43, and µ

might be 0.30. Then by (4.3.4) the ratio

∗

equals 1.43/1.30 = 1.10.

Integrating the reciprocal of the expectation of life as the life table death

rate, an excess of 43 percent in all age-speciﬁc death rates translates into an

excess of 10 percent in the life table overall death rate, roughly consistent

with Table 4.2.

Butthisisonahyperboliccurveforµ(x); in general the proportionate

change of µ(x) does not so easily translate into a change of

, and we need

the ﬂexible diﬀerential calculus to establish a constant useful for describing

life tables. To ﬁnd the eﬀect of a small change δ on the expectation of life,

we seek the derivative of the right-hand side of (4.3.3) with respect to δ

and ﬁnd

∗

dδ



[log l(a)]l(a)

1+δ

da, (4.3.5)

a quantity that cannot be positive, since l(a) cannot be greater than unity.

In the neighborhood of δ =0wehave

∆

≈



[log l(a)]l(a) da



l(a) da

δ = −Hδ, (4.3.6)

say, where H is minus the mean value of log l(a), weighted by l(a). (The

measure H is called entropy or information in other contexts, and has

been applied in population biology by Demetrius (1974, 1983); see Tul-

japurkar (1982) for its relation to the rate of convergence to the stable age

distribution.)

The ratio of integrals in (4.3.6) is necessarily negative, so that H is

positive. We can imagine H as low as zero, if all mortality were concentrated

at one age. If, for instance, everyone lives until 70 and then dies, l(a) will

be unity for all ages up to 70, and its logarithm will be zero. At the other

extreme, if mortality µ is the same at all ages, we will have l(x)=e

−µx

=1/µ, a constant, and

∆

≈



[log 1(a)]l(a) da



l(a) da



∞

−µae

−µa

1/µ

δ = −δ.

In this case H = 1, and the proportional change in the death rates trans-

lates into the same change in the expectation of life, but of course in the

4.3. Eﬀect on

of Change in µ(x)81

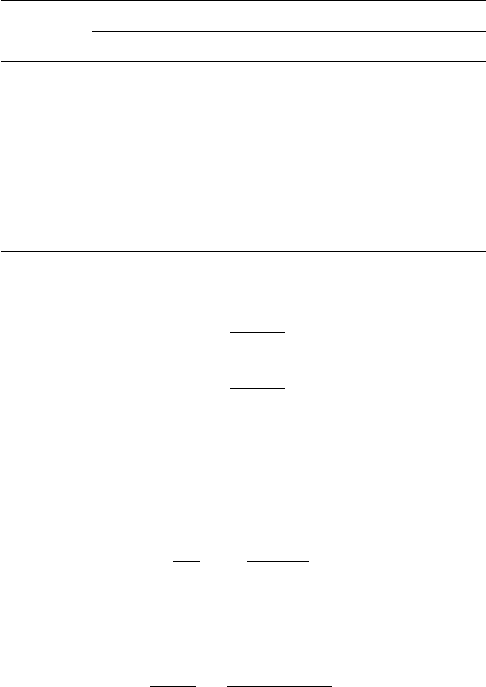
0 Age x

Probability of surviving l(x)

l(x) = 1

l(x) = e

–␮x

H = 1

H = 0.5

H = 0.3

H = 0.2

H = 0

Figure 4.1. Extreme cases of survivorship curves l(x), and three intermedi-

ate cases, showing how H becomes smaller as survivorship moves toward a

rectangular form.

opposite direction. With H = 1, when the death rates at all ages increase

by 1 percent, the expectation of life diminishes by 1 percent. [Show that

for l(x) a straight line, H =0.5.]

Figure 4.1 shows the l(x) for the two extreme cases and for intermediate

ones, with corresponding values of H. Deevey (1950) gives a number of

curves for animal species that resemble the several curves of Figure 4.1,

with fruit ﬂies near H = 0, oysters below H = 1, and hydra near H =

4.3.2 Observed Values of the Constant H

For countries of Europe and America with expectations of life around 70

years, H is now of the order of 0.2 for males and 0.15 for females, down from

the 0.3 to 0.4 of about 30 years earlier. Apparently H is a convenient sum-

mary of the degree of concavity in an l(x) column; as mortality improves,

a larger fraction of deaths occurs in the 60s and 70s of age, and the drop

in the value of H measures this tendency; with improvement in mortality

everyone dies at about the same age, and a proportional improvement in

mortality at all ages makes less and less diﬀerence in the expectation of

life.

When we are told that, of two countries, or two sexes, one has an ex-

pectation of life in a certain ratio to the other, we can approximate to the

ratio of age-speciﬁc death rates, supposing this ratio to be 1 + δ,thesame

82 4. Mortality Comparisons; The Male-Female Ratio

at all ages. Taking

∗



l(x)

1+δ



l(x) dx

as a function of δ,sayf(δ), and expanding as f(δ)=f(0) + δf



(0), where

we know that f



(0) = −H,gives

∗

≈ 1 − δH.

If, for example,

∗

=1.10, we can say that

1.10 =



l(x)

1+δ



l(x) dx

≈ 1 − δH;

and if H is 0.20, we have the equation for δ:

1.10 = 1 − (δ)(0.20),

δ =

1 − 1.10

0.20

= −0.50.

The population with 10 percent greater expectation of life has death rates

50 percent lower. This approach can also be tried when the ratio of death

rates is not uniform, and to this situation we proceed.

4.3.3 An Aspect of the Index Number Problem

The theory developed above seeks to ﬁnd the eﬀect on expectation of life of

a uniform proportional excess in the force of mortality µ(x). What we have

in practice, however, is diﬀerent proportional increases at the several ages.

One way of answering the question of what is the real average increase—

for example, the real percentage excess of male over female mortality—is

to calculate the proportional increase that, applied uniformly to all ages,

will have the same eﬀect on the expectation of life as the observed set of

increases. In application to the excess mortality for the United States, 1967,

which showed (Table 4.1) a ratio of 1.30 for ages under 1, 1.25 for those 1

to 4, etc., we would ask what uniform excess of male over female mortality

would provide the same ratio of expectations of life as observed. Since we

saw that raising µ(x) in the ratio 1 + δ raises l(x)tothepower1+δ,we

4.3. Eﬀect on

of Change in µ(x)83

would need to solve the equation



l(x)

1+δ



l(x) dx

(4.3.7)

for the unknown δ. This can be done directly by computer.

The equation can also be solved approximately in terms of our param-

eter H, along with other derivatives of the numerator of the right-hand

side of the equation. Rewriting l(x)

1+δ

in (4.3.7) as l(x)exp[δ log l(x)] and

expanding the exponential term in a Taylor series changes the equation to

=1− Hδ +

−

+ ···, (4.3.8)

where



[−log l(x)]

l(x) dx



l(x) dx

,i=2, 3,....

Approximating with the linear term only, we have for Italy, 1964, whose

=1.081,

δ =





− 1

−H

(1/1.081) − 1

−(0.207 + 0.163)/2

then using H from Table 4.3. (It seems best to average H for males and

females.) The result is

δ =

0.0749

0.185

=0.405,

or 1 + δ =1.405. This compares with I

=1.441, or an average of I

and

of 1.490. [For greater accuracy the reader may wish to experiment with

further terms of (4.3.8).]

Thus, given the expectations of life of two populations, along with an

average H, we can say what uniform excess of mortality of one over the

other accounts for the ratio of expectations. This is the converse of the

way in which H was originally derived—as the ratio of expectations of life

corresponding to a given uniform ratio of death rates.

For the particular hyperbolic form in which

µ(a)=

ω − a

(4.3.9)

l(a)=



1 −



(4.3.10)

84 4. Mortality Comparisons; The Male-Female Ratio

Table 4.3. Values of

and parameter H = −



[log l(a)]l(a) da/



l(a) da for

males and females, United States, 1919–21 to 1959–61

Male Female

1919–21 54.59 0.3804 56.41 0.3547

1924–26 56.34 0.3401 59.01 0.3113

1929–31 57.27 0.3272 60.67 0.2942

1934–36 58.53 0.3105 62.58 0.2725

1939–41 61.14 0.2747 65.58 0.2361

1944–46 62.26 0.2632 68.11 0.2087

1949–51 65.28 0.2260 70.86 0.1823

1954–56 66.45 0.2134 72.61 0.1660

1959–51 66.84 0.2083 73.40 0.1594

Source: Computed from data in Keyﬁtz and Flieger (1968).

ω − a

(4.3.11)

H =

(4.3.12)

H tells us considerably more than is indicated by its being the ﬁrst deriva-

tive. It happens also to give without approximation the value of the increase

(or

for that matter) when µ(a)goestoµ(a)(1 + δ), and when δ

may be large. For the ratio of expectations, we have

1+δH

under the hyperbolic mortality function assumed. In the example given,

with

=1/1.081, H =0.185; hence the equation for δ is

1.081

1+δ(0.185)

or δ =0.081/0.185 = 0.438. Thus the fact that Italian females of 1964 have

8.1 percent longer expected life than males is the equivalent of their having

43.8 percent as high mortality.

Although this holds exactly only for the special graduation µ(a)=

/(ω − a), it is probably an improvement when δ is substantial for any

life table. Hence the rule for ﬁnding the new

when all rates rise by δ is

to divide the old

by 1 + δH, rather than multiplying by 1 − δH as in

the ﬁrst approximation to (4.3.8).