Jeanblanc M., Yor M., Chesney M. Mathematical Methods for Financial Markets
Подождите немного. Документ загружается.


530 9 General Processes: Mathematical Facts
where f
(T )
(x, h)=
f(x+h)−f(x)−f
(x)h
h
2
1
{h=0}
(here, T stands for Taylor). In
particular,
f
(T )
(X
s
−
,H
s
)=
1
0
dx(1 − x)f
(X
s
−
+ xH
s
) .
We note that the jumps of
t
0
f(X
s
−
+ H
s
) − f (X
s
−
) − f
(X
s
−
)H
s
H
s
1
{H
s
=0}
dM
d
s
are
f(X
s
−
+ H
s
) − f (X
s
−
) − f
(X
s
−
)H
s
H
s
(ΔX
s
)=f
(T )
(X
s
−
,H
s
)(ΔX
s
)
2
.
From the hypothesis, the process
s≤t
f
(T )
(X
s
−
,H
s
)(ΔX
s
)
2
−
t
0
f
(T )
(X
s
−
,H
s
)dA
s
is a martingale, and it has the same jumps as
t
0
f(X
s
−
+ H
s
) − f (X
s
−
) − f
(X
s
−
)H
s
H
s
dM
d
s
,
hence, these two purely discontinuous martingales are equal.
Proposition 9.4.1.2 Let X be a PCSM with decomposition M + V and let
f be a function in C
2
b
.Then,
f(X
t
)=M
f
t
+ V
f
t
= M
f,c
t
+ M
f,d
t
+ V
f
t
where
M
f,c
t
=
t
0
f
(X
s
−
)dM
c
s
=
t
0
f
(X
s
)dM
c
s
,
V
f
t
=
t
0
f
(X
s
−
)dV
s
+
1
2
t
0
f
(X
s
)dM
c
s
+
t
0
f
(T )
(X
s
−
,H
s
)dA
s
,
M
f,d
t
=
t
0
f
(T )
(X
s
−
,H
s
)(d[M
d
,M
d
]
s
− dA
s
) .
Exercise 9.4.1.3 Let N be an inhomogeneous Poisson process with intensity
λ(t), M its compensated martingale, W a Brownian motion and
dX
t
= h
t
dt + f
t
dW
t
+ g
t
dM
t
,
where f, g and h are (bounded) predictable processes. Using the identity
s≤t
φ
s
ΔN
s
=
t
0
φ
s
dN
s
,provethat
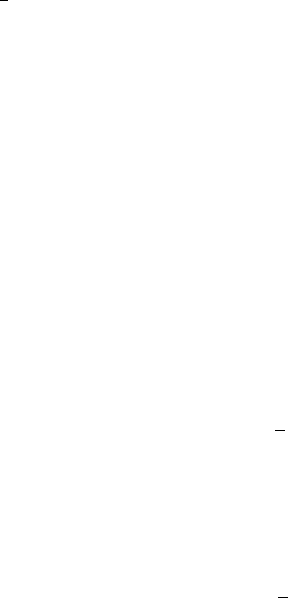
9.4 Itˆo’s Formula and Girsanov’s Theorem 531
F (t, X
t
)=F(0,X
0
)+
t
0
∂
s
F (s, X
s
) ds +
t
0
∂
x
F (s, X
s
)h
s
ds
+
1
2
t
0
∂
xx
F (s, X
s
)f
2
s
ds
+
t
0
[F (s, X
s
+ g
s
) − F (s, X
s
) − ∂
x
F (s, X
s
)g
s
]λ
s
ds
+
t
0
∂
x
F (s, X
s
)f
s
dW
s
+
t
0
[F (s, X
s
−
+ g
s
) − F (s, X
s
−
)] dM
s
.
9.4.2 Semi-martingale Local Times
Let X be a semi-martingale.
Proposition 9.4.2.1 The measure ϕ →
t
0
ϕ(X
s
)dX
c
s
is absolutely contin-
uous with respect to the Lebesgue measure. Its Radon-Nikodym density may
be defined as L
x
t
the local time at x which satisfies the Itˆo-Tanaka formula:
(X
t
− x)
+
=(X
0
− x)
+
+
]0,t]
1
{X
s
−
>x}
dX
s
+
0<s≤t
1
{X
s
−
>x}
(X
s
− x)
−
+
0<s≤t
1
{X
s
−
≤x}
(X
s
− x)
+
+
1
2
L
x
t
.
This result is generalized to:
Theorem 9.4.2.2 Let f be a convex function and X be a real-valued semi-
martingale. Then, f(X) is a semi-martingale and
f(X
t
)=f(X
0
)+
]0,t]
f
(X
s
−
) dX
s
+
1
2
∞
−∞
f
(dx)L
x
t
+ A
t
where f
is the left derivative of f and A is an adapted purely discontinuous
increasing process. Moreover
ΔA
t
= f(X
t
) − f (X
t
−
) − f
(X
t
−
)ΔX
t
.
Proof: See Meyer [647], Protter [727] Chapter IV, Section 7.
Example 9.4.2.3 Az´ema Martingale: Itcanbeproved(seeProtter[727])
that the local times at all levels except 0 of Az´ema’s martingale (see
Subsection 4.3.8 for the definition of this martingale) are 0 and that the
local time at 0 for Brownian motion coincides with the local time at 0 of the
Az´ema martingale. An important consequence is that Az´ema’s martingale
is not a semi-martingale for the Brownian filtration: Indeed, if it were,
then its (continuous) martingale part would have zero quadratic variation,
hence would be zero. Consequently, Az´ema’s martingale would have bounded
variation, which is false.

532 9 General Processes: Mathematical Facts
9.4.3 Exponential Semi-martingales
We generalize the definition of stochastic exponential introduced for bounded
variation processes in Lemma 9.1.2.3.
Definition 9.4.3.1 Let (X
t
,t ≥ 0) be a real-valued F-semi-martingale. The
stochastic differential equation
Y
t
=1+
t
0
Y
s
−
dX
s
has a unique c`adl`ag F–adapted solution denoted by (E(X)
t
,t ≥ 0),andis
called the Dol´eans-Dade exponential of X:
E(X)
t
=exp
X
t
− X
0
−
1
2
X
c
,X
c
t
s≤t
(1 + ΔX
s
)e
−ΔX
s
.
The process E(X) has strictly positive values if and only if ΔX
s
> −1for
all s.IfΔX
s
≥−1, ∀s and τ =inf{t : ΔX
t
= −1}, the exponential is equal
to zero after τ, and is strictly positive before τ.
If X is a local martingale and ΔX
s
> −1, the process E(X)isapositive
local martingale and, therefore, a super-martingale and converges when t goes
to infinity, to some random variable E(X)
∞
with E(E(X)
∞
) ≤ 1. It is a
martingale if and only if its expectation is 1, i.e., E(E(X)
t
) = 1 for all t.
The process E(X) is a uniformly integrable martingale if and only if
E(E(X)
∞
)=1.
The pure jump process
s≤t
(1 + ΔX
s
)e
−ΔX
s
has finite variation.
A condition which ensures the uniform integrability of E(X)isΔX > −1
and
E
exp
1
2
X
c
∞
t
(1 + ΔX
t
)exp
−
ΔX
t
1+ΔX
t
< ∞.
(see L´epingle and M´emin [580]). If the martingale X is continuous, the usual
formula is obtained: the process
Y
t
=exp
X
t
− X
0
−
1
2
X
t
is the solution of dY
t
= Y
t
dX
t
,Y
0
=1. If X and Y are semi-martingales, then
E(X)E(Y )=E(X + Y +[X, Y ]) .
If the martingale X is BMO (i.e., if sup
τ∈T
E([X]
∞
− [X]
τ
−
) <mwhere
T is the set of stopping times) and if ΔX > 1 − δ, with 0 <δ<∞,then
E(X) is a martingale. (See Dol´eans-Dade and Meyer [257]).
The mapping X →E(X)=Z can be inverted under some assumptions
on Z. As Choulli et al. [181], Kallsen and Shiryaev [508, 509] and Jamshidian
9.4 Itˆo’s Formula and Girsanov’s Theorem 533
[475], we call its reciprocal function the stochastic logarithm:ifZ is a
semi-martingale such that Z and Z
−
are R \{0}-valued, there exists a unique
semi-martingale X, denoted L(Z) such that X
0
=0andZ = Z
0
E(X). Indeed,
from
Z
t
= Z
0
+
t
0
Z
s−
dX
s
,
we get X
t
=
t
0
1
Z
s−
dZ
s
.
In particular, any semi-martingale of the form S = e
X
where X is a semi-
martingale can be written as a stochastic exponential S = E(
˜
X)where
˜
X
t
= L(S)
t
= X
t
+
1
2
X
c
t
+
s≤t
(e
ΔX
s
− 1 − ΔX
s
) .
Remark 9.4.3.2 Symbolic Calculus on Semi-martingales. If f is a
smooth function, we denote F (x)=
x
0
dyf(y). If X is a given semi martingale,
one may define, in a similar way, the process F(X)
t
as
F(X)
t
=
t
0
f(X
s−
)dX
s
.
Setting Y
t
= F(X)
t
,iff(X
s
)andf (X
s−
) do not vanish, we can invert the
mapping as
X
t
= x +
t
0
dY
s
f(X
s−
)
this last equality being a SDE, i.e., dX
t
= ϕ(X
t
−
)dY
t
, with ϕ =1/f.The
case ϕ(x)=x leads to X
t
= E(Y )
t
and, if X
s
and X
s−
do not vanish,
Y
t
=
t
0
1
X
s−
dX
s
= L(X)
t
.
Exercise 9.4.3.3 Let Z be an R-valued semi-martingale such that Z and Z
−
do not vanish. Prove that
L(Z)
t
=ln
Z
t
Z
0
+
t
0
1
2Z
2
s−
dZ
c
s
−
s≤t
ln
Z
s
Z
s−
+1−
Z
s
Z
s−
.
Exercise 9.4.3.4 Prove that if X, Y are two semi-martingales such that their
stochastic logarithms are well defined, then
L(XY )=L(X)+L(Y )+[L(X), L(Y )] .
534 9 General Processes: Mathematical Facts
Exercise 9.4.3.5 Let X be a semi-martingale. Check that the solution of the
SDE dS
t
= S
t
−
(b(t)dt + σ(t)dX
t
)is
S
t
= S
0
exp(U
t
)Π
t
where
U
t
=
t
0
σ(s)dX
s
+
t
0
b(s)ds −
1
2
t
0
σ
2
(s)dX
c
s
,
Π
t
=
0<s≤t
(1 + σ(s)ΔX
s
)exp(−σ(s)ΔX
s
) .
9.4.4 Change of Probability, Girsanov’s Theorem
Let Q be equivalent to P on F
t
, for all t and Q|
F
t
= L
t
P|
F
t
where L is a strictly
positive P-martingale. Any P-local martingale X is a Q semi-martingale and
the semi-martingale decompositions are given by the following theorem:
Theorem 9.4.4.1 Let X be a local martingale with respect to P.Then,
(i)
X
t
−
t
0
d[X, L]
s
L
s
is a Q-local martingale.
(9.4.2)
(ii) If [X, L] is P-locally integrable (which implies that X, L exists)
the process
X
t
−
t
0
dX, L
s
L
s
−
is a Q-local martingale.
(9.4.3)
We may call the process in (9.4.2) the optional Girsanov’s transform of X
and the process in (9.4.3) the predictable Girsanov’s transform of X.
Proof: (i) Using Corollary 9.3.7.2 and setting Z
t
= X
t
−
t
0
d[X, L]
s
L
s
,the
integration by parts formula yields
Z
t
L
t
mart
= L
t
X
t
−
t
0
d[X, L]
s
mart
=0,
where
mart
= refers to P.
9.4 Itˆo’s Formula and Girsanov’s Theorem 535
(ii) Note that A
t
:=
t
0
dX,L
s
L
s
−
is a predictable process with bounded
variation. The process Y
t
= X
t
−
t
0
dX,L
s
L
s
−
is a Q-local martingale if and only
if the process YL is a P-local martingale. The integration by parts formula
leads to
Y
t
L
t
= Y
0
L
0
+
t
0
Y
s−
dL
s
+
t
0
L
s−
dY
s
+[Y,L]
t
mart
= −
t
0
L
s−
dA
s
+[Y,L]
t
= −
t
0
L
s−
dA
s
+[X, L]
t
− [A, L]
t
mart
= −X, L
t
+[X, L]
t
− Σ
s≤t
ΔA
s
ΔL
s
.
The difference −X, L
t
+[X, L]
t
and the sum
s≤t
ΔA
s
ΔL
s
=
t
0
ΔA
s
dL
s
are local martingales. (Note that ΔA is predictable.)
As a check, we prove directly the following consequence of (i) and (ii), i.e.,
the process
t
0
d[X, L]
s
L
s
−
t
0
dX, L
s
L
s−
is a Q-local martingale (in other terms, under Q, the predictable compensator
of
t
0
d[X, L]
s
L
s
is
t
0
dX, L
s
L
s−
). Let h be any predictable bounded process.
Let us verify that
E
Q
t
0
h
s
d[X, L]
s
L
s
= E
Q
t
0
h
s
dX, L
s
L
s−
.
The left-hand side is equal to:
E
P
L
t
t
0
h
s
d[X, L]
s
L
s
= E
P
t
0
h
s
d[X, L]
s
.
From (9.3.5), the right-hand side is
E
P
L
t
t
0
h
s
dX, L
s
L
s−
= E
P
t
0
h
s
dX, L
s
,
hence, the equality between the left- and the right-hand sides. The following
exercise amplifies the previous argument:
Exercise 9.4.4.2 Let A be an increasing process, with Q and P as above.
Prove that the Q-compensator of
t
0
dA
s
L
s
is
t
0
dA
p,P
s
L
s−
,whereA
p,P
is the P-
compensator of A.
Hint: Let H be a positive predictable process. Then,
536 9 General Processes: Mathematical Facts
E
Q
t
0
H
s
dA
s
L
s
= E
P
L
t
t
0
H
s
dA
s
L
s
= E
P
t
0
H
s
dA
s
= E
P
t
0
H
s
dA
p,P
s
.
Comment 9.4.4.3 It is worth remarking that any Q-local martingale may
be obtained as a Girsanov transform of a P-local martingale. Indeed, let M be
a Q-local martingale such that M
0
=0.WritingM = N/L where N = ML
is a P-local martingale, we obtain
N
t
L
t
=
t
0
1
L
s−
dN
s
+
t
0
N
s−
d(1/L
s
)+[N,L
−1
]
t
.
We now assume for simplicity that L and N are continuous and leave the
general case to the reader. From the three equalities
d
1
L
t
= −
dL
t
L
2
t
+
dL
t
L
3
t
,
t
0
N
s
d(1/L
s
)=−
t
0
N
s
L
2
s
dL
s
+
t
0
N
s
L
3
s
dL
s
,
N,L
−1
t
= −
t
0
1
L
2
s
dN,L
s
,
we obtain
M
t
=
t
0
1
L
s
dN
s
−
dN,L
s
L
s
−
t
0
N
s
L
2
s
dL
s
−
dL
s
L
s
.
This last formula can be written as
M
t
= X
t
−
t
0
dX, L
s
L
s
where X is the P-local martingale
X
t
=
t
0
L
s
dN
s
− N
s
dL
s
L
2
s
.
It follows that M is obtained from N through a Girsanov’s transformation.
Example 9.4.4.4 Let Q be locally equivalent to the Wiener measure (on F
t
)
and let X be the coordinate process. The process
˜
X
t
:= X
t
−
t
0
dX,L
s
L
s
is
a Brownian motion which generates the (Q, F)-local martingales: any (Q, F)-
local martingale can be represented as a stochastic integral w.r.t.
X.This
fact is widely used in finance: the model is often written under the historical
probability measure, and the representation theorem is written under the risk-
neutral probability measure. This discussion is related to that in Subsection
5.8.1 about strong and weak Brownian filtrations.
9.5 Existence and Uniqueness of the e.m.m. 537
Corollary 9.4.4.5 For ζ a P-local martingale, if Q = E(ζ) P,andifX is a
P-local martingale such that X, ζ exists, then
X = X −X, ζ is a Q-local
martingale.
Proof: Indeed, in that case, L
t
= E(ζ)
t
satisfies dL
t
= L
t
−
dζ
t
, hence
dX, L
t
= L
t
−
dX, ζ
t
.
Example 9.4.4.6 Let W be a Brownian motion and let M
t
= N
t
−
t
0
λ(s)ds
be the compensated martingale associated with an inhomogeneous Poisson
process. Let L be the Radon-Nikod´ym density defined as the solution to
dL
t
= L
t
−
[ψ
t
dW
t
+ γ
t
dM
t
] ,L
0
=1
where (ψ,γ) are predictable processes, and Q|
F
t
= L
t
P|
F
t
. We assume here
that L is a strictly positive martingale. The process W
ψ
defined as
W
ψ
t
:=W
t
−
t
0
ψ
s
ds
is a Q-Brownian motion and
M
γ
t
:=M
t
−
t
0
λ(s)γ
s
ds = N
t
−
t
0
λ(s)(1 + γ
s
)ds
is a Q-local martingale.
9.5 Existence and Uniqueness of the e.m.m.
Let S be a c`adl`ag adapted process on a filtered probability space (Ω,F, F, P) .
This process represents the price of some financial asset. We restrict our study
to the case of a finite horizon T , and we assume that there exists another asset,
the savings account with constant value (in other words, the interest rate is
null).
9.5.1 Predictable Representation Property
We recall the definition given in Chapter 1:
Definition 9.5.1.1 The process (S
t
, 0 ≤ t ≤ T ) admits an F-equivalent
martingale measure (e.m.m.) if there exists a probability measure Q on F
T
,
equivalent to P, such that the process (S
t
,t≤ T ) is a Q-F-local martingale.
We denote by M
P
(S) the set of e.m.m’s, i.e., the set of probabilities
equivalent to P such that S is a Q-local martingale. Of particular importance,
is the case when M
P
(S) is a singleton.

538 9 General Processes: Mathematical Facts
Definition 9.5.1.2 Let S be an (F, P)-semi-martingale and Q ∈M
P
(S).
The process S enjoys the F-predictable representation property (PRP) under
Q if any (Q, F)-local martingale M admits a representation of the form
M
t
= m +
t
0
m
s
dS
s
,t≤ T
where m is a constant and (m
s
,s≤ T ) is F-predictable.
We do not assume that F is the natural filtration of S.
Comment 9.5.1.3 In this section, our aim is to give some important results
on e.m.m’s. In finance, the existence of an e.m.m. is linked with the property
of absence of arbitrage. We do not give a full discussion of this link for which
the reader can refer to the papers of Stricker [809, 808] and Kabanov [500].
The books of Bj¨ork [102] and Steele [806] contain useful comments and the
book of Delbaen and Schachermayer [236] is an exhaustive presentation of
arbitrage theory.
9.5.2 Necessary Conditions for Existence
For Q ∈M
P
(S)wedenoteby(Λ
t
,t≤ T ) the right-continuous version of the
restriction to the σ-algebra F
t
of the Radon-Nikod´ym density of P|
F
t
with
respect to Q|
F
t
defined as P|
F
t
= Λ
t
Q|
F
t
.Weprovethat,ifS is a continuous
process and if M
P
(S) is non-empty, then S is a semi-martingale and that its
finite variation part is absolutely continuous with respect to the bracket of the
semi-martingale S, this last property being called the structure condition.
Theorem 9.5.2.1 Structure condition: If S is a continuous process and
if the set M
P
(S) is non-empty, then S is a P-semi-martingale with decom-
position S = M + A such that the finite variation process A is absolutely
continuous with respect to the bracket S, i.e., there exists a process H such
that A
t
=
t
0
H
s
dS
s
. Moreover, the integrability condition
t
0
H
2
s
dS
s
< ∞ (9.5.1)
holds.
Proof: Let S be a continuous process and Q ∈M
P
(S). The process Λ,
defined as P|
F
t
= Λ
t
Q|
F
t
, is a strictly positive Q-martingale. The process S
is a Q-local martingale (by definition of Q), hence, from Girsanov’s theorem,
the process
S
t
:=S
t
−
t
0
dS, Λ
s
Λ
s−
is a (P, F)-local martingale. The continuity
of S implies that S, Λ = S, Λ
c
. Therefore, S
t
=
S
t
+A
t
where the processes

9.5 Existence and Uniqueness of the e.m.m. 539
S and A are continuous (A
t
=
t
0
dS, Λ
c
s
Λ
s−
), and
S is a P-local martingale.
Hence, S is a P-semi-martingale. Now, from the Kunita-Watanabe inequality,
for any process ϕ such that
T
0
ϕ
s
2
dS
s
< ∞,
T
0
|ϕ
s
||dS, Λ
c
s
|≤
T
0
ϕ
s
2
dS
s
1/2
Λ
c
1/2
T
hence the absolute continuity of dA
s
with respect to dS
s
and the existence
of H such that dA
s
= H
s
dS
s
. From a similar argument,
T
0
|ϕ
s
||H
s
||dS, Λ
c
s
|≤C
T
0
ϕ
s
2
dS
s
1/2
which implies that
T
0
H
2
s
dS, Λ
c
s
< ∞.
Comments 9.5.2.2 (a) In particular, if the continuous process S is not
a semi-martingale, there does not exist an e.m.m. Q such that S is a Q-
martingale. This remark is important in finance: it is well known that a
fractional Brownian motion (except for a BM) is not a semi-martingale
(however, see Cheridito [165] where it is proved that a sum of a BM and a fBM
may be a semi-martingale). Hence, the important problem: are there arbitrage
opportunities in a fractional Brownian motion framework? The difficulty relies
on a definition of a stochastic integral w.r.t. fractional Brownian motion. We
do not give a complete list of references, and we mention only the paper of
Coutin [201]. In [737], Rogers constructs an arbitrage, and in [202], Coviello
and Russo avoid arbitrages using forward integrals. The full discussion is still
open.
(b) The absolute continuity condition for the bounded variation part
with respect to the predictable bracket of S is a cornerstone in the papers
of Delbaen et al. [231, 232] and Mania and Tevzadze [619] while studying
the closedness in L
2
of the set of stochastic integrals, and the existence of a
minimal entropy martingale measure.
Example 9.5.2.3 Example of a Semi-martingale S of the Form M +A
with A
t
=
t
0
λ
s
dS
s
Such That M
P
(S) is Empty. Let us consider the
process:
S
t
:=B
t
+ λ
t
0
ds
s
B
s
.
The process H
s
=
B
s
s
is not square integrable (see Jeulin [493], Jeulin and
Yor [496]):
t
0
B
2
s
s
2
ds = ∞, hence the set M
P
(S) is empty. Delbaen and
Schachermayer [234] define and obtain some examples of immediate arbitrages
that is arbitrages which occur immediately after 0 (in the above example, the
lack of integrability of H
s
is in the neighborhood of 0).
