Jan Svoboda. Magnetic Techniques for the Treatment of Materials
Подождите немного. Документ загружается.

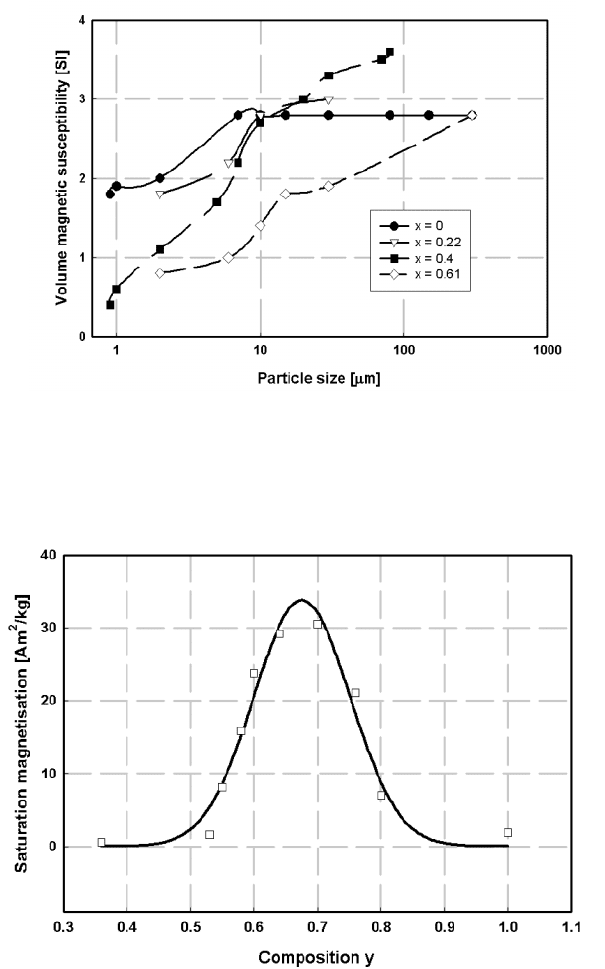
30 CHAPTER 1. PRINCIPLES OF MAGNETIC TREATMENT
Figure 1.17: Variation of the initial magnetic susceptibility, at room tempera-
ture, with grain size, for titanomagnetites (after [O1]).
Figure 1.18: Saturation magnetization of ilmenohematites as a function of their
composition (after [O2]).
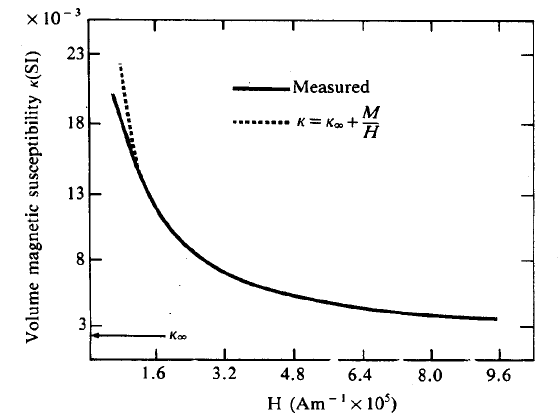
1.5. MAGNETIC PROPERTIES OF MINERALS 31
Figure 1.19: Magnetic susceptibility of hematite as a function of the applied
magnetic field (after [N2]).
1.5.5 Maghemite
The magnetic properties of maghemite (-Fe
2
O
3
) are very similar to those of
magnetite (albeit slightly less pronounced). Both minerals have fundamentally
the same spinel structure, although the composition of maghemite is essentially
that of hematite. Maghemite is metastable and converts to hematite at tem-
peratures in the range from 300
0
Cto350
0
C. The saturation polarization at
room temperature ranges from 0.48 T to 0.51 T.
1.5.6 Hematite
Pure hematite -Fe
2
O
3
has antiferromagnetic structure above the Néel temper-
ature of approximately 680
0
C. Between the Néel temperature and the Morin
temperature W
P
= - 100
0
C hematite is a canted antiferromagnet (”weak ferro-
magnet”). At room temperature, the magnetic polarization is about 0.5 % that
of magnetite, i.e. between 15×10
4
to 30×10
4
T. The weak ferromagnetism
(v=o=) of hematite is not fully understood because of its complex and contra-
dictory behaviour. The properties vary from sample to sample and depend on
their origin. For instance, the ratio of the remanent polarization to saturation
polarization M
U
@M
V
for fine hematite, ranges from close to zero to about 0.8
[A2]. The coercive force of fine hematite is very high, up to 2.5×10
5
A/m, in
contrast to the low values of the order of 8×10
2
A/m[S5,D5],observedwith
crystals, which were a few millimeters in size.
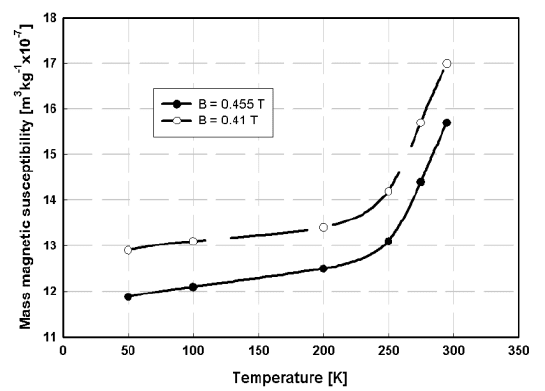
32 CHAPTER 1. PRINCIPLES OF MAGNETIC TREATMENT
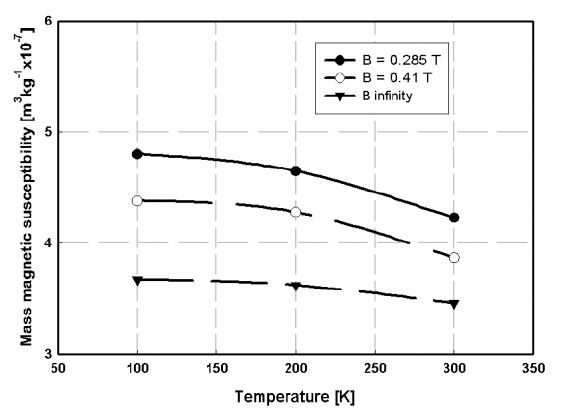
Figure 1.20: The eect of temperature and magnetic field on the magnetic sus-
ceptibility of specularite (after [P2]).
Hematite is also strongly anisotropic, i.e. crystals can be magnetized by
magnetic fields applied along the basal plane, but are almost unaected by
fields perpendicular to this plane, unless extremely strong fields are employed
[T1]. Hematite also tends to flake parallel to its basal plane and the flakes are
often termed specularite.
The magnetic susceptibility of pure hematite is a complicated function of
particle size, magnetic field, and temperature, determined by the interplay be-
tween contributions from weak ferromagnetism and antiferromagnetism [D5].
The eects of magnetic field strength and temperature on magnetic susceptibil-
ity are shown in Figs. 1.19 and 1.20, respectively.
A significant change in magnetic susceptibility for magnetic fields smaller
than 8×10
5
A/m (10 kOe) can be seen in Fig. 1.19. The field-independent
magnetic susceptibility
4
wasfoundtobeequalto1.43×10
3
(SI) [N2]. Pas-
trana and Hopstock [P2] investigated the eect of temperature and magnetic
field on magnetic susceptibility in terms of eq. (1.44). They found that
4
increased only slightly with temperature but the eect of temperature on was
dramatic, as illustrated by Fig. 1.20.
1.5.7 Goethite
Goethite (FeOOH) is an antiferromagnetic mineral, which ideally should
have perfectly compensated spins, and hence no net spontaneous magnetic mo-
ment. It appears, however, that one of the sublattices is usually canted; as
a result the mineral behaves ferrimagnetically. It has a magnetization greater
1.5. MAGNETIC PROPERTIES OF MINERALS 33
Table 1.11: Some physical properties of ferromagnetic (s.l.) minerals.
Mineral Composition Curie/Néel
temperature
[
r
C]
Saturation
polarization
[T]
Density
[kg/m
3
]
Magnetite Fe
3
O
4
575 0.59 - 0.61 5200
Maghemite Fe
2
O
3
350 0.48 - 0.51 4800
Hematite Fe
2
O
3
680 10×10
4
to
30×10
4
5300
Goethite FeOOH 120 - 130 50×10
7
to
50×10
4
4300
Pyrrhotite FeS 320 0.1 4600
Chromite FeCrO
4
-84 1.9×10
5
5090
than that of hematite but weaker than that of magnetite. Goethite also has a
high coercivity, comparable to that of hematite. The mass magnetic susceptibil-
ity ranges from 2.5×10
7
m
3
/kg to 6×10
7
m
3
/kg [A1, P2]. The temperature
and the magnetic field dependence of the magnetic susceptibility of goethite, as
reported by Pastrana and Hopstock [P2] is shown in Fig. 1.21. Physical proper-
ties of selected ferromagnetic (v=o) minerals, including goethite, are summarized
in Table 1.11.
1.5.8 Siderite
Siderite FeCO
3
undergoes an antiferromagnetic transition at the Néel tempera-
ture of about 14 K. When heated under oxidizing conditions, it decomposes into
minerals such as maghemite, magnetite and iron hydroxide. The final decompo-
sition product is hematite [B4]. Results of extensive measurements of magnetic
susceptibility of samples of siderite (- 150 + 105 m) at room temperature [P3]
show that the susceptibility does not vary with the applied magnetic field. The
susceptibility ranges from 8×10
7
m
3
/kgto11×10
7
m
3
/kg and similar values
were reported in [T1, M2].
1.5.9 Iron sulphides
Iron sulphides exhibit magnetic properties over a narrow range of specific com-
positions, but these are strongly aected by impurities, grain size and similar
factors. Pyrrhotite (FeS
1+{
for 0.10 ?{? 0.14) is the most common ferro-
magnetic (v=o=) iron sulphide. Most of these minerals are chemically unstable on
heating and the Curie temperature of pyrrhotite, 320
0
C, is almost the same as
the temperature at which is decomposes to magnetite [T1].
As in hematite, the spin structure consists of ferromagnetic order, the mag-
netic moments of adjacent planes being coupled parallel. Unlike hematite, the
moments of the planes dier in magnitude and a strong ferrimagnetism results
[O2]. Pyrrhotite is generally more strongly magnetic than hematite and the
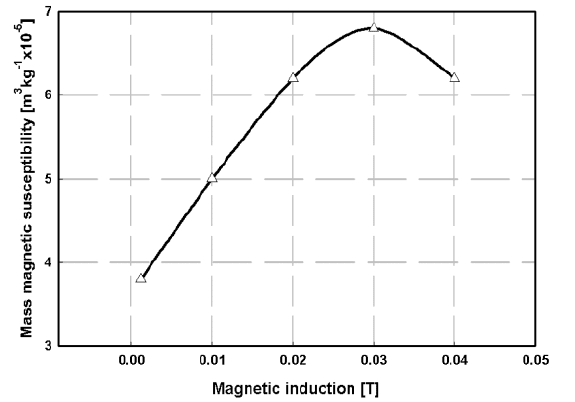
34 CHAPTER 1. PRINCIPLES OF MAGNETIC TREATMENT
Figure 1.21: Magnetic susceptibility of goethite as a function of magnetic field
strength and temperature (after [P2]).
saturation polarization, at room temperature, is about 0.12 T [O2], although
smaller values are often found in literature (e.g. [T1]). The magnetic field
dependence of the mass magnetic susceptibility is shown in Fig. 1.22 [D4].
1.5.10 Chromites
The magnetic properties of natural chromium-bearing spinels or chromites (Fe,
Mg)(Cr,Al,Fe)
2
O
4
are highly variable, the variation being closely related to
the variation in their chemical composition. It is frequently assumed that the
magnetic susceptibility and the hysteresis behaviour of chromites is controlled
by the end-member magnetite [H2, S6]. The magnetic susceptibility is reported
to range from 6×10
7
m
3
/kg to 30×10
7
m
3
/kg at 4.3 K [D2] and from 5×10
7
m
3
/kg to 12×10
7
m
3
/kg at elevated temperatures (78 and 136 K) [D2, S6].
1.6 Measurement of magnetic properties
This section briefly describes experimental techniques used for the measurement
of magnetic quantities. There are hundreds of dierent techniques and their
modifications, each having distinct merits as well as limitations. There is no
single technique which is universally applicable to all types of measurements.
There are several comprehensive sources of information [Z1, F2, B5, B6, C2]
that provide a good starting point in the pertinent literature. It is interesting
1.6. MEASUREMENT OF MAGNETIC PROPERTIES 35
Figure 1.22: Mass magnetic susceptibility of pyrrhotite as a function of the mag-
netic induction (after [D4]).
to note that while the measurement methods have remained virtually unchanged
for a long period, the equipment has been subjected to continuous development.
Practitioners as well as researchers who use magnetic techniques as a tool
for mineral beneficiation, material concentration or removal will be interested
primarily in the measurement of the magnetic field strength and its gradient
and of magnetic susceptibility. There is a wide range of measuring instruments
commercially available, some of them easily aordable and suitable for field
applications.
1.6.1 Measurement of the magnetic field strength
There are several ways of measuring the magnetic field strength or magnetic
induction. The choice of the measurement method depends on several factors.
The field strength, its non-homogeneity, as well as the required accuracy all need
to be considered. Figure 1.23 shows the accuracy of absolute measurements of
magnetic field strength for a few commonly used methods.
The fluxmeter method
This method, which is based on Faraday’s induction law, is one of the oldest, and
still very accurate, techniques of the field measurement. It consists of placing
a coil in the magnetic field so that part of that field is enclosed as a magnetic
flux through the coil area. Switching o the field or removing the coil from the
field gives rise to an induction voltage across the coil terminals. The flux is
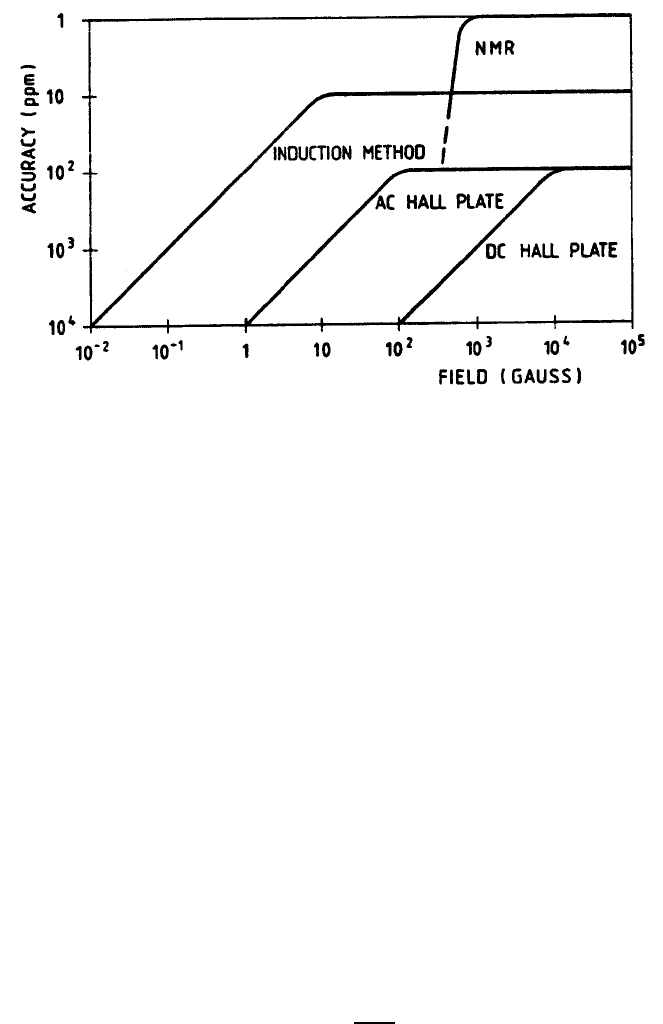
36 CHAPTER 1. PRINCIPLES OF MAGNETIC TREATMENT
Figure 1.23: Measurement of the magnetic field strength: accuracies and ranges
of various methods [H3].
then measured as the time integral of the voltage produced. Measurements are
performed either by using fixed coils in a dynamic magnetic field, or by moving
the coils in a static field.
The Hall Eect method
E.H. Hall discovered in 1879 that a metal strip immersed in a transverse mag-
netic field and carrying a current developed a voltage mutually at the right
angle to the current and the voltage that opposed the Lorentz force on the
electrons. The Hall-probe meters, known as gaussmeters, use very simple elec-
tronic instrumentation and provide an instant measurement. The small size
of the probe sensing area (1 mm
2
and less), its static physical characteristics,
as no motion of the probe is required, and there are no moving parts, make
gaussmeters very useful instruments.
The basic premise of the Hall probe is that when a current L is flowing along
one axis of a conductor or semiconductor, and when the magnetic field E is
applied perpendicularly to the plane of the probe (or generator), then the Hall
voltage X
K
given by eq. (1.46) is generated perpendicular to both the current
and the magnetic field. The situation is depicted in Fig. 1.24.
X
K
= U
K
L · E
g
(1.46)
where g is the thickness of the Hall generator and U
K
is the Hall constant.
The Hall voltage is thus, in the first approximation, proportional to the
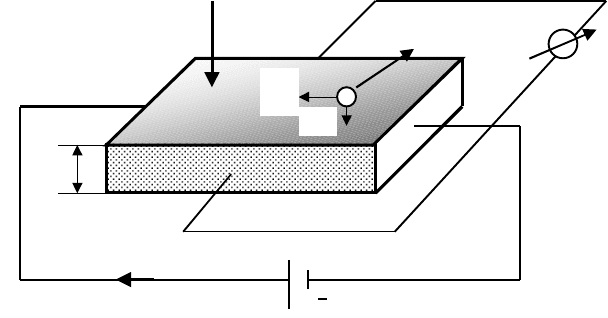
1.6. MEASUREMENT OF MAGNETIC PROPERTIES 37
Figure 1.24: Schematic representation of the Hall-eect probe.
flux density. The Hall constant depends on various material parameters as well
as temperature and, to a small extent, on the flux density E. The resulting
linearity error is kept as small as possible by applying an appropriate ohmic
resistor to the Hall voltage. This allows the construction of measuring devices
that are linear to within ±1 % for a flux density of about 1 T. Equally important
for accuracy is the eect of the temperature coe!cient of the Hall voltage.
The Hall probes, usually made using indium arsenide, are very fragile. The
Hall element is generally only a few hundredths of a millimeter thick and needs
mechanical support. Aluminium is usually used as a substrate.
The Hall probes can be used to measure d.c. fields, a.c. fields and pulsed
magnetic fields. The probes are available in various shapes and their use depends
on the direction of the magnetic field to be measured. The probes are either
transverse or axial. With the transverse probe, the flux density is measured
perpendicular to the probe rod, while with the axial probe the measured mag-
netic field is in the direction of the probe axis. The overall views of hand-held
and bench-scale gaussmeters are shown in Figs. 1.25 and 1.26.
In order to generate a high magnetic force on particles to be separated,
the magnetic field in magnetic separators is generally very non-uniform. The
value of the magnetic field thus changes considerably, often over a scale of several
hundreds of micrometers, particularly in high-gradient type magnetic separators
such as HGMS, or permanent magnet roll separators. In view of the finite size
of the Hall sensing element, it is the mean magnetic field over the relatively
large cross-sectional areas of the sensor, and not the maximum field, that is
being measured by the Hall probe. With common commercially available Hall
probes the measured magnetic field is usually much less than the maximum field.
For instance, magnetic induction usually measured in an NdFeB permanent
magnetic roll separator rarely exceeds 1 T, while a special Hall microprobe can
B
F
L
v
B
d
U
H
I
+

38 CHAPTER 1. PRINCIPLES OF MAGNETIC TREATMENT
Figure 1.25: A portable hand-held gaussmeter (courtesy of Walker LDJ Scien-
tific, Inc.).
Figure 1.26: A precision laboratory gaussmeter (courtesy of Walker LDJ Scien-
tific, Inc.).

1.6. MEASUREMENT OF MAGNETIC PROPERTIES 39
measure the field as high as 1.9 T. Such values agree with the field strength
obtained in computer modelling.
The above discussion indicates that it is rather di!cult to measure accurately
the magnetic field gradient in a magnetic separator. Although the knowledge
of the spatial distribution of the magnetic field and its profile is important for
serious understanding of the process of magnetic separation and of the design
of magnetic separators, commercial Hall probes are usually unsuitable for this
exercise. However, commercially available gaussmeters can be used for mea-
surements of the magnetic field gradient in a low-gradient class of magnetic
separators, such as magnetic pulleys and suspended magnetic separators. Ac-
curacy of the measurement can be enhanced by devising a measuring rig [S7].
1.6.2 Measurement of magnetic susceptibility and magne-
tization
All techniques for the determination of magnetic susceptibility fall into two
principal categories. The first is based on the measurement of a force that acts
on a sample when it is placed in a non-uniform magnetic field. The other method
makes use of the electromagnetic force induced in a coil when the magnetic field
changes, or when there is a relative motion of the sample and the coil.
The force methods
The most familiar of the force techniques for measurement of magnetic suscep-
tibility is the Gouy method. The sample under investigation is packed into a
long cylindrical container, which is positioned so that one end is in a strong
uniform magnetic field E
1
(near the centre of the magnet gap), while the other
is in a much weaker field E
2
, e.g. outside the gap. The magnetic force acting
on the sample is equal to
I
p
=
v
p
2
0
D(E
2
1
E
2
2
) (1.47)
where
v
and
p
are volume magnetic susceptibilities of the sample and of
the surrounding medium, respectively, and D is the cross-sectional area of the
sample. This force is detected as a weight dierential between the field-on and
field-o conditions. The principal merit of the Guoy method lies in its simplicity
- one needs only a balance, a sample container and the magnet [D6].
The Faraday method rests in the measurement of the force exerted on a
small sample by a non-homogeneous magnetic field. This can be achieved, for
instance, by the use of special pole pieces. In this case the force on the sample
can be written
I
p
=
v
p
0
YE
gE
g}
(1.48)
where } is the direction of the magnetic field gradient and Y isthevolumeof
the sample.
