Jackson S.D., Hargreaves J.S.J. Metal Oxide Catalysis

Подождите немного. Документ загружается.

254 6 Photoelectron Spectroscopy of Catalytic Oxide Materials

The chemical shift of the bulk contribution to the oxygen 1s spectrum is domi-

nated by the valence state mixing between oxygen and metal centers. The electro-

negative oxygen tends to abstract electron density from the metal centers, with

resulting high local ground state electron densities at oxygen and low binding

energies of about 528.5 eV for later transition metal systems. In early transition

metal systems, the charge redistribution is less effective, shifting the binding

energy up to 531.5 eV with a large family of binding - energy values at about 530.5 eV.

Main group elements are even less effective charge - donating partners, giving rise

to positions of up to 533 eV for water and silicon oxides. In this latter group, the

geometry of the bonding has a substantial inﬂ uence on the binding energy, making

XPS a structure - sensitive tool. In d - block metal oxides, only major changes in the

d – p rehybridization (for example in copper oxides with 529.5 eV for CuO and

530.5 eV for Cu

O) exert a signiﬁ cant inﬂ uence, otherwise the position is rather

insensitive to, for example, the formal oxidation state of metal center. Compila-

tions of such data with a chemically broad span of samples are available [17, 34,

35] .

The interpretation of the oxygen contribution of the terminating layer to the O

1s spectrum should follow different considerations. It is known that oxides termi-

nate by very strong structural relaxations [36 – 41] , making the bulk chemical

bonding of oxygen a very poor approximation of their surface. In brief, oxides ter-

minate in their most stable form by creating formal oxygen – metal double bond

groups ( “ - yl structures ” ) also for metals where such - yl structures are not known

in the form of bulk compounds. Such terminations are stable and non - reactive,

requiring defect sites for adsorption and catalysis. The nature of defects can be

the incorporation of hydroxyl groups originally thought to be the main terminating

species [42] , but many other conﬁ gurations of weakly held atomic or molecular

oxygen [40, 43 – 47] are also under debate. For the present consideration it follows

that all oxides should exhibit a low - energy contribution, situated below 530 eV for

stable terminations, and further contributions above 531 eV characterizing the

defect oxygen species, from which a discrimination between atomic species and

hydroxyl groups may be very difﬁ cult in the absence of valence band spectra, where

they yield single peak and dual peak structures that allow discrimination.

In practice, asymmetric O 1s peaks are often found for oxide systems, and these

ﬁ nd their explanation in the coexistence of a surface spectrum and a bulk spec-

trum. The contribution of the surface spectrum for polar systems and in the pres-

ence of water strongly bound via hydrogen bridges (and thus not pumped away

in a normal XPS system without thermal desorption) may then be larger than the

estimated 15% and could amount to a clearly visible structure. The contribution

of the - yl species, however, is frequently not easily detectable, as its abundance is

strictly limited to a maximum of one monolayer, being sub - stoichiometric with

respect to a close packed metal layer as it saturates two dangling coordinations per

oxygen atom.

Efforts to separate the oxygen 1s spectrum into bulk and surface components

are desirable, as the description of chemical reactivity requires a description of the

terminating oxide structure. In cases where well characterized surface - science

6.3 Case Studies 255

model compounds are available [36, 37, 48 – 54] one can justify the ﬁ tting of a broad

spectrum to different dominating and minor components, using the spectral

parameters of the model systems to account for the bulk and the - yl contributions

and assigning the unexplained part of the spectral weight to defects and adsorbate

features. In the majority of the other cases, one may use in situ thermal desorption

and/or re - oxidation as means to justify the splitting of the convoluted oxygen PES

into bulk and surface contributions. Care must be taken not to choose such severe

conditions of surface modiﬁ cation that the bulk becomes affected. This can become

a matter of considerable experimental effort, as many oxides (in particular early

transition metal oxides) do exhibit pronounced tendencies to form complex sub -

oxides [55 – 59] . It is, however, almost never right to assume one type of oxygen

species to be sufﬁ cient to describe the bulk and the surface of an oxide system.

If the separation of the spectrum into bulk and surface contributions was suc-

cessful, then an estimation of the chemical reactivity can be given using the ratio-

nale as a basis that the reactivity will be nucleophilic for low binding - energy species

and electrophilic for species with high binding energies (above 531 eV). This

assignment of nomenclature [60] refers to the reactivity of oxygen with respect to

−

H bonds, representing a very common case of the reactivity required in hydro-

carbon transformation (partial and deep oxidation). The suggestions given here

should serve as guidelines rather than as strict rules for assigning spectra. A large

number of peculiarities, such as those mentioned above, of the system under study

need to be considered, as well as the strict and conclusive elimination of binding -

energy scale artefacts already described. Only then can peak deconvolution be

made with the degree of chemical resolution required for reactivity assignments

without simply converting wishful thoughts into unresolved lines.

6.3

Case Studies

6.3.1

Applications of XPS to Vanadium Oxides

Metal oxides play an important role in many ﬁ elds of our society, one of which is

heterogeneous catalysis. These materials can be applied as carriers of an active

component, and also as the active material itself. We have selected vanadium – and

vanadium - containing – oxides as our topic, because they are involved in many cata-

lytic processes, most likely due to their versatile electronic structure. Vanadium,

with its valence atomic electron conﬁ guration of 3d

, forms different types of

oxides ranging from insulators to metal, depending on the valence shell conﬁ gura-

tion and temperature. Their electronic structure was comprehensively studied by

several research groups [50, 61 – 67] . Zimmermann and coworkers [63] demon-

strated a strong hybridization between the V 3d and O 2p orbitals, and owing to

the covalency in bonding, such early transition metal oxides (e.g. V

) cannot be

considered as simple Mott – Hubbard compounds. Density - functional theory ( DTF )

256 6 Photoelectron Spectroscopy of Catalytic Oxide Materials

calculations [64, 67] conﬁ rmed the strong hybridization of the valence oxygen and

vanadium orbitals in V

, and related the distortion of the VO

octahedra to the

unique electronic structure of the conduction band. A recent DFT cluster study

[50] mimicking V

has clearly indicated that the local charges (Mulliken) of the

different cluster atoms are much smaller than formal valence charges (V

+1.4

; O

− 0.26

− 0.58

, O

− 0.78

for the three different lattice O positions), in line with the suggested

covalent bonding contribution. DFT calculations on other oxides reported similar

discrepancies between partial and formal charges [68 – 71] . It is well known among

quantum chemists, and we would also stress, that although Mulliken charges and

formal valence charges may yield the same qualitative picture, they cannot be

compared on a quantitative basis. Formal valence charges may be useful in certain

cases, but if considered as a universal tool, they can easily lead to erroneous con-

clusions and reaction models, as often observed in the heterogeneous catalysis

community. In what follows, we will use integer valence charges (e.g. V

) to rep-

resent formal oxidation states, while fractional numbers (e.g. V

+1.4

) will indicate

local charges calculated by DFT methods.

The electronic structure of vanadium oxides is crucial to their reactivity. Deter-

mination of even the formal oxidation state of vanadium from XPS can, however,

be non - trivial, owing to a variety of factors. Firstly, examination of binary vanadium

oxides has highlighted that there appear to be differences in binding - energy posi-

tions and FWHM for single crystal and powder samples (see Table 6.1 ). In general,

the binding energy quoted for V

is quite consistent, ranging from 516.9 to

517.2 eV. However, Table 6.1 shows that the ranges of energies given for V

and

in the literature are considerably wider, and in some cases overlap. This can

be for a number of reasons, including variations in equipment/analyzer, surface

cleaning method, background and satellite subtraction and subsequent calibration

of the binding - energy scale. A relatively reliable binding - energy calibration method,

at least in the case of reduced oxides, may be to use the band gap transition of the

V 3d peak in the valence band [63] . Additionally, the widths of V 2p core levels

increase from V

to open valence VO

and to V

. This behavior is due to the

increasing number of available multiplet conﬁ gurations in the corresponding

photoelectron ﬁ nal states, that is, non - resolved multiplet splitting occurs in V 2p

core levels of lower valence vanadium oxides. This effect is also observed in the

mixed valence vanadium oxides such as V

2 n

5 n − 2

, Wadsley phase) or V

and V

2 n − 1

, Magn é li phase). An XPS study of these phases [74] has shown

similarities to the studies of V

, VO

and V

, where strong hybridization

between the O 2p and V 3d states is observed. The broad nature of the V 2p peak

of the mixed oxide phases was attributed to the mixture of oxidation states present

as well as to surface defects.

Further difﬁ culties in measuring vanadium oxides arise from reliable prepara-

tion of reference compounds and reduction of vanadium caused by UHV condi-

tions and beam damage [78, 79] . Hence, it is advantageous to conﬁ rm the phases

present by additional analytical techniques.

As expected, the difﬁ culties in relating binding energies to formal oxidation

states of vanadium becomes more complex in the case of supported vanadium

6.3 Case Studies 257

oxide catalysts (see Table 6.2 ). Again, accurate calibration of the binding - energy

scale is essential as, in the case of the catalysts shown, there is a difference of

∼ 0.5 eV in the calibration energy chosen for the C 1s peak alone. As the catalyst

support is normally an insulating material, charge compensation is made by cali-

brating the binding - energy scale to a known value. In many cases the choice of

value (support peak or carbon) can cause discrepancies between quoted binding

energy values. Achieving an accurate peak ﬁ t of the V 2p

3/2

region ideally requires

prior knowledge of the position and FWHM of each oxidation state, as determined

from reference compounds. However, Tables 6.1 and 6.2 highlight the difﬁ culties

surrounding this simple strategy.

Perhaps the starting place for investigations of supported metal oxide catalysts

by XPS should be the examination of well characterized model systems. Several

authors have investigated V

ﬁ lms supported on a number of metal substrates

Table 6.1 XPS binding energy positions of a selection of V

materials from literature.

Sample Binding Energy of V2p

3/2

and O1s/eV (FWHM)

Calibration Reference

O1s

on V foil 516.9 515.8 – – – Au 4f

7/2

[72]

(001) on

W(100) and

Au(111)

517.15 – 515.15 –

∼ 530

W 4f

7/2

[52]

on V 517.2 515.8 515.2 512.4 – Au 4f

7/2

84.0 eV [73]

Single crystals

, VO

, V (foil)

516.9 (1.6) 516.2 (3.2) 515.7 (4.2) 512.4 (2.0) 529.8 – 530.1 F.E. [61]

, VO

, V

517.2 (1.2) 516 (1.95) 515.85 512.2 – C 1s 285 eV [74]

Powders

, VO

, V

(foil)

516.4 (3.0) 516.1 (3.4) – 512.7 529.8 – 530.0 C 1s 284.6 eV [75]

(s.c.),

, VO

517.0 (1.3) 515.65 (4.0) 515.1 (4.8) – 529.8 – 530.0 O 1s

529.8/530 eV

[76]

, VO

517.2 516.0 514.0 – – C 1s 284.8 eV [77]

a FWHM in brackets if given in text.

258 6 Photoelectron Spectroscopy of Catalytic Oxide Materials

[36, 52, 72, 73, 88, 89] . The beneﬁ ts of such studies are that in the case of insulat-

ing oxides, charging is reduced owing to the enhanced conductivity of the thin

ﬁ lm in contact with a metal substrate. Nevertheless, even XPS spectra from model

systems can prove challenging to interpret. It has been reported that satellite peaks

from V 2p may have to be taken into account when ﬁ tting peaks to the V 2p enve-

lope [52, 63] . Dupuis and coworkers [52] investigated V

on W(110) and used

two peaks in their description of the V 2p

3/2

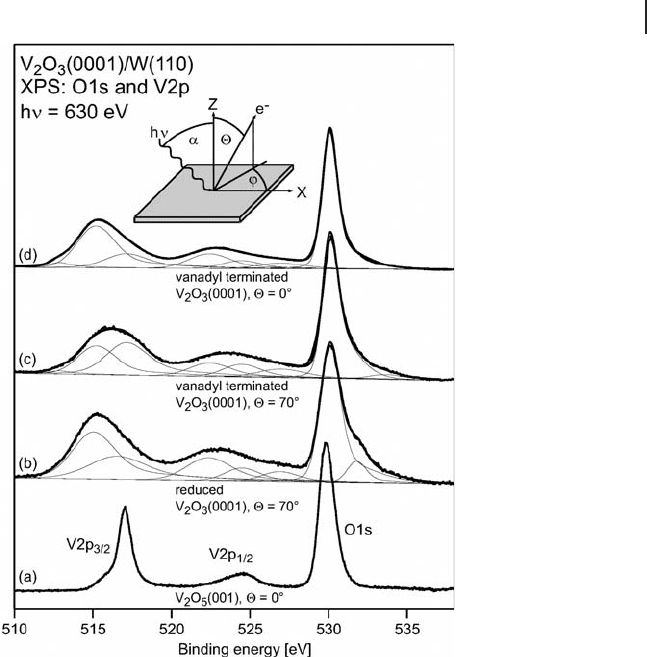
curve (Figure 6.2 ). They attributed the

higher energy feature (517.5 eV) to vanadyl groups with a formal oxidation state

of 5+ and the lower peak (515.15 eV) to bulk V

. This assignment was aided by

angle - resolved depth proﬁ ling, which showed an enhancement of the peak attrib-

uted to vanadyl groups closer to the surface. Although the peak position is in good

agreement with that of V

, the FWHM is considerably broader than that of the

crystal shown for comparison [52] . Additionally, broadening of the O 1s peak

Table 6.2 XPS binding energy positions of deconvoluted V 2p

3/2

peaks of V

/support catalysts from literature

Sample Binding energy of V2p

3/2

and O1s (eV) (FWHM)

Calibration Reference

V? O1s

/ γ - Al

517.2 –

517.3

– 515.5 –

515.8

Al 2p 74.5 eV

[80]

, V - Al - O

catalyst

517.0 515.75 515.2

∼ 531

C 1s 285 eV

[81]

VPO,

/ γ - Al

518.0 516.9 –

< 3+

515.1

C 1 s

284.5 eV,

Al 2p 74.5 eV

[79]

/ γ - Al

518.0 516.9 – V 2p of

VOPO

518 eV

[82]

/Al

517.4 – 517.6 516.4 – 516.5 515.8 – 515.9 Al 2p 74.5 eV

[83]

/Al

- ZrO

518.1 – 2

(1.8 – 2.0)

– – 531.8 – 532 C 1s 284.6 eV

[84]

/SBA - 15 517.3 (2.1) – 515.9 (2.1)

518.7

(2.1)

– Si 2p

103.6 eV

[85, 86]

/SBA - 15 517.1 516.1 – 532.8 – 533.0 C 1s 284.6

[87]

a FWHM in brackets if given in text.

b Eberhardt and coworkers used principal component analysis and iterative transformation factor

analysis to determine number of components and position.

c High E

caused by ﬁ nal state effects; due to presence of small conducting particles on insulating

substrate (see references for further details).

6.3 Case Studies 259

was observed, which may be due to the formation of hydroxyl groups. Dupuis and

colleagues suggested that in addition to oxidation state, screening effects can have

an inﬂ uence on the observed binding energy, the effect of which is greater for

bulk atoms. The same group reports a correlation between intensity of the V 3d

contribution and increasing vanadium reduction [36, 52] . Studies of similar

systems [36, 88, 89] under various conditions – hydrated, reduced, oxidized –

conﬁ rm that combined analysis of the core level and valence band XPS provides

greater information about changes in the vanadium oxide ’ s surface. The presence

of a more oxidized surface of vanadium oxide ﬁ lms compared with deeper layers

was conﬁ rmed by Alov and coworkers [73] . They used XPS and detected a higher

ratio of V

and V

on the outer part of the ﬁ lm, whereas lower oxidation states

were concentrated at greater depths.

Fresh and post - reaction supported vanadium oxide catalysts have been exam-

ined by several authors (references and E

values of some examples are given in

Figure 6.2 V 2p and O 1s region of V

(0001) on W(110)

in comparison with V

according to Dupuis and coworkers

[52] . The spectra indicate that the V

(0001) surface is

terminated with vanadyl groups.

260 6 Photoelectron Spectroscopy of Catalytic Oxide Materials

Table 6.2 ). In general, XPS of “ fresh ” catalysts shows mainly oxidized vanadium

). After reduction treatments, a decrease in oxidation state is observed. However,

the extent of reduction depends on a number of factors, such as reduction tem-

perature, reducing agent and partial pressure of the reducing gas, as well as the

method used to transfer the reduced sample to the measurement chamber. Ideally,

contact with air should be minimized, or excluded if possible, to prevent re - oxida-

tion of the catalyst when the sample is transferred from a reactor to the UHV

measurement chamber. This problem can be circumvented by in situ instrumenta-

tion as discussed later.

In the following sections, we present a critical overview of the application of

X - ray photoelectron spectroscopy in vanadium - oxide related catalytic literature

(including dehydrogenation, oxidative dehydrogenation and selective oxidation

processes). Our focus will concentrate on the strengths and weaknesses of the

method and will explore what in situ experimentation can contribute to this

ﬁ eld.

6.3.2

Direct and Oxidative Dehydrogenation

Removal of hydrogen from a hydrocarbon to form its unsaturated derivative is an

important step towards the formation of chemical feedstocks. Unsaturated hydro-

carbons are often more reactive and in high demand, hence the process is of great

value within industry. An additional beneﬁ t is the formation of hydrogen, which

if separated from the products would prove to be highly valuable. Dehydrogenation

is usually an endothermic reaction, requiring high temperature and low pressure

to ensure a sufﬁ cient yield of product. The exception is for dehydrogenation of

compounds such as cyclohexane where the formation of more stable aromatic

derivatives creates more favorable thermodynamics. Fortunately, the thermody-

namics of the reaction can be enhanced by addition of oxygen (oxidative dehydro-

genation). In this case water is formed as a product, resulting in a thermodynamically

favorable reaction. The major goal within the dehydrogenation reaction is to ﬁ nd

catalysts that are highly selective to the required product while limiting carbon

deposition, which can lead to catalyst poisoning. The majority of processes for the

dehydrogenation of light alkanes use catalysts containing chromia or platinum

supported on alumina [90 – 92] . However supported vanadium oxides have also

shown applicability for the catalysis of oxidative dehydrogenation [93 – 96] and

dehydrogenation [83, 97 – 99] of light alkanes.

6.3.2.1 Selective Dehydrogenation of n - Butane over V

/Alumina

In each of the studies described so far, XPS was measured under UHV conditions.

However, previous studies of oxide catalysts [100] have shown that by using a

specially designed high - pressure in situ XPS apparatus, XP spectra can be mea-

sured under reaction conditions in the millibar pressure range. This technique

was applied to a selection of V

/ δ - alumina catalysts (1 – 8 wt% V) to determine

their electronic structure under oxidative and reaction ( n - butane) atmospheres

6.3 Case Studies 261

[101] . Initially the “ fresh ” catalysts were examined, in an atmosphere of 0.5 mbar

of oxygen at elevated temperature (623 K). From XPS, the predominant vanadium

species was V

, independently of the vanadium loading (1, 3.5 and 8 wt% V on

alumina). Typically less than 4% of reduced species was observed under these

conditions. This is in agreement with other measurements of “ fresh ” catalysts as,

for example, Harlin and coworkers [83] reported that their calcined catalyst con-

tained 100% V

, according to conventional XPS. In contrast, several authors [82]

suggest a higher ratio of reduced vanadium in fresh catalysts, but this may be due

to the absence of any oxidative pre - treatment or to damage caused by beam or

UHV conditions. By measuring XPS in an oxygen atmosphere and at elevated

temperature, as in the work of the present authors, both reduction and charging

of insulating samples are reduced compared with conventional XPS systems.

Although in our case the oxidation state remains unchanged with loading, it is

likely according to the literature [102, 103] that the structures of vanadium oxide

on the alumina surface vary greatly. By using the complementary technique of

XAS (Section 6.2.2.2 ), information about not only the oxidation state but also the

local environment of the element under investigation can be determined. The

vanadium L

edge was measured for each of the three catalysts, under the same

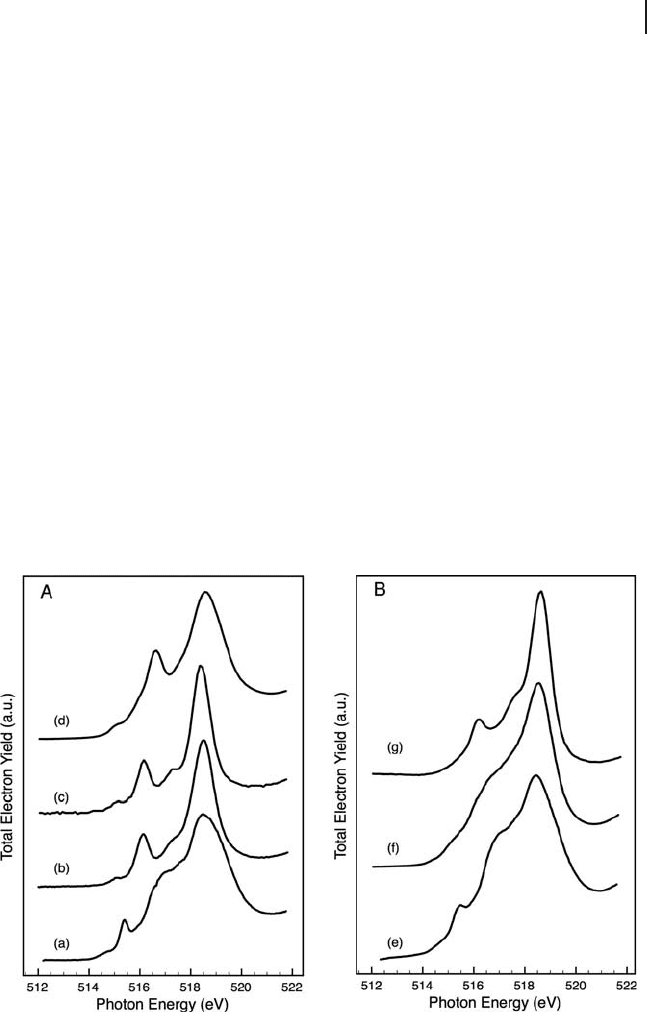
conditions as for XPS, as shown in Figure 6.3 . This edge is due to electronic

transitions from vanadium 2p

3/2

to 3d. The results were compared with a series of

Figure 6.3 V L

NEXAFS edge of (A) reference materials:

(a) V

single crystal, (b) Mg

(d) MgV

and (B) fresh catalysts: (e) 8% V/alumina, (f) 3.5% V/alumina

and (g) 1% V/alumina. All spectra were measured in an

oxygen atmosphere (0.5 mbar) at 623 K and an angle of 55 ° .

262 6 Photoelectron Spectroscopy of Catalytic Oxide Materials

reference compounds as shown in Figure 6.3 A. The spectra shown in the ﬁ gure

represent a selection of possible vanadium - oxygen linkages. Comparison of the

spectra indicate that 8% V/alumina corresponds well with the spectrum of crystal-

line V

. The low - loading catalyst (1% V/alumina) corresponds well with the

(monomeric) and the Mg

(dimeric) compounds, whereas the 3.5%

V/alumina catalyst did not appear to match any speciﬁ c reference compound.

Although a stable reference material for the polyvanadate structure was not avail-

able, from the appearance of the vanadium L

edge of the 3.5% V/alumina catalyst,

it is likely that a mixture of species was present, possibly including V

crystallites

and polyvanadates as suggested from (UV - )Raman spectroscopy of the same cata-

lysts [103] . Owing to a high degree of complexity, theoretical calculations to deﬁ ne

the origin of the features of the vanadium L

2,3

edge are limited. De Fransco and

coworkers [104] have presented a density - functional investigation of V

. Although

able to calculate theoretical absorption K edges that are similar to experimental

results, vanadium L - edge calculations still contained discrepancies from experi-

ment. In different studies [105, 106] , ﬁ rst principles multi - electron calculations of

and V

were found to ﬁ t well to experimental ﬁ ndings, although there was

still some disagreement with the V

system. Therefore, detailed calculations of

the vanadium L edge of magnesium vanadate compounds would be necessary

to relate the ﬁ ne structure of the electronic transitions to the structure of the

compounds.

Additionally, the catalysts were examined under reaction conditions. The results

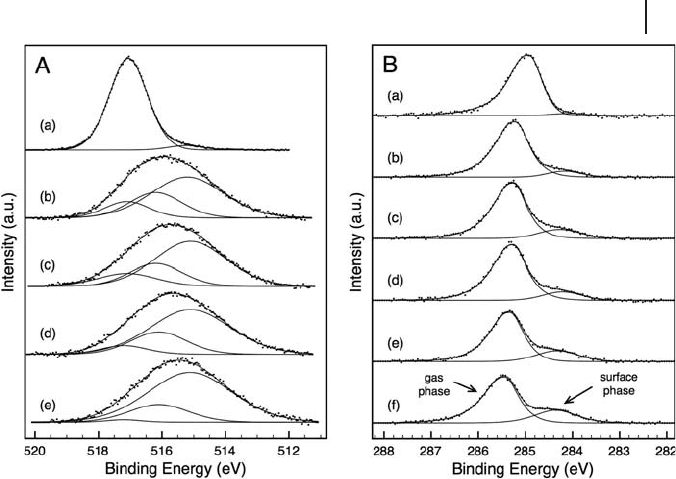
of XPS analysis of an 8% V/alumina catalyst under n - butane dehydrogenation

conditions can be seen in Figure 6.4 . During the dehydrogenation reaction in n -

butane (0.4 mbar) and at a reaction temperature of 723 K, the formal oxidation state

of vanadium was greatly reduced from the initial mixed oxidation state of 5+/4+

after O

pre - treatment. The broadening of the V 2p

3/2

peak and the position of

the lower binding energy component is in agreement with the ﬁ ndings of Dupuis

and coworkers [52] for V

. One group reports the presence of only V

and V

a vanadium/alumina catalyst as determined by statistical methods [80] . In that

study , both the number of major components and their peak position were deter-

mined by principal component analysis and iterative transformation factor analy-

sis. However, as shown in Tables 6.1 and 6.2 , various authors report a value in

between that of V

and V

, which they assign to VO

or a V

species. In our case

it is reasonable to ﬁ t the V 2p envelope with three peaks representing V

, V

and

(see Figure 6.4 A). Adsorption studies of probe molecules (e.g. NO or CO) also

suggest the presence of vanadium species with formal oxidation states of 3, 4 and

5+ [107, 108] . This is in agreement with the work of Harlin and coworkers [83] ,

who proposed that the active center for the dehydrogenation reaction (of n - butane

and i - butane at 853 K) is as a result of surface vacancies due to V

and V

at the

catalyst surface. They also suggest that reduction of vanadium is due to reaction

not only with the feedstock but also with the reaction products (butenes, butadiene

and with small amounts of cracked products). In addition to surface reduction,

these lines broaden with lower oxidation state, owing to multiplet splitting.

However, it can also be seen that the FWHM of the 5+ component increases. This

6.3 Case Studies 263

effect can be seen in the V 2p ﬁ ttings of Dupuis and coworkers [52] , hence it is

not an effect restricted to powder samples. Hess and coworkers [85, 86] observed

an additional feature in their XPS of V

/SBA - 15 samples, at even higher binding

energy than expected for V

. This was attributed to charging in the ﬁ nal state by

the presence of small particles on an insulating support. Hence, the broadening

of the V

contribution may have a ﬁ nal state origin or it could be due to the pres-

ence of neighboring reduced atoms.

Concomitantly to the XPS measurements, on - line mass spectrometry shows the

main products as butenes, butadiene and, to a lesser extent, benzene. Although

this study was performed at reduced pressure, reaction products were similar to

those found in high - pressure (1 bar) studies [83, 97] . (Previous studies of n - butane

dehydrogenation have also detected benzene in the product stream [83] .) The

combination of low partial pressure of feed with parallel surface monitoring pro-

vides a unique tool to follow the processes occurring during the initial period of

reaction/deactivation in “ slow - motion. ” Indeed, even under our low - pressure con-

ditions, deactivation of the catalyst and deposition of carbon were observed. Owing

to these processes, regeneration cycles were performed by treating the catalyst ﬁ rst

in oxygen then in hydrogen at 723 K. After each regeneration, the activity of the

Figure 6.4 (A) XP spectra of V 2p

3/2

region of 8% V/alumina

in (a) 0.5 mbar oxygen and 0.4 mbar n - butane (723 K),

after (b) 53 min, (c) 87 min, (d) 129 min and (e) 186 min.

(B) XPS of C1s region during reaction in n - butane (0.4 mbar,

723 K) after (a) 60 min, (b) 96 min, (c) 114 min, (d) 118 min,

(e) 134 min and (f) 174 min in the reaction mixture.