Hirsch M.J., Pardalos P.M., Murphey R. Dynamics of Information Systems: Theory and Applications
Подождите немного. Документ загружается.


56 K.D. Pham
continuous-time composite state matrix F
ai
(s) is exponentially stable on [t
0
,t
f
].
According to the results in [1], the state transition matrix, Φ
ai
(s, t
0
) associated with
the continuous-time composite state matrix F
ai
(s) has the following properties
d
ds
Φ
ai
(s, t
0
) =F
ai
(s)Φ
ai
(s, t
0
); Φ
ai
(t
0
,t
0
) =I
lim
t
f
→∞
Φ
ai
(t
f
,σ)
=0; lim
t
f
→∞
t
f
t
0
Φ
ai
(t
f
,σ)
2
dσ < ∞.
By the matrix variation of constant formula, the unique and time-continuous solu-
tions to the time-backward matrix differential equations (3.25)–(3.26) together with
the terminal-value conditions are then written as follows
H
i
(s, 1) = Φ
T
ai
(t
f
,s)Q
f
ai
Φ
ai
(t
f
,s)+
t
f
s
Φ
T
ai
(σ, s)N
ai
(σ )Φ
ai
(σ, s) dσ
H
i
(s, r) =
t
f
s
Φ
T
ai
(σ, s)
r−1
v=1
2r!
v!(r −v)!
H
i
(σ, v)G
ai
(σ )W
ai
G
T
ai
(σ )
×H
i
(σ, r −v)Φ
ai
(σ, s) dσ
for any s ∈[t
0
,t
f
] and 2 ≤ r ≤k
i
. It is observed that as long as the growth rate of
the integrals is not faster than the exponentially decreasing rate of two factors of
Φ
ai
(·, ·), it is therefore concluded that there exist upper bounds on the unique and
time-continuous solutions {H
i
(s, r)}
k
i
r=1
for any time interval [t
0
,t
f
]. With the exis-
tence and boundedness of the solutions of the equations (3.25) and (3.26), it is rea-
sonable to conclude that the unique and time-continuous solutions {
˘
D
i
(s, r)}
k
i
r=1
and
{D
i
(s, r)}
k
i
r=1
of the remaining linear vector- and scalar-valued equations (3.28)–
(3.30) also exist and are bounded on the time interval [t
0
,t
f
].
As for the problem statements of the control decision optimization, the results
(3.25)–(3.30) are now interpreted in terms of variables and matrices of the local
dynamical system by letting H
i
(s, r),
˘
D
i
(s, r) and G
ai
(s)W
ai
G
T
ai
(s) be partitioned
as follows
H
i
(s, r)
⎡
⎢
⎣
H
i
00
(s, r) H
i
01
(s, r) H
i
02
(s, r)
H
i
10
(s, r) H
i
11
(s, r) H
i
12
(s, r)
H
i
20
(s, r) H
i
21
(s, r) H
i
22
(s, r)
⎤
⎥
⎦
;
˘
D
i
(s, r)
⎡
⎢
⎣
˘
D
i
0
(s, r)
˘
D
i
1
(s, r)
˘
D
i
2
(s, r)
⎤
⎥
⎦
G
ai
(s)W
ai
G
T
ai
(s)
⎡
⎢
⎣
Π
i
00
(s) Π
i
01
(s) Π
i
02
(s)
Π
i
10
(s) Π
i
11
(s) Π
i
12
(s)
Π
i
20
(s) Π
i
21
(s) Π
i
22
(s)
⎤
⎥
⎦

3 Performance-Information Analysis and Distributed Feedback Stabilization 57
=
⎡
⎢
⎣
L
i
(s)V
i
L
T
i
(s) −L
i
(s)V
i
L
T
i
(s) 0
−L
i
(s)V
i
L
T
i
(s) L
i
(s)V
i
L
T
i
(s) +G
i
(s)W
i
G
T
i
(s) 0
00G
zi
(s)W
mi
G
T
zi
(s)
⎤
⎥
⎦
H
i
(s, r)G
ai
(s)W
ai
G
T
ai
(s)
⎡
⎢
⎣
P
i
00
(s) P
i
01
(s) P
i
02
(s)
P
i
10
(s) P
i
11
(s) P
i
12
(s)
P
i
20
(s) P
i
21
(s) P
i
22
(s)
⎤
⎥
⎦
whose the matrix components for agent i are defined by
P
i
00
(s) H
i
00
(s, r)Π
i
00
(s) +H
i
01
(s, r)Π
i
10
(s) +H
i
02
(s, r)Π
i
20
(s)
P
i
01
(s) H
i
00
(s, r)Π
i
01
(s) +H
i
01
(s, r)Π
i
11
(s) +H
i
02
(s, r)Π
i
21
(s)
P
i
02
(s) H
i
00
(s, r)Π
i
02
(s) +H
i
01
(s, r)Π
i
12
(s) +H
i
02
(s, r)Π
i
22
(s)
P
i
10
(s) H
i
10
(s, r)Π
i
00
(s) +H
i
11
(s, r)Π
i
10
(s) +H
i
12
(s, r)Π
i
20
(s)
P
i
11
(s) H
i
10
(s, r)Π
i
01
(s) +H
i
11
(s, r)Π
i
11
(s) +H
i
12
(s, r)Π
i
21
(s)
P
i
12
(s) H
i
10
(s, r)Π
i
02
(s) +H
i
11
(s, r)Π
i
12
(s) +H
i
12
(s, r)Π
i
22
(s)
P
i
20
(s) H
i
20
(s, r)Π
i
00
(s) +H
i
21
(s, r)Π
i
10
(s) +H
i
22
(s, r)Π
i
20
(s)
P
i
21
(s) H
i
20
(s, r)Π
i
01
(s) +H
i
21
(s, r)Π
i
11
(s) +H
i
22
(s, r)Π
i
21
(s)
P
i
22
(s) H
i
20
(s, r)Π
i
02
(s) +H
i
21
(s, r)Π
i
12
(s) +H
i
22
(s, r)Π
i
22
(s)
Then the previous result can be expanded as follows.
Theorem 3 (Performance-measure statistics) For each agent i, let the pairs
(A
i
,B
i
) and (A
i
,C
i
) be uniformly stabilizable and the pair (A
i
,H
i
) be uniformly
detectable on [t
0
,t
f
] in the autonomous system governed by (3.3) through (3.7).
Then, for any given k
i
∈Z
+
, the k
i
-th cumulant associated with (3.23) is given by
κ
i
k
i
=
x
0
i
T
H
i
00
(t
0
,k
i
)x
0
i
+2
x
0
i
T
˘
D
i
0
(t
0
,k
i
) +D
i
(t
0
,k
i
) (3.31)
where the cumulant components {H
i
00
(s, r)}
k
i
r=1
, {H
i
01
(s, r)}
k
i
r=1
, {H
i
02
(s, r)}
k
i
r=1
,
{H
i
10
(s, r)}
k
i
r=1
, {H
i
11
(s, r)}
k
i
r=1
, {H
i
12
(s, r)}
k
i
r=1
, {H
i
20
(s, r)}
k
i
r=1
, {H
i
21
(s, r)}
k
i
r=1
,
{H
i
22
(s, r)}
k
i
r=1
, {
˘
D
i
0
(s, r)}
k
i
r=1
, {
˘
D
i
1
(s, r)}
k
i
r=1
, {
˘
D
i
2
(s, r)}
k
i
r=1
and {D
i
(s, r)}
k
i
r=1
eval-
uated at s =t
0
satisfy the supporting matrix-valued differential equations
d
ds
H
i
00
(s, 1) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
H
i
00
(s, 1)
−H
i
00
(s, 1)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−K
T
i
(s)R
i
(s)K
i
(s) −Q
i
(s) −K
T
zi
(s)R
zi
(s)K
zi
(s),
58 K.D. Pham
H
i
00
(t
f
, 1) =Q
f
i
(3.32)
d
ds
H
i
01
(s, 1) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
H
i
01
(s, 1) −Q
i
(s)
−H
i
00
(s, 1)L
i
(s)H
i
(s) −H
i
01
(s, 1)
A
i
(s) −L
i
(s)H
i
(s)
,
H
i
01
(t
f
, 1) =Q
f
i
(3.33)
d
ds
H
i
02
(s, 1) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
H
i
02
(s, 1)
−H
i
02
(s, 1)A
zi
(s), H
i
02
(t
f
, 1) =0 (3.34)
d
ds
H
i
10
(s, 1) =−
L
i
(s)H
i
(s)
T
H
i
00
(s, 1) −
A
i
(s) −L
i
(s)H
i
(s)
T
H
i
10
(s, 1)
−H
i
10
(s, 1)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−Q
i
(s),
H
i
10
(t
f
, 1) =Q
f
i
(3.35)
d
ds
H
i
11
(s, 1) =−
L
i
(s)H
i
(s)
T
H
i
01
(s, 1) −
A
i
(s) −L
i
(s)H
i
(s)
T
H
i
11
(s, 1)
−H
i
10
(s, 1)L
i
(s)H
i
(s) −H
i
11
(s, 1)
A
i
(s) −L
i
(s)H
i
(s)
,
H
i
11
(t
f
, 1) =Q
f
i
(3.36)
d
ds
H
i
12
(s, 1) =−
L
i
(s)H
i
(s)
T
H
i
02
(s, 1) −
A
i
(s) −L
i
(s)H
i
(s)
T
H
i
12
(s, 1)
−H
i
12
(s, 1)A
zi
(s), H
i
12
(t
f
, 1) =0 (3.37)
d
ds
H
i
20
(s, 1) =−H
i
20
(s, 1)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−A
T
zi
(s)H
i
20
(s, 1) +2R
zi
(s)K
zi
(s), H
i
20
(t
f
, 1) =0 (3.38)
d
ds
H
i
21
(s, 1) =−A
T
zi
(s)H
i
21
(s, 1) −H
i
20
(s, 1)
L
i
(s)H
i
(s)
−H
i
21
(s, 1)
A
i
(s) −L
i
(s)H
i
(s)
,H
i
21
(t
f
, 1) =0 (3.39)
d
ds
H
i
22
(s, 1) =−A
T
zi
(s)H
i
22
(s, 1) −H
i
22
(s, 1)A
zi
(s) −R
zi
(s), H
i
22
(t
f
, 1) =0
(3.40)
d
ds
H
i
00
(s, r) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
H
i
00
(s, r)
−H
i
00
(s, r)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
00
(s)H
i
00
(s, r −v) +P
i
01
(s)H
i
01
(s, r −v)
3 Performance-Information Analysis and Distributed Feedback Stabilization 59
+P
i
02
(s)H
i
20
(s, r −v)
,H
i
00
(t
f
,r)=0, 2 ≤r ≤k
i
(3.41)
d
ds
H
i
01
(s, r) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
H
i
01
(s, r)
−H
i
00
(s, r)
L
i
(s)H
i
(s)
−H
i
01
(s, r)
A
i
(s) −L
i
(s)H
i
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
00
(s)H
i
01
(s, r −v) +P
i
01
(s)H
i
11
(s, r −v)
+P
i
02
(s)H
i
21
(s, r −v)
,H
i
01
(t
f
,r)=0, 2 ≤r ≤k
i
(3.42)
d
ds
H
i
02
(s, r) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
H
i
02
(s, r)
−
r−1
r=1
2r!
v!(r −v)!
P
i
00
(s)H
i
02
(s, r −v) +P
i
01
(s)H
i
12
(s, r −v)
+P
i
02
(s)H
i
22
(s, r −v)
−H
i
02
(s, r)A
zi
(s), H
i
02
(t
f
,r)=0,
2 ≤r ≤k
i
(3.43)
d
ds
H
i
10
(s, r) =−
L
i
(s)H
i
(s)
T
H
i
00
(s, r) −
A
i
(s) −L
i
(s)H
i
(s)
T
H
i
10
(s, r)
−H
i
10
(s, r)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
10
(s)H
i
00
(s, r −v) +P
i
11
(s)H
i
10
(s, r −v)
+P
i
12
(s)H
i
20
(s, r −v)
,H
i
10
(t
f
,r)=0, 2 ≤r ≤k
i
(3.44)
d
ds
H
i
11
(s, r) =−
L
i
(s)H
i
(s)
T
H
i
01
(s, r) −
A
i
(s) −L
i
(s)H
i
(s)
T
H
i
11
(s, r)
−H
i
10
(s, r)
L
i
(s)H
i
(s)
−H
i
11
(s, r)
A
i
(s) −L
i
(s)H
i
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
10
(s)H
i
01
(s, r −v) +P
i
11
(s)H
i
11
(s, r −v)
+P
i
12
(s)H
i
21
(s, r −v)
,H
i
11
(t
f
,r)=0, 2 ≤r ≤k
i
(3.45)
d
ds
H
i
12
(s, r) =−
L
i
(s)H
i
(s)
T
H
i
02
(s, r) −
A
i
(s) −L
i
(s)H
i
(s)
T
H
i
12
(s, r)
−
r−1
v=1
2r!
v!(r −v)!
P
i
10
(s)H
i
02
(s, r −v) +P
i
11
(s)H
i
12
(s, r −v)
+P
i
12
(s)H
i
22
(s, r −v)
−H
i
12
(s, r)A
zi
(s), H
i
12
(t
f
,r)=0,
2 ≤r ≤k
i
(3.46)
60 K.D. Pham
d
ds
H
i
20
(s, r) =−A
T
zi
(s)H
i
20
(s, r) −H
i
20
(s, r)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
20
(s)H
i
00
(s, r −v) +P
i
21
(s)H
i
10
(s, r −v)
+P
i
22
(s)H
i
20
(s, r −v)
,H
i
20
(t
f
,r)=0, 2 ≤r ≤k
i
(3.47)
d
ds
H
i
21
(s, r) =−A
T
zi
(s)H
i
21
(s, r) −H
i
20
(s, r)
L
i
(s)H
i
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
20
(s)H
i
01
(s, r −v) +P
i
21
(s)H
i
11
(s, r −v)
+P
i
22
(s)H
i
21
(s, r −v)
−H
i
21
(s, r)
A
i
(s) −L
i
(s)H
i
(s)
,
H
i
21
(t
f
,r)=0, 2 ≤r ≤k
i
(3.48)
d
ds
H
i
22
(s, r) =−A
T
zi
(s)H
i
22
(s, r) −H
i
22
(s, r)A
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
20
(s)H
i
02
(s, r −v) +P
i
21
(s)H
i
12
(s, r −v)
+P
i
22
(s)H
i
22
(s, r −v)
,H
i
22
(t
f
,r)=0, 2 ≤r ≤k
i
(3.49)
d
ds
˘
D
i
0
(s, 1) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
˘
D
i
0
(s, 1)
−H
i
00
(s, 1)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−H
i
02
(s, 1)E
zi
(s)d
mi
(s) −K
T
i
(s)R
i
(s)p
i
(s)
−K
T
zi
(s)R
zi
(s)p
zi
(s),
˘
D
i
0
(t
f
, 1) =0 (3.50)
d
ds
˘
D
i
1
(s, 1) =−
L
i
(s)H
i
(s)
T
˘
D
i
0
(s, 1) −
A
i
(s) −L
i
(s)H
i
(s)
T
˘
D
i
1
(s, 1)
−H
i
10
(s, 1)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−H
i
12
(s, 1)E
zi
(s)d
mi
(s),
˘
D
i
1
(t
f
, 1) =0 (3.51)
d
ds
˘
D
i
2
(s, 1) =−H
i
20
(s, 1)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−A
T
zi
(s)
˘
D
i
2
(s, 1) −H
i
22
(s, 1)E
zi
(s)d
mi
(s) +R
zi
(s)p
zi
(s),
˘
D
i
2
(t
f
, 1) =0 (3.52)
d
ds
˘
D
i
0
(s, r) =−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
˘
D
i
0
(s, r)
3 Performance-Information Analysis and Distributed Feedback Stabilization 61
−H
i
00
(s, r)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−H
i
02
(s, r)E
zi
(s)d
mi
(s),
˘
D
i
0
(t
f
,r)=0, 2 ≤r ≤k
i
(3.53)
d
ds
˘
D
i
1
(s, r) =−
L
i
(s)H
i
(s)
T
˘
D
i
0
(s, r) −
A
i
(s) −L
i
(s)H
i
(s)
T
˘
D
i
1
(s, r)
−H
i
10
(s, r)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−H
i
12
(s, r)E
zi
(s)d
mi
(s),
˘
D
i
1
(t
f
,r)=0, 2 ≤r ≤k
i
(3.54)
d
ds
˘
D
i
2
(s, r) =−H
i
20
(s, r)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−A
T
zi
(s)
˘
D
i
2
(s, r) −H
i
22
(s, r)E
zi
(s)d
mi
(s),
˘
D
i
2
(t
f
,r)=0,
2 ≤r ≤k
i
(3.55)
d
ds
D
i
(s, 1) =−2
˘
D
i
0
T
(s, 1)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−2
˘
D
i
2
T
(s, 1)E
zi
(s)d
mi
(s)
−Tr
H
i
00
(s, 1)Π
i
00
(s) +H
i
01
(s, 1)Π
i
10
(s) +H
i
02
(s, 1)Π
i
20
(s)
−Tr
H
i
10
(s, 1)Π
i
01
(s) +H
i
11
(s, 1)Π
i
11
(s) +H
i
12
(s, 1)Π
i
21
(s)
−Tr
H
i
20
(s, 1)Π
i
02
(s) +H
i
21
(s, 1)Π
i
12
(s) +H
i
22
(s, 1)Π
i
22
(s)
−p
T
i
(s)R
i
(s)p
i
(s) −p
T
zi
(s)R
zi
(s)p
zi
(s), D
i
(t
f
, 1) =0 (3.56)
d
ds
D
i
(s, r) =−2
˘
D
i
0
T
(s, r)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−2
˘
D
i
2
T
(s, r)E
zi
(s)d
mi
(s)
−Tr
H
i
00
(s, r)Π
i
00
(s) +H
i
01
(s, r)Π
i
10
(s)
−Tr
H
i
02
(s, r)Π
i
20
(s)
−Tr
H
i
10
(s, r)Π
i
01
(s) +H
i
11
(s, r)Π
i
11
(s)
−Tr
H
i
12
(s, r)Π
i
21
(s)
−Tr
H
i
20
(s, r)Π
i
02
(s) +H
i
21
(s, r)Π
i
12
(s)
−Tr
H
i
22
(s, r)Π
i
22
(s)
,D
i
(t
f
,r)=0, 2 ≤r ≤k
i
(3.57)
Clearly, then, the compactness offered by logic from the state-space model de-
scription (3.3) through (3.7) has been successfully combined with the quantitativity
from the a priori knowledge about probabilistic descriptions of uncertain environ-
ments. Thus, the uncertainty of performance (3.23) at agent i can now be repre-
sented in a compact and robust way. Subsequently, the time-backward differential
equations (3.32)–(3.57) not only offer a tractable procedure for the calculation of
(3.31) but also allow the incorporation of a class of linear feedback syntheses so
that agent i is actively mitigating its performance uncertainty. Such performance-
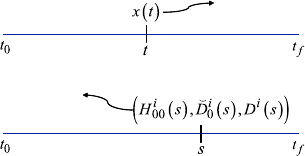
62 K.D. Pham
Fig. 3.3 The initial cost
problem (top) vs. the terminal
cost problem (bottom)
measure statistics are therefore, referred as “information” statistics which are ex-
tremely valuable for shaping the local performance distribution.
3.4 Problem Statements
Suffice it to say here that all the performance-measure statistics (3.31) depend in part
on the known initial condition x
i
(t
0
). Although different states x
i
(t) will result in
different values for “performance-to-come”, the values of (3.31) are, however, func-
tions of time-backward evolutions of the cumulant-generating variables H
i
00
(s, r),
˘
D
i
0
(s, r) and D
i
(s, r) that totally ignore all the intermediate values of x
i
(t) except at
the a priori initial system state x
i
(t
0
). This fact therefore makes this new optimiza-
tion problem in statistical control particularly unique as compared with the more
traditional dynamic programming class of investigations. In other words, the time-
backward trajectories (3.32)–(3.57) should be considered as the “new” dynamical
equations for which the resulting Mayer optimization and associated value function
in the framework of dynamic programming [4] thus depend on these “new” state
variables H
i
00
(s, r),
˘
D
i
0
(s, r) and D
i
(s, r). See Fig. 3.3 for further illustrations of
the initial cost problem in statistical control as opposed to the terminal cost problem
in classical control.
For notational simplicity, it is now convenient to denote the right members of the
cumulant-generating equations (3.32)–(3.57) as the mappings on the finite horizon
[t
0
,t
f
] with the rules of action
F
i,1
00
s,Y
i
00
;K
i
,K
zi
=−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
Y
i,1
00
(s)
−Y
i,1
00
(s)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−K
T
i
(s)R
i
(s)K
i
(s) −Q
i
(s) −K
T
zi
(s)R
zi
(s)K
zi
(s)
F
i,1
01
s,Y
i
00
,Y
i
01
;K
i
,K
zi
=−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
Y
i,1
01
(s)
−Y
i,1
00
(s)
L
i
(s)H
i
(s)
−Y
i,1
01
(s)
A
i
(s) −L
i
(s)H
i
(s)
−Q
i
(s)
F
i,1
02
s,Y
i
02
;K
i
,K
zi
=−Y
i,1
02
(s)A
zi
(s) −
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
Y
i,1
02
(s)

3 Performance-Information Analysis and Distributed Feedback Stabilization 63
F
i,1
10
s,Y
i
00
,Y
i
10
;K
i
,K
zi
=−
A
i
(s) −L
i
(s)H
i
(s)
T
Y
i,1
10
(s) −
L
i
(s)H
i
(s)
T
Y
i,1
00
(s)
−Y
i,1
10
(s)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−Q
i
(s)
F
i,1
11
s,Y
i
01
,Y
i
10
,Y
i
11
=−Y
i,1
10
(s)
L
i
(s)H
i
(s)
−Y
i,1
11
(s)
A
i
(s) −L
i
(s)H
i
(s)
−
L
i
(s)H
i
(s)
T
Y
i,1
01
(s) −
A
i
(s) −L
i
(s)H
i
(s)
T
Y
i,1
11
(s)
F
i,1
12
s,Y
i
02
,Y
i
12
=−Y
i,1
12
(s)A
zi
(s) −
L
i
(s)H
i
(s)
T
Y
i,1
02
(s)
−
A
i
(s) −L
i
(s)H
i
(s)
T
Y
i,1
12
(s)
F
i,1
20
s,Y
i
20
;K
i
,K
zi
=−Y
i,1
20
(s)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−A
T
zi
(s)Y
i,1
20
(s) +2R
zi
(s)K
zi
(s)
F
i,1
21
s,Y
i
20
,Y
i
21
=−A
T
zi
(s)Y
i,1
21
(s) −Y
i,1
20
(s)
L
i
(s)H
i
(s)
−Y
i,1
21
(s)
A
i
(s) −L
i
(s)H
i
(s)
F
i,1
22
s,Y
i
22
=−A
T
zi
(s)Y
i,1
22
(s) −Y
i,1
22
(s)A
zi
(s) −R
zi
(s)
F
i,r
00
s,Y
i
00
,Y
i
01
,Y
i
20
;K
i
,K
zi
=−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
Y
i,r
00
(s)
−Y
i,r
00
(s)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
00
(s)Y
i,r−v
00
(s) +P
i
01
(s)Y
i,r−v
01
(s) +P
i
02
(s)Y
i,r−v
20
(s)
F
i,r
01
s,Y
i
00
,Y
i
01
,Y
i
11
,Y
i
21
;K
i
,K
zi
=−Y
i,r
01
(s)
A
i
(s) −L
i
(s)H
i
(s)
−Y
i,r
00
(s)
L
i
(s)H
i
(s)
−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
Y
i,r
01
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
00
(s)Y
i,r−v
01
(s) +P
i
01
(s)Y
i,r−v
11
(s) +P
i
02
(s)Y
i,r−v
21
(s)
F
i,r
02
s,Y
i
02
,Y
i
12
,Y
i
22
;K
i
,K
zi
=−Y
i,r
02
(s)A
zi
(s) −
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
Y
i,r
02
(s)
−
r−1
r=1
2r!
v!(r −v)!
P
i
00
(s)Y
i,r−v
02
(s) +P
i
01
(s)Y
i,r−v
12
(s) +P
i
02
(s)Y
i,r−v
22
(s)

64 K.D. Pham
F
i,r
10
s,Y
i
00
,Y
i
10
,Y
i
20
;K
i
,K
zi
=−
A
i
(s) −L
i
(s)H
i
(s)
T
Y
i,r
10
(s)
−
L
i
(s)H
i
(s)
T
Y
i,r
00
(s) −Y
i,r
10
(s)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
10
(s)Y
i,r−v
00
(s) +P
i
11
(s)Y
i,r−v
10
(s) +P
i
12
(s)Y
i,r−v
20
(s)
F
i,r
11
s,Y
i
01
,Y
i
10
,Y
i
11
,Y
i
21
=−
A
i
(s) −L
i
(s)H
i
(s)
T
Y
i,r
11
(s) −
L
i
(s)H
i
(s)
T
Y
i,r
01
(s)
−Y
i,r
10
(s)
L
i
(s)H
i
(s)
−Y
i,r
11
(s)
A
i
(s) −L
i
(s)H
i
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
10
(s)Y
i,r−v
01
(s) +P
i
11
(s)Y
i,r−v
11
(s) +P
i
12
(s)Y
i,r−v
21
(s)
F
i,r
12
s,Y
i
02
,Y
i
12
,Y
i
22
=−Y
i,r
12
(s)A
zi
(s) −
L
i
(s)H
i
(s)
T
Y
i,r
02
(s) −
A
i
(s) −L
i
(s)H
i
(s)
T
Y
i,r
12
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
10
(s)Y
i,r−v
02
(s) +P
i
11
(s)Y
i,r−v
12
(s) +P
i
12
(s)Y
i,r−v
22
(s)
F
i,r
20
(s) =−A
T
zi
(s)Y
i,r
20
(s) −Y
i,r
20
(s)
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
20
(s)Y
i,r−v
00
(s) +P
i
21
(s)Y
i,r−v
10
(s)
+P
i
22
(s)Y
i,r−v
20
(s)
F
i,r
21
s,Y
i
01
,Y
i
11
,Y
i
20
,Y
i
21
=−A
T
zi
(s)Y
i,r
21
(s) −Y
i,r
20
(s)
L
i
(s)H
i
(s)
−
r−1
v=1
2r!
v!(r −v)!
P
i
20
(s)Y
i,r−v
01
(s) +P
i
21
(s)Y
i,r−v
11
(s) +P
i
22
(s)Y
i,r−v
21
(s)
−Y
i,r
21
(s)
A
i
(s) −L
i
(s)H
i
(s)
F
i,r
22
s,Y
i
02
,Y
i
12
,Y
i
22
=−A
T
zi
(s)Y
i,r
22
(s) −Y
i,r
22
(s)A
zi
(s) −
r−1
v=1
2r!
v!(r −v)!
P
i
20
(s)Y
i,r−v
02
(s)
+P
i
21
(s)Y
i,r−v
12
(s) +P
i
22
(s)Y
i,r−v
22
(s)
3 Performance-Information Analysis and Distributed Feedback Stabilization 65
˘
G
i,1
0
s,
˘
Z
i
0
,Y
i
00
,Y
i
02
;K
i
,K
zi
;p
i
,p
zi
=−Y
i,1
02
(s)E
zi
(s)d
mi
(s) −Y
i,1
00
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−K
T
zi
(s)R
zi
(s)p
zi
(s) −
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
˘
Z
i,1
0
(s)
−K
T
i
(s)R
i
(s)p
i
(s)
˘
G
i,1
1
s,Y
i
10
,Y
i
12
,
˘
Z
i
0
,
˘
Z
i
1
;p
i
,p
zi
=−
A
i
(s) −L
i
(s)H
i
(s)
T
˘
Z
i,1
1
(s)
−Y
i,1
10
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−
L
i
(s)H
i
(s)
T
˘
Z
i,1
0
(s) −Y
i,1
12
(s)E
zi
(s)d
mi
(s)
˘
G
i,1
2
s,Y
i
20
,Y
i
22
,
˘
Z
i
2
;p
i
,p
zi
=−Y
i,1
20
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−A
T
zi
(s)
˘
Z
i,1
2
(s) −Y
i,1
22
(s)E
zi
(s)d
mi
(s) +R
zi
(s)p
zi
(s)
˘
G
i,r
0
s,Y
i
00
,Y
i
02
,
˘
Z
i
0
;K
i
,K
zi
;p
i
,p
zi
=−Y
i,r
02
(s)E
zi
(s)d
mi
(s) −Y
i,r
00
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−
A
i
(s) +B
i
(s)K
i
(s) +C
i
(s)K
zi
(s)
T
˘
Z
i,r
0
(s)
˘
G
i,r
1
s,Y
i
10
,Y
i
12
,
˘
Z
i
0
,
˘
Z
i
1
;p
i
,p
zi
=−
A
i
(s) −L
i
(s)H
i
(s)
T
˘
Z
i,r
1
(s)
−Y
i,r
10
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−
L
i
(s)H
i
(s)
T
˘
Z
i,r
0
(s) −Y
i,r
12
(s)E
zi
(s)d
mi
(s)
˘
G
i,r
2
s,Y
i
20
,Y
i
22
,
˘
Z
i
2
;p
i
,p
zi
=−A
T
zi
(s)
˘
Z
i,r
2
(s) −Y
i,r
22
(s)E
zi
(s)d
mi
(s)
−Y
i,r
20
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
G
i,1
s,Y
i
00
,Y
i
01
,Y
i
02
,Y
i
10
,Y
i
11
,Y
i
12
,Y
i
20
,Y
i
21
,Y
i
22
,
˘
Z
i
0
,
˘
Z
i
2
;p
i
,p
zi
=−2
˘
Z
i,1
0
T
(s)
B
i
(s)p
i
(s) +C
i
(s)p
zi
(s) +E
i
(s)d
i
(s)
−2
˘
Z
i,1
2
T
(s)E
zi
(s)d
mi
(s)
−Tr
Y
i,1
00
(s)Π
i
00
(s) +Y
i,1
01
(s)Π
i
10
(s) +Y
i,1
02
(s)Π
i
20
(s)
−Tr
Y
i,1
10
(s)Π
i
01
(s) +Y
i,1
11
(s)Π
i
11
(s) +Y
i,1
12
(s)Π
i
21
(s)
