Hillert M. Phase Equilibria, Phase Diagrams and Phase Transformations: Their Thermodynamic Basis
Подождите немного. Документ загружается.


18.10 Mixtures of gas species 415
18.10 Mixtures of gas species
Gases dissolve in each other so readily and with so little interaction that the resulting
phase is regarded as a mixture of the component species (free atoms, molecules and
ions). In principle, it may also be regarded as a solution. We shall first discuss a mixture
of several species, each one of which forms an ideal gas when pure. Let y
k
be the fraction
of species k and thus
y
k
= 1. Let us consider a mixture of one mole of species. Each
gas will fill the complete volume V,but in line with the ideal gas concept we shall assume
that there is no interaction between them. We shall thus assume that the ideal gas law
applies to the mixture, PV
m
= RT, and that the chemical potential of a component k,µ
k
,
has the same value it would have, had it been alone in the same volume V. The pressure
of that component would then be P
k
= y
k
RT/V = y
k
P where P is the pressure of the
mixture
µ
k
= G
m
(k) =
o
G
k
(T , P
0
) + RT ln P
k
= H
REF
k
+ K
k
(T ) + RT ln P
k
= H
REF
+ K
k
(T ) + RT ln y
k
+ RT ln P,
(18.70)
and we get for the mixture
G
m
= y
i
µ
i
= y
o
i
G
i
(T , P
0
) + RTy
i
ln P
i
= H
REF
+ y
i
K
i
(T ) + RTy
i
ln y
i
+ RT ln P (18.71)
G
m
− H
REF
= y
i
K
i
(T ) + RT ln P + RTy
i
ln y
i
. (18.72)
The last term may be regarded as the contribution from the mixing of different molecules.
It is proportional to T and is thus of pure entropy character. −Ry
i
ln y
i
may be regarded
as the ideal entropy of mixing.
Foramixture of ideal gases it can be imagined that gas k actually has the pressure P
k
in the gas mixture and that the total pressure of the mixture is the sum of the pressures
of the individual gases. We have thus used
P
i
= y
i
P = P. (18.73)
P
k
is regarded as the partial pressure of gas k in the mixture. It is interesting to note
that the expression for ideal entropy of mixing appears in Eq. (18.72)asadirect result
of the model. For real gases it is no longer possible to define the partial pressure in
a strict sense but it is common to use this concept and the relation P
i
= y
i
P even
in such cases. As a first attempt to model G
m
for a mixture of real gases, one could
add the terms for slightly imperfect gases in the form y
i
L
i
P
i
which can be written
as y
2
i
L
i
P The second power of y
i
indicates that these terms describe the interaction
of molecules with other molecules of the same kind. It would be natural also to add
terms representing interactions between different kinds of molecules, y
i
y
j
L
ij
P.
We thus get
G
m
− H
REF
= y
i
K
i
(T ) + RT ln P + RTy
i
ln y
i
+ Py
i
y
j
L
ij
(18.74)

416 Methods of modelling
It is interesting to calculate what pressure, P
, the same amount of gas k would have if
it were alone in the same volume. For pure k we get
◦
G
k
− H
REF
k
= K
k
(T ) + RT ln P
+ P
L
kk
. (18.75)
The molar volume would be RT/P
+ L
kk
and the volume of the actual amount y
k
would
be
V = y
k
(RT/P
+ L
kk
). (18.76)
However, this is supposed to be equal to the molar volume of the mixture,
y
k
(RT/P
+ L
kk
) = RT/P + y
i
y
j
L
ij
. (18.77)
We thus find
1/P
= 1/y
k
P +
y
i
y
j
L
ij
− y
k
L
kk
/RTy
k
= 1/ P
k
+
y
i
y
j
L
ij
− y
k
L
kk
/RTy
k
. (18.78)
We have here expressed y
k
P through the partial pressure and it is evident that it is no
longer equal to the pressure of the same amount of pure k, unless all the interactions are
zero. The concept of partial pressure is thus less useful for real gas mixtures than for
ideal ones. The concept of fugacity is more useful. For a component k in a mixture it is
defined from
µ
k
− H
REF
= K
k
(T ) + RT ln f
k
, (18.79)
where K
k
(T) is formulated in such a way that f
k
approaches y
k
P for low P.Inorder to
evaluate the fugacity from a particular model one must first derive an expression for µ
k
from the model. From Eq. (18.74)weget
µ
k
− H
REF
= K
k
(T ) + RT ln(y
k
P) + P
2y
k
L
kj
− y
i
y
j
L
ij
, (18.80)
and for the fugacity coefficient we obtain
f
k
/P
k
= f
k
/y
k
P = exp[P(2y
k
L
kj
− y
i
y
j
L
ij
)/RT]. (18.81)
Foranideal gas mixture we get
f
k
/P
k
= f
k
/y
k
P = 1. (18.82)
A similar model based upon the Helmholtz energy is generally written as
P = RT
1/V
m
+
y
2
i
B
ii
+ y
i
y
j
B
ij
V
2
m
= RT
1/V
m
+
y
i
B
ii
+ y
i
y
j
B
ij
−
B
ii
+ B
jj
)
2
V
2
m
(18.83)
and it is often found that B
ij
− (B
ii
+ B
jj
)/2issmall.
Exercise 18.8
Examine a slightly imperfect gas with two kinds of molecules, A and B. Is there any rela-
tion between the L coefficients which would make the fugacity coefficients independent
of the composition?

18.11 Black-body radiation 417
Hint
Use Eq. (18.81). For a binary system only one composition variable is independent,
say y
B
.
Solution
ln( f
A
/P
A
) = (P/RT) · (2y
A
L
AA
+ 2y
B
L
AB
− y
2
A
L
AA
− 2y
A
y
B
L
AB
− y
2
B
L
BB
) =
(P/RT) · [L
AA
− (L
AA
+ L
BB
− 2L
AB
)y
2
B
]. We thus find that L
AA
+ L
BB
− 2L
AB
= 0
makes f
A
/P
A
independent of composition. For symmetry reasons, it also makes f
B
/P
B
independent of composition.
18.11 Black-body radiation
It is illustrative to compare the ideal gas behaviour with the properties of black-body
radiation and with an electron gas. For the former we may start from a result from
quantum mechanics saying that the energy of black-body radiation is proportional to the
volume and T
4
. The constant of proportionality, a,iscalled the Stefan constant and it
has a value of 8π
5
k
4
/15c
3
h
3
= 7.57 · 10
−16
J/m
3
K
4
,where k is Boltzmann’s constant,
c is the speed of light and h is Planck’s constant.
U = aT
4
V. (18.84)
However, in order to derive other quantities we need U(S, V). By simple manipulations
we obtain
C
V
=
∂U
∂T
V
= 4aT
3
V (18.85)
S =
T
0
C
V
T
dT =
4
3
aT
3
V ; T
3
= 3S/4aV (18.86)
U (S, V ) = a(3S/4aV)
4/3
V = (3S/4)
4/3
/(aV)
1/3
. (18.87)
We have thus derived a characteristic state function for black-body radiation and can
now calculate any thermodynamic quantity.
F = U − ST =−
1
3
aT
4
V (18.88)
P =−
∂U
∂V
S
=−(3S/4)
3/4
·
−1
3
(aV)
−4/3
=
1
3
(3S/4aV)
4/3
=
1
3
aT
4
(18.89)
PV =
1
3
aT
4
V (18.90)
G = U + PV − TS = aT
4
V +
1
3
aT
4
V −
4
3
aT
4
V = 0. (18.91)

418 Methods of modelling
The last result may seem surprising but is natural because P and T are not indepen-
dent variables in the particular case of black-body radiation. Consider a container con-
taining no matter and with its walls kept at a certain temperature T and an external
pressure P is applied, then the container will collapse if P > aT
4
/3 and all the radi-
ation will vanish. If P < aT
4
/3 then the container will expand indefinitely and new
radiation will be created with no limitation as long as the walls are kept at the same
temterature.
Exercise 18.9
Derive an expression for the isothermal compressibility of black-body radiation.
Hint
It may be easier to derive (∂ P/∂V )
T
than (∂V/∂ P)
T
.
Solution
We get κ
T
=−(1/V
m
) · (∂ V
m
/∂ P)
T
=−(1/V ) · (∂ V /∂ P)
T
from the definition. From
P = aT
4
/3weget (∂ P/∂ V )
T
= 0 and (∂V/∂ P)
T
=∞and κ
T
=∞. This is in com-
plete agreement with the last two sentences in the text.
18.12 Electron gas
The heat capacity for the electron gas in a metal is often given as
C
V
= γ
e
T. (18.92)
In real metals, the coefficient γ
e
has different values at low and high T and a single
expression cannot be used for the whole range of T. The low-T value is often about
1.4 times the high-T value. However, γ
e
is independent of T in a free electron gas. We
shall now examine the properties of such a gas. Its γ
e
value depends upon the density
which can be expressed through V
m
. According to the electron theory, γ
e
is actually
proportional to V
2/3
m
.For one mole of electrons we obtain
U
m
=
T
0
C
V
dT = U
m
(0K) + γ
e
T
2
/2 (18.93)
S
m
=
T
0
C
V
T
dT = γ
e
T (18.94)
F
m
= U
m
− TS
m
= U
m
(0K) − γ
e
T
2
/2. (18.95)

18.12 Electron gas 419
In order to derive an expression for G
m
we must calculate P from F
m
as a function of T,
V
m
. Then we must remember that the electron theory predicts that γ
e
is proportional to
V
2/3
m
and we should treat γ
e
/V
2/3
m
as a constant
F
m
= U
m
(0K) −
γ
e
/V
2/3
m
· V
2/3
m
T
2
/2 (18.96)
P =−
∂ F
m
∂V
m
T
=
1
2
γ
e
T
2
V
2/3
m
·
2
3
V
−1/3
m
=
1
3
γ
e
T
2
/V
m
(18.97)
G
m
= F
m
+ PV
m
= U
m
(0K) − γ
e
T
2
/2 + γ
e
T
2
/3 = U
m
(0K) − γ
e
T
2
/6. (18.98)
We have neglected to discuss the possible dependence of U
m
(0 K) on V
m
but that could
only contribute a T-independent term in G
m
.
If we want to apply the treatment of an electron gas to a metal we should realize
that there is a strong interaction between the electrons and the ionized atoms which
more or less eliminates the pressure of the electron gas. It is thus common to give their
contribution to G
m
as −γ
e
T
2
/2.
It should be realized that published values of γ
e
for metals refer to one mole of
atoms, and not electrons. The values of γ
e
are relatively small and its contribution to
C
V
only grows proportional to T.Even though it predominates at very low T it will
soon be negligible in comparison with the contribution from thermal vibrations of the
atoms which initially increases proportional to T
3
.However, at very high T the latter
contribution levels off at the value of 3R and the electronic contribution again becomes
important.
Exercise 18.10
Estimate the ratio of the electronic contribution to C
V
of pure Ni and the contribution
from thermal vibrations at the melting point, 1728 K, if γ
e
= 54.4 · 10
−4
J/mol K
2
.
Hint
Suppose the temperature is so high that the vibrational contribution can be approximated
as 3R and the γ
e
value may be taken as the tabulated value divided by 1.4.
Solution
(54.5 · 10
−4
/1.4)1728/3 · 8.3145 = 0.27.

19
Modelling of disorder
19.1 Introduction
In this chapter we shall model the thermodynamic effect of some physical phenomena.
In each case we shall start by defining an internal variable representing the extent of the
physical phenomenon to be discussed. We shall proceed by deriving an expression for
one of the characteristic state functions in terms of the internal variable together with a
set of external variables. The choice of characteristic state function depends upon what
set of external variables is most convenient. Then we shall calculate the equilibrium
value of the internal variable by putting the driving force for its change equal to zero.
Finally, we shall try to eliminate the internal variable by inserting the expression for its
equilibrium value in the characteristic state function.
Our derivation of an expression for the characteristic state function will usually be
based upon two separate evaluations, one concerned with the entropy due to the disorder
created by the physical phenomenon and the other concerned with what may be called
the non-configurational contribution. The entropy will be evaluated from Boltzmann’s
relation which is here preferred because it is felt that it gives a better physical insight
than the more general and elegant method of statistical thermodynamics based upon the
use of partition functions. The purpose of statistical thermodynamics is to model the
thermodynamic properties of various types of systems from statistical considerations
on the atomic level. The relation proposed by Boltzmann can be derived from such
considerations.
19.2 Thermal vacancies in a crystal
We shall start by considering vacancies in a crystal of a pure element. A convenient
internal variable would be the number of vacancies, N
Va
,inacrystal with N atoms. This
internal variable is sufficient for defining the contribution of the vacancies to the internal
energy if it is assumed that the interaction between the vacancies can be neglected. This
may be a reasonable approximation for low contents of vacancies and we shall construct
a model from this approximation.
The state with N
Va
vacancies can be realized in a number of ways by placing
the vacancies in different arrangements on the lattice sites. The number of different
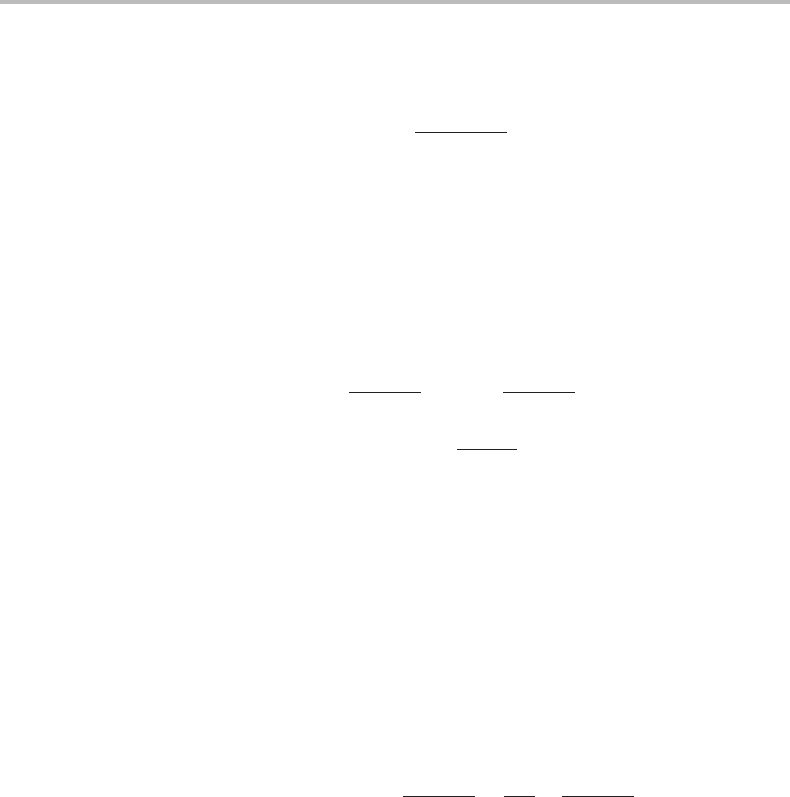
19.2 Thermal vacancies in a crystal 421
arrangements is
W =
(N + N
Va
)!
N !N
Va
!
, (19.1)
since there must be N + N
Va
lattice sites and we cannot distinguish between two atoms,
nor between two vacancies. From Boltzmann’s relation we can now calculate the contri-
bution to the entropy from the vacancies and we can use the following approximation if
we consider a system where N and N
Va
are both large numbers,
S/k = ln W = ln(N + N
Va
)! − ln N! − ln N
Va
!
∼
=
(N + N
Va
) ln(N + N
Va
) − N ln N − N
Va
ln N
Va
=−N ln
N
N + N
Va
− N
Va
ln
N
Va
N + N
Va
=−N
ln(1 − y
Va
) +
y
Va
1 − y
Va
ln y
Va
, (19.2)
where y
Va
= N
Va
/(N + N
Va
), the fraction of sites that are vacant.
When choosing a characteristic state function we should realize that it is very awkward
to treat the energy of a vacancy, u,asafunction of S
m
and V
m
or of T and V
m
because V
m
is usually defined for one mole of atoms, not lattice sites. Thus V
m
will naturally increase,
or the lattice must be compressed, when a vacancy is introduced by the creation of a new
lattice site. It is most convenient to treat u as a function of T and P and we should thus
choose G as our characteristic state function. In order to be rigorous in the derivation to
follow, we should actually introduce a Gibbs energy of formation of a vacancy, g, instead
of the energy, u, and obtain for the contribution due to N
Va
vacancies, when added to N
atoms,
G = N
Va
g + kNT
ln
N
N + N
Va
+
N
Va
N
ln
N
Va
N + N
Va
. (19.3)
The quantity g may be regarded as the non-configurational Gibbs energy per vacancy. We
have thus achieved our first goal. It is worth emphasizing that we managed to derive an
expression for G without introducing any further internal variable. Before proceeding
it is important to realize that N
Va
is in fact an internal variable because we do not need
an external reservoir from which to take vacancies. Alternatively, we may imagine that
we have an external reservoir of vacancies with a chemical potential equal to zero. We
may thus regard g as the non-configurational Gibbs energy of formation of a vacancy or
of dissolution of a vacancy from an external reservoir.
We have found an expression for the contribution to the Gibbs energy from a given
number of vacancies, N
Va
.Itcan be used to evaluate the equilibrium number of vacancies
under constant T and P.Ifthere is a mechanism, or ‘reaction’, by which vacancies can
form, then there should be a spontaneous change as long as the driving force for change
is positive. Assuming that g is independent of N
Va
under constant T, P and N,weobtain

422 Modelling of disorder
by expressing the extent of reaction with N
Va
,
− D = (∂G/∂ξ)
T,P,N
≡ (∂G/∂ N
Va
)
T,P,N
= g + kNT
−1
N + N
Va
+
1
N
ln
N
Va
N + N
Va
+
N
Va
N
·
1
N
Va
−
N
Va
N
ln
N
Va
N + N
Va
= g + kT ln
N
Va
N + N
Va
= g + kT ln y
Va
. (19.4)
By putting this equal to zero we obtain, for the equilibrium number of vacancies,
y
eq
Va
= exp(−g/kT). (19.5)
This is an expression characteristic of so-called Boltzmann statistics. It reveals how the
fraction of vacant sites varies with temperature. The higher the temperature, the larger
the fraction is. This is why such vacancies are often called thermal vacancies.
If we instead focus our interest upon the number of vacancies per mole of atoms we
obtain, for its equilibrium value,
y
eq
Va
1 − y
eq
Va
=
N
eq
Va
N
=
1
exp(g/kT) − 1
. (19.6)
This kind of expression is usually connected with the so-called Bose–Einstein statistics.
In the present case it will be possible to eliminate the internal variable at equilibrium
and obtain the contribution of thermal vacancies to the Gibbs energy,
G = N
Va
g + kT N ln
1 − y
eq
Va
+ kT N
Va
ln y
eq
Va
= N
Va
g + kT N ln
1 − y
eq
Va
+ kT N
Va
(−g/kT)
= kT N ln
1 − y
eq
Va
∼
=
−kT Ny
eq
Va
. (19.7)
Forasystem containing one mole of atoms, i.e. with N equal to Avogadro’s number, we
obtain G
m
∼
=
−RTy
eq
Va
,avalue that is negligible in most contexts.
Foratypical metal we can estimate the value of g from Eq. (19.5) using the information
that the fraction of vacant sites is between 10
−3
and 10
−4
close to the melting temperature,
T
m.p.
.Wethus obtain g/kT
m.p.
= 2.3 · (3 to 4)
∼
=
8.
The result of Eq. (19.7)issurprisingly simple. It gives the complete information on G
at equilibrium as function of T and, if one is only interested in thermodynamic properties
at equilibrium, this expression will be sufficient. On the other hand, the number of
vacancies may itself be of importance because of effects on other properties like volume
or diffusivity. The model can even be applied for evaluating G when the number of
vacancies differs from the equilibrium number. In such cases one must use the basic
equation of G as a function of T and N
Va
. One may, for instance, be interested in the
process by which the number of vacancies could approach the equilibrium value. There
are many possible mechanisms. If the actual number of vacancies is much higher than
the equilibrium value, then the driving force could be large enough for the nucleation
of a pore. If it is lower, mechanisms involving condensation on dislocations could still
operate. For such considerations it is essential to know the driving force. Eliminating g

19.3 Topological disorder 423
between Eqs (19.4) and (19.5)weobtain
D = kT ln y
eq
Va
− kT ln y
Va
=−kT ln
y
Va
/y
eq
Va
(19.8)
This is the driving force for the formation of a new vacancy. The driving force per mole
of disappeared vacancies is obtained as
D = RT ln
y
Va
/y
eq
Va
(19.9)
Exercise 19.1
Consider a pure metal A with an equilibrium amount of vacancies. Derive an expression
for the chemical potential of vacancies.
Hint
We need an expression for the total G and should then start from pure A without any
vacancies. That G is proportional to N,sayNg
A
.
Solution
G = Ng
A
+ N
Va
g
Va
+ kNT{ln[N/(N + N
Va
)] + (N
Va
/N) ln[N
Va
/(N + N
Va
)]}; µ
Va
= ∂G/∂ N
Va
= g
Va
+ kNT{−1/(N + N
Va
) + (1/N ) ln[N
Va
/(N + N
Va
)] + (N
Va
/N)
(1/N
Va
) − (N
Va
/N)[1/(N + N
Va
)]}=g
Va
+ kT ln[N
Va
/(N + N
Va
)] = g
Va
+kT ln y
Va
.
At equilibrium we get from Eq. (19.5): µ
Va
= g
Va
− g
Va
= 0.
19.3 Topological disorder
Even though the structure of a melt may intuitively be imagined as completely disordered,
in reality the short-range arrangement of the atoms in a liquid metal is rather similar to
that in the crystalline state. It may thus be useful to describe the liquid as a crystalline
phase with so much disorder that all the long-range order has been destroyed. In this
connection one talks about topological disorder.Avery simple and crude model of
the topological disorder in a liquid metal is based upon the assumption that the amount
of thermal vacancies increases discontinuously during melting. This model can be sup-
ported by many semi-quantitative considerations involving such properties as density,
compressibility, thermal expansion and diffusivity. X-ray measurements have indicated
that the coordination number in liquid metals is about 11, as compared to 12 for the
close-packed fcc and hcp structures. This result immediately suggests that the vacancy
concentration would be about 1/12, i.e. 8%.
This value compares favourably with a value we obtain by evaluating how many
vacancies can form when the heat of melting is added to the solid. According to Richards’
rule the entropy of melting is approximately R and the heat of melting is RT
m.p.
.With
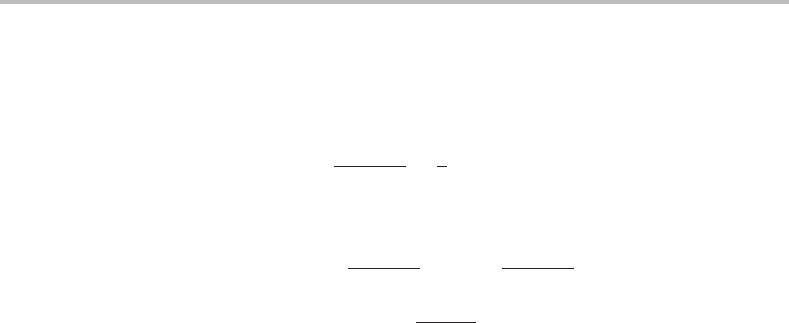
424 Modelling of disorder
the approximate value of 8 for g/kT
m.p.
given above, we get
N
Va
= RT
m.p.
/g = RT
m.p.
/8kT
m.p.
= N /8 (19.10)
y
Va
=
N
Va
N + N
Va
=
1
9
∼
=
11%. (19.11)
It does not compare so nicely with a value obtained from the entropy of melting
S/k =−N ln
N
N + N
Va
− N
Va
ln
N
Va
N + N
Va
= R/k = N (19.12)
ln(1 − y
Va
) +
y
Va
1 − y
Va
ln y
Va
=−1. (19.13)
By solving this equation numerically one finds approximately y
Va
= 1/3or35%. It is
evident that this simple model is too crude to give a quantitative description of melting.
At the melting point of a metal, C
P
is often larger in the melt than in the solid. This
difference grows for the undercooled melt and the difference in entropy between the two
phases will thus decrease with decreasing temperature. Extrapolations have indicated
that one may approach a temperature below which the undercooled melt would have
lower entropy than the crystalline solid. This is regarded as impossible and is called
Kauzmann’s paradox [38]. It has instead been suggested that the liquid has turned amor-
phous on cooling to the temperature where the difference in entropy disappears. Below
that critical point the amorphous solid is assumed to have almost the same entropy as
the crystalline solid. Experimentally one has observed a so-called glass transition where
the liquid turns extremely viscous and it has been related to the temperature defined by
equal entropy. According to this picture, the topological disorder in the amorphous solid
should be very limited. It is not sufficient to make the phase liquid and it has rather low
entropy. On heating above the glass transition temperature a large amount of defects are
created. They make the amorphous phase more liquid and contribute to the entropy and
heat capacity. An increasing amount of defects are created as the temperature is raised
towards the melting point. From the thermodynamic point of view, the melting of the
amorphous solid is thus a gradual process which continues to or even continues above
the melting point. Figure 19.1 illustrates this behaviour schematically.
Exercise 19.2
Estimate the difference in H at 0 K between the amorphous and crystalline states of a
metal from the following simplifying assumptions. The two states have the same entropy
at0K.UptoT
glass
the two states have the same C
P
. T
glass
falls at T
m.p.
/3 and above
T
m.p.
/3 the difference in C
P
is linear in T. Let C
P
= 0atT
m.p.
.
Hint
Estimate the difference in enthalpy and entropy at T
m.p.
from Richards’ rule,
H
m
/T
m.p.
= S
m
∼
=
R. The assumptions give S
m
= 0. at T
m.p.
/3.
