Helms L.L. Potential Theory
Подождите немного. Документ загружается.

328 8 Newtonian Potential
(2 − n)σ
n
w(x
,x
n
)
=
R
n
0
Γ
y
u(|(x
,x
n
) − (y
,y
n
)|)f
(y
,y
n
) dy
n
dy
=
R
n
0
Γ
y
u(|(x
, −x
n
) − (y
, −y
n
)|)f
(y
,y
n
) dy
n
dy
=
R
n
0
Γ
r
y
u(|(x
, −x
n
) − (y
,z
n
)|)f
(y
, −z
n
) d(−z
n
)
dy
=
R
n
0
Γ
y
u(|(x
, −x
n
) − (y
,z
n
)|)f
(y
,z
n
) dz
n
dy
=(2− n)σ
n
w(x
, −x
n
).
The change from d(−z
n
)todz
n
in going from the fourth line to the fifth
is justified since Γ
r
y
is replaced by Γ
y
. It follows that w is symmetric with
respect to R
n
0
. By the argument used above to show that D
x
n
H(h
)(x
, 0) =
0,D
n
w =0onΣ.
The sets Ω and Σ of the following lemma need not satisfy the standing
assumption stated at the beginning of this section.
Lemma 8.5.4 (Boundary Point Lemma [33]) If Ω is a bounded open
subset of R
n
,Σ is a relatively open subset of ∂Ω, u ∈ C
1
(Ω ∪ Σ) ∩ C
2
(Ω)
with Δu ≥ 0 on Ω,x
0
∈ Σ, u(x
0
) >u(x) for all x ∈ Ω,andthereisaball
B ⊂ Ω internally tangent to Σ at x
0
,thenD
n
u(x
0
) > 0.
Proof: Let B = B
y,ρ
,x ∈ B
y,ρ
,r = |x − y|,v(x)=e
−kr
2
− e
−kρ
2
,where
k is a constant to be chosen, and 0 <δ<ρ.Thenv =0on∂B
y,ρ
,Δv =
(4k
2
r
2
− 2kn)e
−kr
2
,andk can be chosen so that Δv ≥ 0ontheannulus
A
ρ,δ
= B
y,ρ
∼ B
y,δ
.Sinceu(x)−u(x
0
) < 0on∂B
y,δ
and v = e
−kδ
2
−e
−kρ
2
on
∂B
y,δ
, there is a constant >0 such that u(x)−u(x
0
)+v ≤ 0on∂B
y,δ
.This
inequality also holds on ∂B
y,ρ
where v =0.SinceΔ(u−u(x
0
)+v) ≥ Δv ≥ 0
on A
ρ,δ
and u − u(x
0
)+v ≤ 0on∂A
ρ,δ
,u− u(x
0
)+v ≤ 0onA
ρ,δ
by the
maximum principle. Thus,
u(x) − u(x
0
)
|x − x
0
|
≤−
v(x) − v(x
0
)
|x − x
0
|
,x∈ A
ρ,δ
.
As x → x
0
in A
ρ,δ
normal to Σ, the limit of the left term is the inner normal
derivative of u at x
0
. Thus, the outer normal derivative of u at x
0
is
D
n
u(x
0
) ≥−D
n
v(x
0
)=−D
r
v|
r=ρ
=2kρe
−kρ
2
> 0.
Lemma 8.5.5 If f ∈ H
0+α
(Ω),α∈ (0, 1],andg ∈ C
0
(∂Ω ∼ Σ), then there
is a unique u ∈ C
0
(Ω
−
)∩C
1
(Ω∪Σ)∩C
2
(Ω) such that Δu = f on Ω, D
n
u =0
on Σ,andu = g on ∂Ω ∼ Σ.

8.5 The Reflection Principle 329
Proof: By Lemma 8.5.3, there is a w ∈ C
0
(Ω
−
) ∩ C
1
(Ω ∪ Σ) ∩ C
2
(Ω)such
that Δw = f on Ω and D
n
w =0onΣ. Extending f to be 0 on R
n
+
∼ Ω,the
function w constructed by way of Lemma 8.5.2 can be regarded as an element
of C
0
b
(R
n
+
∪ R
n
0
) by Theorem 8.2.5. In particular, w|
∂Ω
∈ C
0
(∂Ω ∼ Σ). By
Theorem 8.5.1, there is a v ∈ C
1
(Ω ∪ Σ) ∩ C
2
(Ω) such that Δv =0on
Ω,D
n
v =0onΣ,and
lim
y→x,y∈Ω
v(y)=g(x) − w|
∂Ω
(x)
for all x ∈ ∂Ω ∼ Σ.Sincev ∈ C
1
(Ω ∪Σ), lim
y→x,y∈Ω
v(y)existsinR for all
x ∈ Σ. As noted in Section 0.1, the function v has a continuous extension on
Ω
−
. Letting u = w + v, Δu = Δw + Δv = f on Ω, D
n
u = D
n
w + D
n
v =0
on Σ,and
lim
y→x,y∈Ω
u(y) = lim
y→x,y∈Ω
w(y) + lim
y→x,y∈Ω
v(y)
= w|
∂Ω
(x)+g(x) − w|
∂Ω
(x)=g(x)
for all x ∈ ∂Ω ∼ Σ.Sincew is continuous on Ω
−
and v has a continuous
extension on Ω
−
,u∈ C
0
(Ω
−
). To prove uniqueness, assume that both w
1
and
w
2
satisfy the conclusions of the lemma. The difference w = w
1
−w
2
satisfies
Δw =0onΩ, D
n
w =0onΣ,andw =0on∂Ω ∼ Σ.Sincew ∈ C
0
(Ω
−
), it
attains it maximum value at some point x
0
∈ Ω
−
.Ifx
0
is an interior point of
Ω,thenw is constant by the maximum principle for harmonic functions, and
therefore w =0onΩ
−
because w =0on∂Ω ∼ Σ.Ifw does not attain its
maximum value at an interior point of Ω,thenx
0
∈ ∂Ω and w(x) <w(x
0
)
for all x ∈ Ω. By the preceding lemma, x
0
∈ Σ because D
n
w(x)=0for
x ∈ Σ.Inthiscase,x
0
∈ ∂Ω ∼ Σ and w(x) ≤ w(x
0
) = 0 for all x ∈ Ω
−
.
Since −w has the same properties as w, w ≥ 0onΩ
−
and so w = w
1
−w
2
=0
on Ω
−
.
A standard method for passing from the homogeneous boundary condition
on Σ to the nonhomogeneous condition is to incorporate the boundary func-
tion into the function f. In addition to the standing assumptions regarding
the spherical chip, another condition will be imposed in the following lem-
mas. Suppose Ω = B
+
= B ∩R
n
+
where B is ball with center at (0, −ρ
0
)and
of radius ρ where 0 <ρ
0
<ρ. If the condition ρ
0
>ρ/
√
2 is imposed and
x =(x
,x
n
) ∈ Ω, then the ball in R
n
0
with center x
of radius x
n
will lie in
Σ. This can be seen by considering the intersection of Ω with hyperplanes
containing the x
n
-axis. If g : Σ → R is a boundary function, recall that
|g|
1+α,Σ
=inf{|u|
1+α,Ω
; u ∈ H
1+α
(Ω),u|
Σ
= g}.
Lemma 8.5.6 If Ω = B
+
where B is a ball with center at (0, −ρ
0
) and of
radius ρ, ρ
0
>ρ/
√
2,α ∈ (0, 1],andg ∈ H
1+α
(Σ), then there is a function
ψ ∈ H
2+α
(Ω) satisfying ψ =0on Σ, D
n
ψ = g on Σ,|ψ|
2+α,Ω
≤ C|g|
1+α,Σ
,
and Δψ ∈ H
0+α
(Ω).

330 8 Newtonian Potential
Proof: According to Theorem 7.2.6, it can be assumed that g ∈ H
1+α
(Ω).
Consider any η ∈ C
2
(R
n
0
)withsupportinB
0,1
⊂ R
n
0
and
R
n
0
η(z) dz =1.
Let
ψ(x
,x
n
)=x
n
R
n
0
g(x
− x
n
z,0)η(z) dz. (8.30)
Under the hypothesis that ρ
0
>ρ/
√
2, it can be seen from geometrical
arguments that the point x
− x
n
z ∈ Σ for all z ∈ B
0,1
⊂ R
n
0
.For
x =(x
,x
n
) ∈ Ω,ψ(x
, 0) = 0,D
n
ψ(x
, 0) = g(x
, 0). Therefore, [ψ|
0,Ω
≤
[g]
0,Σ
≤ C|g|
1+α,Ω
.Fori = n, integrating by parts with respect to z
i
and
making use of the fact that η(z)=0forz outside the ball with center at x
of radius x
n
,
D
x
i
ψ(x
,x
n
)=x
n
R
n
0
D
x
i
g(x
− x
n
z,0)η(z) dz
= −
R
n
0
D
z
i
g(x
− x
n
z,0)η(z) dz
=
R
n
0
g(x
− x
n
z,0)D
z
i
η(z) dz. (8.31)
For i, j = n,
D
x
i
x
j
ψ(x
,x
n
)=
R
n
0
D
x
j
g(x
− x
n
z,0)D
z
i
η(z) dz. (8.32)
Similarly,
D
x
n
ψ(x
,x
n
)=x
n
R
n
0
n−1
j=1
D
x
j
g(x
− x
n
z,0)(−z
j
)η(z) dz
+
R
n
0
g(x
− x
n
z,0)η(z) dz
= x
n
n−1
j=1
R
n
0
D
z
j
g(x
− x
n
z,0)
−
1
x
n
(−z
j
η(z)) dz
+
R
n
0
g(x
− x
n
z,0)η(z) dz
= −
n−1
j=1
R
n
0
g(x
− x
n
z,0)D
z
j
(z
j
η(z))dz
+
R
n
0
g(x
− x
n
z,0)η(z) dz
= −
R
n
0
g(x
− x
n
z,0)
∇η(z) · z +(n − 2)η(z)
dz. (8.33)
8.5 The Reflection Principle 331
By Equations (8.31) and (8.33), [ψ]
1+0,Ω
≤ C[g]
0,Σ
≤ C|g|
1+α,Ω
.Fori = n,
D
x
n
x
i
ψ(x
,x
n
)=−
R
n
0
D
x
i
g(x
−x
n
z,0)
∇η(z)·z +(n−2)η(z)
dz. (8.34)
Similarly,
D
x
n
x
n
ψ(x
,x
n
)=
R
n
0
∇g(x
−x
n
z,0)·z
∇η(z)·z+(n−2)η(z)
dz. (8.35)
As before, [ψ]
2+0,Ω
≤ C[g]
1+0,Σ
≤ C|g|
1+α,Ω
= C|g|
1+α,Σ
.Since|(x
−x
n
z)−
(y
− y
n
z)|≤|x − y|(1 + |z|), for i = n
|D
x
i
x
j
ψ(x
,x
n
) − D
x
i
x
j
ψ(y
,y
n
)|
|x − y|
α
≤
R
n
0
|D
x
j
g(x
− x
n
z,0) − D
x
j
g(y
− y
n
z), 0|
|(x
− x
n
z) − (y
− y
n
z)|
α
(1 + |z|)
α
|D
z
i
η(z)|dz
≤ C[g]
1+α,Σ
≤ C|g|
1+α,Ω
= C|g|
1+α,Σ
. (8.36)
Taking the supremum over x, x
∈ Ω,[ψ]
2+α,Ω
≤ C|g|
1+α,Σ
. Therefore,
|ψ|
2+α,Ω
≤ C|g|
1+α,Σ
.
Theorem 8.5.7 If Ω satisfies the conditions of the preceding lemma and
f ∈ H
0+α
(Ω),α ∈ (0, 1],g ∈ H
1+α
(Σ) and h ∈ C
0
(∂Ω ∼ Σ), then there is a
unique w ∈ C
0
(Ω
−
) ∩C
1
(Ω ∪Σ) ∩C
2
(Ω) satisfying Δw = f on Ω,D
n
w = g
on Σ,andw = h on ∂Ω ∼ Σ.
Proof: Consider the function ψ of the preceding lemma for which D
n
ψ = g
on Σ and Δψ ∈ H
0+α
(Ω) and the problem
(i) Δv = f −Δψ on Ω,
(ii) D
n
v =0onΣ,and
(iii) v = h − ψ on ∂Ω ∼ Σ.
Since ψ ∈ H
2+α
(Ω), it is uniformly continuous on Ω and has a continuous
extension to ∂Ω.Sincef − Δψ ∈ H
0+α
(Ω)andh − ψ ∈ C
0
(∂Ω ∼ Σ), by
Lemma 8.5.5 there is a v ∈ C
0
(Ω
−
) ∩C
1
(Ω ∪Σ) ∩C
2
(Ω) satisfying (i)-(iii).
Letting w = v + ψ, Δw = Δv + Δψ = f on Ω, D
n
w = D
n
v + D
n
ψ = g on
Σ,andw = v + ψ = h − ψ + ψ = h on ∂Ω ∼ Σ.Sinceψ ∈ H
2+α
(Ω) implies
that ψ ∈ C
0
(Ω
−
) ∩C
1
(Ω ∪Σ) ∩C
2
(Ω), w ∈ C
0
(Ω
−
) ∩C
1
(Ω ∪Σ) ∩C
2
(Ω).
Uniqueness of w follows as in the proof of Lemma 8.5.5.
This page intentionally left blank
Chapter 9
Elliptic Operators
9.1 Introduction
Up to this point, all of the concepts pertain to the Dirichlet and Neumann
problems for the Laplacian. In this and later chapters, the Laplacian will
be replaced by an elliptic operator as described below and mixed boundary
conditions will be allowed that specify the value of a function on part of
the boundary and the value of its normal derivative on the remainder of the
boundary as in Theorem 8.5.7.
Let Ω be a bounded open subset of R
n
with a smooth boundary ∂Ω.A
precise smoothness requirement will be specified later. For i, j =1,...,n,
let a
ij
,b
i
,c be real-valued functions on Ω with a
ij
= a
ji
. In addition, for
i =1,...,n,letβ
i
,γ be real-valued functions on ∂Ω.Anelliptic operator
is an operator acting on real-valued functions u on Ω of the form
Lu(x)=
n
i,j=1
a
ij
(x)
∂
2
u
∂x
i
∂x
j
(x)+
n
i=1
b
i
(x)
∂u
∂x
i
(x)+c(x)u(x),x∈ Ω,
with suitable conditions imposed on the coefficients. In addition, a boundary
condition can be specified by an operator M defined by the equation
Mu(x)=
n
i=1
β
i
(x)
∂u
∂x
i
(x)+γ(x)u(x)=0,x∈ ∂Ω.
In terms of the operators L and M, the Dirichlet problem is a special case of
the problem
Lu = f on Ω,u = g on ∂Ω,
and the Neumann problem is a special case of the problem
Lu = f on Ω,Mu = g on ∂Ω.
The latter problem is known as the oblique derivative problem.
L.L. Helms, Potential Theory, Universitext, 333
c
Springer-Verlag London Limited 2009

334 9 Elliptic Operators
Each of the results of this chapter are stated under minimal conditions on
the coefficients of L; all of these conditions are satisfied if
sup
1≤i,j≤n
a
ij
0,Ω
+|a
ij
; d|
(0)
α,Ω
+b
i
0,Ω
+|b
i
; d|
(1)
α,Ω
+c
0,Ω
+|c; d|
(2)
α,Ω
< +∞.
9.2 Linear Spaces
Results pertaining to the Dirichlet problem for the Laplacian operator can
be carried over to a general elliptic operator by the method of continuity.
This method requires the use of some theorems about mappings between
normed linear spaces.
AmapT from a Banach space B into a normed linear space X is bounded
if there is a constant C such that Tx
X
≤ Cx
B
. The smallest C for which
this is true is denoted by T ;thatis,T =sup
x∈B,x=0
Tx
X
/x
B
.
AmapT : B → B is a contraction map if there is a constant λ<1such
that Tx − Ty
B
≤ λx − y
B
for all x, y ∈ B.ThemapT : B → X is a
linear map if T(ax + by)=aTx + bTy for all a, b ∈ R and all x, y ∈ B.
AmapT : B → B is a bounded linear operator or simply a bounded
operator on T if bounded and linear as defined above.
Theorem 9.2.1 If T is a contraction map on the Banach space B,then
there is a unique x ∈ B such that Tx = x.
Proof: Let x
0
be an arbitrary point of B and let λ<1beaconstantsuch
that Tx≤λx for all x ∈ B. It is easy to see that T
j
x≤λ
j
x for all
j ≥ 1,x∈ B.Foreachj ≥ 1, let x
j
= T
j
x
0
.Ifj ≥ i,then
x
j
− x
i
≤
j
k=i+1
x
k
− x
k−1
=
j
k=i+1
T
k−1
x
1
− T
k−1
x
0
≤
j
k=i+1
λ
k−1
x
1
− x
0
≤
λ
i
1 − λ
x
1
− x
0
→0asi →∞.
This shows that the sequence {x
j
} is Cauchy in B and therefore converges
to some x ∈ B.SinceT is a continuous map, Tx = lim
j→∞
Tx
j
=
lim
j→∞
x
j+1
= x.IfTy = y also, then x − y = Tx − Ty≤λx − y
with λ<1 and therefore x = y.
Theorem 9.2.2 (Method of Continuity) Let B be a Banach space, let X
be a normed linear space, and let T
0
, T
1
be bounded linear operators from B
into X.Foreacht ∈ [0, 1],letT
t
=(1−t)T
0
+ tT
1
. If there is a constant C,
not depending upon on t, such that
x
B
≤ CT
t
x
X
, for all t ∈ [0, 1],x∈ B, (9.1)
then T
1
maps B onto X if and only if T
0
maps B onto X.

9.3 Constant Coefficients 335
Proof: Suppose T
s
maps B onto X for some s ∈ [0, 1]. By Inequality (9.1),
T
s
is one-to-one and therefore has an inverse T
−1
s
: X → B.Forfixedt ∈ [0, 1]
and y ∈ X,thereisanx ∈ B satisfying the equation T
t
x = y if and only if
T
s
x = y +(T
s
− T
t
)x = y +(t − s)T
0
− (t − s)T
1
.
This equation holds if and only if
x = T
−1
s
y +(t − s)T
−1
s
(T
0
− T
1
)x;
that is, if and only if the map T : B → B defined by
Tx = T
−1
s
y +(t − s)T
−1
s
(T
0
− T
1
)x
has a fixed point. Since T
−1
s
y
B
≤ Cy
X
for all y ∈ X,
Tx
1
− Tx
2
X
= (t − s)T
−1
s
(T
0
− T
1
)(x
1
− x
2
)
B
≤ C|t − s|T
0
− T
1
x
1
− x
2
B
≤ C|t − s|(T
0
+ T
1
) x
1
− x
2
B
so that T is a contraction operator on B if
|t − s| <δ=
1
C(T
0
+ T
1
)
.
By the preceding theorem, T has a fixed point whenever |t − s| <δ;that
is, if |t − s| <δ,thenT
t
maps B onto X whenever T
s
maps B onto X.
By subdividing the interval [0, 1] into subintervals of length less than δ,the
mapping T
t
is onto for all t ∈ [0, 1] provided it is onto for some s ∈ [0, 1], in
particular if s =0ors =1.
9.3 Constant Coefficients
It will be assumed in this section that α ∈ (0, 1) and that the coefficients
a
ij
,i,j =1,...,n, of the elliptic operator defined in Section 9.1 are all con-
stants, that a
ij
= a
ji
,i,j =1,...,n, and that the b
i
,i =1,...n,andc are
all 0. In this case, the operator will be denoted by
L
0
u(x)=
n
i,j=1
a
ij
D
ij
u(x).
336 9 Elliptic Operators
It also will be assumed that there are positive constants m and M such
that
m|x|
2
≤
n
i,j=1
a
ij
x
i
x
j
≤ M|x|
2
. (9.2)
This assumption implies that the matrix A =[a
ij
] is positive definite.
The following facts from elementary matrix theory will be needed (c.f.,
Hohn [31]). There is an n ×n orthogonal matrix O =[o
ij
] such that OAO
t
=
diag[λ
1
,...,λ
n
]whereλ
1
...,λ
n
are the eigenvalues of A and the superscript
t signifies the transpose matrix. Regarding elements of R
n
as column vectors
for purposes of this discussion, if y = Ox,then
x
t
Ax =
n
i,j=1
a
ij
x
i
x
j
=
n
i=1
λ
i
y
2
i
.
It also is known from matrix theory that O preserves norms; that is, |Ox| =
|x|,x∈ R
n
. It follows that m =min(λ
1
,...,λ
n
)andM =max(λ
1
,...,λ
n
).
Now let
D =[λ
−
1
2
i
δ
ij
]=diag[1/
λ
1
,...,1/
λ
n
]
and define T : R
n
→ R
n
by the equation y = Tx= DOx.Since
x
t
Ax =
n
i=1
λ
2
i
(Tx)
2
i
,
m|x|
2
≤
n
i=1
λ
2
i
(Tx)
2
i
≤ M |x|
2
.
Thus,
m
M
2
|x|
2
≤
n
i=1
(Tx)
2
i
≤
M
m
2
|x|
2
,
and there is a constant c = c(m, M) such that
1
c
|x|≤|Tx|≤c|x|. (9.3)
If Ω is an open subset of R
n
,letΩ
∗
be the image of Ω under T . Recalling
that d(y)=dist(y, ∼ Ω), the d notation will be augmented by adding the
subscript Ω in order to deal with the two sets Ω and Ω
∗
simultaneously. It
is easily seen using the above inequality that
1
c
d
Ω
(x) ≤ d
Ω
∗
(y) ≤ cd
Ω
(x). (9.4)
Consider now any function u on Ω. The transformation T defines a function
u∗ on the image Ω
∗
of Ω by the equation u
∗
(y)=u(x)wherey = Tx.If
u ∈ C
2
(Ω)oru ∈ H
α
(Ω), then u
∗
∈ C
2
(Ω
∗
)oru
∗
∈ H
α
(Ω
∗
), respectively. If
u ∈ C
2
(Ω)andf ∈ H
α
(Ω), the equation L
0
u = f on Ω is transformed into
the equation
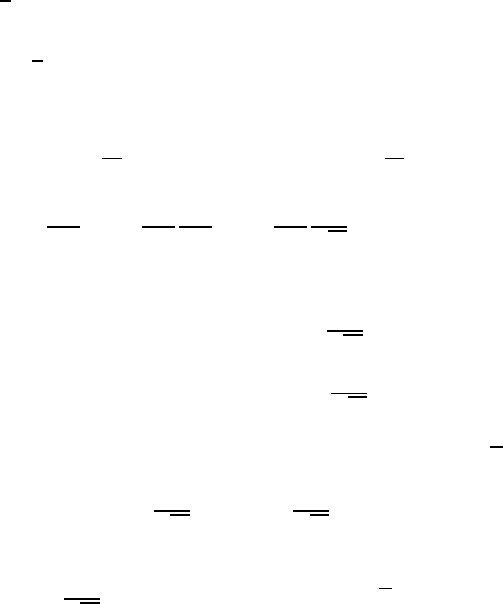
9.3 Constant Coefficients 337
Δu
∗
(y)=f
∗
(y),y∈ Ω
∗
with u
∗
∈ C
2
(Ω
∗
)andf
∗
∈ H
α
(Ω
∗
). This result provides a means of solving
the equation L
0
u = f on the bounded open set Ω by solving the equation
Δu
∗
= f
∗
on Ω
∗
.
The norms |u; d
Ω
|
2+α,Ω
and |f ; d
Ω
|
(b)
2+α,Ω
are related to |u
∗
; d
Ω
∗
|
2+α,Ω
∗
and |f
∗
; d
Ω
∗
|
(b)
2+α,Ω
∗
, respectively, as in
1
c
|u; d
Ω
|
2+α,Ω
≤|u
∗
; d
Ω
∗
|
2+α,Ω
∗
≤ c|u; d
Ω
|
2+α,Ω
, (9.5)
1
c
|f; d
Ω
|
(b)
α,Ω
≤|f
∗
; d
Ω
∗
|
(b)
α,Ω
∗
≤ c|f; d
Ω
|
(b)
α,Ω
(9.6)
where c = C(n, m, M ). These inequalities follow from Inequalities (9.3) and
(9.4). Just one of the inequalities will be proven as an illustration; namely,
|u; d
Ω
|
2+α,Ω
≤ c|u
∗
; d
Ω
∗
|
2+α,Ω
∗
. Clearly, u
0,Ω
= u
∗
0.Ω
∗
. Suppose y =
Tx.Theny
i
=
n
j=1
(1/
√
λ
i
)o
ij
x
j
so that ∂y
i
/∂x
j
=(1/
√
λ
i
)o
ij
.Foreachj,
∂u
∂x
j
=
n
i=1
∂u
∗
∂y
i
∂y
i
∂x
j
=
n
i=1
∂u
∗
∂y
i
1
√
λ
i
o
ij
and therefore
|d
Ω
(x)D
j
u(x)|≤c
n
i=1
|d
Ω
∗
(y)D
i
u
∗
(y)|
1
√
λ
i
|o
ij
|
≤ c[u
∗
; d
Ω
∗
]
1+0,Ω
∗
n
i=1
1
√
λ
i
|o
ij
|
. (9.7)
With j fixed, let z
i
=1ifo
ij
≥ 0andz
i
= −1ifo
ij
< 0. Then |z| =
√
n and
(Tz)
i
=
n
i=1
1
√
λ
i
o
ij
z
j
=
n
i=1
1
√
λ
i
|o
ij
|.
Thus,
n
i=1
1
√
λ
i
|o
ij
| =(Tz)
i
≤|Tz|≤c|z| = c
√
n (9.8)
and it follows that
[u; d
Ω
]
1+0,Ω
≤ C(n, m, M )[u
∗
; d
Ω
∗
]
1+0,Ω
∗
.
Similarly, [u; d
Ω
]
2+0,Ω
≤ C(n, m, M )[u
∗
; d
Ω
∗
]
2+0,Ω
∗
and
[u; d
Ω
]
2+α,Ω
≤ C(n, m, M )[u
∗
; d
Ω
∗
]
2+α,Ω
∗
.
Therefore,
|u; d
Ω
|
2+α,Ω
=
2
j=0
[u; d
Ω
]
j+0,Ω
+[u; d
Ω
]
2+α,Ω
≤ C(n, m, M )|u
∗
; d
Ω
∗
|
2+α,Ω
∗
.
