Heitjans P., Karger J. (Eds.). Diffusion in Condensed Matter: Methods, Materials, Models

Подождите немного. Документ загружается.

7 Fluctuations and Growth Phenomena in Surface Diﬀusion 313

and the calculated time constant τ

1/2

can be smaller than the expected one

for zero ﬁeld (τ = A/4D

Biased diﬀusion in the presence of an electric ﬁeld was modeled with

Monte Carlo simulations and simple site-exclusion interaction [40]. The cor-

relation function was calculated for diﬀerent starting times t

(starting from

= 0 when there is a random distribution of atoms on the surface) as atoms

diﬀuse towards the tunneling area. For shorter times t

, strong deviations

from the expected form (7.27) are observed, as a result of both the coverage

rearrangement and the biased character of diﬀusion. For long enough times

the shape of the correlation function becomes identical to (7.27) for the

non-biased potential energy surface, although the value of the time constant

is half the expected one (A/4D

), i.e. an initial ﬂuctuation decays faster on

the “tilted” than on the ﬂat surface. It is left to further studies to justify

these results analytically.

7.4 Non-Equilibrium Experiments

Two growth experiments shall be described where diﬀusion controls the ﬁnal

outcome. Because such experiments are non-equilibrium ones (i.e. carried out

at low temperatures or high ﬂux rates so that equilibrium cannot be estab-

lished), a single microscopic process is suﬃcient to account for the observa-

tions. There is no need for averaging over the diﬀerent microscopic processes,

as in ﬂuctuation experiments.

7.4.1 Uniform-Height Pb Islands on Si(111)

Growth of Uniform-Height Islands in Pb/Si(111)

at Low Temperatures

Epitaxial growth is widely used to fabricate custom-made structures with

nano-scale dimensions that do not exist in nature. Because the conﬁnement

of the electrons in these small structures leads to sharp quantization of their

energy levels, these structures can have important technological applications

as lasing devices, sensors, ultrafast switches, etc. The spacing of these energy

levels (and thus the electronic properties of the nano-scale devices) can be

adjusted if the size of the growing structure can be controlled.

Since epitaxial growth is commonly carried out far from equilibrium the

grown structures are metastable. The key controlling processes for the growth

in a speciﬁc system are the ones with time constants smaller than the dura-

tion of the experiment. Because it is not clear which microscopic process is the

controlling one, it is important to check the full space of growth parameters

(i. e. coverage, temperature, ﬂux rate, kinetic pathway, etc.) in order to de-

termine the types of structures which form, especially whether it is possible,

to observe structures which are self-organized and regular.

314 Michael C. Tringides and Myron Hupalo

A lot of eﬀort in growth experiments is necessary to produce smooth (i. e.

layer-by-layer) ﬁlms where a layer is completed before a new one starts to

grow. This type of growth results in ﬁlms of minimal roughness and well-

deﬁned electronic states. On the other hand, if growth is rough (i.e. three-

dimensional) the electronic levels are smeared out which degrades their poten-

tial technological applications. Great eﬀorts have been made to understand

the factors that determine the type of growth mode and, in case it is 3D,

to ﬁnd ways to modify it to layer-by-layer growth. One way to accomplish

this is with the use of surfactants [41] i. e. by involving a small amount of

impurities that modify the kinetic barriers and enhance interlayer diﬀusion.

It is far more challenging to fabricate regular structures where conﬁne-

ment is in more than the normal direction as in an ultrathin ﬁlm. Sharper

electronic levels are expected. For example, islands of suﬃciently small lateral

size, the so-called quantum dots, conﬁne the electrons in all three dimensions.

By adjusting the geometrical dimensions, energy selectivity and improved

monochromaticity of the emitted radiation during electronic transitions be-

come possible.

Strain can play an important role in fabricating quantum dots, like in

Ge-Si alloys [42]. Islands, already buried within a Si-capped layer, generate a

strain ﬁeld propagating through the capped layer producing deep energy min-

ima at speciﬁc locations on the surface. These minima “stir” the nucleation

of new islands deposited on the capped layer to positions highly correlated to

the buried islands below and can lead to highly uniform island size/separation

distributions, if several consecutive cycles of deposition/capping are used.

Next we describe a diﬀerent method to produce uniform-height metallic

quantum dots on semiconductor substrates observed during low-temperature

growth. Such uniformity has been observed in the Pb/Si(111)-(7 ×7) [43,44]

system and is not driven by stress, since a wetting layer of one ML forms

initially to relieve the stress and to allow perfect Pb(111) crystallites to grow

on top of it.

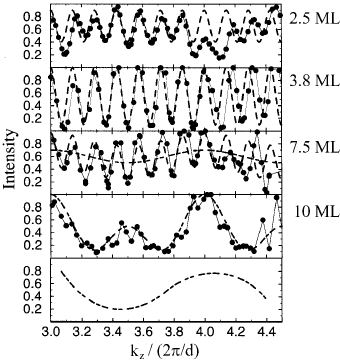
In these experiments two complementary techniques are used, STM and

high resolution electron diﬀraction (SPA-LEED) to determine the growth

mode, as a function of coverage and temperature. With SPA-LEED at 185 K

intensity oscillations are observed as a function of perpendicular momen-

tum transfer k

of the scattered wave, for diﬀerent deposited Pb amounts

as shown in Fig. 7.10. Oscillations are produced as the scattering condition

changes from in-phase (i. e. Hk

=2π(integer), when the scattered inten-

sity has maxima, with H denoting the island height) to out-of-phase (i. e.

= π(odd), when the scattered intensity has minima) as discussed in

Sect. 7.3.1. The island height H can be found from the oscillation period

∆k

, H =2π/∆k

. If single steps were present (H = d) the maxima would

correspond to integer values of k

/(2π/d)=3, 4 (the variable on the abscissa)

shown schematically at the bottom of Fig. 7.10. Since the observed period

∆k

is 1/7 of the oscillation for single steps, it implies that H =7d.More

7 Fluctuations and Growth Phenomena in Surface Diﬀusion 315

Fig. 7.10. 7-fold diﬀraction os-

cillations of the (00) intensity vs

normal-component momentum

transfer k

/(2π/d) indicating 7-

step islands for Pb/Si(111)-(7 ×

7) grown at T = 185 K for cov-

erage θ ≤ 7.5 ML. The bottom

curve indicates the expected 1-

fold oscillation, if single steps

were present.

detailed analysis of the amplitude of the oscillations, to extract all Fourier

components, suggests that the islands have ﬂat tops and steep edges. In fact,

the width of the facets regions at the sides of the island turns out to be

smaller than 10% of the island size.

These diﬀraction results have been conﬁrmed with variable temperature

STM. Figure 7.11 shows an STM 200 nm × 200 nm image for θ =3.3ML

Pb deposited on Si(111)-(7 × 7) at T = 190 K [44]. This unusual growth has

been also reproduced with STM in [45]. One sees directly the regular Pb

islands with striking ﬂat tops and steep edges in excellent agreement with

the diﬀraction results. A histogram of the island heights is shown at the

bottom of Fig. 7.11. The main peak in the histogram corresponds to islands

with 6.7-single-step and a smaller peak is present at 3.8-single-step islands.

The non-integer height values are related to the diﬃculty in deﬁning the

origin of the layer between the islands with STM, since in STM one measures

the electron density contours and not the atomic centers. The presence of

a sharper height distribution in diﬀraction is because of the vastly superior

statistical average (10

islands are included in collecting the data of Fig. 7.10

while only 40 islands are considered for the histogram of Fig. 7.11). At 190 K,

from Fig. 7.11 the average island diameter is found to be 10 nm (consistent

with the inverse of the FWHM of the Pb(10) spot) and the average island

separation is 30 nm (consistent with the satellite spots close to the (00) spot

expected for a spatially correlated island separation). The island size and

separation increase by approximately a factor of 3 as the temperature changes

from 130 K to 240 K.

Extensive studies on Pb/Si(111)-(7 × 7) were carried out by varying the

growth temperature, coverage, annealing temperature and annealing time to

measure how the selected island height depends on the growth conditions.

Although for a given T and θ only one island height is the preferred one

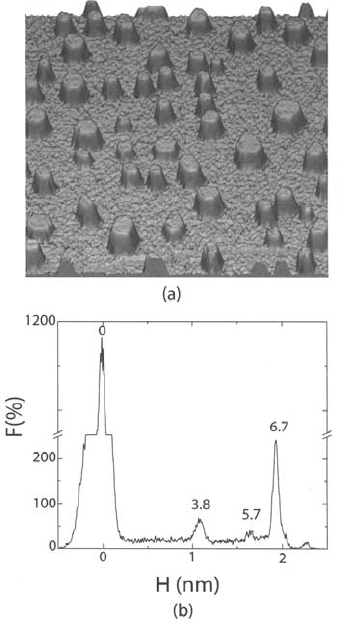
316 Michael C. Tringides and Myron Hupalo

Fig. 7.11. (a) 200 nm × 200 nm

STM image and (b) height his-

togram of Pb/Si(111)-(7 × 7) at

190 K and θ =3.3ML con-

ﬁrming the diﬀraction data of

Fig. 7.10 that the islands grow

in preferred 7-step heights.

(for example at T = 185 K 7-step is the preferred height) the selected height

changes with growth conditions by bilayer 2d =0.58 nm height increments

(d =0.29 nm is the single-step height of Pb(111)). 5-step oscillations are

observed at the lower temperatures 130–160 K, followed by 7-step islands,

followed by 9-step islands for T>210 K etc.. From these studies a T − θ

kinetic phase diagram shown in Fig. 7.12 [44] was constructed and by choosing

the appropriate T −θ range a given height can be selected in the experiment.

The growth experiments were performed by direct deposition of a ﬁxed

amount or by stepwise deposition of small increments. No diﬀerence in the

island dimensions was found which indicates that diﬀusion in the system is

extremely fast, since these islands are completed within the deposition time

(i.e. 4 minutes to deposit 4 ML). Annealing experiments were also performed

with the surface heated from the initial lower temperature T

to a higher

ﬁnal temperature T

. Although the same island height is selected in annealing

and growth experiments (for the same T

), islands larger in size form after

annealing than after growth experiments. However, once a ﬁnal temperature

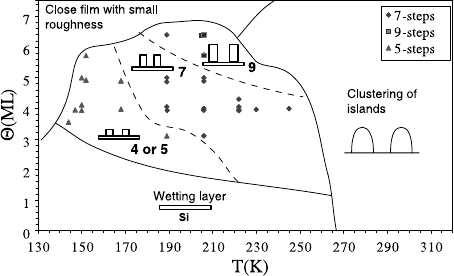
7 Fluctuations and Growth Phenomena in Surface Diﬀusion 317

Fig. 7.12. θ-vs-T kinetic phase diagram for growth on Pb/Si(111)-(7 × 7) show-

ing that preferred island heights 4, 7, 9 ... can be selected for diﬀerent growth

conditions.

is reached no further increase in island height and minor increase in size

is observed despite prolonged (≈ 1/2 hour) heating at constant T

,which

indicates the extraordinary mobility of ∼ 10

deposited Pb atoms to diﬀuse

and build the islands within ∼ 4 minutes and, most likely, some type of

non-thermal diﬀusion.

Quantum Size Eﬀects (QSE) and Charge Transfer

in Pb/Si(111) Growth

What is the driving force for the formation of the uniform-height islands?

An explanation was proposed in terms of quantum size eﬀects (QSE), i. e.

the dependence of the energy of the conﬁned electrons occupying discrete

energy levels on island thickness [46, 47]. The boundary conditions on the

electron wavefunction imposed by the two interfaces (metal-semiconductor

and metal-vacuum) is matched for preferred ﬁlm thickness nd = sλ

/2(with

n and s integers), d =0.29 nm and Fermi wavelength λ

=0.393 nm [48]

with approximately n =5, 7, 9 .... Because of the special relation between

and d (i. e., d ≈ 3/4λ

) the bilayer height increments provide evidence of

QSE. Since bilayer height increments add approximately three nodes to the

electron wavefunction, 2d ≈ 3λ

/2, they are favored. These simple estimates

have been fully supported by ﬁrst-principle calculations [49].

Other experiments using He-scattering on Pb/Cu(111) [50], X-ray scat-

tering on Pb/Si(111) [51] and in-situ conductivity on Pb/Si(111) at T =90K

[48], show bilayer intensity oscillations consistent with bilayer height incre-

ments. He-scattering studies of Pb/Ge(001) [52] growth show that the in-

terlayer spacing adjusts in a way that the ﬁlm height is always close to

an integer multiple of λ

/2. Ni on hydrogen-terminated C(111) surfaces at

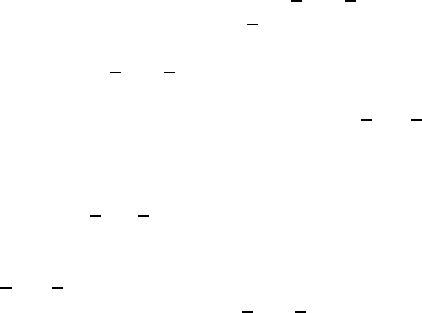
318 Michael C. Tringides and Myron Hupalo

T = 800 K show the formation of multiple-height islands with ﬂat tops [53].

Other low-temperature studies on Ag/GaAs(100) [54] of ﬁlms which normally

grow three-dimensionally, show “magic” thickness eﬀects after annealing to

room temperature. Depending on the deposited amount the ﬁlm separates

into regions of “magic” 7-layer thickness and empty “pits” indicating that

the electronic energy of the ﬁlm dictates its structure, a novel growth mode

labeled “electronic growth” [55].

A test whether the conﬁnement energy of the electrons in the Pb islands is

suﬃcient to explain the height selection or whether other factors (i.e. charge

transfer at the metal/semiconductor interface) are needed, is to grow on a

diﬀerent reconstructed surface the Si(111)-Pb

√

3 ×

√

3 phase [56, 57]. The

electronic structures on the (7 × 7) and

√

3 phases are known from angle-

resolved photoemission [58]. They show that the Fermi level is 0.19 eV above

the valence band on the

√

3 ×

√

3 phase vs 0.42 eV on the (7 × 7) phase.

This implies that initially there is a larger diﬀerence ∆E

between the Fermi

levels on the metal and the semiconductor side for the

√

3 ×

√

3thanforthe

(7 × 7) interface. The charge transfer energy ∆U, to a ﬁrst approximation,

can be estimated as the energy stored in a capacitor of capacitance C and

potential diﬀerence (∆E

/e)so∆U =(1/2)C(∆E

/e)

Experiments on the

√

3 ×

√

3 phase show that for the same growth condi-

tions (θ =4ML,T = 190 K) 5-step islands are those of the preferred height,

while for growth on the (7 × 7) phase it is 7-steps. Since the Fermi level is

lower on the

√

3 ×

√

3 phase than on the (7 × 7) phase, the charge transfer

contribution is larger for growth on the

√

3 ×

√

3 interface, with larger en-

ergy gain at 5-step compared to the 7-step thickness [56]. This conﬁrms that

charge transfer (and diﬀerent initial reconstructions on the substrate) play a

role in island height selection.

Low-Temperature Mobility

While QSE provide energetic reasons that Pb islands with preferred height

are formed, it is still not clear what are the key kinetic processes that lead to

the unusual self-organization. Although QSE explain why at preferred island

heights the energy have minima, it leaves wide open the question of how

mass transport gives rise to the ordering. Speciﬁcally, how do the deposited

Pb atoms move from initially random positions where they land on the surface

to well-deﬁned positions in speciﬁc island layers, within the relatively short

time of the deposition rate (∼ minutes)andatsuchlowtemperatures?

Other growth experiments at low temperatures have shown that reg-

ular structures can form, as evidenced from diﬀraction oscillations during

growth [59]. Such oscillations were observed for Pb/Si(111) [60] even at 16 K.

STM and FIM studies in other systems have shown that impinging atoms

at low temperatures can have ﬁnite mobility. A review of low-temperature

growth and the presence of ﬁnite mobility is given in [59]. Despite numerous

studies suggesting some type of mobility at low temperatures its origin is still

7 Fluctuations and Growth Phenomena in Surface Diﬀusion 319

unclear. One explanation is based on the eﬃciency of the energy transfer from

the incoming adatom to the substrate. Depending on the desorption energy,

this additional energy can amount to a few eV. While it is diﬃcult to observe

the accommodation process experimentally because it is ultrafast (i. e. with

time constants of some picoseconds), Molecular Dynamics simulations of the

landing process have been performed [61]. The simulations give mixed results

since the evidence for non-thermal mobility depends on the interatomic po-

tential used to describe the interactions between the adatom and substrate

atoms. Depending on the softness of the potential, the energy transfer can be

completed within the ﬁrst landing or if the adatom retains some of its energy

after the impact, it can come to rest after several hops. Such mechanism was

invoked to explain the diﬀraction oscillations in Pb/Si(111) [51]. It is still an

open question whether the necessary mass transport for the buildup of the

uniform-height Pb islands can be accounted for only by thermal diﬀusion or

whether some type of non-thermal mobility is necessary.

The buildup of the Pb uniform-height islands is the outcome of the bal-

anced interplay between several kinetic processes: thermal and possibly non-

thermal mobility on the terrace, hopping on the island side planes (facets)

from lower to higher levels, and atom retainment at preferred heights. Nor-

mally, atoms are retained on top of islands because a barrier is present at step

edges (to be discussed more extensively for Ag/Ag(111) in 4.2). Experiments

with SPA-LEED (similar to the Ag/Ag(111) experiments) were carried out

to identify which of these barriers is the controlling one. An initial predomi-

nantly 5-step island height distribution is prepared followed by an additional

deposition of ∆θ =0.5 ML Pb at diﬀerent growth temperatures T

to deter-

mine the transition temperature when 2-step islands nucleate on top of the

5-step islands. It is found that the transition is at T

≈ 175 K, which indicates

that the transfer of atoms from the surrounding region to the island top is

the rate limiting step. Terrace diﬀusion is faster than transfer to the top,

since growth and stepwise growth (by depositing small coverage increments)

give identical results. Diﬀusion at the island facets is also faster than transfer

over the edges, otherwise a decrease in the facet slope would be observed as

the atoms accumulate on the facets.

The metastable phases in the kinetic phase diagram of Fig. 7.12 are the

kinetically stabilized phases from the interplay of energy gain at the potential

energy minima and kinetics with incoming atoms overcoming the island edge

barrier. It was also shown that with oxygen the island height and size can be

stabilized over a wider temperature range (up to room temperature) because

oxygen increases the step edge barrier and limits the Pb transfer to the top

[62].

320 Michael C. Tringides and Myron Hupalo

7.4.2 Measurements of Interlayer Diﬀusion on Ag/Ag(111)

Second-Layer Nucleation Experiments

to Measure Interlayer Probability on Ag/Ag(111)

The growth mode (2D or 3D) observed in a system depends on the so-called

interlayer probability of an atom encountering a step, i. e. the probability to

hop from a higher to a lower level [1,2]. Usually the interlayer probability is

described by an Arrhenius expression p =(ν

/ν

−(∆E

T )

where ν

is the

prefactor at the step edge, ν

the prefactor on the terrace and ∆E

is the step

edge barrier. For Ag/Ag(111) the growth was found to be three-dimensional

for all temperatures studied [63–65]. This has been attributed to the presence

of a strong step edge barrier and therefore a low interlayer probability.

Several methods have been applied with diﬀerent experimental techniques

(STM, Diﬀraction) and over diﬀerent temperature ranges to measure the step

edge barrier and to separate the two contributions to p, i.e. the ratio ν

/ν

and the step edge barrier ∆E

Although the simple Arrhenius expression for the interlayer probability

has been widely used in the analysis of experiments, several factors have been

considered which can complicate the picture of hopping over a single step edge

barrier and can result in a more complex dependence of p on temperature.

The step edge barrier can be lower at a kink than at a straight step [66].

Furthermore, diﬀerent step edge barriers can be present at diﬀerent step

types (i. e. A- or B-types on fcc(111) substrates). Such complications can

lead to a non-Arrhenius temperature dependence since the step morphology,

the relative population of A- or B-type steps or the number of atoms at kink

sites etc. can change with temperature.

Experiments to measure the interlayer probability for Ag/Ag(111) with

diﬀerent methods and over diﬀerent temperature ranges have resulted in the

same high barrier ∆E

=0.13–0.15 eV but in diﬀerent prefactor ratios ν

/ν

[67]. Low-temperature (T<150 K) experiments under growth conditions

indicate ν

/ν

> 1 while quasi-equilibrium experiments carried out close to

room temperature show ν

/ν

∼ 1. In this section we review the diﬀerent

experiments and examine possible reasons for the diﬀerence in ν

/ν

The low-temperature experiments [68,69] were carried out under growth

conditions, with a constant ﬂux of atoms incident on the surface. In one ex-

periment, performed with STM [68], an initial island distribution is prepared

by annealing the surface to diﬀerent temperatures T

anne

so the average island

size R(T

anne

) can be varied. After a second deposition with a smaller amount

∆θ =0.1 ML at a lower temperature T

the fraction of islands with second-

layer occupation on top f(T

,R) is measured as a function of temperature

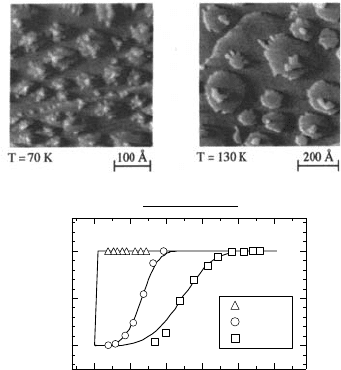
and R. Figure 7.13(a) shows STM results for two growth temperatures

T = 70 K, 130 K [68]. At 70 K practically all islands have second-layer occu-

pation (f = 1), while at T = 130 K only a fraction of the islands is occupied,

despite the islands being larger in size. This behavior of f(T

,R)istheone

7 Fluctuations and Growth Phenomena in Surface Diﬀusion 321

(a)

(b)

1.0

0.5

0.0

fraction of covered islands

10080604020

island radius [Å]

70 K

120 K

130 K

Ag / Ag(111)

Fig. 7.13. (a) STM images of second-layer nucleation experiments on Ag/Ag(111)

at T = 70 K, 130 K showing how interlayer diﬀusion increases with temperature.

(b) Fractional occupation of ﬁrst-layer islands vs island size at ﬁxed temperatures

(70 K, 120 K, 130 K).

expected from the temperature dependence of the interlayer probability p.At

70 K, p is very low, so practically no atoms overcome the step edge barrier,

even for small size islands. At 130 K, however, p increases, so many atoms can

hop to the lower level. The f (T

,R)-vs-R function for ﬁxed T

is expected

to have a step-like shape, with no second-layer occupation (f = 0) for small

R, complete occupation (f =1)forlargeR and a transition region deﬁned by

a characteristic critical size R

where the function shows a sudden jump. At

lower T

the transition becomes steeper and R

moves to smaller sizes. The

data for Ag/Ag(111) are shown in Fig. 7.13(b). By measuring the f(T

,R)

curves at least at two temperatures, it is possible to separate the two contri-

butions to p, the prefactor ratio ν

/ν

and the barrier ∆E

. Qualitatively one

expects that the larger the diﬀerence in the two occupation curves f (T

,R),

measured for the same two temperatures, the larger the barrier ∆E

will be;

while the absolute value of R

determines the prefactor ratio ν

/ν

. Larger

values of R

imply larger values of ν

/ν

since the atoms must have larger

interlayer probability to compensate for the reduced probability to reach the

island edge.

322 Michael C. Tringides and Myron Hupalo

Theoretical Analysis

of Second-Layer Nucleation Experiments

The form of the function f(R, T ) depends on the theory used for the analysis.

Initially a mean ﬁeld theory was proposed [70] to relate the rate of second-

layer nucleation (Ω, i. e. number of nucleated islands/time), on top of an

island of size R,top. It is assumed that on top of the island there is a

distance-dependent monomer density c(r)(wherer is the distance from the

center of the island) as a result of the incident ﬂux. Because of the absorptive

boundary conditions at r = R, c(r)dropsoﬀwithr; but as p becomes smaller

(i.e. an atom has higher probability to be reﬂected at the island edge), the

diﬀerence between the density at r =0andr = R is reduced according to

c(r)=

(1 + 2a/pR) −

. (7.34)

Following nucleation theory [71] monomers diﬀusing in the presence of

the density c(r) on top of an island of size R will irreversibly aggregate into

second-layer islands with the rate

Ω(R)=

κD



2πr dr

[c(r)a

]

i+1

(7.35)

where D

denotes the terrace diﬀusion coeﬃcient. Integrating this expression

over the island area, it simpliﬁes in the limit of a strong step edge barrier

(p  1) to

Ω(R)=

(7.36)

with α

being a geometric factor related to the shape of the islands. The

fractional occupation of the islands, shown in Fig. 7.13b, can be obtained by

integrating the rate of change df/dt = Ω(1 − f) over the time of the second

deposition, since only islands free of second-layer nucleation can increase f.

In [70] as a main assumption of the mean ﬁeld theory it is supposed

that the monomer density c(r) on top of the ﬁrst layer island and monomer

diﬀusion determines the second layer nucleation rate Ω. This assumption has

been questioned in [72]. Instead, the nucleation of a second-layer island has

been analyzed in terms of the probability of two atoms (deposited successively

on top of the island) to diﬀuse and encounter each other. This analysis leads

to diﬀerent form of f(R,T) than the mean ﬁeld theory. Ω(R)isgivenbythe

rate of monomer deposition on top of the island of area A, FA,(withF

the ﬂux rate) times the probability for two of the deposited monomers to

encounter each other. In the limit of very fast terrace diﬀusion (e. g. about

hops/s at 130 K for Ag(111)) it can be shown that this probability is given

by the ratio of the residence time of the ﬁrst atom τ

to the time between

depositions τ =(FA)

−1

,namely