Haug H., Koch S. Quantum theory of the optical and electronic properties of semiconductors
Подождите немного. Документ загружается.


January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
Oscillator Model 9
(- )/ww w
00
ew’()
ew’’()
Fig. 1.1 Dispersion of the real and imaginary part of the dielectric function, Eq. (1.37)
and (1.38), respectively. The broadening is taken as γ/ω
0
=0.1 and
max
= ω
2
pl
/2γω
o
.
In order to understand the physical information contained in
(ω) and
(ω), we consider how a light beam propagates in the dielectric medium.
From Maxwell’s equations
curl H(r,t)=
1
c
∂
∂t
D(r,t) (1.39)
curl E(r,t)=−
1
c
∂
∂t
B(r,t) (1.40)
we find with B(r,t)=H(r,t), which holds at optical frequencies,
curl curl E(r,t)=−
1
c
∂
∂t
curl H(r,t)=−
1
c
2
∂
2
∂t
2
D(r,t) . (1.41)
Using curl curl =graddiv− ∆, we get for a transverse electric field with
divE(r, t) = 0, the wave equation
∆E(r,t) −
1
c
2
∂
2
∂t
2
D(r,t)=0 . (1.42)
Here, ∆ ≡∇
2
is the Laplace operator. A Fourier transformation of
Eq. (1.42) with respect to time yields
∆E(r,ω)+
ω
2
c
2
(ω)E(r,ω)+i
ω
2
c
2
(ω)E(r,ω)=0 . (1.43)

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
10 Quantum Theory of the Optical and Electronic Properties of Semiconductors
For a plane wave propagating with wave number k(ω) and extinction coef-
ficient κ(ω) in the z direction,
E(r,ω)=E
0
(ω)e
i[k(ω)+iκ(ω)]z
, (1.44)
we get from Eq. (1.43)
[k(ω)+iκ(ω)]
2
=
ω
2
c
2
[
(ω)+i
(ω)] . (1.45)
Separating real and imaginary part of this equation yields
k
2
(ω) − κ
2
(ω)=
ω
2
c
2
(ω) , (1.46)
2κ(ω)k(ω)=
ω
2
c
2
(ω) . (1.47)
Next, we introduce the index of refraction n(ω) as the ratio between the
wave number k(ω) in the medium and the vacuum wave number k
0
= ω/c
k(ω)=n(ω)
ω
c
(1.48)
and the absorption coefficient α(ω) as
α(ω)=2κ(ω) . (1.49)
The absorption coefficient determines the decay of the intensity I ∝|E|
2
in
real space. 1/α is the length, over which the intensity decreases by a factor
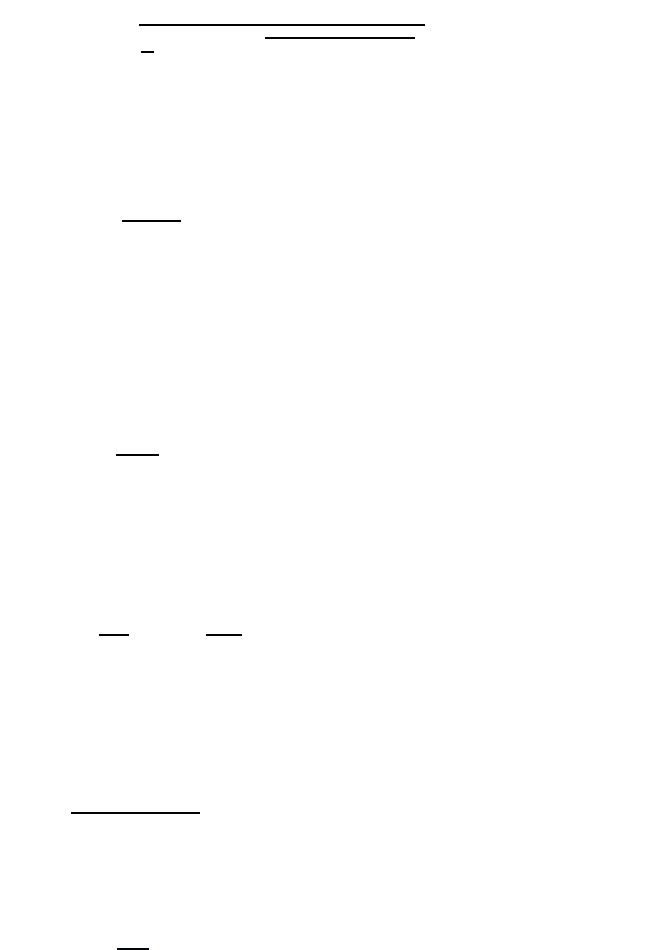
January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
Oscillator Model 11
1/e. From Eqs. (1.46) – (1.49) we obtain the relations
n(ω)=
1
2
(ω)+
2
(ω)+
2
(ω)
(1.50)
index of refraction
and
α(ω)=
ω
n(ω)c
(ω) . (1.51)
absorption coefficient
Hence, Eqs. (1.38) and (1.51) yield a Lorentzian absorption line, and
Eqs. (1.37) and (1.50) describe the corresponding frequency-dependent in-
dex of refraction. Note that for
(ω) <<
(ω),whichisoftentruein
semiconductors, Eq. (1.50) simplifies to
n(ω)
(ω) . (1.52)
Furthermore, if the refractive index n(ω) is only weakly frequency-
dependent for the ω-values of interest, one may approximate Eq. (1.51)
as
α(ω)
ω
n
b
c
(ω)=
4πω
n
b
c
χ
(ω) , (1.53)
where n
b
is the background refractive index.
For the case γ → 0, i.e., vanishing absorption line width, the line-shape
function approaches a delta function (see problem 1.3)
lim
γ→0
2γ
(ω − ω
0
)
2
+ γ
2
=2πδ (ω − ω
0
) . (1.54)
In this case, we get
(ω)=π
ω
2
pl
2ω
0
δ(ω − ω
0
) (1.55)

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
12 Quantum Theory of the Optical and Electronic Properties of Semiconductors
and the real part becomes
(ω)=1−
ω
2
pl
2ω
0
1
ω − ω
0
. (1.56)
1.3 Retarded Green’s Function
An alternative way of solving the inhomogeneous differential equation
m
0
∂
2
∂t
2
+2γ
∂
∂t
+ ω
2
0
x(t)=eE(t) (1.57)
is obtained by using the Green’s function of Eq. (1.57). The so-called
retarded Gren’s function G(t − t
) is defined as the solution of Eq. (1.57),
where the inhomogeneous term eE(t) is replaced by a delta function
m
0
∂
2
∂t
2
+2γ
∂
∂t
+ ω
2
0
G(t − t
)=δ(t −t
) . (1.58)
Fourier transformation yields
G(ω)=−
1
m
0
1
ω
2
+ i2γω − ω
2
0
= −
1
2m
0
ω
0
1
ω − ω
0
+ iγ
−
1
ω + ω
0
+ iγ
, (1.59)
retarded Green’s function of an oscillator
where ω
0
is defined in Eq. (1.8). In terms of G(t − t
), the solution of
Eq. (1.57) is then
x(t)=
+∞
−∞
dt
G(t − t
)eE(t
) , (1.60)
as can be verified by inserting (1.60) into (1.57). Note, that the general solu-
tion of an inhomogeneous linear differential equation is obtained by adding
the solution (1.60) of the inhomogeneous equation to the general solution
of the homogeneous equation. However, since we are only interested in the
induced polarization, we just keep the solution (1.60).

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
Oscillator Model 13
In general, the retarded Green’s function G(t − t
) has the properties
G(t − t
)=
finite
0
for
t ≥ t
t<t
(1.61)
or
G(τ) ∝ θ(τ) ,
where θ(τ) is the unit-step or Heavyside function
θ(τ )=
1
0
for
τ ≥ 0
τ<0
. (1.62)
For τ<0 we can close in (1.60) the integral by a circle with an infinite
radius in the upper half of the complex frequency plane since
lim
|ω|→∞
e
i(ω
+iω
)|τ |
= lim
|ω|→∞
e
iω
τ
e
−ω
|τ |
=0 . (1.63)
As can be seen from (1.59), G(ω) has no poles in the upper half plane
making the integral zero for τ<0.Forτ ≥ 0 we have to close the contour
integral in the lower half plane, denoted by C
,andget
G(τ)=−
1
2m
0
ω
0
θ(τ )
C
dω
2π
e
−iωτ
1
ω − ω
0
+ iγ
−
1
ω + ω
0
+ iγ
= iθ(τ)
1
2m
0
ω
0
[e
−(iω
0
+γ)τ
− e
(iω
0
−γ)τ
] . (1.64)
The property that G(τ)=0for τ<0 is the reason for the name retarded
Green’s function which is often indicated by a superscript r, i.e.,
G
r
(τ)=0for τ<0 ←→ G
r
(ω)= analytic for ω
≥ 0 . (1.65)
The Fourier transform of Eq. (1.60) is
x(ω)=
+∞
−∞
dt
+∞
−∞
dt
e
iω(t−t
)
G(t − t
)e
iωt
eE(t
)
= eG(ω)E(ω) . (1.66)
With P(ω)=en
0
x(ω)=χ(ω)E(ω) we obtain
χ(ω)=n
0
e
2
G(ω) (1.67)

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
14 Quantum Theory of the Optical and Electronic Properties of Semiconductors
χ(ω)=−
n
0
e
2
2mω
0
1
ω − ω
0
+ iγ
−
1
ω + ω
0
+ iγ
(1.68)
in agreement with Eq. (1.7).
This concludes the introductory chapter. In summary, we have dis-
cussed the most important optical coefficients, their interrelations, analytic
properties, and explicit forms in the oscillator model. It turns out that this
model is often sufficient for a qualitatively correct description of isolated
optical resonances. However, as we progress to describe the optical proper-
ties of semiconductors, we will see the necessity to modify and extend this
simple model in many respects.
REFERENCES
For further reading we recommend:
J.D. Jackson, Classical Electrodynamics,2nded., Wiley, New York, (1975)
L.D. Landau and E.M. Lifshitz, The Classical Theory of Fields,3rded.,
Addison–Wesley, Reading, Mass. (1971)
L.D. Landau and E.M. Lifshitz, Electrodynamics of Continuous Media,
Addison–Wesley, Reading, Mass. (1960)
PROBLEMS
Problem 1.1: Prove the Dirac identity
1
r ∓ i
= P
1
r
± iπδ(r) , (1.69)
where → 0 and use of the formula under an integral is implied.
Hint: Write Eq. (1.69) under the integral from −∞ to +∞ and integrate
in pieces from −∞ to −,from− to + and from + to +∞.
Problem 1.2: Derive the Kramers–Kronig relation relating χ
(ω) to the
integral over χ
(ω).
Problem 1.3: Show that the Lorentzian
f(ω)=
1
π
γ
(ω − ω
0
)
2
+ γ
2
(1.70)
January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
Oscillator Model 15
approaches the delta function δ(ω − ω
0
) for γ → 0.
Problem 1.4: Verify Eq. (1.56) by evaluating the Kramers–Kronig trans-
formation of Eq. (1.55). Note, that only the resonant part of Eq. (1.22)
should be used in order to be consistent with the resonant term approxi-
mation in Eq. (1.36).
Problem 1.5: Use Eq. (1.13) to show that
∞
−∞
dω e
iωt
=2πδ(t).
This page intentionally left blank

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
Chapter 2
Atoms in a Classical Light Field
Semiconductors like all crystals are periodic arrays of one or more types
of atoms. A prototype of a semiconductor is a lattice of group IV atoms,
e.g. Si or Ge, which have four electrons in the outer electronic shell. These
electrons participate in the covalent binding of a given atom to its four
nearest neighbors which sit in the corners of a tetrahedron around the given
atom. The bonding states form the valence bands which are separated by an
energy gap from the energetically next higher states forming the conduction
band.
In order to understand the similarities and the differences between op-
tical transitions in a semiconductor and in an atom, we will first give an
elementary treatment of the optical transitions in an atom. This chap-
ter also serves to illustrate the difference between a quantum mechanical
derivation of the polarization and the classical theory of Chap. 1.
2.1 Atomic Optical Susceptibility
The stationary Schrödinger equation of a single electron in an atom is
H
0
ψ
n
(r)=
n
ψ
n
(r) , (2.1)
where
n
and ψ
n
are the energy eigenvalues and the corresponding eigen-
functions, respectively. For simplicity, we discuss the example of the hy-
drogen atom which has only a single electron. The Hamiltonian H
0
is then
given by the sum of the kinetic energy operator and the Coulomb potential
in the form
H
0
= −
2
∇
2
2m
0
−
e
2
r
. (2.2)
17

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2
18 Quantum Theory of the Optical and Electronic Properties of Semiconductors
An optical field couples to the dipole moment of the atom and introduces
time-dependent changes of the wave function
i
∂ψ(r,t)
∂t
=[H
0
+ H
I
(t)]ψ(r,t) (2.3)
with
H
I
(t)=−exE(t)=−dE(t) . (2.4)
Here, d is the operator for the electric dipole moment and we assumed that
the homogeneous electromagnetic field is polarized in x-direction. Expand-
ing the time-dependent wave functions into the stationary eigenfunctions
of Eq. (2.1)
ψ(r,t)=
m
a
m
(t)e
−i
m
t
ψ
m
(r) , (2.5)
inserting into Eq. (2.3), multiplying from the left by ψ
∗
n
(r) and integrating
over space, we find for the coefficients a
n
the equation
i
da
n
dt
= −E(t)
m
e
−i
mn
t
n|d|ma
m
, (2.6)
where
mn
=
m
−
n
(2.7)
is the frequency difference and
n|d|m =
d
3
rψ
∗
n
(r)dψ
m
(r) ≡ d
nm
(2.8)
is the electric dipole matrix element. We assume that the electron was
initially at t →−∞in the state |l, i.e.,
a
n
(t →−∞)=δ
n,l
. (2.9)
Now we solve Eq. (2.6) iteratively taking the field as perturbation. For this
purpose, we introduce the smallness parameter ∆ and expand
a
n
= a
(0)
n
+∆a
(1)
n
+ ... (2.10)
and
E(t) → ∆E(t) . (2.11)
