Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

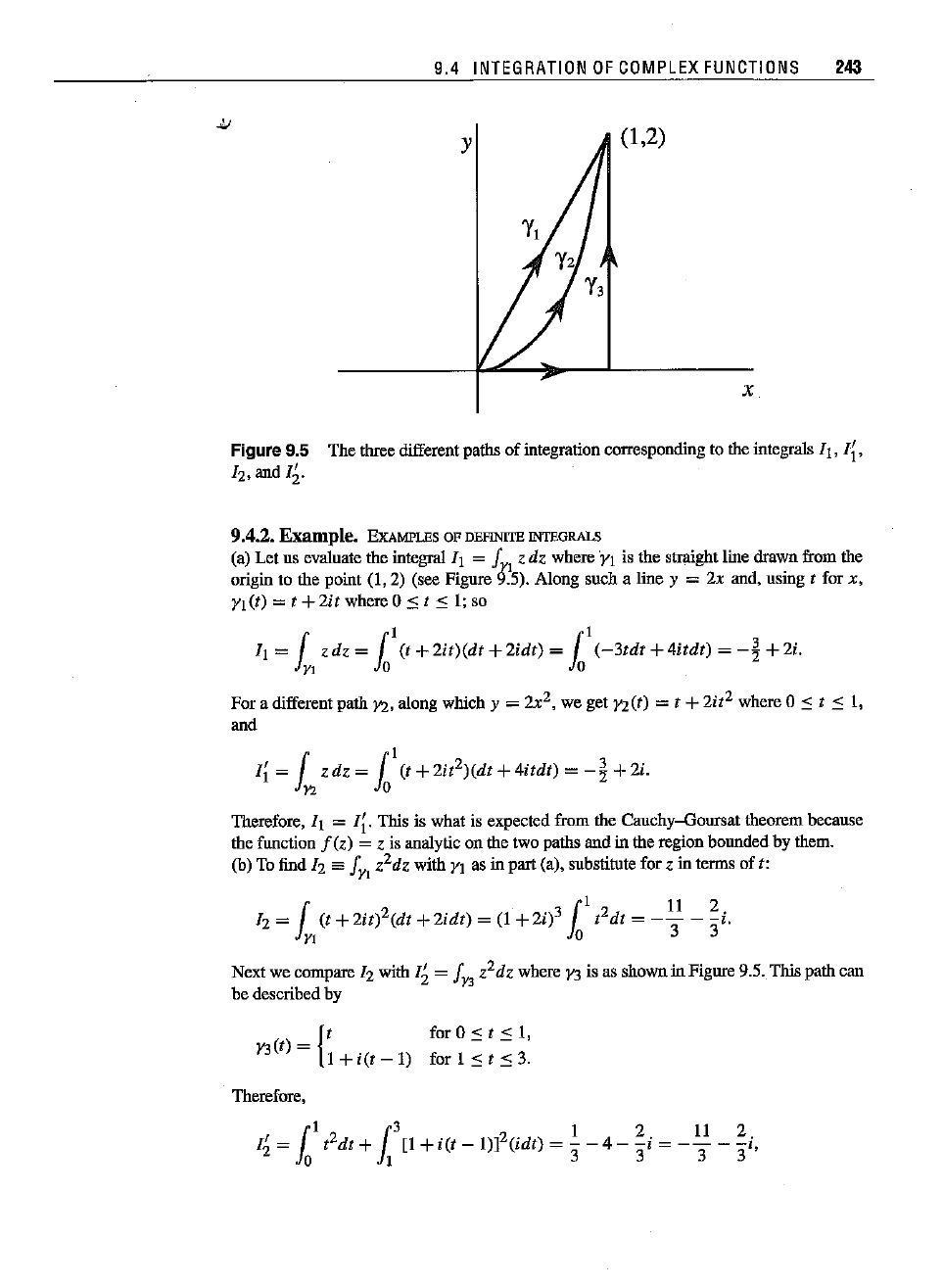
9.4
INTEGRATION
OF
COMPLEX
FUNCTIONS
243
y
(1,2)
x
Figure9.5 The
three
different
paths
of
integration
corresponding
tothe
integrals
II. If.
Iz.
and
I~.
9.4.2. Example. EXAMPLESOF
DEFINITE
INTEGRALS
(a) Let us evaluate the integral II = f
Y1
z dz where ·YI is the straight line drawn from the
origin to the point
(1.2)
(see Figure 9.5). Along such a line y = 2x and. using t for x.
YI(t) =t
+2it
where 0
:s
t:s 1; so
II
= 1z dz =
[\t
+
2it)(dt
+
2idt)
=
[I
(-3tdt
+
4itdt)
=
-~
+u.
)'1
io
10
For a different path)'2. along which y = 2x
2
•
we get )'2(t) = t +
2it
2
where 0
:s
t
:s
1.
and
Ii =
Ir,
zdz
=
10
1
(t +
2it
2)(dt
+
4itdt)
=
-~
+2i.
Therefore,
II = If. Thisis
what
is
expected
fromthe
Cauchy-Goursat
theorem
because
the
function
fez)
= zis
analytic
onthetwo
paths
andintheregion
bounded
by
them.
(h) To find
Iz
es f
Y1
z
2dz
with
YI
as in part (a). suhstitute for z in terms of t:
1
10
1 11 2
Iz
= (t +2it)2(dt +
2idt)
= (1+2i)3 t
2dt
=
--3
-
-3
i,
n 0
Nextwe
compare
h
with
I~
= f
Y3
z
2
dz
where
Y3
is as shown
in
Figure 9.5. Thispathcan
be
described
by
n(t)
= g
+i(t
-1)
Therefore,
for 0 s t
.:::
1,
for 1 s t
:::::
3.
,
10
1
2
/,3
2 1 2 11 2
1
2
= t dt + [1 +
itt
- 1)]
(idt)
= - - 4 -
-i
=
--
- -zt,
o 1 3 3 3 3
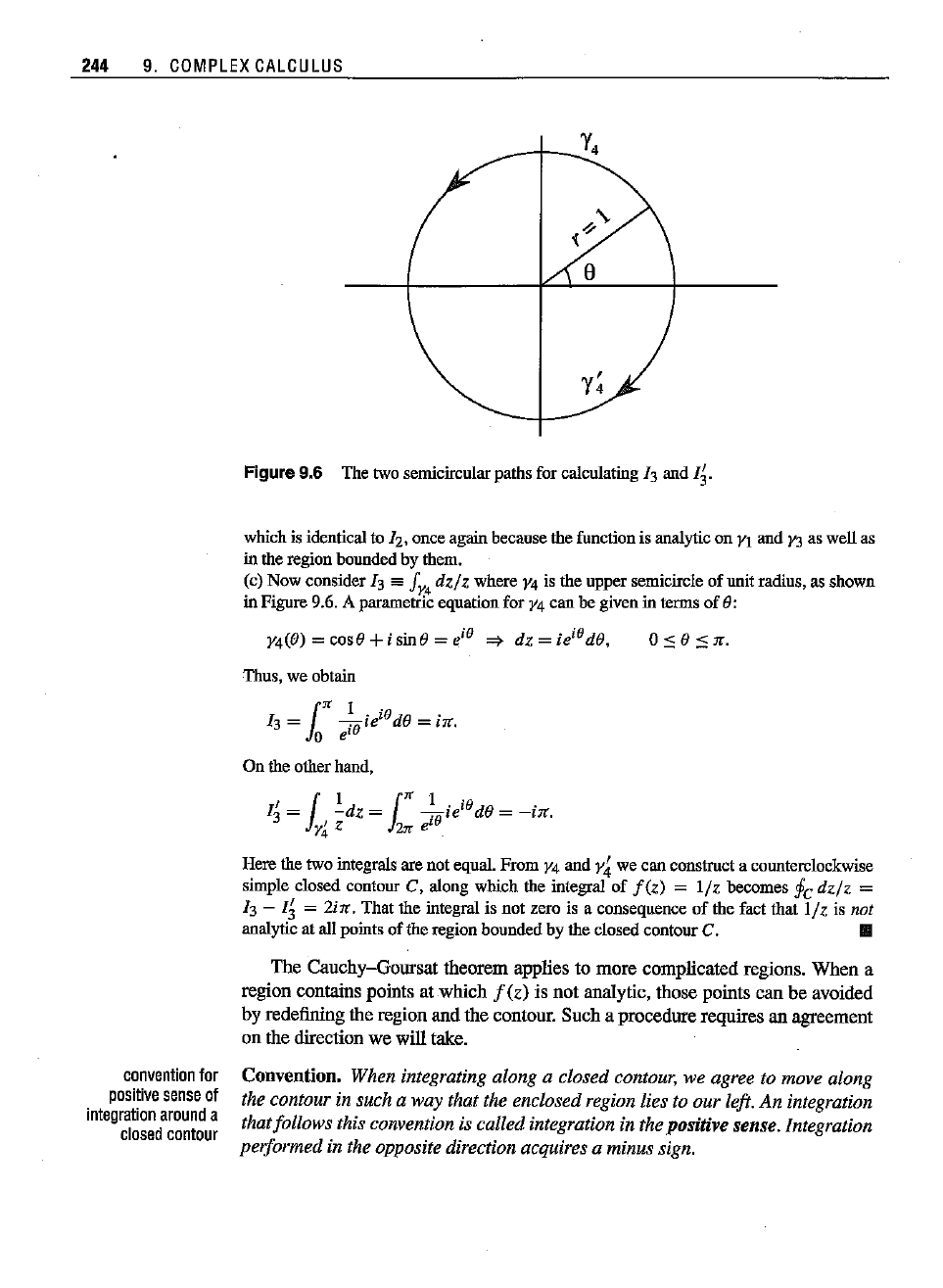
244 9.
COMPLEX
CALCULUS
Figure 9.6 Thetwo
semicircular
paths
forcalculating h and
I~.
whichis
identical
to12.onceagainbecausethefunction is
analytic
on Yland
Y3
aswell as
in theregion
bounded
by
them.
(c) Now consider /3 es f
Y4
dz/z where
Y4
is the
upper
semicircle
of
unit
radius,
asshown
in Figure 9.6. A
parametric
equation
for
Y4
canbegiveninterms of
():
O:::::8::S1L
convention
for
positive
sense
of
integretion
around
a
closed
contour
Thus,we
obtain
Onthe otherhand,
, 1t
j,"
t . te .
1
3
=
-dz
=
~,e
dO =
-llr.
Y~
Z 2n e
l
Herethetwo
integrals
arenot
equal.
From
Y4
and
Y~
we can
construct
acounterclockwise
simpteclosed contour C, along which the integral of
f(z)
=
l/z
becomes
Jc
dz/z
=
/3 -
I~
= liT(. Thatthe
integral
is not zero is a consequence of thefact
that
liz
is not
analytic
atallpointsof theregion
bounded
bytheclosed
contour
C.
..
The Cauchy-Goursat theorem applies to more complicated regions. When a
region contains points at which
f(z)
is not analytic, those points can be avoided
by redefining the region and the contour. Such a procedure requires an agreement
on the direction we will take.
Convention.
When integrating along a closed contour, we agree to move along
the contour in such a way that the enclosedregion lies to our left. An integration
thatfollows this convention is called integration in the positive sense. Integration
performed in the opposite direction acquires a minus sign.

9.4
INTEGRATION
OF
COMPLEX
FUNCTIONS
245
y
o
x
simply
and
multiply
connected
regions
Cauchy
Integral
Formula
Figure 9.7 A complicatedcontourcan be brokenup into simpler ones. Note thatthe
boundaries
of the
"eyes"
andthe
"mouth"
are forced to be
traversed
in the
(negative)
clockwise
direction.
For asimple closed contour, movementin the counterclockwisedirectionyields
integration inthe positive sense. However, as the contour becomes more compli-
cated, this conclusionbreaks down. Figure9.7 shows a complicatedpath enclosing
a region (shaded) in which the integrand is analytic. Note that it is possible to tra-
verse a portion of the region twice in opposite directions without affecting the
integral, which may be a sum of integrals for different pieces of the contour. Also
note
that
the
"eyes"
and
the
"mouth"
are
traversed
clockwise!Thisis necessary
because
of
the convention above. A region such as that shown in Figure 9.7, in
which holes are "punched out," is called
multiply
connected. In contrast, a sim-
ply connected region is one in which every simple closed contour encloses only
points
of
the region.
One important consequence of the Cauchy-Goursat theorem is the following:
9.4.3.
Theorem.
(Cauchy iutegral formula) Let ! be analytic on and within a
simple closed contour C integrated in the positive sense. Let
zo
be
any interior
point to C. Then
I i
!(z)
!(ZO) =
-.
--dz
2:m c z - zo
To prove the Cauchy integral formula (CIF), we need the following lemma.
9.4.4.
Lemma.
(Darboux inequality) Suppose ! : C
-+
iC
is continuous and
boundedon a path
y,
i.e.•there exists a positive number M such that
1!(z)1
:::
M
246 9.
COMPLEX
CALCULUS
for all values z E y. Then
where L
y
is the length
of
the path
of
integration.
Proof
11
f(Z)dZI
=
IN~ootf(Zi)!!.Zil
=
J!!!'oo
It
f(Zi)!!.ZiI
y
Li.Zi--+O
l=l
.6.Zj--+O
1=1
N N
s lim
''If(zi)!!.ziI
= lim L If(Zi)1
l!!.ziI
N--+oo !-- N--+oo .
..6.zi--+O
1=1 8Zj--+O 1=1
N
s M lim L
l!!.ziI
=
ML
y
•
N-*oo.
1
Li.zi--+O
1=
The first inequalityfollows from the triangle inequality, the second from the bound-
edness of
f,
and the last equality follows .from the definition
of
the length
of
a
path. D
Now we are ready to prove the Cauchy integral formula.
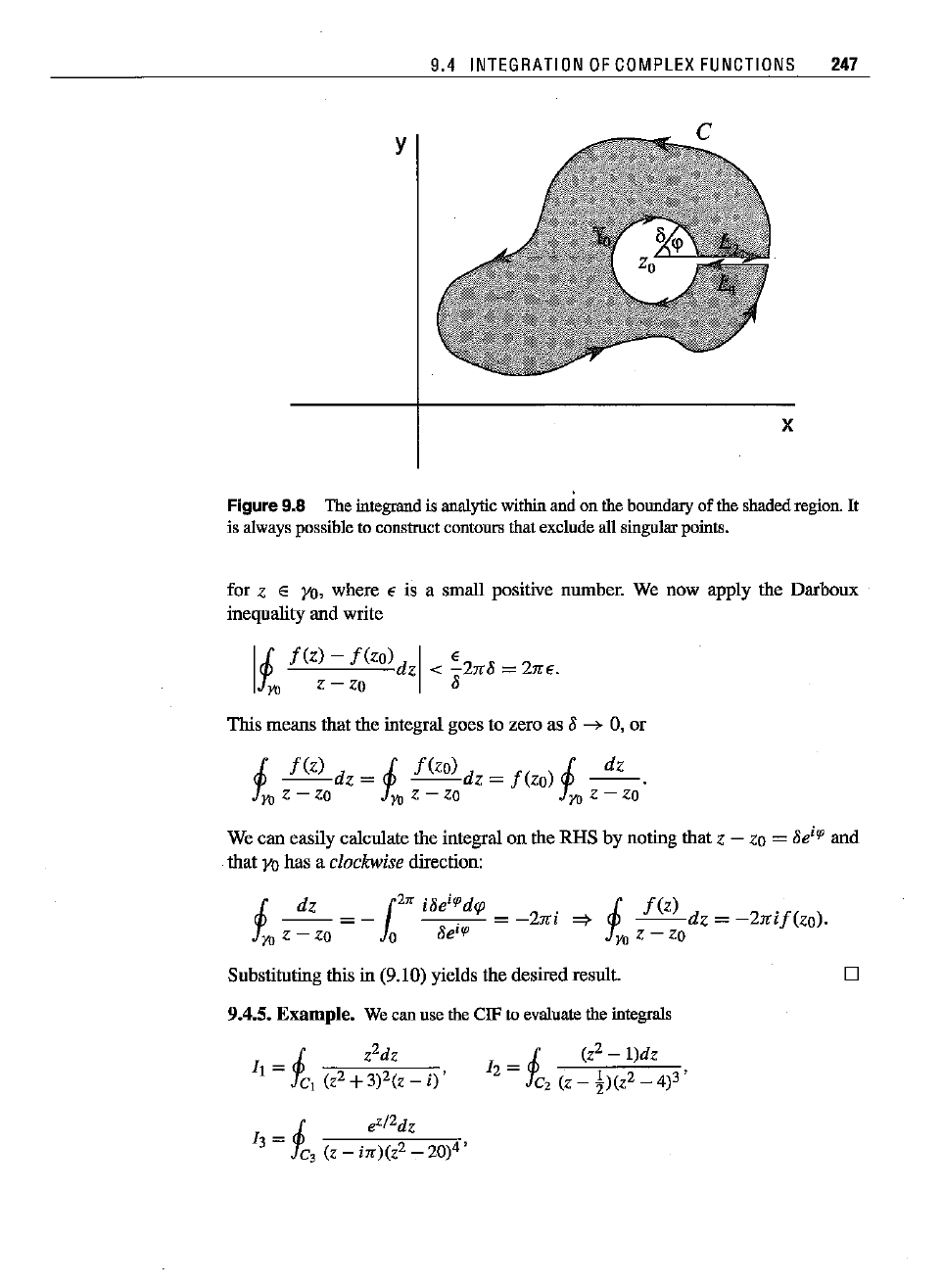
ProofofCIF. Consider the shaded region in Figure 9.8, which is bounded by C,
by
YO
(a circle of arbitrarily small radius 8 centered at zo), and by
Ll
and L2, two
straightline segments infinitesimally close to one another (we can, in fact, assnme
that
L1 and L2 are right on top
of
one another; however, they are separated in the
figure for clarity). Let us use
C'
to denote the union of all these curves.
Since
f(z)/(z
- zo) is analytic everywhere on the contour
C'
and inside the
shaded region, we can write
0=
_1_ 1
f(z)
dz (9.10)
2ni f
e
! z -
zo
=
_1_[1
f(z)
dz
+1
f(z)
dz
+1
f(z)
dz
+1
f(z)
dzJ.
2ni
rc
z -
zo
fro
Z -
zo
hI Z - zo h
l
Z -
zo ,
=0
The contributions from L1 and L2 cancel becausethey are integrals along the sarne
line segment in opposite directions. Let us evaluate the contribution from the in-
finitesimal circle
yo.First we note that becausef (z) is continuous (differentiability
implies continnity), we can write
I
f(z)
-
f(zo)
I=
If(z)
-
f(zo)
I =
If(z)
-
f(zo)!
z-zo
Iz-zol 8
•
<-
8
9.4
INTEGRATION
OF
COMPLEX
FUNCTIONS
247
y
x
Figure 9.8 The
integrand
is
analytic
withinandonthe
boundary
of the
shaded
region.
It
is alwayspossibleto
construct
contours
that
excludeall
singular
points.
for Z E
JIO,
where.
is a small positive number. We now apply the Darbonx
inequality and write
1
1.
!(z)
- !(zo)dzi < =-2,,8 =
2"
•.
fro
z-zo
8
This means that the integral goes to zero as 8
--->
0, or
1.
!(z)
dz =
1.
!(zo) dz = !(zo)
1.
.ss.;
froz-zo froz-zo
froz-zo
We can easily calculate the integral on the RHS by noting that z -
zo
= 8e
i
•
and
that
JIO
has a
clockwise
direction:
i
dz 1
2
"
i8e
i·d<p
i
!(z)
--
= - . =
-2"i
=}
--dz
=
-2"i!(zo).
Yo
z - zo 0 8e
' qJ
Yo
Z - zo
Snbstitnting this in (9.10) yields the desired result.
9.4.5. Example. Wecanusethe
elf
to
evaluate
theintegrals
D
fc
(z2 -
I)dz
Iz=·
,
c,
(z-
iHz
2
-
4)3

248
9.
COMPLEX
CALCULUS
where
CI, e2, and
C3
are
circles
centered
atthe
origin
with
radii
71 = 3/2,72 = I,
and
73
=4.
For
lj
wenotethat
/(z)
= z2/(z2 + 3)2is analyticwithinandonC\oandzc = i lies
inthe
interior
of C1.
Thus,
t
/(z)dz
..
. i
2
.st
II =
--.
=
2",/(,)
=
21"'2
2 =
-'-2'
c, z
-,
(, + 3)
Similarly,
/(z)
= (z2 - 1)/(z2 - 4)3 forthe integral
Iz
is analytic on andwithinC2, and
ZQ
=
1/2
is aninterior point of C2. Thus, the Cllt gives
i
/(z)dz
32".
Iz
=
--1
=
2".i/(1/2)
=
--5
i
.
C,z-:;:
112
Forthe lastintegral, / (z) = e
z/
2/
(z2 - 20)4,andtheinteriorpointis zu =
iit
:
2".
III
(9.11)
Explanation
at
why
the
Cauchy
integral
formula
works!
The Cauchy integral formula gives the value
of
an analytic function at every
point inside a simple closed contour when it is given the value of the function
only at points on the contour.
It
seems as though an analytic function is not free to
change
insidea
region
onceitsvalueis fixedonthe
contour
enclosing
that
region.
Thereis an analogons sitnation in electrostatics: The specification
of
the poten-
tial at the boundaries, such as the surfaces
of
conductors, automatically determines
the potential at any other point in the region
of
space bounded by the conductors.
This is the contentof the uniqueness theoremused in electrostatic boundary value
problems. However, the electrostatic potential
<I>
is bound by another condition,
Laplace's equation; and the
combination
of
Laplace's equation and the boundary
conditions furnishes the uniqueness of
<1>.
Similarly, the real and imaginary parts
of
an analytic function separately satisfy Laplace's equation in two dimensions!
Thus, it should come as no surprise that the value
of
an analytic function on a
boundary (contour) determines the function at all points inside the boundary.
9.5 Derivatives as Integrals
The Cauchy Integral Formula is a very powerful tool for working with analytic
functions. One
of
the applications of this formula is in evaluating the derivatives
of such functions.
It
is convenient to change the dununy integration variable to
~
and write the
ClF
as
f(z)
=
---.!..."
1.
f(~)
d~
,
2".,
fc
~
- z
where C is a simple closed contour in the
~
-plane and z is a point within C.
As preparation for defining the derivative
of
an analytic function, we need the
following result.

(9.12)
derivative
of
an
analytic
function
given
in
terms
of
an
integral
9.5
DERIVATIVES
AS
INTEGRALS
249
9.5.1. Proposition.
Let
y be any
path---<z
contour,for
example-and
g a contin-
uousfunction on that path. Thefunction
f(z)
defined by
f(z)
=
~
1
g(;)
d;
2:n:z
y ; - z
is analytic at every pointz ¢ y.
Proof The
proof
follows immediately from differentiation
of
the integral:
df
=_1
~lg(;)d;
=_1
19(;)d;~(_I_)=_1
1
g(;)d;.
dz
2:n:i
dz
y ; - z
2:n:i
y
dz
; - z
2:n:i
y
(;
- Z)2
This is defined for all values
of
z not on y.9 Thus,
f(z)
is analytic there. D
We can generalize the formula above to the
nth
derivative, and obtain
d
n
f nl 1
g(;)d;
dz
n
=
2:n:i
y
(;
- z)n+1.
Applyingthis resulttoan analytic function expressedby Equation
(9.11),
weobtain
the following important theorem.
9.5.2.
Theorem.
The derivatives
of
all orders
of
an analytic function f (z) exist
in the domain
of
analyticity
of
the function and are themselves analytic in that
domain. The nth derivative
of
f(z)
is given by
f(n)(z) = d
n
f =
~
1
f(;)
d;
dz"
2:n:i
ic
(;
- z)n+1
9.5.3. Example. Let us apply Bquation (9.12) directly 10some simple functions. In all
. cases,we will assume
that
the
contour
is a circleof
radius
r centeredatz.
(a) Lei
f(z)
= K, a constant. Then, for n = 1 we have
df
__
1_1
~
dz -
2"i
f
c
(I; - z)2.
Since
~
is onthe
circle
C
centered
atz,g- z =re
i
fJ
and
dg=
riei~
d8.Sowe
have
df
= _1_
r:
Kire
iO
dO
=
~
f2rr
e-lO
so
=
O.
dz
2"i
1
0
(re
lO
)2
Zatr
10
.
(b) Given f (z) = z, its first derivative will be
df
= _1_ 1
~
= _1_ r: (z+relO)irelOdO
dz
2ni
f
c
(I; - z)2
2"i
10
(re,O)2
=
~
(:
t"
e-lOdO
+
t"
-)
=
~(O+2")
=
I.
2n
rio
10
21l'
,
9The
interchange
of
differentiation
and
integration
requires
justification. Suchan
interchange
canbe doneif the
integral
has
some
restrictive
properties.
Weshallnot
concern
ourselves withsuch
details.
Infact,one canachievethe same
result
byusing
the
definition
of
derivatives
andtheusual
properties
of
integrals.

250 9.
COMPLEX
CALCULUS
(c)Given
f(z)
= z2, for the firstderivative Equation (9.12)yields
df
= _1_
1.
g2dg = _1_
{2n
(z +re
i9)2ireiO
dB
dz 'Iitl
fc
(g
- z)2
2"i
10
(re,0)2
=
~
r:
[z2 +(rei9)2"+
2zre'0j
(reiO)-ldB
2"
1
0
I
(z2
fo2n"
fo2n .
foh)
=- -
e-'OdB+r
e'OdB+2z
dB
ee
Zz,
2n
r 0
'0
0
It can be shown that, in general,
(d/dz)z"
=
mz
..
-
l.
The proof is left as Problem
9.30. III
The
CIF
is a central formula in complex aualysis, and we shall see its sig-
nificance in much
of
the later development
of
complex analysis.
For
now, let us
demonstrate its usefulness in proving a couple
of
important properties
of
analytic
functions.
9.5.4.
Proposition.
The absolute value
of
an analyticfunction
f(z)
cannot have
a local maximum within the region
of
analyticity
of
the function.
Proof
Let
S c
<C
be the region
of
analyticity
of
f.
Suppose zo E S were a local
maximum. Thenwe couldfind a circle
jn
of
small
enough
radius 8, centered at zo,
such that If(zo)1 > If(z)1 for all Z on )/0. We now show that this cannothappen.
Using the CIF, and noting that z -
zo = 8e
iO
,
we have
If(zo)1 =
I~,[
f(z)
dzl
=
_I
I
{2n
f(z)i8e
i9de
I
2".,
fro
z-
zo
2"
10
8e'0
s
~
{2n
If(z)!de
s
_I
r:
use =
M,
2"
1
0
2"
10
where M is the maximum value
of
If(z)1 for z E )/0. This inequality says that
there is at least one
point
z on the circle )/0 (the
point
at which the maximum
of
!f(z)1
is attained) such that If(zo)1 :::
If(z)l.
This contradicts
our
assumption.
Therefore, there can be no local
maximum
within S. D
9.5.5.
Proposition.
A boundedentire function is necessarily a constant.
Proof
We show that the derivative
of
such a function is zero. Consider
df
1,[
f(g)
dg
dz
=
2"i
f
c
(g
-
Z)2
•
Since f is an entirefunction, the closedcontour C can be chosen to be a very large
circle
of
radius R with center at z. Taking the absolute value
of
both sides yields

fundamental
theorem
of
algebra
proved
9.5
DERIVATIVES
AS
INTEGRALS
251
where M is
the
maximum
of
the
function in
the
complexplane. Now, as R
--->
00,
the
derivative goes to zero, and
the
function
must
be a constant. D
Proposition 9.5.5 is a very powerful statement
about
analytic functions. There
are
many
interestingand nontrivialreal functions that
are
bounded
and
have
deriva-
tivesof all
orders
onthe
entire
real line. For
instance,
e-
x2
is sucha
function.
No
such freedom exists for complex analytic functions.
Any
nontrivial analytic func-
tion is either
not
bounded (goes to infinity somewhere on
the
complex plane) or
not entire (it is
not
analytic at some point(s)
of
the
complex plane).
A consequence
of
Proposition 9.5.5 is
the
fundamental
theorem
of
algebra,
which states that any polynomial
of
degree n
2':
1 has n roots (some
of
which
may
be repeated).
In
other words,
the
polynomial
p(x)
=
aD
+alX
+...
+anx
n
for n,
2':
I
can
be factored completely as
p(x)
=
c(x
- Zl)(X -
zz)···
(x - Zn) where c is a
constant
and
theZi
are,
in
general.
complexnumbers.
To see how Proposition 9.5.5 implies
the
fundamental theorem
of
algebra, we
let
f(z)
=
l/p(z)
and assume the contrary, i.e., that
p(z)
is never zero for any
(finite)
Z E C.
Then
f(z)
is
bounded
and
analytic for all z E
C,
and Proposition
9.5.5 says that
f (z) is a constant.
This
is obviously wrong. Thus, there
mnst
be at
leastone z, say z
=
zi,
for which
p(z)
is zero. So, we
can
factor
out
(z -
Zl)
from
p(z)
and
write
p(z)
= (z - Zl)q(Z) where
q(z)
is
of
degree n - 1. Applying
the
above argument to
q(z),
we
have
p(z)
= (z -
Zl)(Z
-
zz)r(z)
where
r(z)
is
of
degree n - 2. Continning in this way, we
can
factor
p(z)
into linear factors.
The
last
polynomial will be a constant (a polynomial
of
degree zero) which we have
denoted by c.
The
primitive (indefinite integral)
of
an analytic function
can
be defined using
definite integrals
just
as in the real case.
Let
f : C
--->
C be analytic in a region
S
of
the
complex plane.
Let
zo
and
z be two points in S,
and
defmel''
F(z)
es
fz:
f(l;)
d~.
We
can
show that
F(z)
is the primitive
of
f(z)
by showing that
lim
I
F(z
+t>z) -
F(z)
-
f(z)1
= o.
.6.z--+O
f).z
We leave the details as a problem for the reader.
9.5.6.
Proposition.
Let
f : C
--->
C be analytic in a region S
of
C. Then at every
point
z E S,there exists an analyticfunction F : C
--->
C such that
dF
dz
=
f(z).
lONate
that
the
integral
is
path-independent
duetothe
analyticity
of
f.
Thus,
F is well-defined.

252 9.
COMPLEX
CALCULUS
In the sketchof the proofof Proposition9.5.6, we used only the continuityof f
and the fact that the integral was well-defined. These two conditions are sufficient
to establish the analyticity
of
F
and
f,
since the latter is the derivative of the
former. The following theorem, due to Morera, states this fact and is the converse
of the Cauchy-Goursat theorem.
9.5.7.
Theorem.
(Morera's theorem)
Let
afunction f :
iC
-+
iC
be continuous in
a simply connected region
S.
If
for
each simple closed contour C in S we have
:Fe
f(~)
d~
= 0, then f is analytic throughout S.
9.6 Taylor and Laurent Series
The expansion
of
functions in terms of polynomials or monomials is important
in calculus and was emphasized in the analysis of Chapter 5. We now apply this
concept to analytic functions.
9.6.1 Properties of Series
absolute
convergence
power
series
circle
of
convergence
The readeris assumedto have some familiarity with complexseries. Nevertheless,
we state (without proof) the most important properties of complex series before
discussing Taylor and Laurent series.
A complexseries is said to converge
absolutely
if
the real series
L~o
IZkl
=
L~o
J
x~
+
y~
converges. Clearly, absolute convergence implies convergence.
9.6.1. Proposition.
If
the
power
series
L~o
ak(Z -
ZO)k
converges
for
Zl
i'
zo,
then it converges absolutely
for
every value
of
Z such that Iz-
zol
< IZI -
zol.
Similarly ifthe power series
L~o
bk/(Z
-
ZO)k
converges
for
Z2
i'
zo.
then it
converges absolutely
for
every value
of
z such that [z-
zol
>
IZ2
- zn].
A geometric interpretation
of
this proposition is that if a power
series-with
positive powers--converges for a point at a distance 71 from ZQ, then it converges
for all interior points of the circle whose center is zo, and whose radius is
r\.
Similarly, if a power
series-with
negative
powers-converges
for a point at a
distance r2 from zo, then it converges for all exterior points
of
the circle whose
center is zo and whose radius is
r:
(see Figure 9.9). Generally speaking, positive
powers are used for points inside a circle and negative powers for points outside
it.
The largest circle about zo such that the first power series
of
Proposition9.6.1
converges is called the circle
of
convergence of the power series. The propo-
sition implies that the series carmot converge at any point outside the circle of
convergence. (Why?)
In determining the convergence of a power series
00
S(z) es
I>n
(z-
zo)".
n=O
(9.13)
