Hassani S. Mathematical Methods: For Students of Physics and Related Fields
Подождите немного. Документ загружается.


214 Planar and Spatial Vectors
6.22. Acoordinatesystem(R, Θ,φ) in space is defined by
x = R cos Θ cos φ + b cos φ
y = R cos Θ sin φ + b sin φ
z = R sin Θ
where b is a constant, and 0 <R<b. Using the Jacobian for three variables
(6.66), find the element of volume for this coordinate system.
Chapter 7
Finite-Dimensional Vector
Spaces
Human visual perception of dimension is limited to two and three, the plane
and space. However, his mental perception, and his ability to abstract, rec-
ognizes no bounds. If this abstraction were a mere useless mental exercise,
we would not bother to add this chapter to the book. It is an intriguing
coincidence that Nature plays along with the tune of human mental abstrac-
tion in the most harmonious way. This harmony was revealed to Hermann
Minkowski in 1908 when he convinced physicists and mathematicians alike,
that the most natural setting for the newly discovered special theory of rel-
ativity was a four-dimensional space. Eight years later, Einstein used this
concept to formulate his general theory of relativity which is the only viable
theory of gravity for the large-scale structure of space and time. In 1921,
Kaluza, in a most beautiful idea, unified the electromagnetic interaction with
gravity using a five-dimensional spacetime. Today string theory, one of the
most promising candidates for the unification of all forces of nature, uses
11-dimensional spacetime; and the language of quantum mechanics—a the-
ory that describes atomic, molecular, and solid-state physics, as well as all
of chemistry—is best spoken in an infinite-dimensional space, called Hilbert
space.
The key to this multidimensional abstraction is Descartes’ ingenious idea
of translating Euclid’s geometry into the language of coordinates whereby the
abstract Euclidean point in a plane is given the two coordinates (x, y), and
that in space, the three coordinates (x, y, z), where x, y,andz are real num-
bers. Once this crucial step is taken, the generalization to multidimensional
spaces becomes a matter of adding more and more coordinates to the list:
(x, y, z, w) is a point in a four-dimensional space, and (x, y, z, w, u) describes
a point in a five-dimensional space. In the spirit of this chapter, we want to
identify points with vectors as in the plane and space, in which we drew a

216 Finite-Dimensional Vector Spaces
directed line segment from the origin to the point in question. In general, an
n-dimensional Cartesian vector x is
n-dimensional
Cartesian vector
x =(x
1
,x
2
,...,x
n
) (7.1)
in which x
j
is called the jth component of the vector. These have all the
properties expected of vectors: You can add them
x + y =(x
1
,x
2
,...,x
n
)+(y
1
,y
2
,...,y
n
) ≡ (x
1
+ y
1
,x
2
+ y
2
,...,x
n
+ y
n
),
you can multiply a vector by a number
αx = α(x
1
,x
2
,...,x
n
) ≡ (αx
1
,αx
2
,...,αx
n
),
and the zero vector is 0 =(0, 0,...,0). Two vectors are equal if and only if
their corresponding components are equal. Sometimes, it will be convenient
to denote these vectors as columns rather than rows.
The set of real numbers, or the set of points on a line, is denoted by R.
It is common to denote the set of points in a plane—or, in the language of
Cartesian coordinates, the set of pairs of real numbers (x, y)—by R
2
,and
the set of points in space by R
3
. Generalizing this notation, we denote the
set of points in the n-dimensional Cartesian space by R
n
.Wenowhavean
infinite collection of “spaces” of various dimensions, starting with the one-
dimensional real line R
1
= R, moving on to the two-dimensional plane R
2
,
and the three-dimensional space R
3
, and continuing to all the abstract spaces
R
n
with n ≥ 4. The concepts of linear combination, linear independence,andstandard basis of
R
n
basis are exactly the same as before. The vectors
ˆ
e
1
≡ (1, 0,...,0),
ˆ
e
2
≡ (0, 1,...,0), ...
ˆ
e
n
≡ (0, 0,...,1) (7.2)
form a basis for R
n
, called the standard basis.
7.1 Linear Transformations
A linear transformation or a linear operator is a correspondence thatformal definition
of a linear
transformation or
a linear operator
takes a vector in one space and produces a vector in another space in such a
way that the operation of summation of vectors and multiplication of vectors
by numbers is preserved. If we denote the linear transformation by T,then
in mathematical symbolism, the above statement becomes
T(αx + βy)=αT(x)+βT(y). (7.3)
Matrices are prototypes of linear transformations. In fact, we saw earlier
that it was possible to transform vectors in the plane to vectors in space
and vice versa via 3 × 2or2× 3 matrices. We did not attempt to verify
Equation (7.3) for those transformations, but the reader can easily do so.

7.1 Linear Transformations 217
In fact, denoting vectors of R
n
and R
m
by column vectors, we can immediately
generalize Equations (6.36) and (6.37) to
⎛
⎜
⎜
⎜
⎝
α
1
α
2
.
.
.
α
m
⎞
⎟
⎟
⎟
⎠
=
⎛
⎜
⎜
⎜
⎝
a
11
a
12
... a
1n
a
21
a
22
... a
2n
.
.
.
.
.
.
.
.
.
a
m1
a
m2
... a
mn
⎞
⎟
⎟
⎟
⎠
⎛
⎜
⎜
⎜
⎝
α
1
α
2
.
.
.
α
n
⎞
⎟
⎟
⎟
⎠
or a
= Aa, (7.4)
where A is an m × n matrix—i.e., it has m rows and n columns—whose
elements a
ij
are real numbers. The reader may verify that Equation (7.4) is
a linear transformation that maps vectors of R
n
to those of R
m
.
Other linear operators of importance are various differential operators,
derivative is a
linear operator
i.e., derivatives of various order. For example, it is easily verified that d/dx
is a linear operator acting on the space of differentiable functions.
1
This is
because
d
dx
(αf + βg)=α
df
dx
+ β
dg
dx
for α and β real constants. Similarly d
2
/dx
2
and derivative of higher orders, as
well as partial derivatives of various kinds and orders, are all linear operators.
In fact, even when these derivatives are multiplied by functions (on the left),
they are still linear. In particular, the second-order linear differential operator
second-order linear
differential
operator
L ≡ p
2
(x)
d
2
dx
2
+ p
1
(x)
d
dx
+ p
0
(x)
is indeed a linear operator.
If a linear transformation T maps vectors of R
n
to vectors of R
m
,andS
maps vectors of R
m
to vectors of R
k
, then we can “compose” or “multiply”
the two transformations to obtain a linear transformation ST which maps
vectors of R
n
to vectors of R
k
. In terms of matrices, T is represented by an
m ×n matrix T, S is represented by a k ×m matrix S,andST is represented
by an k × n matrix which is the product of S and T with S to the left of T.
The product of matrices is as outlined in Box 6.1.3.
Box 7.1.1. If A is a k × m matrix, and B is an m × n matrix, then AB
is a k × n matrix whose entries are given by Box 6.1.3.
The product BA is not defined unless k = n,inwhichcaseBA will be an
m × m matrix.
Using polynomials, we can generate multidimensional vector spaces by
polynomials can
generate
multidimensional
vector spaces!
adding increasing powers of t. Then, the collection P
n
[t] of polynomials of
degree n and less becomes an (n + 1)-dimensional vector space. A convenient
basis for this vector space is {1,t,t
2
,...,t
n
} which we call the standard
1
The reader may want to check that the collection of differentiable functions is indeed a
vector space with the “zero function” being the zero vector.

218 Finite-Dimensional Vector Spaces
basis of P
n
[t]. The reader may verify that the operation of differentiation (of
any order) is a linear transformation on P
n
[t] which can be represented by
matrices as done in Example 6.2.2.
Example 7.1.1.
Let us find the matrix that represents the operation of second
differentiation on P
3
[t] using the standard basis of P
3
[t]. Recall that we only need to
apply the second derivative to the basis vectors f
1
=1,f
2
= t, f
3
= t
2
,andf
4
= t
3
.
We use a prime to denote the transformed vector:
f
1
=
d
2
dt
2
(1) = 0 = 0 ·f
1
+0· f
2
,
f
2
=
d
2
dt
2
(t)=0=0·f
1
+0· f
2
,
f
3
=
d
2
dt
2
(t
2
)=2=2·f
1
+0· f
2
,
f
4
=
d
2
dt
2
(t
3
)=6t =0· f
1
+6· f
2
,
where we have anticipated the fact that double differentiation of P
3
[t]resultsin
P
1
[t]. Following the rule of Box 6.2.2, we can write the transformation matrix as
0020
0006
.
We may verify that the coefficients in P
1
[t] of the second derivative of an arbi-
trary polynomial f (t)=α
0
+ α
1
t + α
2
t
2
+ α
3
t
3
can be obtained by the product of
the matrix of second derivative and the 4 × 1 column vector representing f(t). In
fact,
0020
0006
⎛
⎜
⎜
⎝
α
0
α
1
α
2
α
3
⎞
⎟
⎟
⎠
=
2α
2
6α
3
.
These are the two coefficients of the resulting polynomial in P
1
[t]. The polynomial
itself is 2α
2
+6α
3
t which is indeed the derivative of the third degree polynomial
f(t).
7.2 Inner Product
Since the concepts of length and angle are not familiar for R
n
, we need to
define the inner product first and then deduce those concepts. We can gener-
alize the usual inner product of R
2
and R
3
intermsofcomponentsofvectors.
Let
a =(a
1
,a
2
,...,a
n
)andb =(b
1
,b
2
,...,b
n
).
Then
inner product in
R
n
defined in
terms of
components in the
standard basis
a ·b = a
1
b
1
+ a
2
b
2
+ ···+ a
n
b
n
(7.5)
is the immediate generalization of the dot product to R
n
.

7.2 Inner Product 219
This, of course, is not the most general inner product. For that, we need
an inner product matrix G. As in the case of the plane and space, this is
simply a symmetric n ×n matrix whose elements determine the dot products
of the vectors of the basis in which we are working.
G =
⎛
⎜
⎜
⎜
⎝
g
11
g
12
... g
1n
g
21
g
22
... g
2n
.
.
.
.
.
.
.
.
.
g
n1
g
n2
... g
nn
⎞
⎟
⎟
⎟
⎠
,g
ij
= g
ji
,i,j=1, 2,...,n. (7.6)
Example 7.2.1.
Let us find the inner product matrix for the basis {1,t,t
2
,t
3
} of
P
3
[t]. As usual, we assume that the interval of integration for the inner product is
(0, 1). Because of the symmetry of the matrix and the fact that we have already
calculated the 3 × 3 submatrix of G, we need to find g
14
, g
24
, g
34
,andg
44
.Once
again, let f
1
= f
1
(t)=1,f
2
= f
2
(t)=t, f
3
= f
3
(t)=t
2
,andf
4
= f
4
(t)=t
3
;
then
g
14
= f
1
· f
4
=
#
1
0
f
1
(t)f
4
(t) dt =
#
1
0
t
3
dt =
1
4
,
g
24
= f
2
· f
4
=
#
1
0
f
2
(t)f
4
(t) dt =
#
1
0
t
4
dt =
1
5
.
Similarly, g
34
=
1
6
and g
44
=
1
7
. It follows that
G =
⎛
⎜
⎜
⎜
⎝
1
1
2
1
3
1
4
1
2
1
3
1
4
1
5
1
3
1
4
1
5
1
6
1
4
1
5
1
6
1
7
⎞
⎟
⎟
⎟
⎠
.
This matrix can be used to find the dot product of any two vectors in terms of their
components in the basis {1,t,t
2
,t
3
} of P
3
[t].
If a and b have components (a
1
,a
2
,...,a
n
)and(b
1
,b
2
,...,b
n
), then their
inner product is given by
inner product in
R
n
defined in
terms of the
metric matrix and
components in a
general basis
8
aGb =
a
1
a
2
... a
n
!
⎛
⎜
⎜
⎜
⎝
g
11
g
12
... g
1n
g
21
g
22
... g
2n
.
.
.
.
.
.
.
.
.
g
n1
g
n2
... g
nn
⎞
⎟
⎟
⎟
⎠
⎛
⎜
⎜
⎜
⎝
b
1
b
2
.
.
.
b
n
⎞
⎟
⎟
⎟
⎠
. (7.7)
As usual, if this expression is zero, we say that a and b are G-orthogonal. For
an orthonormal basis, the inner product matrix G becomes the unit matrix
2
and we recover the usual inner product of vectors in terms of components.
With a positive definite inner product at hand, we can define the length of
length of a vector
defined in terms of
inner product
a vector as the (positive) square root of the inner product of the vector with
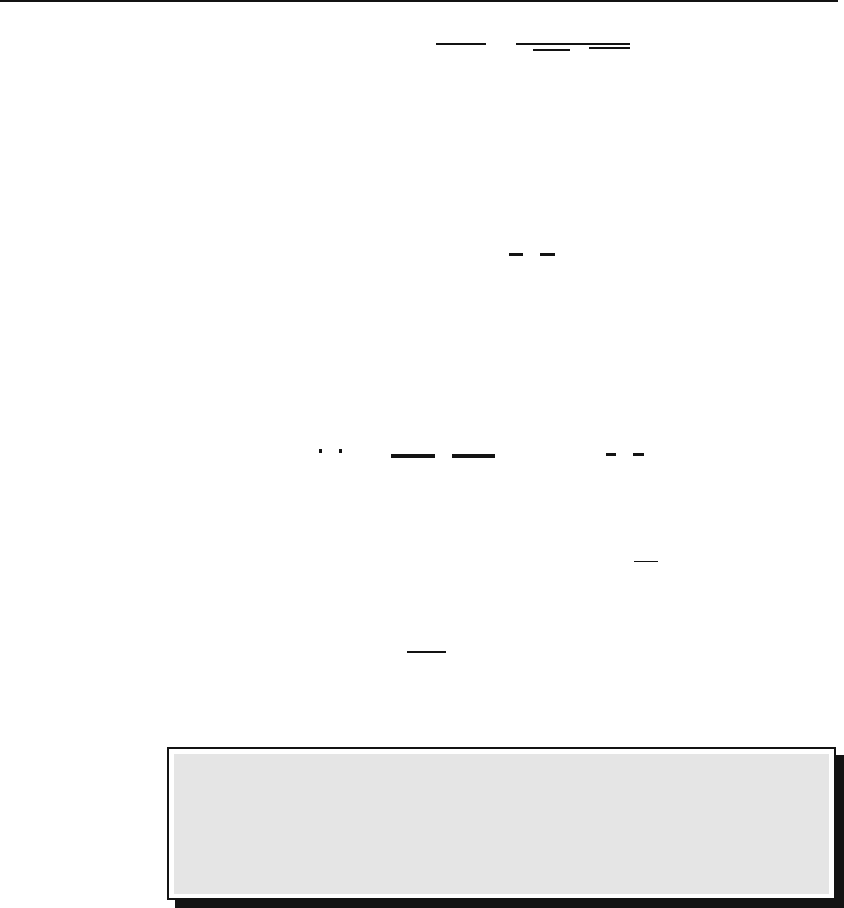
itself. Can we define the angle as well? We can always define
2
Only if the inner product is positive definite.
220 Finite-Dimensional Vector Spaces
angle defined in
terms of inner
product
cos θ ≡
a ·b
|a||b|
=
a ·b
√
a ·a
√
b · b
.
But how do we know that the ratio on the RHS is less than one? After all,
a true cosine must have this property! It is an amazing fact of nature that
any positive definite inner product has precisely this property. To show this,
let a and b be two vectors in any vector space on which an inner product is
defined. Denote the unit vector in the a direction by
ˆ
e
a
, and construct the
vector
b
= b − (b ·
ˆ
e
a
)
anumber
ˆ
e
a
(7.8)
which is easily seen to be perpendicular to
ˆ
e
a
(and therefore to a). If the
inner product is positive definite, then
derivation of the
Schwarz inequality
b
· b
≥ 0 ⇒ [b − (b ·
ˆ
e
a
)
ˆ
e
a
] · [b − (b ·
ˆ
e
a
)
ˆ
e
a
] ≥ 0
or
b ·b
=|b|
2
−2 b ·[(b ·
ˆ
e
a
)
ˆ
e
a
]
=(b·
ˆ
e
a
)
2
+(b ·
ˆ
e
a
)
2
ˆ
e
a
·
ˆ
e
a
=1
≥ 0.
It follows that
|b|
2
− (b ·
ˆ
e
a
)
2
≥ 0 ⇒|b|
2
≥
b ·
a
|a|
2
and
|b|
2
≥
b · a
|a|
2
⇒|b|
2
|a|
2
≥ (b ·a)
2
.
This is the desired inequality.
Box 7.2.1. (Schwarz Inequality).Ifa and b are two nonzero vectors
of a vector space for which a positive definite inner product is defined,
then
|a||b|≥|a ·b|.
The equality holds only if b is a multiple of a.
The last statement follows from the fact that b
· b
= 0 only if b
=0when
the inner product is positive definite [see Equation (7.8)].
The Schwarz inequality holds not only for finite-dimensional vector spaces
Schwarz inequality
holds in all inner
product spaces
regardless of their
dimensionality.
such as R
n
or P
n
[t], but also for infinite-dimensional vector spaces. It is
one of the most important inequalities in mathematical physics. One of its
consequences is that we can actually define the angle between two nonzero
vectors in R
n
or P
n
[t] (or any other vector space, finite or infinite, for which
a positive definite inner product exists).
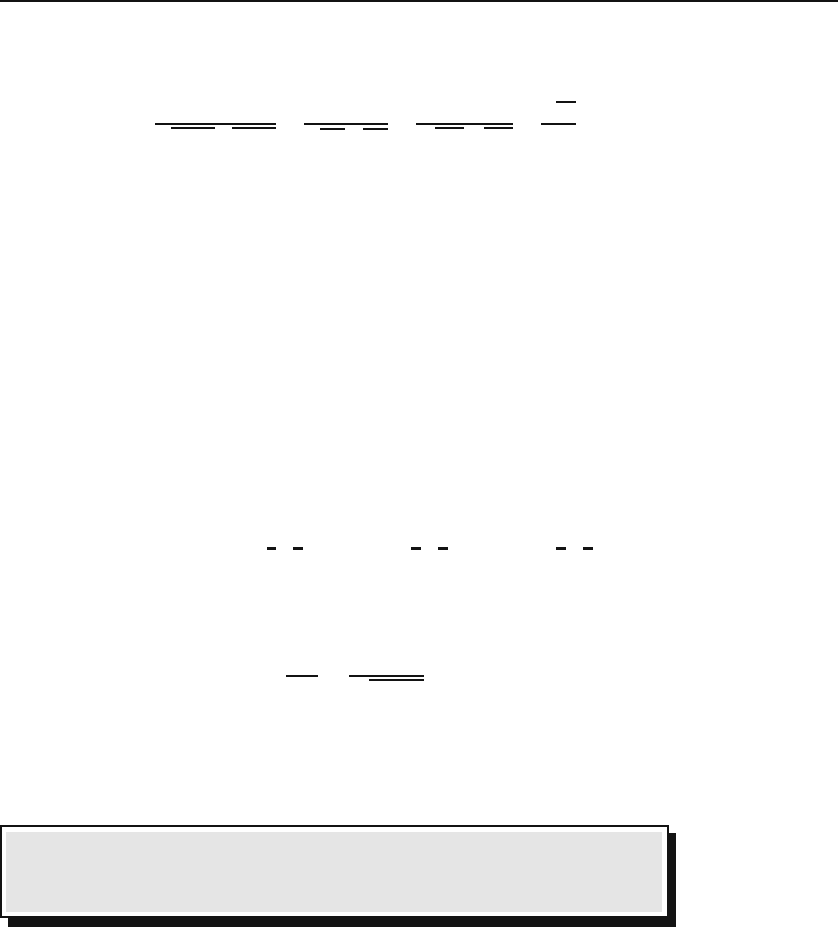
7.2 Inner Product 221
Example 7.2.2. What is the angle between the third and fourth vectors in the
standard basis of P
3
[t] when the interval of integration of the inner product is (0, 1)?
All the inner products are calculated in Example 7.2.1. Therefore,
cos θ =
f
3
· f
4
√
f
3
· f
3
√
f
4
· f
4
=
g
34
√
g
33
√
g
44
=
1/6
1/5
1/7
=
√
35
6
or θ =9.594
◦
.
As in the case of the plane and space, it is convenient to construct or-
thonormal basis vectors in R
n
. This is done by the Gram–Schmidt process Gram–Schmidt
process
which can easily be generalized. Suppose B = {a
1
, a
2
,...,a
n
} is a basis for
R
n
. Again, to avoid complications, we assume that the inner product is Eu-
clidean so that the inner product of every nonzero vector with itself is positive.
We know how to construct three orthonormal vectors out of {a
1
, a
2
, a
3
},we
did that in our discussion of the space vectors. Call these new orthonormal
vectors {
ˆ
e
1
,
ˆ
e
2
,
ˆ
e
3
}. Now construct the vector a
4
,
a
4
= a
4
− (a
4
·
ˆ
e
1
)
ˆ
e
1
− (a
4
·
ˆ
e
2
)
ˆ
e
2
− (a
4
·
ˆ
e
3
)
ˆ
e
3
which is obtained from a
4
by taking away its projections along
ˆ
e
1
,
ˆ
e
2
,and
ˆ
e
3
.
Now note that
ˆ
e
1
·a
4
=
ˆ
e
1
· a
4
− (a
4
·
ˆ
e
1
)
ˆ
e
1
·
ˆ
e
1
=1
−(a
4
·
ˆ
e
2
)
ˆ
e
2
·
ˆ
e
1
=0
−(a
4
·
ˆ
e
3
)
ˆ
e
3
·
ˆ
e
1
=0
=0.
Similarly,
ˆ
e
2
· a
4
=0and
ˆ
e
3
· a
4
= 0; i.e., a
4
is orthogonal to
ˆ
e
1
,
ˆ
e
2
,and
ˆ
e
3
.
This suggests defining
ˆ
e
4
as
ˆ
e
4
≡
a
4
|a
4
|
=
a
4
a
4
· a
4
.
This process can continue until we come up with n orthonormal vectors. This
will happen only if the n vectors with which we started are linearly indepen-
dent.
Box 7.2.2. If {a
1
, a
2
,...,a
n
} are linearly independent vectors of R
n
,
then we can construct a set of n orthonormal vectors out of them by the
Gram–Schmidt process.
An orthonormal basis will be denoted by {
ˆ
e
1
,
ˆ
e
2
,...,
ˆ
e
n
},where,asusual,
the symbol
ˆ
e stands for unit vectors. We can abbreviate the orthonormal
property of these vectors by writing
ˆ
e
i
·
ˆ
e
j
=
0
1ifi = j,
0ifi = j.

222 Finite-Dimensional Vector Spaces
There is a symbol that shortens the above statement even further. It is called
the Kronecker delta and denoted by δ
ij
. It is defined byKronecker delta
and its use in
discussing
orthonormal
vectors
δ
ij
=
0
1ifi = j,
0ifi = j.
(7.9)
Therefore, the orthonormality condition can be expressed as
ˆ
e
i
·
ˆ
e
j
= δ
ij
. (7.10)
We shall see many examples of the use of the Kronecker delta in the sequel.
Transformations that leave the inner products unchanged can be obtained
in exactly the same way as for the plane and the space. For A to preserve the
inner product, we need to have
G-orthogonal
matrix
8
AGA = G, (7.11)
i.e., it has to be G-orthogonal. If G is the identity matrix, then A can be
thought of as an n-dimensional rigid rotation and is simply called orthogonal;
it satisfies
8
AA = 1 (7.12)
or
⎛
⎜
⎜
⎜
⎝
a
11
a
12
... a
1n
a
21
a
22
... a
2n
.
.
.
.
.
.
.
.
.
a
n1
a
n2
... a
nn
⎞
⎟
⎟
⎟
⎠
⎛
⎜
⎜
⎜
⎝
a
11
a
21
... a
n1
a
12
a
22
... a
n2
.
.
.
.
.
.
.
.
.
a
1n
a
2n
... a
nn
⎞
⎟
⎟
⎟
⎠
=
⎛
⎜
⎜
⎜
⎝
10... 0
01... 0
.
.
.
.
.
.
.
.
.
00... 1
⎞
⎟
⎟
⎟
⎠
.
It should be clear from this that the columns of the matrix A,consideredas
vectors, have unit length and are orthogonal to other columns in the usual
Euclidean inner product.
7.3 The Determinant
The determinant of an n×n matrix is obtained in terms of cofactors in exactly
the same way as in the case of 3 × 3 matrices. The cofactors are themselves
determinants of (n − 1) × (n − 1) matrices which can be expanded in terms
of cofactors of their elements which are determinants of (n − 2) × (n − 2)
matrices, etc. Continuing this process, we finally end up with determinants
of 2 ×2 matrices. The determinant is also related to the inverse of a matrix
[see Equations (6.60) and (6.61)]:
Theorem 7.3.1. The matrix A has an inverse if and only if det A =0in
which case
A
−1
=
1
det A
8
A =
1
det A
⎛
⎜
⎜
⎜
⎝
A
11
A
21
... A
n1
A
12
A
22
... A
n2
.
.
.
.
.
.
.
.
.
A
1n
A
2n
... A
nn
⎞
⎟
⎟
⎟
⎠
, (7.13)
where A
ij
is the cofactor of a
ij
as defined in Box 6.3.2.

7.3 The Determinant 223
Calculation of the determinant becomes extremely cumbersome when the
dimension of the matrix increases beyond 4 or 5. However, there are certain
properties of the determinant which may sometimes facilitate its calculation.
The determinant has the following properties:
some properties of
the determinant
1. To obtain the determinant of an n ×n matrix, multiply each element of
one row (or one column) by its cofactor and then add the results.
2. The determinant of the unit matrix is 1.
3. The determinant of a matrix is equal to the determinant of its transpose:
det A =detA
t
.
4. If two rows (or two columns) of a matrix are proportional (in particular,
equal), the determinant of the matrix is zero.
5. If a row or column—treated as a vector in R
n
—of a matrix is multiplied
by a constant, the determinant of the matrix will be multiplied by the
same constant.
6. If two rows (or two columns) of a matrix are interchanged, the determi-
nant changes sign.
7. The determinant will not change if we add to one row (or one column)
a multiple of another row (or another column). The addition of rows or
columns and their multiplication by numbers are to be understood as
operations in R
n
.
An important relation, which we state without proof,
3
is the determinant of
a product is the
product of
determinants.
det(AB)=detA det B. (7.14)
This, in combination with det 1 =1andAA
−1
= 1,gives
det(AA
−1
)=det1 ⇒ det A det(A
−1
)=1 ⇒ det(A
−1
)=
1
det A
. (7.15)
In words, the determinant of the inverse of a matrix is the inverse of its
determinant of
inverse is inverse
determinant
determinant.
Recall that an orthogonal matrix A satisfies AA
t
= 1. The third property
of the determinant given above and (7.14) can be used to obtain
det(AA
t
)=det1 ⇒ (det A)
2
=1 ⇒ det A = ±1. (7.16)
So
Box 7.3.1. The determinant of an orthogonal matrix is either +1 or −1.
3
See Hassani, S. Mathematical Physics: A Modern Introduction to Its Foundations,
Springer-Verlag, 1999, Chapters 3 and 25.
