Hahn Th. (ed.) International tables for crystallography. Vol. A. Space-group symmetry
Подождите немного. Документ загружается.

F 4
1
32 No. 210 CONTINUED
Maximal non-isomorphic subgroups
I
[2] F 231(F 23, 196) (1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12)+
[3] F 4
1
12(I 4
1
22, 98) (1; 2; 3; 4; 13; 14; 15; 16)+
[3] F 4
1
12(I 4
1
22, 98) (1; 2; 3; 4; 17; 18; 19; 20)+
[3] F 4
1
12(I 4
1
22, 98) (1; 2; 3; 4; 21; 22; 23; 24)+
⎧
⎪
⎨
⎪
⎩
[4] F 132(R32, 155) (1; 5; 9; 14; 19; 24)+
[4] F 132(R32, 155) (1; 6; 12; 13; 18; 24)+
[4] F 132(R32, 155) (1; 7; 10; 13; 19; 22)+
[4] F 132(R32, 155) (1; 8; 11; 14; 18; 22)+
IIa
⎧
⎪
⎪
⎪
⎪
⎪
⎪
⎨
⎪
⎪
⎪
⎪
⎪
⎪
⎩
[4] P4
1
32 (213) 1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12; 13; 14; 15; 16; 17; 18; 19; 20; 21; 22; 23; 24
[4] P4
1
32 (213) 1; 2; 3; 4; 13; 14; 15; 16; (9; 10; 11; 12; 17; 18; 19; 20)+(0,
1
2
,
1
2
); (5; 6; 7; 8; 21; 22; 23;
24)+(
1
2
,0,
1
2
)
[4] P4
1
32 (213) 1; 2; 3; 4; 17; 18; 19; 20; (9; 10; 11; 12; 21; 22; 23; 24)+(
1
2
,0,
1
2
); (5; 6; 7; 8; 13; 14; 15;
16)+(
1
2
,
1
2
,0)
[4] P4
1
32 (213) 1; 2; 3; 4; 21; 22; 23; 24; (5; 6; 7; 8; 17; 18; 19; 20)+(0,
1
2
,
1
2
); (9; 10; 11; 12; 13; 14; 15;
16)+(
1
2
,
1
2
,0)
⎧
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎨
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎪
⎩
[4] P4
3
32 (212) 1; 5; 9; 14; 19; 24; (4; 6; 11; 16; 18; 23)+(0,
1
2
,
1
2
); (3; 8; 10; 15; 20; 22)+(
1
2
,0,
1
2
); (2; 7; 12;
13; 17; 21)+(
1
2
,
1
2
,0)
[4] P4
3
32 (212) 1; 6; 12; 13; 18; 24; (4; 5; 10; 15; 19; 23)+(0,
1
2
,
1
2
); (3; 7; 11; 16; 17; 22)+(
1
2
,0,
1
2
); (2; 8; 9;
14; 20; 21)+(
1
2
,
1
2
,0)
[4] P4
3
32 (212) 1; 7; 10; 13; 19; 22; (4; 8; 12; 15; 18; 21)+(0,
1
2
,
1
2
); (3; 6; 9; 16; 20; 24)+(
1
2
,0,
1
2
); (2; 5; 11;
14; 17; 23)+(
1
2
,
1
2
,0)
[4] P4
3
32 (212) 1; 8; 11; 14; 18; 22; (4; 7; 9; 16; 19; 21)+(0,
1
2
,
1
2
); (3; 5; 12; 15; 17; 24)+(
1
2
,0,
1
2
); (2; 6; 10;
13; 20; 23)+(
1
2
,
1
2
,0)
IIb none
Maximal isomorphic subgroups of lowest index
IIc
[27] F 4
1
32(a
= 3a,b
= 3b,c
= 3c) (210)
Minimal non-isomorphic supergroups
I
[2] Fd
¯
3m (227); [2] Fd
¯
3c (228)
II [2] P4
2
32(a
=
1
2
a,b
=
1
2
b,c
=
1
2
c) (208)
644
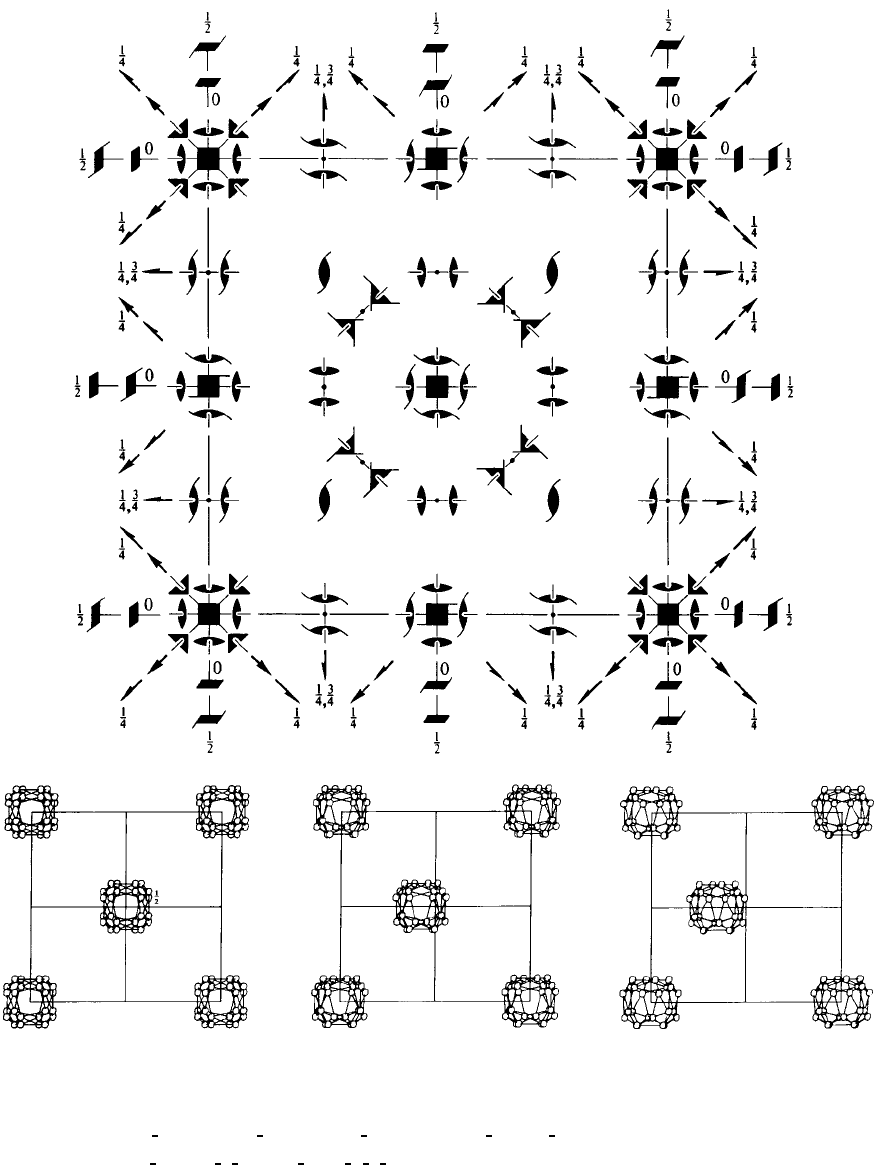
I 432 O
5
432 Cubic
No. 211 I 432
Patterson symmetry Im
¯
3m
Origin at 432
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
1
2
;0≤ z ≤
1
4
; z ≤ min(x,
1
2
− x,y,
1
2
− y)
Vertices 0,0, 0
1
2
,0, 0
1
2
,
1
2
,00,
1
2
,0
1
4
,
1
4
,
1
4
646
International Tables for Crystallography (2006). Vol. A, Space group 211, pp. 645–647.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 211 I 432
Symmetry operations
For (0,0,0)+ set
(1) 1 (2) 2 0,0,z (3) 2 0,y,0(4)2x,0,0
(5) 3
+
x,x,x (6) 3
+
¯x,x, ¯x (7) 3
+
x, ¯x, ¯x (8) 3
+
¯x, ¯x,x
(9) 3
−
x,x,x (10) 3
−
x, ¯x, ¯x (11) 3
−
¯x, ¯x,x (12) 3
−
¯x,x, ¯x
(13) 2 x,x,0 (14) 2 x, ¯x,0 (15) 4
−
0,0,z (16) 4
+
0,0,z
(17) 4
−
x,0,0 (18) 2 0,y,y (19) 2 0,y, ¯y (20) 4
+
x,0,0
(21) 4
+
0,y,0 (22) 2 x,0,x (23) 4
−
0,y,0 (24) 2 ¯x,0,x
For (
1
2
,
1
2
,
1
2
)+ set
(1) t(
1
2
,
1
2
,
1
2
) (2) 2(0,0,
1
2
)
1
4
,
1
4
,z (3) 2(0,
1
2
,0)
1
4
,y,
1
4
(4) 2(
1
2
,0,0) x,
1
4
,
1
4
(5) 3
+
(
1
2
,
1
2
,
1
2
) x,x,x (6) 3
+
(
1
6
,−
1
6
,
1
6
) ¯x+
1
3
,x+
1
3
, ¯x (7) 3
+
(−
1
6
,
1
6
,
1
6
) x+
2
3
, ¯x−
1
3
, ¯x (8) 3
+
(
1
6
,
1
6
,−
1
6
) ¯x+
1
3
, ¯x+
2
3
,x
(9) 3
−
(
1
2
,
1
2
,
1
2
) x,x,x (10) 3
−
(−
1
6
,
1
6
,
1
6
) x+
1
3
, ¯x+
1
3
, ¯x (11) 3
−
(
1
6
,
1
6
,−
1
6
) ¯x+
2
3
, ¯x+
1
3
,x (12) 3
−
(
1
6
,−
1
6
,
1
6
) ¯x−
1
3
,x+
2
3
, ¯x
(13) 2(
1
2
,
1
2
,0) x,x,
1
4
(14) 2 x, ¯x+
1
2
,
1
4
(15) 4
−
(0,0,
1
2
)
1
2
,0,z (16) 4
+
(0,0,
1
2
) 0,
1
2
,z
(17) 4
−
(
1
2
,0,0) x,
1
2
,0 (18) 2(0,
1
2
,
1
2
)
1
4
,y,y (19) 2
1
4
,y+
1
2
, ¯y (20) 4
+
(
1
2
,0,0) x,0,
1
2
(21) 4
+
(0,
1
2
,0)
1
2
,y,0 (22) 2(
1
2
,0,
1
2
) x,
1
4
,x (23) 4
−
(0,
1
2
,0) 0,y,
1
2
(24) 2 ¯x+
1
2
,
1
4
,x
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); t(
1
2
,
1
2
,
1
2
); (2); (3); (5); (13)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates
(0,0, 0)+ (
1
2
,
1
2
,
1
2
)+
Reflection conditions
h,k,l permutable
General:
48 j 1(1)x, y,z (2) ¯x, ¯y,z (3) ¯x,y, ¯z (4) x, ¯y, ¯z
(5) z,x,y (6) z, ¯x, ¯y (7) ¯z, ¯x,y (8) ¯z,x, ¯y
(9) y,z,x (10) ¯y,z, ¯x (11) y, ¯z, ¯x (12) ¯y, ¯z,x
(13) y,x,
¯z (14) ¯y, ¯x, ¯z (15) y, ¯x, z (16) ¯y,x,z
(17) x,z, ¯y (18) ¯x,z,y (19) ¯x, ¯z, ¯y (20) x, ¯z,y
(21) z,y, ¯x (22) z, ¯y,x (23) ¯z,y,x (24) ¯z, ¯y, ¯x
hkl : h + k + l = 2n
0kl : k + l = 2 n
hhl : l
= 2n
h00 : h = 2n
Special: as above, plus
24 i ..2
1
4
,y, ¯y +
1
2
3
4
, ¯y, ¯y +
1
2
3
4
,y,y +
1
2
1
4
, ¯y, y +
1
2
¯y+
1
2
,
1
4
,y ¯y +
1
2
,
3
4
, ¯yy+
1
2
,
3
4
,yy+
1
2
,
1
4
, ¯y
y, ¯y +
1
2
,
1
4
¯y, ¯y +
1
2
,
3
4
y, y +
1
2
,
3
4
¯y,y +
1
2
,
1
4
no extra conditions
24 h ..20,y,y 0, ¯y,y 0,y, ¯y 0, ¯y, ¯yy,0 , yy, 0, ¯y
¯y,0, y ¯y,0, ¯yy,y, 0¯y,y, 0 y, ¯y,0¯y, ¯y,0
no extra conditions
24 g 2 .. x,
1
2
,0¯x,
1
2
,00, x,
1
2
0, ¯x,
1
2
1
2
,0, x
1
2
,0, ¯x
1
2
,x,0
1
2
, ¯x, 0 x,0,
1
2
¯x,0,
1
2
0,
1
2
, ¯x 0,
1
2
,x
no extra conditions
16 f . 3 . x,x, x ¯x, ¯x,x ¯x,x, ¯xx, ¯x, ¯x
x,x, ¯x ¯x, ¯x, ¯xx, ¯x,x ¯x,x,x
no extra conditions
12 e 4 .. x, 0,0¯x,0, 00, x,00, ¯x,00, 0,x 0,0, ¯x no extra conditions
12 d 2 . 22
1
4
,
1
2
,0
3
4
,
1
2
,00,
1
4
,
1
2
0,
3
4
,
1
2
1
2
,0,
1
4
1
2
,0,
3
4
no extra conditions
8 c . 32
1
4
,
1
4
,
1
4
3
4
,
3
4
,
1
4
3
4
,
1
4
,
3
4
1
4
,
3
4
,
3
4
hkl : k,l = 2n
6 b 42.20,
1
2
,
1
2
1
2
,0,
1
2
1
2
,
1
2
,0 no extra conditions
2 a 432 0,0,0 no extra conditions
Symmetry of special projections
Along [001] p4mm
a
=
1
2
(a − b) b
=
1
2
(a + b)
Origin at 0,0,z
Along [111] p3m1
a
=
1
3
(2a − b− c) b
=
1
3
(−a + 2b − c)
Origin at x, x,x
Along [110] p2mm
a
=
1
2
(−a + b) b
=
1
2
c
Origin at x,x, 0
(Continued on page 645)
647

CONTINUED
(from page 647)
No. 211 I 432
Maximal non-isomorphic subgroups
I
[2] I 231(I 23, 197) (1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12)+
[3] I 412(I 422, 97) (1; 2; 3; 4; 13; 14; 15; 16)+
[3] I 412(I 422, 97) (1; 2; 3; 4; 17; 18; 19; 20)+
[3] I 412(I 422, 97) (1; 2; 3; 4; 21; 22; 23; 24)+
⎧
⎪
⎨
⎪
⎩
[4] I 132(R32, 155) (1; 5; 9; 14; 19; 24)+
[4] I 132(R32, 155) (1; 6; 12; 13; 18; 24)+
[4] I 132(R32, 155) (1; 7; 10; 13; 19; 22)+
[4] I 132(R32, 155) (1; 8; 11; 14; 18; 22)+
IIa [2] P4
2
32 (208) 1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12; (13; 14; 15; 16; 17; 18; 19; 20; 21; 22; 23; 24)+(
1
2
,
1
2
,
1
2
)
[2] P432 (207) 1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12; 13; 14; 15; 16; 17; 18; 19; 20; 21; 22; 23; 24
IIb none
Maximal isomorphic subgroups of lowest index
IIc
[27] I 432(a
= 3a,b
= 3b,c
= 3c) (211)
Minimal non-isomorphic supergroups
I
[2] Im
¯
3m (229)
II [4] P432(a
=
1
2
a,b
=
1
2
b,c
=
1
2
c) (207)
645
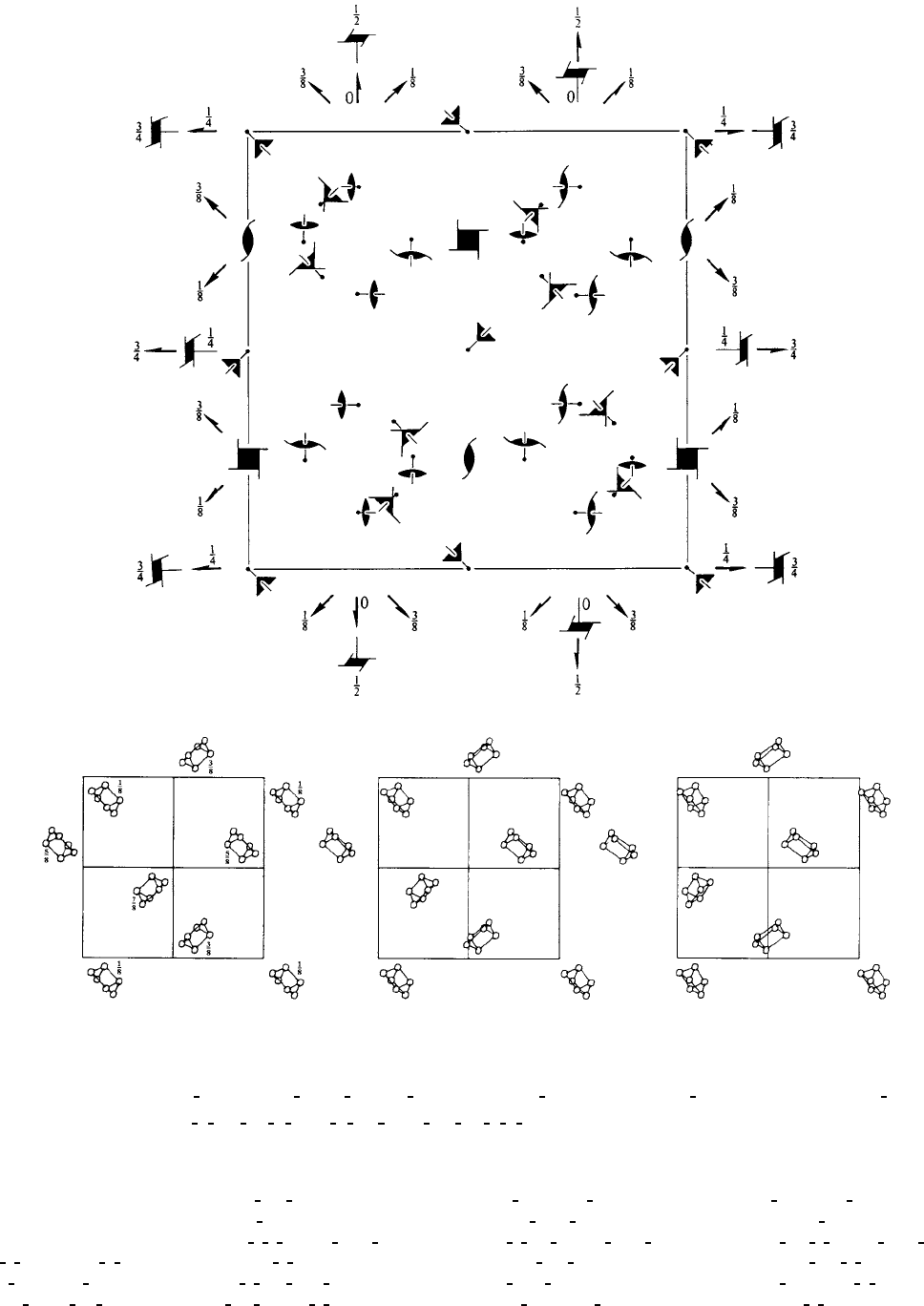
P4
3
32 O
6
432 Cubic
No. 212 P4
3
32 Patterson symmetry Pm
¯
3m
Origin on 3[111] at midpoint of three non-intersecting pairs of parallel screw axes 4
3
and 2
1
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
3
4
; −
1
2
≤ z ≤
1
4
;max(−y,x −
1
2
) ≤ z ≤ min(−y +
1
2
,2x − y,2y − x,y − 2x +
1
2
)
Vertices 0,0, 0
3
8
,
1
8
,−
1
8
1
2
,
1
2
,0
1
4
,
3
4
,−
1
4
0,
1
2
,−
1
2
1
4
,
1
4
,
1
4
Symmetry operations
(1) 1 (2) 2(0,0,
1
2
)
1
4
,0,z (3) 2(0,
1
2
,0) 0,y,
1
4
(4) 2(
1
2
,0,0) x,
1
4
,0
(5) 3
+
x,x,x (6) 3
+
¯x+
1
2
,x, ¯x (7) 3
+
x+
1
2
, ¯x−
1
2
, ¯x (8) 3
+
¯x, ¯x+
1
2
,x
(9) 3
−
x,x,x (10) 3
−
(−
1
3
,
1
3
,
1
3
) x+
1
6
, ¯x+
1
6
, ¯x (11) 3
−
(
1
3
,
1
3
,−
1
3
) ¯x+
1
3
, ¯x+
1
6
,x (12) 3
−
(
1
3
,−
1
3
,
1
3
) ¯x−
1
6
,x+
1
3
, ¯x
(13) 2(
1
2
,
1
2
,0) x,x+
1
4
,
3
8
(14) 2 x, ¯x+
1
4
,
1
8
(15) 4
−
(0,0,
1
4
)
3
4
,0,z (16) 4
+
(0,0,
3
4
)
1
4
,
1
2
,z
(17) 4
−
(
1
4
,0,0) x,
3
4
,0 (18) 2(0,
1
2
,
1
2
)
3
8
,y−
1
4
,y (19) 2
1
8
,y+
1
4
, ¯y (20) 4
+
(
3
4
,0,0) x,
1
4
,
1
2
(21) 4
+
(0,
3
4
,0)
1
2
,y,
1
4
(22) 2(
1
2
,0,
1
2
) x+
1
4
,
3
8
,x (23) 4
−
(0,
1
4
,0) 0,y,
3
4
(24) 2 ¯x+
1
4
,
1
8
,x
648
International Tables for Crystallography (2006). Vol. A, Space group 212, pp. 648–649.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 212 P4
3
32
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5); (13)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
h,k,l permutable
General:
24 e 1(1)x,y, z (2) ¯x +
1
2
, ¯y, z +
1
2
(3) ¯x,y +
1
2
, ¯z +
1
2
(4) x +
1
2
, ¯y +
1
2
, ¯z
(5) z,x,y (6) z +
1
2
, ¯x +
1
2
, ¯y (7) ¯z +
1
2
, ¯x, y +
1
2
(8) ¯z,x +
1
2
, ¯y +
1
2
(9) y,z,x (10) ¯y,z +
1
2
, ¯x +
1
2
(11) y +
1
2
, ¯z +
1
2
, ¯x (12) ¯y+
1
2
, ¯z, x +
1
2
(13) y +
1
4
,x +
3
4
, ¯z +
3
4
(14) ¯y +
1
4
, ¯x +
1
4
, ¯z +
1
4
(15) y +
3
4
, ¯x +
3
4
,z +
1
4
(16) ¯y +
3
4
,x +
1
4
,z +
3
4
(17) x +
1
4
,z +
3
4
, ¯y +
3
4
(18) ¯x +
3
4
,z +
1
4
,y +
3
4
(19) ¯x +
1
4
, ¯z +
1
4
, ¯y +
1
4
(20) x +
3
4
, ¯z +
3
4
,y +
1
4
(21) z +
1
4
,y +
3
4
, ¯x +
3
4
(22) z +
3
4
, ¯y +
3
4
,x +
1
4
(23) ¯z +
3
4
,y +
1
4
,x +
3
4
(24) ¯z+
1
4
, ¯y +
1
4
, ¯x +
1
4
h00 : h = 4n
Special: as above, plus
12 d ..2
1
8
,y, ¯y +
1
4
3
8
, ¯y, ¯y +
3
4
7
8
,y +
1
2
,y +
1
4
5
8
, ¯y +
1
2
,y +
3
4
¯y+
1
4
,
1
8
,y ¯y +
3
4
,
3
8
, ¯yy+
1
4
,
7
8
,y +
1
2
y +
3
4
,
5
8
, ¯y +
1
2
y, ¯y +
1
4
,
1
8
¯y, ¯y +
3
4
,
3
8
y +
1
2
,y +
1
4
,
7
8
¯y +
1
2
,y +
3
4
,
5
8
no extra conditions
8 c . 3 . x,x,x ¯x +
1
2
, ¯x, x +
1
2
¯x,x +
1
2
, ¯x +
1
2
x +
1
2
, ¯x +
1
2
, ¯x
x +
1
4
,x +
3
4
, ¯x +
3
4
¯x +
1
4
, ¯x +
1
4
, ¯x +
1
4
x +
3
4
, ¯x +
3
4
,x +
1
4
¯x +
3
4
,x +
1
4
,x +
3
4
0kl : k = 2n + 1
or l = 2n + 1
or k + l = 4n
4 b . 32
5
8
,
5
8
,
5
8
7
8
,
3
8
,
1
8
3
8
,
1
8
,
7
8
1
8
,
7
8
,
3
8
4 a . 32
1
8
,
1
8
,
1
8
3
8
,
7
8
,
5
8
7
8
,
5
8
,
3
8
5
8
,
3
8
,
7
8
hkl : h,k = 2n + 1
or h = 2n + 1, k = 4n and
l = 4n + 2
or h, k, l = 4n + 2
or h, k, l = 4n
Symmetry of special projections
Along [001] p4gm
a
= ab
= b
Origin at
1
4
,
1
2
,z
Along [111] p3m1
a
=
1
3
(2a − b− c) b
=
1
3
(−a + 2b − c)
Origin at x, x,x
Along [110] p2gm
a
=
1
2
(−a + b) b
= c
Origin at x, x +
1
4
,
3
8
Maximal non-isomorphic subgroups
I
[2] P2
1
31(P2
1
3, 198) 1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12
[3] P4
3
12(P4
3
2
1
2, 96) 1; 2; 3; 4; 13; 14; 15; 16
[3] P4
3
12(P4
3
2
1
2, 96) 1; 2; 3; 4; 17; 18; 19; 20
[3] P4
3
12(P4
3
2
1
2, 96) 1; 2; 3; 4; 21; 22; 23; 24
⎧
⎪
⎨
⎪
⎩
[4] P132 (R32, 155) 1; 5; 9; 14; 19; 24
[4] P132 (R32, 155) 1; 6; 12; 13; 18; 24
[4] P132 (R32, 155) 1; 7; 10; 13; 19; 22
[4] P132 (R32, 155) 1; 8; 11; 14; 18; 22
IIa none
IIb none
Maximal isomorphic subgroups of lowest index
IIc
[27] P4
1
32(a
= 3a,b
= 3b,c
= 3c) (213); [125] P4
3
32(a
= 5a,b
= 5b,c
= 5c) (212)
Minimal non-isomorphic supergroups
I
none
II [2] I 4
1
32 (214); [4] F 4
1
32 (210)
649
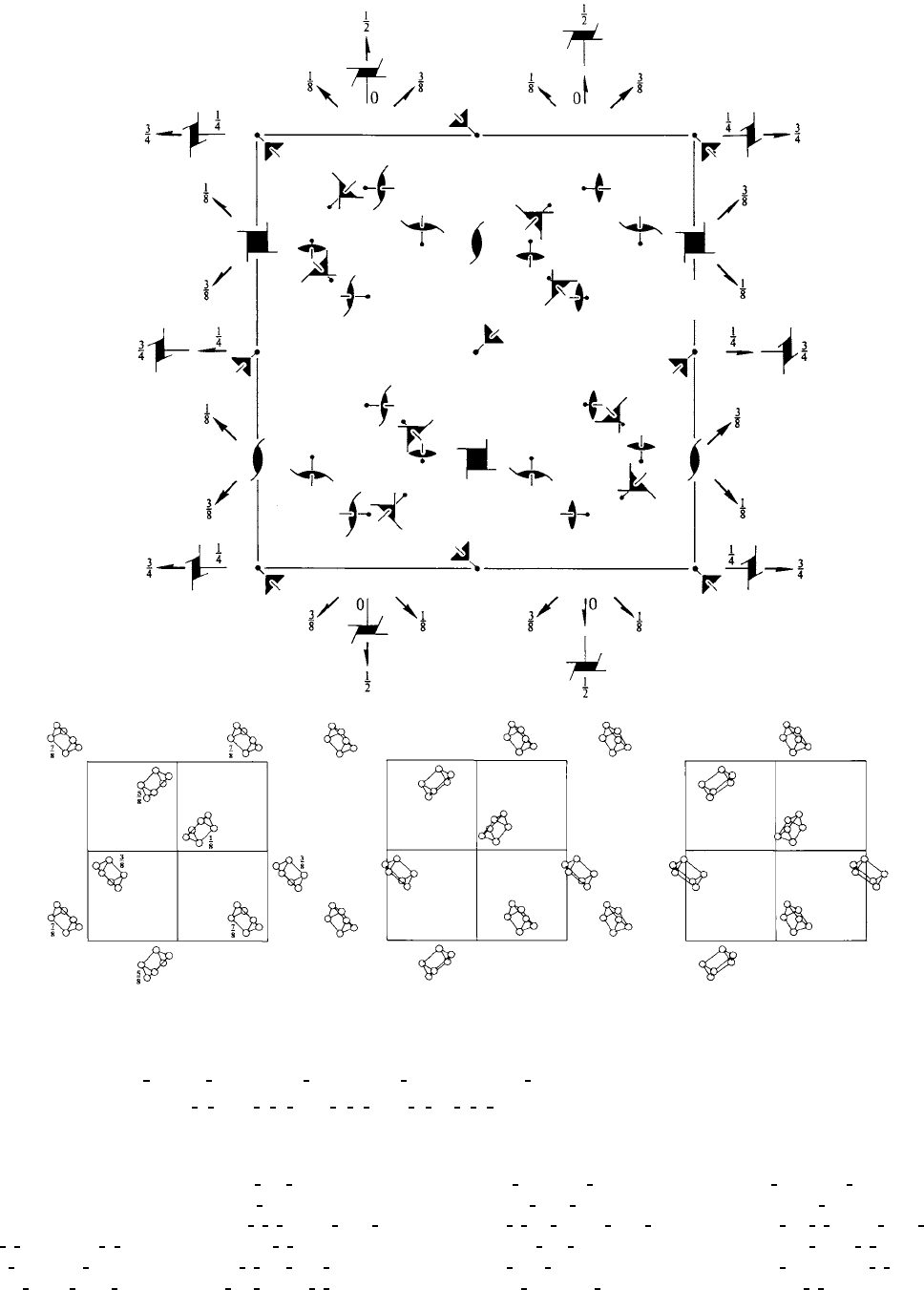
P4
1
32 O
7
432 Cubic
No. 213 P4
1
32 Patterson symmetry Pm
¯
3m
Origin on 3[111] at midpoint of three non-intersecting pairs of parallel screw axes 4
1
and 2
1
Asymmetric unit −
1
4
≤ x ≤
1
2
;0≤ y ≤
3
4
;0≤ z ≤
1
2
; x ≤ y ≤ x+
1
2
; (y− x)/2 ≤ z ≤ min(y, (−4x− 2y+3)/2,(3− 2x− 2y)/4)
Vertices 0,0, 0
1
2
,
1
2
,0
1
4
,
3
4
,
1
4
−
1
4
,
1
4
,
1
4
0,
1
2
,
1
2
3
8
,
3
8
,
3
8
Symmetry operations
(1) 1 (2) 2(0,0,
1
2
)
1
4
,0,z (3) 2(0,
1
2
,0) 0,y,
1
4
(4) 2(
1
2
,0,0) x,
1
4
,0
(5) 3
+
x,x,x (6) 3
+
¯x+
1
2
,x, ¯x (7) 3
+
x+
1
2
, ¯x−
1
2
, ¯x (8) 3
+
¯x, ¯x+
1
2
,x
(9) 3
−
x,x,x (10) 3
−
(−
1
3
,
1
3
,
1
3
) x+
1
6
, ¯x+
1
6
, ¯x (11) 3
−
(
1
3
,
1
3
,−
1
3
) ¯x+
1
3
, ¯x+
1
6
,x (12) 3
−
(
1
3
,−
1
3
,
1
3
) ¯x−
1
6
,x+
1
3
, ¯x
(13) 2(
1
2
,
1
2
,0) x,x−
1
4
,
1
8
(14) 2 x, ¯x+
3
4
,
3
8
(15) 4
−
(0,0,
3
4
)
1
4
,0,z (16) 4
+
(0,0,
1
4
) −
1
4
,
1
2
,z
(17) 4
−
(
3
4
,0,0) x,
1
4
,0 (18) 2(0,
1
2
,
1
2
)
1
8
,y+
1
4
,y (19) 2
3
8
,y+
3
4
, ¯y (20) 4
+
(
1
4
,0,0) x,−
1
4
,
1
2
(21) 4
+
(0,
1
4
,0)
1
2
,y,−
1
4
(22) 2(
1
2
,0,
1
2
) x−
1
4
,
1
8
,x (23) 4
−
(0,
3
4
,0) 0,y,
1
4
(24) 2 ¯x+
3
4
,
3
8
,x
650
International Tables for Crystallography (2006). Vol. A, Space group 213, pp. 650–651.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 213 P4
1
32
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5); (13)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
h,k,l permutable
General:
24 e 1(1)x,y, z (2) ¯x +
1
2
, ¯y, z +
1
2
(3) ¯x,y +
1
2
, ¯z +
1
2
(4) x +
1
2
, ¯y +
1
2
, ¯z
(5) z,x,y (6) z +
1
2
, ¯x +
1
2
, ¯y (7) ¯z +
1
2
, ¯x, y +
1
2
(8) ¯z,x +
1
2
, ¯y +
1
2
(9) y,z,x (10) ¯y,z +
1
2
, ¯x +
1
2
(11) y +
1
2
, ¯z +
1
2
, ¯x (12) ¯y+
1
2
, ¯z, x +
1
2
(13) y +
3
4
,x +
1
4
, ¯z +
1
4
(14) ¯y +
3
4
, ¯x +
3
4
, ¯z +
3
4
(15) y +
1
4
, ¯x +
1
4
,z +
3
4
(16) ¯y +
1
4
,x +
3
4
,z +
1
4
(17) x +
3
4
,z +
1
4
, ¯y +
1
4
(18) ¯x +
1
4
,z +
3
4
,y +
1
4
(19) ¯x +
3
4
, ¯z +
3
4
, ¯y +
3
4
(20) x +
1
4
, ¯z +
1
4
,y +
3
4
(21) z +
3
4
,y +
1
4
, ¯x +
1
4
(22) z +
1
4
, ¯y +
1
4
,x +
3
4
(23) ¯z +
1
4
,y +
3
4
,x +
1
4
(24) ¯z+
3
4
, ¯y +
3
4
, ¯x +
3
4
h00 : h = 4n
Special: as above, plus
12 d ..2
1
8
,y,y +
1
4
3
8
, ¯y, y +
3
4
7
8
,y +
1
2
, ¯y +
1
4
5
8
, ¯y +
1
2
, ¯y +
3
4
y +
1
4
,
1
8
,yy+
3
4
,
3
8
, ¯y ¯y +
1
4
,
7
8
,y +
1
2
¯y +
3
4
,
5
8
, ¯y +
1
2
y, y +
1
4
,
1
8
¯y,y +
3
4
,
3
8
y +
1
2
, ¯y +
1
4
,
7
8
¯y +
1
2
, ¯y +
3
4
,
5
8
no extra conditions
8 c . 3 . x,x,x ¯x +
1
2
, ¯x, x +
1
2
¯x,x +
1
2
, ¯x +
1
2
x +
1
2
, ¯x +
1
2
, ¯x
x +
3
4
,x +
1
4
, ¯x +
1
4
¯x +
3
4
, ¯x +
3
4
, ¯x +
3
4
x +
1
4
, ¯x +
1
4
,x +
3
4
¯x +
1
4
,x +
3
4
,x +
1
4
0kl : k = 2n + 1
or l = 2n + 1
or k + l = 4n
4 b . 32
7
8
,
7
8
,
7
8
5
8
,
1
8
,
3
8
1
8
,
3
8
,
5
8
3
8
,
5
8
,
1
8
4 a . 32
3
8
,
3
8
,
3
8
1
8
,
5
8
,
7
8
5
8
,
7
8
,
1
8
7
8
,
1
8
,
5
8
hkl : h,k = 2n + 1
or h = 2n + 1, k = 4n and
l = 4n + 2
or h, k, l = 4n + 2
or h, k, l = 4n
Symmetry of special projections
Along [001] p4gm
a
= ab
= b
Origin at
1
4
,0, z
Along [111] p3m1
a
=
1
3
(2a − b− c) b
=
1
3
(−a + 2b − c)
Origin at x, x,x
Along [110] p2gm
a
=
1
2
(−a + b) b
= c
Origin at x, x +
1
4
,
1
8
Maximal non-isomorphic subgroups
I
[2] P2
1
31(P2
1
3, 198) 1; 2; 3; 4; 5; 6; 7; 8; 9; 10; 11; 12
[3] P4
1
12(P4
1
2
1
2, 92) 1; 2; 3; 4; 13; 14; 15; 16
[3] P4
1
12(P4
1
2
1
2, 92) 1; 2; 3; 4; 17; 18; 19; 20
[3] P4
1
12(P4
1
2
1
2, 92) 1; 2; 3; 4; 21; 22; 23; 24
⎧
⎪
⎨
⎪
⎩
[4] P132 (R32, 155) 1; 5; 9; 14; 19; 24
[4] P132 (R32, 155) 1; 6; 12; 13; 18; 24
[4] P132 (R32, 155) 1; 7; 10; 13; 19; 22
[4] P132 (R32, 155) 1; 8; 11; 14; 18; 22
IIa none
IIb none
Maximal isomorphic subgroups of lowest index
IIc
[27] P4
3
32(a
= 3a,b
= 3b,c
= 3c) (212); [125] P4
1
32(a
= 5a,b
= 5b,c
= 5c) (213)
Minimal non-isomorphic supergroups
I
none
II [2] I 4
1
32 (214); [4] F 4
1
32 (210)
651
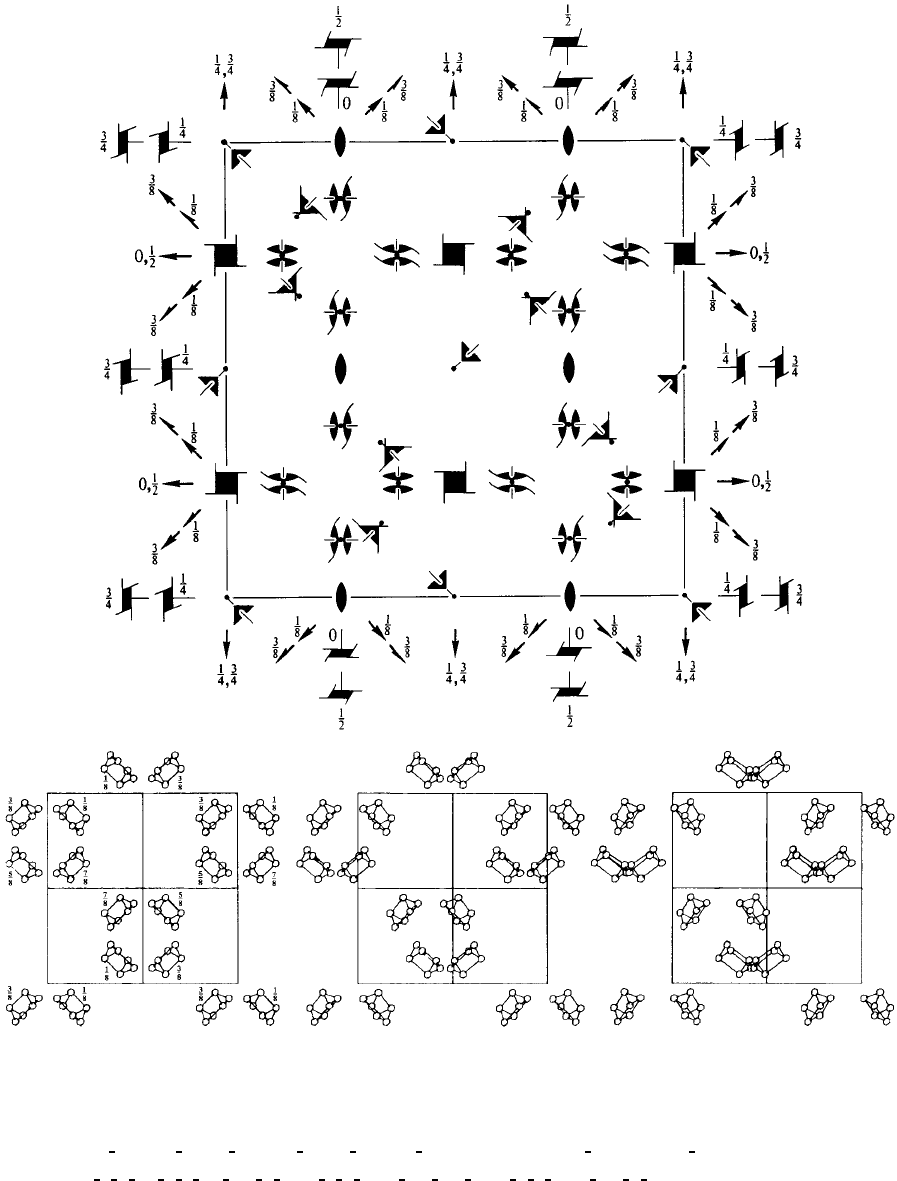
I 4
1
32 O
8
432 Cubic
No. 214 I 4
1
32 Patterson symmetry Im
¯
3m
Origin on 3[111] at midpoint of three non-intersecting pairs of parallel screw axes 4
1
and 4
3
and of three
non-intersecting pairs of parallel 2 axes
Asymmetric unit −
3
8
≤ x ≤
1
8
; −
1
8
≤ y ≤
1
8
; −
1
8
≤ z ≤
3
8
;max(x,y, y − x −
1
8
) ≤ z ≤ y +
1
4
Vertices
1
8
,
1
8
,
1
8
1
8
,
1
8
,
3
8
1
8
,−
1
8
,
1
8
−
1
8
,
1
8
,
1
8
−
1
8
,−
1
8
,−
1
8
−
3
8
,
1
8
,
3
8
−
3
8
,−
1
8
,
1
8
652
International Tables for Crystallography (2006). Vol. A, Space group 214, pp. 652–654.
Copyright © 2006 International Union of Crystallography
CONTINUED No. 214 I 4
1
32
Symmetry operations
For (0,0,0)+ set
(1) 1 (2) 2(0,0,
1
2
)
1
4
,0,z (3) 2(0,
1
2
,0) 0,y,
1
4
(4) 2(
1
2
,0,0) x,
1
4
,0
(5) 3
+
x,x,x (6) 3
+
¯x+
1
2
,x, ¯x (7) 3
+
x+
1
2
, ¯x−
1
2
, ¯x (8) 3
+
¯x, ¯x+
1
2
,x
(9) 3
−
x,x,x (10) 3
−
(−
1
3
,
1
3
,
1
3
) x+
1
6
, ¯x+
1
6
, ¯x (11) 3
−
(
1
3
,
1
3
,−
1
3
) ¯x+
1
3
, ¯x+
1
6
,x (12) 3
−
(
1
3
,−
1
3
,
1
3
) ¯x−
1
6
,x+
1
3
, ¯x
(13) 2(
1
2
,
1
2
,0) x,x−
1
4
,
1
8
(14) 2 x, ¯x+
3
4
,
3
8
(15) 4
−
(0,0,
3
4
)
1
4
,0,z (16) 4
+
(0,0,
1
4
) −
1
4
,
1
2
,z
(17) 4
−
(
3
4
,0,0) x,
1
4
,0 (18) 2(0,
1
2
,
1
2
)
1
8
,y+
1
4
,y (19) 2
3
8
,y+
3
4
, ¯y (20) 4
+
(
1
4
,0,0) x,−
1
4
,
1
2
(21) 4
+
(0,
1
4
,0)
1
2
,y,−
1
4
(22) 2(
1
2
,0,
1
2
) x−
1
4
,
1
8
,x (23) 4
−
(0,
3
4
,0) 0,y,
1
4
(24) 2 ¯x+
3
4
,
3
8
,x
For (
1
2
,
1
2
,
1
2
)+ set
(1) t(
1
2
,
1
2
,
1
2
) (2) 2 0,
1
4
,z (3) 2
1
4
,y,0(4)2x,0,
1
4
(5) 3
+
(
1
2
,
1
2
,
1
2
) x,x,x (6) 3
+
(
1
6
,−
1
6
,
1
6
) ¯x−
1
6
,x+
1
3
, ¯x (7) 3
+
(−
1
6
,
1
6
,
1
6
) x+
1
6
, ¯x+
1
6
, ¯x (8) 3
+
(
1
6
,
1
6
,−
1
6
) ¯x+
1
3
, ¯x+
1
6
,x
(9) 3
−
(
1
2
,
1
2
,
1
2
) x,x,x (10) 3
−
(
1
6
,−
1
6
,−
1
6
) x+
1
6
, ¯x+
1
6
, ¯x (11) 3
−
(−
1
6
,−
1
6
,
1
6
) ¯x+
1
3
, ¯x+
1
6
,x (12) 3
−
(−
1
6
,
1
6
,−
1
6
) ¯x−
1
6
,x+
1
3
, ¯x
(13) 2(
1
2
,
1
2
,0) x,x+
1
4
,
3
8
(14) 2 x, ¯x+
1
4
,
1
8
(15) 4
−
(0,0,
1
4
)
3
4
,0,z (16) 4
+
(0,0,
3
4
)
1
4
,
1
2
,z
(17) 4
−
(
1
4
,0,0) x,
3
4
,0 (18) 2(0,
1
2
,
1
2
)
3
8
,y−
1
4
,y (19) 2
1
8
,y+
1
4
, ¯y (20) 4
+
(
3
4
,0,0) x,
1
4
,
1
2
(21) 4
+
(0,
3
4
,0)
1
2
,y,
1
4
(22) 2(
1
2
,0,
1
2
) x+
1
4
,
3
8
,x (23) 4
−
(0,
1
4
,0) 0,y,
3
4
(24) 2 ¯x+
1
4
,
1
8
,x
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); t(
1
2
,
1
2
,
1
2
); (2); (3); (5); (13)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates
(0,0, 0)+ (
1
2
,
1
2
,
1
2
)+
Reflection conditions
h,k,l permutable
General:
48 i 1(1)x, y,z (2) ¯x +
1
2
, ¯y, z +
1
2
(3) ¯x,y +
1
2
, ¯z +
1
2
(4) x +
1
2
, ¯y +
1
2
, ¯z
(5) z,x,y (6) z +
1
2
, ¯x +
1
2
, ¯y (7) ¯z +
1
2
, ¯x, y +
1
2
(8) ¯z,x +
1
2
, ¯y +
1
2
(9) y,z,x (10) ¯y,z +
1
2
, ¯x +
1
2
(11) y +
1
2
, ¯z +
1
2
, ¯x (12) ¯y+
1
2
, ¯z, x +
1
2
(13) y +
3
4
,x +
1
4
, ¯z +
1
4
(14) ¯y +
3
4
, ¯x +
3
4
, ¯z +
3
4
(15) y +
1
4
, ¯x +
1
4
,z +
3
4
(16) ¯y +
1
4
,x +
3
4
,z +
1
4
(17) x +
3
4
,z +
1
4
, ¯y +
1
4
(18) ¯x +
1
4
,z +
3
4
,y +
1
4
(19) ¯x +
3
4
, ¯z +
3
4
, ¯y +
3
4
(20) x +
1
4
, ¯z +
1
4
,y +
3
4
(21) z +
3
4
,y +
1
4
, ¯x +
1
4
(22) z +
1
4
, ¯y +
1
4
,x +
3
4
(23) ¯z +
1
4
,y +
3
4
,x +
1
4
(24) ¯z+
3
4
, ¯y +
3
4
, ¯x +
3
4
hkl : h + k + l = 2n
0kl : k + l = 2n
hhl : l = 2n
h00 : h = 4n
Special: as above, plus
24 h ..2
1
8
,y, ¯y +
1
4
3
8
, ¯y, ¯y +
3
4
7
8
,y +
1
2
,y +
1
4
5
8
, ¯y +
1
2
,y +
3
4
¯y+
1
4
,
1
8
,y ¯y +
3
4
,
3
8
, ¯yy+
1
4
,
7
8
,y +
1
2
y +
3
4
,
5
8
, ¯y +
1
2
y, ¯y +
1
4
,
1
8
¯y, ¯y +
3
4
,
3
8
y +
1
2
,y +
1
4
,
7
8
¯y +
1
2
,y +
3
4
,
5
8
no extra conditions
24 g ..2
1
8
,y,y +
1
4
3
8
, ¯y, y +
3
4
7
8
,y +
1
2
, ¯y +
1
4
5
8
, ¯y +
1
2
, ¯y +
3
4
y +
1
4
,
1
8
,yy+
3
4
,
3
8
, ¯y ¯y +
1
4
,
7
8
,y +
1
2
¯y +
3
4
,
5
8
, ¯y +
1
2
y, y +
1
4
,
1
8
¯y,y +
3
4
,
3
8
y +
1
2
, ¯y +
1
4
,
7
8
¯y +
1
2
, ¯y +
3
4
,
5
8
no extra conditions
24 f 2 .. x, 0,
1
4
¯x +
1
2
,0,
3
4
1
4
,x,0
3
4
, ¯x +
1
2
,00,
1
4
,x 0,
3
4
, ¯x +
1
2
3
4
,x +
1
4
,0
3
4
, ¯x +
3
4
,
1
2
x +
3
4
,
1
2
,
1
4
¯x +
1
4
,0,
1
4
0,
1
4
, ¯x +
1
4
1
2
,
1
4
,x +
3
4
hkl : h = 2n + 1
or h = 4n
hhl : h = 2n + 1
or h + k + l = 4n
16 e . 3 . x, x,x ¯x +
1
2
, ¯x,x +
1
2
¯x,x +
1
2
, ¯x +
1
2
x +
1
2
, ¯x +
1
2
, ¯x
x +
3
4
,x +
1
4
, ¯x +
1
4
¯x +
3
4
, ¯x +
3
4
, ¯x +
3
4
x +
1
4
, ¯x +
1
4
,x +
3
4
¯x+
1
4
,x +
3
4
,x +
1
4
0kl : k = 2n + 1
or k + l = 4n
12 d 2 . 22
5
8
,0,
1
4
7
8
,0,
3
4
1
4
,
5
8
,0
3
4
,
7
8
,00,
1
4
,
5
8
0,
3
4
,
7
8
12 c 2 . 22
1
8
,0,
1
4
3
8
,0,
3
4
1
4
,
1
8
,0
3
4
,
3
8
,00,
1
4
,
1
8
0,
3
4
,
3
8
hkl : h,k = 2n, h + k + l = 4n
or h, k = 2n + 1, l = 4n + 2
or h = 8n, k = 8n + 4and
h + k + l = 4n + 2
or h, k = 8n + 1, l = 4n
or h = 8n + 1and
k = 8n − 1, l = 4n
or h, k = 8n + 3, l = 4
n
or h = 8n + 3and
k = 8n − 3, l = 4 n
8 b . 32
7
8
,
7
8
,
7
8
5
8
,
1
8
,
3
8
1
8
,
3
8
,
5
8
3
8
,
5
8
,
1
8
8 a . 32
1
8
,
1
8
,
1
8
3
8
,
7
8
,
5
8
7
8
,
5
8
,
3
8
5
8
,
3
8
,
7
8
hkl : h = 2n + 1
or h, k, l = 4n + 2
or h, k, l = 4n
653
